Mean Value Theorem with Example
TLDRIn this video, Mario from Mario's Math Tutoring explains the Mean Value Theorem (MVT) in calculus. He breaks down the theorem's conditions: a function must be continuous on a closed interval [a, b] and differentiable on the open interval (a, b). The MVT states that there's at least one point, c, in (a, b) where the instantaneous rate of change (slope of the tangent line) equals the average rate of change between the endpoints. Mario uses the example of the function f(x) = x^3 + 4x over the interval [-1, 1] to illustrate this concept and solves for the point where the slopes match.
Takeaways
- 📚 The video is about understanding and applying the Mean Value Theorem.
- 📝 The Mean Value Theorem states that if a function is continuous on a closed interval [a, b] and differentiable on the open interval (a, b), there exists a number c in (a, b) such that f'(c) equals the average rate of change (f(b) - f(a)) / (b - a).
- 🔍 Continuity means the graph of the function has no breaks, and differentiability means the graph is smooth without corners or cusps.
- 📈 The theorem guarantees at least one point (c) where the tangent line's slope equals the slope of the secant line connecting the endpoints (a, f(a)) and (b, f(b)).
- 📊 An example function x^3 + 4x is analyzed over the interval [-1, 1] to illustrate the theorem.
- ✅ The function x^3 + 4x is continuous and differentiable because it is a polynomial.
- 🔢 Calculations show f(-1) = -5 and f(1) = 5, so the slope of the secant line between these points is (5 - (-5)) / (1 - (-1)) = 5.
- ✏️ The derivative of the function is f'(x) = 3x^2 + 4, and solving 3x^2 + 4 = 5 gives x = ±√(1/3) = ±√3/3.
- 📐 Graphing the function and the secant line demonstrates that the points where x = ±√3/3 have tangent lines with the same slope as the secant line.
- 📺 The video encourages viewers to subscribe for more math tutorials and resources.
Q & A
What is the Mean Value Theorem?
-The Mean Value Theorem states that if a function F is continuous on the closed interval [a, b] and differentiable on the open interval (a, b), there exists a number c in the interval (a, b) such that F'(c) equals (F(b) - F(a)) / (b - a).
What are the conditions for the Mean Value Theorem to apply?
-The function must be continuous on the closed interval [a, b] and differentiable on the open interval (a, b).
What does it mean for a function to be continuous?
-A function is continuous if there are no breaks in the graph, meaning you can draw the graph without lifting your pen or pencil.
What does it mean for a function to be differentiable?
-A function is differentiable if you can find the derivative at any point along the curve, indicating the curve is smooth without any corners or cusps.
What is the geometric interpretation of the Mean Value Theorem?
-There exists at least one point c in the interval (a, b) where the slope of the tangent line to the curve at c is equal to the average rate of change between the endpoints a and b.
In the example provided, what function is being analyzed?
-The function being analyzed is F(x) = x^3 + 4x.
How do you verify if the function F(x) = x^3 + 4x is continuous and differentiable on the interval [-1, 1]?
-Since F(x) = x^3 + 4x is a polynomial, it is continuous and differentiable on all real numbers, including the interval [-1, 1].
What are the values of F(-1) and F(1) for the function F(x) = x^3 + 4x?
-F(-1) = -1^3 + 4(-1) = -1 - 4 = -5, and F(1) = 1^3 + 4(1) = 1 + 4 = 5.
How do you calculate the average rate of change for the function on the interval [-1, 1]?
-The average rate of change is calculated as (F(1) - F(-1)) / (1 - (-1)) = (5 - (-5)) / (1 + 1) = 10 / 2 = 5.
How do you find the values of c where the instantaneous rate of change equals the average rate of change?
-First, find the derivative of the function: F'(x) = 3x^2 + 4. Set the derivative equal to the average rate of change (5): 3x^2 + 4 = 5. Solve for x to get x = ±√(1/3) or ±√3/3.
What do the values ±√3/3 represent in the context of the Mean Value Theorem?
-These values represent the points c in the interval (-1, 1) where the slope of the tangent line to the curve is equal to the average rate of change between the endpoints.
Outlines
📘 Introduction to the Mean Value Theorem
In this video, Mario introduces the Mean Value Theorem (MVT), explaining its importance in mathematics. He states that if a function \(f\) is continuous on a closed interval \([a, b]\) and differentiable on the open interval \((a, b)\), there exists a number \(c\) in \((a, b)\) such that the derivative at \(c\) equals the average rate of change of the function over \([a, b]\). Mario emphasizes understanding the concepts of continuity and differentiability before breaking down the theorem with a graphical explanation.
🔍 Understanding Continuity and Differentiability
Mario further clarifies the prerequisites for the MVT: continuity means the graph has no breaks, and differentiability means the graph is smooth without corners. He illustrates these concepts using a graph where a continuous function between points \(a\) and \(b\) is shown. The goal is to find at least one point \(c\) where the slope of the tangent equals the average rate of change between \(a\) and \(b\). This explanation is visually supported with multiple tangent lines to reinforce understanding.
📈 Example of the Mean Value Theorem
Mario presents an example using the function \(f(x) = x^3 + 4x\) over the interval \([-1, 1]\). He verifies the function's continuity and differentiability and computes the function values at the endpoints. Then, he calculates the average rate of change and sets it equal to the derivative \(f'(x)\). Solving for \(x\), he finds the points \(x = \pm \sqrt{1/3}\), demonstrating the MVT's application. He concludes by graphing the function to show the points where the instantaneous rate of change matches the average rate of change.
🧩 Graphical Visualization and Conclusion
Mario wraps up by graphically representing the function and the key points \((-1, -5)\) and \((1, 5)\). He illustrates how the tangent lines at \(x = \pm \sqrt{1/3}\) have slopes equal to the average rate of change. This visual aid reinforces the MVT's concept, making it easier to grasp. Mario encourages viewers to subscribe, check out previous videos, and look forward to more math content aimed at improving understanding and reducing stress.
Mindmap
Keywords
💡Mean Value Theorem
💡Continuous
💡Differentiable
💡Interval
💡Derivative
💡Average Rate of Change
💡Tangent Line
💡Polynomial
💡Instantaneous Rate of Change
💡Graph
Highlights
Introduction to the Mean Value Theorem and its importance in understanding calculus.
Explanation of the conditions for the Mean Value Theorem: continuity on a closed interval and differentiability on an open interval.
Clarification that a continuous function has no breaks in its graph, ensuring a smooth curve.
Differentiability implies the ability to find a derivative at any point on the curve, indicating a smooth function.
The theorem guarantees the existence of at least one point where the instantaneous rate of change equals the average rate of change.
Graphical representation of the Mean Value Theorem, illustrating the concept with a sample graph.
Identification of the average rate of change formula: (y2 - y1) / (x2 - x1).
Example of applying the Mean Value Theorem to a polynomial function f(x) = x^3 + 4x on the interval from -1 to 1.
Verification of the function's continuity and differentiability, confirming the applicability of the Mean Value Theorem.
Calculation of the function's values at the endpoints, establishing the context for finding the average rate of change.
Derivation of the average rate of change for the given interval, resulting in a slope of 5.
Differentiation of the function to find the derivative, which is necessary to apply the Mean Value Theorem.
Setting the derivative equal to the average rate of change to solve for the critical points.
Solution of the equation to find the x-values where the instantaneous rate of change equals the average rate of change.
Graphical demonstration of the points on the graph where the instantaneous rate of change matches the average rate of change.
Conclusion summarizing the application of the Mean Value Theorem and its significance in understanding calculus concepts.
Encouragement for viewers to subscribe for more educational content and a promise of future assistance.
Transcripts
Browse More Related Video
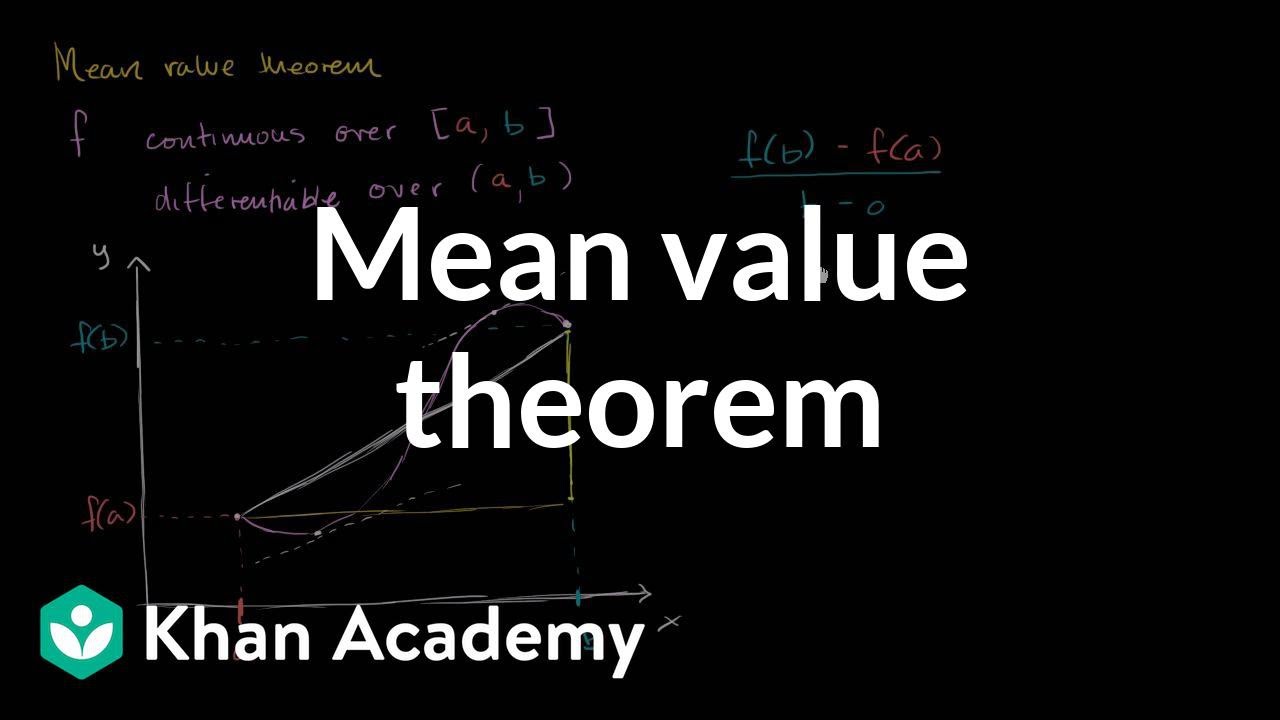
Mean value theorem | Existence theorems | AP Calculus AB | Khan Academy

Calculus 1 Lecture 3.2: A BRIEF Discussion of Rolle's Theorem and Mean-Value Theorem.
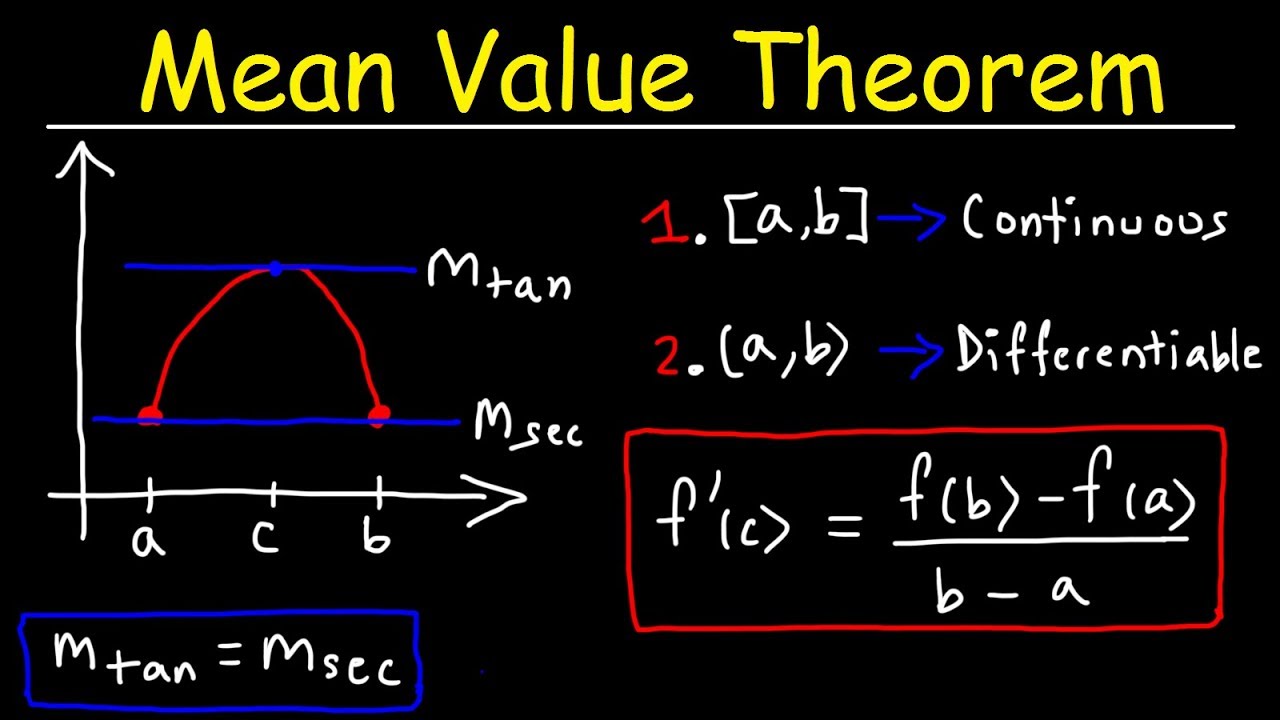
Mean Value Theorem
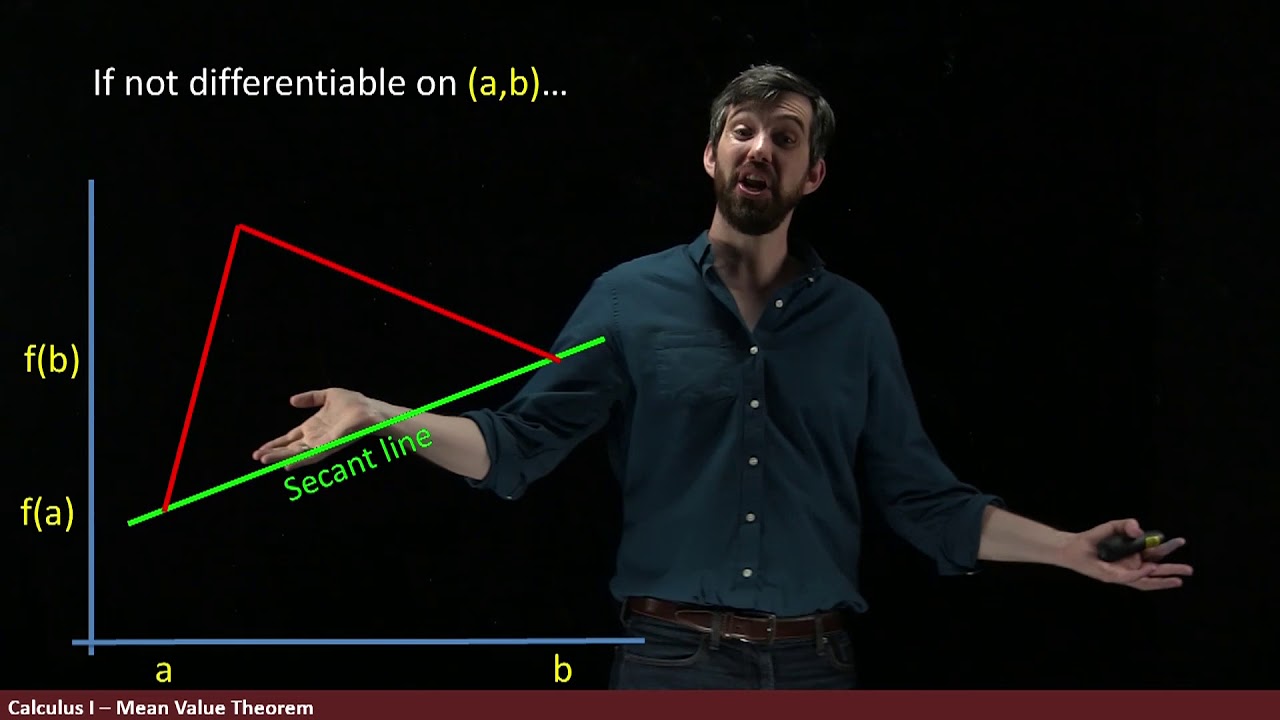
The MEAN Value Theorem is Actually Very Nice

AP Calculus Review Three Theorems You Must Know (EVT, IVT, MVT)

Mean Value Theorem and Rolle's Theorem
5.0 / 5 (0 votes)
Thanks for rating: