Mean value theorem for integrals | AP Calculus AB | Khan Academy

Khan Academy
16 Jul 201409:07
EducationalLearning
32 Likes 10 Comments

TLDRThe video script delves into the Mean Value Theorem, highlighting its significance in connecting differential calculus with the concept of average value via definite integrals. It explains that for a continuous function on a closed interval, there exists a point where the derivative equals the average rate of change over that interval. This concept is further extended to the Mean Value Theorem for Integrals, demonstrating that the average value of a continuous function on an interval can be found by evaluating the function at a specific point within that interval. The explanation is enriched with visual analogies and a clear mathematical connection between derivatives and integrals.

Takeaways
  • πŸ“š The Mean Value Theorem connects concepts from differential calculus to those in integral calculus.
  • πŸ” For a continuous function f on a closed interval [a, b], there exists a c such that the derivative at c equals the average rate of change over the interval.
  • 🌟 The average rate of change is calculated as the change in function value (Ξ”y) divided by the change in x (Ξ”x), i.e., (f(b) - f(a)) / (b - a).
  • πŸ“ˆ The slope between endpoints (a and b) is analogous to the average value of the function over the interval.
  • πŸ€” The Mean Value Theorem implies there is at least one point c in the interval where the slope of the tangent line equals the average slope.
  • πŸ”— The average value of a function can be related to the definite integral of the function over the interval, with the formula 1/(b-a) * ∫[a, b] f(x) dx.
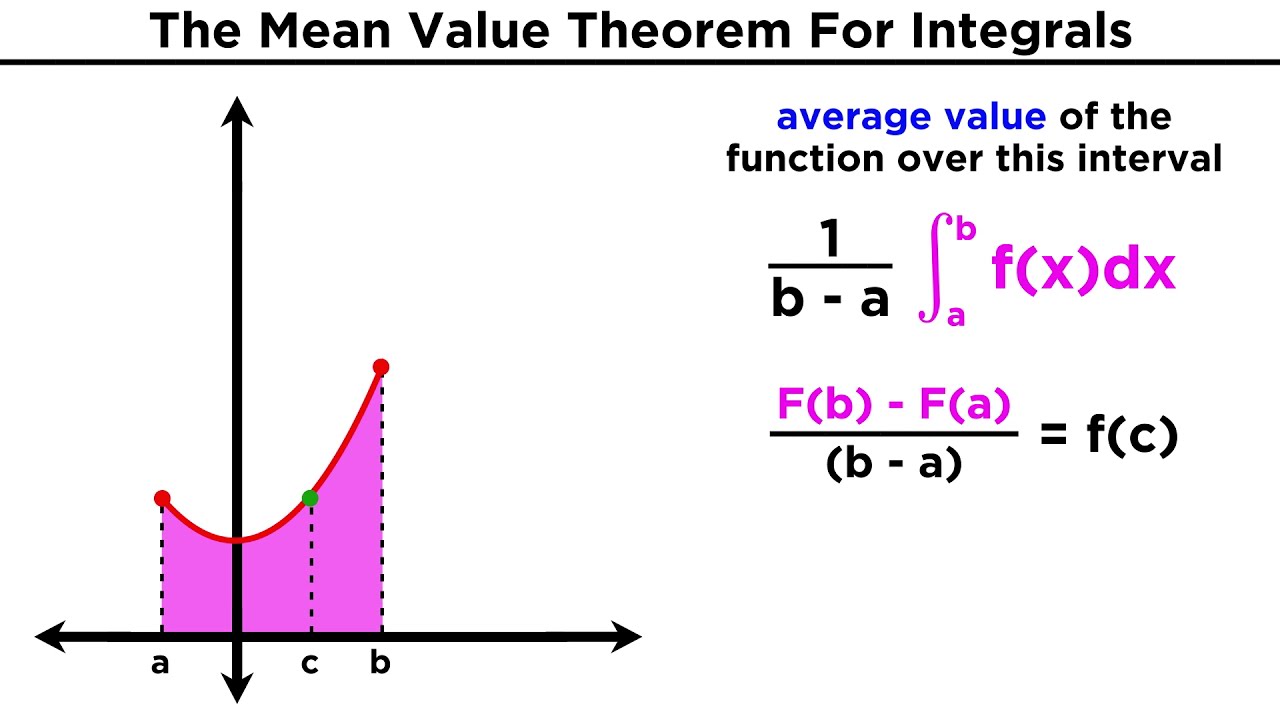
  • 🌐 The Mean Value Theorem for Integrals states that if g(x) is continuous on [a, b], there exists a c in the interval where g(c) equals the average value of g(x).
  • πŸ“Š The function g(x) can be thought of as f'(x), the derivative of f(x), to connect the concepts of derivatives and integrals.
  • 🧠 The Mean Value Theorem for Integrals is another form of the theorem, focusing on the average value of a function rather than the slope of a tangent line.
  • πŸ”„ The theorem shows a deep connection between differential calculus (slopes and derivatives) and integral calculus (average values and integrals).
  • πŸ“ The Mean Value Theorem is a fundamental concept that helps bridge the understanding of local behavior of functions (differentials) with their global properties (integrals).
Q & A

  • What is the Mean Value Theorem in the context of differential calculus?

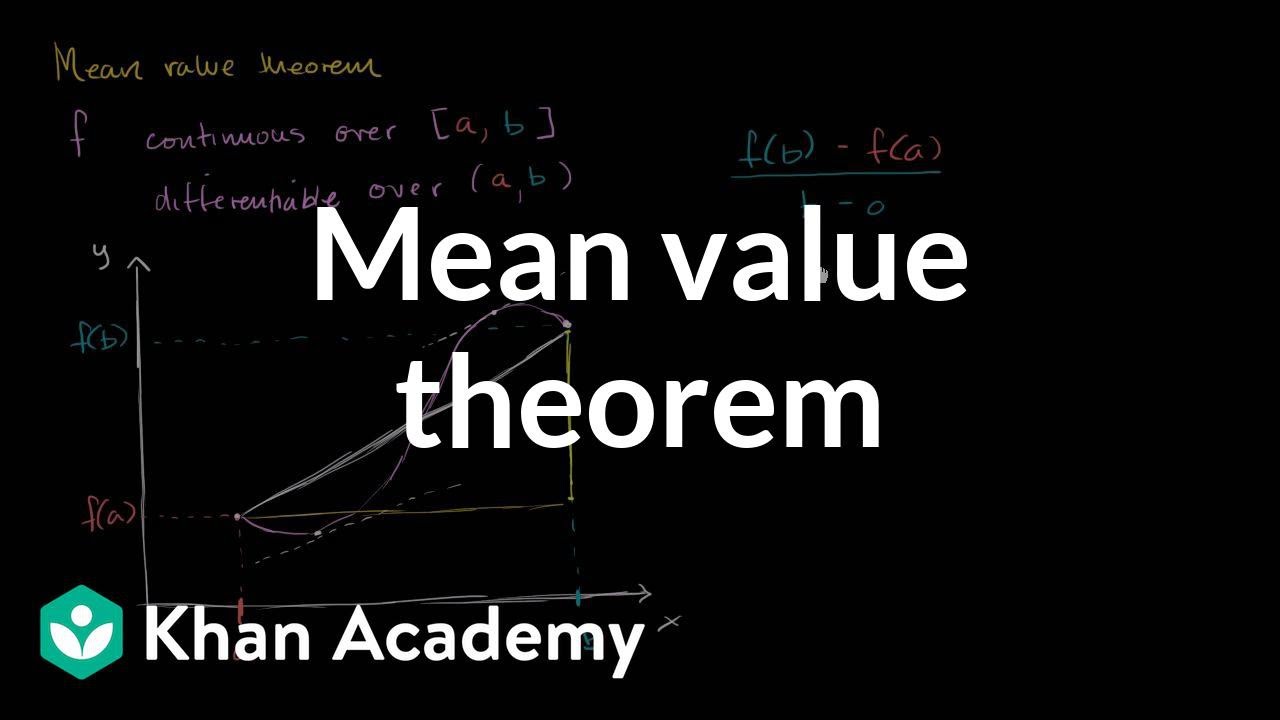
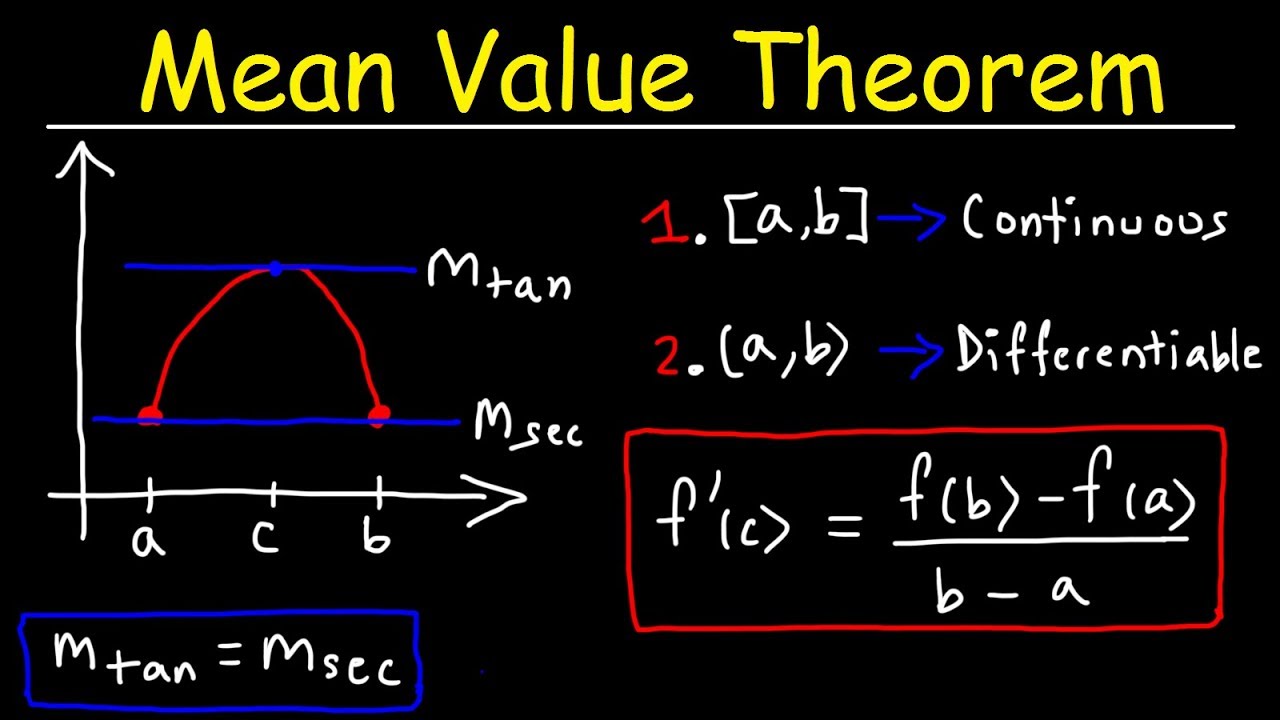
    -The Mean Value Theorem states that for a function f that is continuous on a closed interval [a, b] and differentiable on the open interval (a, b), there exists a number c in the interval (a, b) such that the derivative of f at c is equal to the average rate of change of f over the interval [a, b].

  • What does it mean for a function to be continuous on a closed interval?

    -A function is said to be continuous on a closed interval if it is defined and its graph contains no breaks or jumps at any point within the interval, including the endpoints.

  • How is the average rate of change of a function calculated?

    -The average rate of change of a function over an interval [a, b] is calculated as the change in the function's values (f(b) - f(a)) divided by the change in the x-values (b - a).

  • What is the significance of the point c in the Mean Value Theorem?

    -The point c in the Mean Value Theorem is significant because it is the value within the interval (a, b) where the derivative of the function, representing the slope of the tangent line, is equal to the average rate of change of the function over the interval.

  • How does the Mean Value Theorem relate to the concept of definite integrals?

    -The Mean Value Theorem can be connected to definite integrals by considering the average value of a function over an interval. The theorem implies that there exists a point c in the interval where the function's value at c equals the average value, which can be calculated using the definite integral of the function's derivative over the interval.

  • What is the Mean Value Theorem for integrals?

    -The Mean Value Theorem for integrals states that if a function g is continuous on a closed interval [a, b], then there exists a number c in the interval such that g(c) is equal to the average value of the function over the interval, which is the integral of g over the interval divided by the length of the interval (b - a).

  • How can the Mean Value Theorem for integrals be expressed in terms of a function's derivative?

    -The Mean Value Theorem for integrals can be expressed by considering g(x) as the derivative of the original function f(x), so that g(c) = (1 / (b - a)) * ∫[a, b] g(x) dx, which is equivalent to f'(c) = (1 / (b - a)) * ∫[a, b] f'(x) dx.

  • What is the connection between the average value of a function and its definite integral?

    -The average value of a function over an interval [a, b] is given by the definite integral of the function over the interval divided by the length of the interval (b - a). This connection allows us to find the average value by integrating the function's derivative.

  • How does the concept of the average value of a function differ from the slope of its tangent line?

    -The average value of a function over an interval is a single value that represents the overall average level of the function on that interval, while the slope of the tangent line at a point represents the instantaneous rate of change at that specific point. The Mean Value Theorem connects these two concepts by showing that there exists a point where the function's value equals the average value and the slope of the tangent line equals the average rate of change.

  • What is the role of anti-derivatives in the Mean Value Theorem for integrals?

    -In the Mean Value Theorem for integrals, the anti-derivative of the function g(x), which is the same as the derivative of the original function f(x), is used to evaluate the function at the endpoints of the interval and find the average value. The anti-derivative is essential for calculating the definite integral and connecting the concepts of differentiation and integration.

  • How does the Mean Value Theorem for integrals demonstrate the close relationship between differentiation and integration?

    -The Mean Value Theorem for integrals shows that the average value of a function over an interval can be found by integrating its derivative and evaluating the resulting anti-derivative at the interval's endpoints. This highlights the connection between differentiation, which deals with rates of change and slopes, and integration, which deals with summing areas under curves and finding average values.

Outlines
00:00
πŸ“š Introduction to Mean Value Theorem and its Connection to Definite Integrals

This paragraph introduces the Mean Value Theorem, emphasizing its significance in understanding the relationship between differential calculus and definite integrals. It explains that for a continuous function f on a closed interval [a, b], there exists a point c within the interval such that the derivative of f at c equals the average rate of change of f over the interval. This concept is visualized by comparing the slope of the tangent line at point c to the slope of the line connecting the endpoints a and b. The paragraph also hints at a connection between the Mean Value Theorem and the average value of a function, suggesting that the derivative at a point c is equivalent to the average value of the function's derivative over the interval, which can be expressed as the definite integral of f'(x) from a to b.

05:03
πŸ” Deepening Understanding: Mean Value Theorem for Integrals

The second paragraph delves deeper into the Mean Value Theorem for Integrals, which is an extension of the original Mean Value Theorem. It posits that for a continuous function g on a closed interval [a, b], there exists a point c within the interval where the value of g at c is equal to the average value of g over the interval. This is mathematically expressed as g(c) = (1 / (b - a)) * the definite integral from a to b of g(x) dx. The explanation draws parallels between the concepts of the average value of a function and the Mean Value Theorem for Integrals, highlighting that they are essentially the same idea but expressed with different notations. The paragraph emphasizes the shift in perspective from the differential calculus viewpoint, focused on slopes and tangent lines, to the integral calculus viewpoint, centered on the average value of a function.

Mindmap
Keywords
πŸ’‘Mean Value Theorem
The Mean Value Theorem is a fundamental concept in calculus that establishes a connection between the average rate of change of a function over an interval and the instantaneous rate of change (the derivative) at some point within that interval. In the video, it is explained that if a function is continuous on a closed interval and differentiable on the open interval, there exists at least one point 'c' where the derivative of the function equals the average rate of change over that interval.
πŸ’‘Differential Calculus
Differential calculus is a branch of mathematics that deals with the study of rates of change and slopes of curves. It is one of the two main branches of calculus, the other being integral calculus. In the context of the video, differential calculus is used to understand the concept of derivatives and how they relate to the Mean Value Theorem, particularly in terms of the slope of the tangent line to a curve at a given point.
πŸ’‘Definite Integrals
Definite integrals are a mathematical concept used to calculate the area under a curve over a specified interval. They are a fundamental part of integral calculus and are used to find quantities such as the accumulated change in a quantity over time. In the video, the connection between definite integrals and the Mean Value Theorem is explored, showing how the average value of a function over an interval can be found using integration.
πŸ’‘Continuous Function
A continuous function is one where there are no breaks, jumps, or holes in its graph over a certain interval. This property is important for the Mean Value Theorem to hold, as it ensures that the function behaves well over the interval of interest and allows for the existence of a point 'c' where the derivative equals the average rate of change.
πŸ’‘Differentiable
A function is said to be differentiable at a point if it has a derivative at that point, meaning the rate of change or slope of the function is well-defined. Differentiability is a key assumption for many theorems in calculus, including the Mean Value Theorem, as it allows us to analyze the local behavior of functions and their tangents.
πŸ’‘Average Rate of Change
The average rate of change of a function over an interval is the ratio of the change in the function's output (y-value) to the change in its input (x-value) over that interval. It gives a general idea of how a function is changing as its input varies. In the video, the average rate of change is used to illustrate the connection between the Mean Value Theorem and the concept of the average value of a function.
πŸ’‘Tangent Line
A tangent line is a line that touches a curve at a single point and has the same slope as the curve at that point. In the context of the video, the tangent line is used to visualize the derivative of a function at a particular point 'c', which is the rate of change or slope at that point.
πŸ’‘Anti-Derivative
An anti-derivative, also known as an integral, is a function whose derivative is the given function. In the process of integration, one finds an anti-derivative to evaluate definite integrals and understand the accumulated change under the curve. The video connects the concept of anti-derivatives to the Mean Value Theorem for integrals.
πŸ’‘Average Value of a Function
The average value of a function over an interval is the integral of the function over that interval divided by the length of the interval. It represents the mean level of the function's output over the given range. The video discusses how the Mean Value Theorem for integrals shows that there exists a point 'c' where the function takes on the average value.
πŸ’‘Integration
Integration is the process of finding an anti-derivative or the area under a curve. It is one of the two main branches of calculus, complementary to differentiation. In the video, integration is used to connect the Mean Value Theorem with the average value of a function, demonstrating that there is a point 'c' where the function equals the average value over an interval.
Highlights

The Mean Value Theorem connects differential calculus and definite integrals, providing a comprehensive understanding of functions' behavior.

A function f must be continuous on a closed interval [a, b] and differentiable on the open interval (a, b) to apply the Mean Value Theorem.

The theorem states that there exists a value c in the interval (a, b) where the derivative of f at c equals the average rate of change of f over the interval.

The average rate of change is calculated as the change in f(b) - f(a) divided by the change in x from a to b.

The Mean Value Theorem can be visualized by considering the slope of the tangent line at a point c equivalent to the average slope between the endpoints a and b.

The Mean Value Theorem for integrals, or integration, extends the concept to average values and definite integrals.

A function g must be continuous on a closed interval [a, b] for the Mean Value Theorem for integrals to hold.

There exists a point c in the interval [a, b] where g(c) equals the average value of the function g over that interval.

The average value of a function is defined as the definite integral of the function from a to b divided by the interval length (b - a).

The Mean Value Theorem for integrals can be seen as a reformulation of the theorem from differential calculus, connecting the concepts of derivatives and integrals.

The theorem's implications are vast, impacting both theoretical understanding and practical applications in calculus.

Understanding the Mean Value Theorem is crucial for deeper insights into the properties of functions and their graphical representations.

The Mean Value Theorem bridges the gap between differential and integral calculus, enhancing the overall calculus knowledge base.

The theorem provides a powerful tool for analyzing the behavior of functions, particularly in relation to their slopes and average values.

The Mean Value Theorem for integrals reinforces the connection between the rate of change and the area under the curve of a function.

By applying the theorem, one can find a specific point where the function's value equals the average value, offering a unique perspective on function analysis.

The Mean Value Theorem is a fundamental concept that underpins many advanced topics in calculus and mathematical analysis.

Transcripts

Browse More Related Video

Calculus 1 Lecture 3.2: A BRIEF Discussion of Rolle's Theorem and Mean-Value Theorem.

The Mean Value Theorem For Integrals: Average Value of a Function

AP Calculus Review Three Theorems You Must Know (EVT, IVT, MVT)

2011 Calculus AB free response #4d | AP Calculus AB | Khan Academy

Mean value theorem | Existence theorems | AP Calculus AB | Khan Academy

Mean Value Theorem

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
MathematicsCalculusMean Value TheoremDifferential EquationsIntegralsSlope AnalysisAverage RateTangent LinesContinuous FunctionsEducational Content