INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 14

TIKLE'S ACADEMY OF MATHS
5 Oct 202009:37
EducationalLearning
32 Likes 10 Comments

TLDRThe video script is an educational lecture on integral calculus, focusing on solving a specific problem involving the function of x^2 - 1/x^2. The lecture begins by welcoming viewers to the YouTube channel and encourages them to review previous videos for context. The problem is solved step by step, converting the given function into its standard form and applying integral calculus concepts, including limits and the fundamental theorem of calculus. The solution process is explained in detail, emphasizing the importance of understanding the standard form of functions and the application of calculus rules. The video concludes with an encouragement for viewers to practice and a reminder to subscribe for more educational content.

Takeaways
  • 📚 The video is a lecture on Integral Calculus, focusing on solving a specific problem involving the function of x and its exponent.
  • 🔢 The problem given in the video is to evaluate the integral of a function, which is written in the question and involves logarithms and exponents.
  • 📝 The讲师 converts the given integral into its standard form, which is essential for solving the problem efficiently.
  • 🎯 The讲师 emphasizes the importance of understanding the definition of the function, which is crucial for the problem-solving process.
  • 🤔 The video demonstrates the process of converting the right-hand side of the integral into its standard form, which simplifies the problem.
  • 🔄 The讲师 uses properties of integration to change the limits of integration, which is a key step in solving the problem.
  • 📈 The讲师 also discusses how to deal with the limits of integration, particularly when they involve infinity, by using the properties of logarithms.
  • 🌟 The video concludes with the讲师 showing the final result of the integral, which matches the expected form based on the function's definition.
  • 👍 The讲师 encourages viewers to practice the problems covered in previous videos to gain a better understanding of Integral Calculus.
  • 📌 The video description contains a playlist of previous videos for viewers to catch up on any missed content.
  • 💡 The讲师 provides a clear and detailed explanation, making complex concepts in Integral Calculus more accessible to the audience.
Q & A

  • What is the main topic of the video?

    -The main topic of the video is solving an integral calculus problem involving a function of the form ∫(x^n * e^(-x^2))dx.

  • What is the standard form of the function given in the problem?

    -The standard form of the function is x^n * e^(-x^2), where n is the power to which x is raised.

  • How does the video approach the problem-solving process?

    -The video approaches the problem-solving process by first converting the given integral into its standard form, then applying the properties of integration to simplify and solve the problem.

  • What is the significance of the limits of integration in this problem?

    -The limits of integration are crucial as they define the interval over which the function is to be integrated. In the problem, the limits are from 0 to infinity, which affects the evaluation of the integral.

  • How does the video handle the exponential function in the problem?

    -The video handles the exponential function by keeping it as part of the integrand and then applying the appropriate integration techniques that are compatible with exponential functions.

  • What is the role of the power rule in this integral?

    -The power rule is used to determine the form of the integral after conversion. It helps in simplifying the expression by reducing the power of x in the integrand when integrated.

  • What is the significance of the 'u-substitution' method mentioned in the video?

    -The 'u-substitution' method is a technique used in integration to simplify the integrand and make it easier to integrate. It involves replacing the variable of integration with a new variable (u) that is functionally related to the original variable.

  • How does the video address the concept of limits at infinity?

    -The video addresses the concept of limits at infinity by setting the upper limit of integration to infinity and discussing how this affects the integral. It also mentions the lower limit being 0 and how this contributes to the evaluation of the integral.

  • What is the final result of the integral as presented in the video?

    -The final result of the integral is not explicitly stated in the transcript, but the video concludes with the integral being converted into a form that allows for the application of known integration techniques to find the result.

  • What advice does the video give for further study and practice?

    -The video advises viewers to study the problems covered in the video and to practice more integral calculus problems for better understanding and proficiency. It also encourages watching more videos in the series for additional practice problems.

  • How can viewers access more related content?

    -Viewers can access more related content by visiting the video's description, where they will find a playlist of previous videos on similar topics. They are also encouraged to subscribe to the channel for more educational content.

Outlines
00:00
📚 Introduction to Integral Calculus - Lecture 14

The paragraph introduces the viewer to a new video on Integral Calculus, specifically focusing on the unit of Integral Calculus. The speaker welcomes the audience to their YouTube channel and mentions that today's video will involve solving a problem related to a specific function. The speaker also encourages viewers to review previous videos for context and understanding of the topic. The problem to be solved is presented, involving a function of 'x' and its integral squared, with certain power and minus one over 'x'. The speaker explains the process of converting the given problem into a standard form of the integral function, which will then be solved.

05:00
🔢 Problem Solving and Limit Conversion in Integral Calculus

In this paragraph, the speaker continues to delve into the problem-solving process of Integral Calculus. The focus is on converting the given integral function into its standard form and solving it. The speaker explains the steps of replacing certain elements in the integral, such as 'x' with 't', and the process of limit conversion. The concept of limits, both upper and lower, is discussed in detail, along with the use of integration properties. The speaker also touches upon the concept of infinite limits and how to handle them in the context of the integral. The problem is then solved by applying the integral function and comparing it with the given function to ensure a match. The speaker concludes by summarizing the integral function in its standard form and encourages further practice and study of additional problems.

Mindmap
Keywords
💡Integral Calculus
Integral Calculus is a branch of mathematics that deals with the study of integrals, which are used to find the area under a curve or the volume of a solid. In the video, the speaker is solving a problem related to Integral Calculus, specifically focusing on the unit of the function and its application to solve real-world problems.
💡Function
In mathematics, a function is a relation that maps each element from one set (the domain) to exactly one element of another set (the range). Functions are central to the video's content as they represent the mathematical relationships used to describe phenomena and solve problems in Integral Calculus.
💡Limits
Limits in calculus refer to the value that a function or sequence approaches as the input (or index) approaches some value. They are fundamental to understanding continuity, differentiability, and the behavior of functions at points of interest. In the context of the video, limits are crucial for evaluating integrals.
💡Standard Form
A standard form is a set of rules or properties that define the structure of something, such as a mathematical expression or a function. In the video, the standard form of a function is important for applying the principles of Integral Calculus to solve problems.
💡Differential
In calculus, a differential represents a small change in a function's value. It is the basic building block for understanding how functions change as their inputs change. Differentials are used to approximate functions and find derivatives and integrals.
💡Exponential Function
An exponential function is a function of the form f(x) = a * b^x, where a and b are constants, and b > 0. These functions are important in many areas of mathematics and science, as they model growth and decay processes. In the video, exponential functions are part of the integral being solved.
💡Logarithm
A logarithm is the inverse operation to exponentiation, used to find the power to which a number (the base) must be raised to produce a given value. Logarithmic functions are essential in solving equations and understanding the behavior of certain types of functions. In the video, logarithms appear as part of the function being integrated.
💡Power Rule
The power rule in calculus is a fundamental rule that allows the differentiation of functions raised to a power. It states that the derivative of x^n, where n is a constant, is nx^(n-1). This rule is essential for differentiating and integrating polynomial functions.
💡Substitution
Substitution is a technique used in calculus to evaluate integrals by replacing the variable of integration with another variable or expression. This method simplifies the integral, making it easier to solve complex problems.
💡Integration by Parts
Integration by parts is a technique used in calculus to evaluate integrals by reversing the process of differentiation. It involves integrating one part of the integrand and differentiating the other, allowing for the simplification of complex integrals.
💡Antidifferentiation
Antidifferentiation, also known as indefinite integration, is the process of finding a function whose derivative is a given function. It is the inverse operation of differentiation and is fundamental to solving integrals in calculus.
Highlights

Welcome to the YouTube channel for tips on the Academy of Mathematics.

Today's video will cover an integral calculus unit and solve a problem involving a function.

The problem to be solved is related to the function of x to the power of n, specifically x^2 - 1, for n=2.

The solution process involves converting the given integral into its standard form.

The standard form of the function is defined and involves a report of -x * x^2 and -1 to the power of n.

The integral is converted into its standard form, which simplifies the problem-solving process.

The problem-solving approach includes shifting the limit to the left side and dealing with the exponent.

The solution involves understanding the properties of integration and applying them correctly.

The video provides a detailed explanation of the steps to solve the integral, making it easier for viewers to follow along.

The final solution is presented in a clear and concise manner, demonstrating the effectiveness of the method used.

The video encourages viewers to practice solving more problems to gain a better understanding of the concepts.

A playlist of previous videos is provided in the video description for viewers to catch up on any missed content.

The video concludes with an invitation to subscribe to the channel for more educational content.

The presenter's teaching style is engaging and informative, making complex mathematical concepts accessible.

The video emphasizes the importance of understanding the fundamentals of calculus for solving more complex problems.

The video provides practical applications of the concepts learned, helping viewers to see the relevance of the subject matter.

The presenter encourages interaction by inviting viewers to ask questions in the comments section.

The video concludes with a thank you note and a prompt for viewers to like and share the video with others who might find it useful.

Transcripts

Browse More Related Video

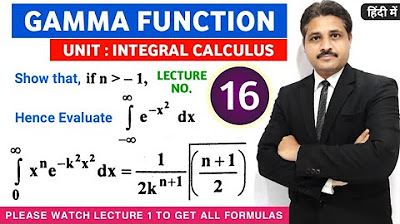
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 16

INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 13

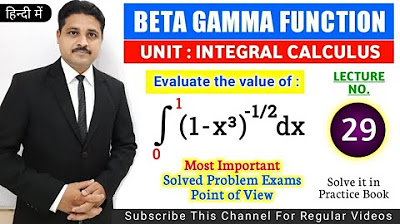
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 29 | BETA GAMMA FUNCTION SOLVED PROBLEM IN HINDI

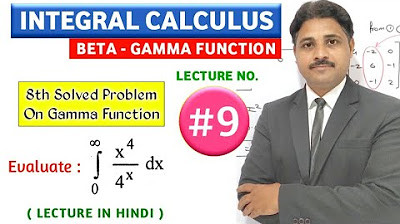
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 9

INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 28 | BETA GAMMA FUNCTION SOLVED PROBLEM IN HINDI

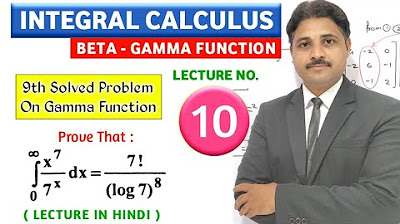
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 10

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
IntegralCalculusMathTutorialsFunctionTransformationProblemSolvingYouTubeEducationLectureSeriesComplexAnalysisMathematicsEducationalContentOnlineLearning