INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 13

TIKLE'S ACADEMY OF MATHS
25 Sept 202012:48
EducationalLearning
32 Likes 10 Comments

TLDRThe video script revolves around the topic of integral calculus, focusing on the concept of limits and functions. The speaker welcomes viewers to their YouTube channel and introduces the problem at hand, which involves solving an integral using the properties of functions. The script delves into the standard definition of functions, discusses the process of converting a particular integral into its standard form, and explores the concept of limits in detail. The speaker also touches upon the application of limits in solving problems and provides a step-by-step approach to tackle the given integral. The video aims to simplify complex mathematical concepts and encourages viewers to practice more problems for better understanding and mastery of integral calculus.

Takeaways
  • 📚 The video is a part of an integral calculus unit and focuses on knowledge and function topics.
  • 🔢 The problem-solving approach involves converting expressions into standard integral form and evaluating them.
  • 📈 The process includes understanding the definition of functions and converting particular integrals accordingly.
  • 🌐 The video introduces the concept of limits and how they are applied in integral calculus.
  • 🔄 The technique used for problem-solving is consistent with previously discussed methods, utilizing integration by parts and substitution.
  • 📊 The script discusses the importance of converting expressions to the standard form of integral calculus before solving.
  • 🎯 The video emphasizes the need to match the limit forms correctly when setting up integral expressions.
  • 🛠️ The method involves shifting certain terms and simplifying expressions to find the solution.
  • 📋 The script provides a detailed example of solving a complex integral problem step by step.
  • 📈 The video also touches on the concept of infinite limits and how they are handled in integral calculus.
  • 🎓 The content is educational and designed to help viewers understand and practice integral calculus problems.
Q & A

  • What is the main topic of the video?

    -The main topic of the video is integral calculus, specifically focusing on the concept of integrals and their applications in solving problems.

  • What is the first step in solving the given problem according to the video?

    -The first step in solving the given problem is to convert the particular integral into its standard form.

  • What does the standard form of an integral represent?

    -The standard form of an integral represents the integral of a function from a lower limit to an upper limit, often denoted as ∫ from a to b of function dx.

  • How does the video suggest handling limits that are not directly given in the problem?

    -The video suggests converting the problem into a form where the limits can be controlled and matched, and if necessary, shifting the limits to make them suitable for the standard form of the integral.

  • What is the significance of the 'minus one' in the integral's power in the video example?

    -The 'minus one' in the integral's power indicates that the integral is of the inverse function, which is a common concept in integral calculus when dealing with functions that have been exponentiated.

  • How does the video address the concept of 'extrema' in the context of the problem?

    -The video mentions 'extrema' in the context of finding the maximum or minimum values of the function within the given limits of integration.

  • What is the role of the 'reciprocal' function in the problem-solving process?

    -The reciprocal function is used to invert the function, which can simplify the integral and make it easier to solve, especially when dealing with functions that have been multiplied by their reciprocal.

  • How does the video handle the concept of 'infinite limits' in integrals?

    -The video explains that when dealing with infinite limits, the focus should be on converting the problem into a form that can be controlled and matched, and then applying the appropriate techniques to handle the infinite nature of the limits.

  • What is the importance of 'differentiation' in the context of this video?

    -Differentiation is important as it is the reverse process of integration and is used to find the derivative of a function, which can help in understanding the behavior of the function and solving related problems.

  • What is the final step in solving the problem as per the video?

    -The final step in solving the problem, as per the video, is to perform the substitution and evaluation of the integral, ensuring that all limits and forms are correctly converted and applied.

Outlines
00:00
📚 Introduction to Integral Calculus

The paragraph introduces the topic of Integral Calculus, welcoming viewers to the educational YouTube channel. It sets the stage for exploring the concepts of knowledge and function within the context of Integral Calculus. The speaker mentions that the video will focus on solving a specific problem involving integrals, and encourages viewers to refer to the description for a playlist of previous videos on related topics. The paragraph emphasizes the importance of understanding the standard form of functions and integrals, and how converting a particular integral into this form is crucial for solving the problem at hand.

05:02
🔢 Dealing with Limits and Functions

This paragraph delves into the intricacies of dealing with limits and functions within the Integral Calculus unit. It discusses the process of converting a given integral into its standard form, which involves understanding the definition of the function and its limit. The speaker uses a specific example to illustrate the conversion process and highlights the importance of matching the standard form to the given integral. The paragraph also touches on the concept of reciprocal functions and their role in Integral Calculus, as well as the impact of converting limits on the overall problem-solving process.

10:03
📈 Setting up Equations and Solving

The final paragraph focuses on setting up equations and solving them within the context of Integral Calculus. The speaker explains how to convert various elements of the problem into a standard form that can be solved using Integral Calculus techniques. This includes dealing with limits, converting expressions, and understanding the implications of different forms on the solution process. The paragraph concludes with a mention of the next video, where more problems will be solved to provide a comprehensive understanding of the topic.

Mindmap
Keywords
💡Integral Calculus
Integral calculus is a branch of mathematics that deals with the study of integrals, which are used to find areas under curves, volumes of solids, and other similar quantities. In the context of the video, integral calculus is the main subject being discussed, with a focus on understanding and solving problems related to it.
💡Functions
In mathematics, a function is a relation that pairs each element from a set (called the domain) to exactly one element of another set (known as the codomain). Functions are central to the video's theme as they are the building blocks for integral calculus, and understanding their properties is crucial for solving the problems presented in the script.
💡Limits
Limits in calculus refer to the value that a function approaches as the input (or sometimes output) gets arbitrarily close to a certain point. Limits are fundamental to understanding the behavior of functions, especially when dealing with continuity, derivatives, and integrals. In the video, limits are discussed as part of the process of converting functions into a standard form for easier integration.
💡Infinite
The term 'infinite' refers to something that is endless or limitless. In the context of the video, infinity is used to describe certain values or limits that the function approaches, such as when the input approaches positive or negative infinity. This concept is crucial in understanding the behavior of functions at extreme values.
💡Power Functions
Power functions are a family of functions that have the form f(x) = x^n, where n is a real number. These functions are significant in the video as they are a type of function that can be integrated using integral calculus. Understanding the properties of power functions is essential for solving problems involving them in the calculus unit.
💡Standard Form
A standard form is a set format or template to which something is conformed. In mathematics, it often refers to expressing mathematical expressions or equations in a simplified, conventional format. In the video, converting functions into their standard form is a key step in the process of integration, allowing for the application of calculus rules and techniques.
💡Integration
Integration is the process of finding the area under a curve or the accumulated quantity of a variable as it changes. It is a fundamental concept in calculus and is used to solve a variety of problems. In the video, integration is the primary operation being performed, with the aim of finding the accumulated values of different functions.
💡Limits of Integration
The limits of integration are the boundaries within which the integration is performed. They are crucial in defining the interval over which the area under the curve is to be calculated. In the video, setting the correct limits of integration is a critical step in solving integral calculus problems, ensuring that the calculated area is for the desired segment of the function.
💡Reciprocal
The reciprocal of a number is the value which, when multiplied by the original number, results in a product of 1. In the context of the video, the reciprocal function, or 1/x, is an example of a function that is encountered in integral calculus. Understanding the properties and integration techniques for reciprocal functions is important for solving related calculus problems.
💡Exponential Functions
Exponential functions are mathematical functions of the form f(x) = a^x, where 'a' is a constant and 'x' is the independent variable. These functions are significant in the video as they represent growth or decay processes and are commonly integrated in various applications of calculus. Understanding the behavior and integration of exponential functions is key to solving problems in integral calculus.
💡Differentiation
Differentiation is the process of finding the derivative of a function, which represents the rate of change or the slope of the function at any given point. While not explicitly mentioned in the script, differentiation is closely related to integration and is a fundamental concept in calculus. Understanding differentiation aids in comprehending the behavior of functions and their integrals.
Highlights

Welcome to the Math YouTube channel where we delve into integral calculus and problem-solving techniques.

Today's topic focuses on integral calculus, specifically the concept of knowledge and function.

The problem at hand involves converting expressions into the standard form of integral calculus.

The standard definition of a function is crucial for understanding and solving integral calculus problems.

The process of converting an integral into its standard form is demonstrated with a specific example.

The concept of limits is fundamental in integral calculus and is discussed in detail.

The lecture covers the technique of shifting and converting expressions within the context of integral calculus.

The importance of understanding the lower and upper limits of an integral is emphasized.

The practical application of integral calculus in solving real-world problems is highlighted.

The transcript includes a complex problem-solving process that integrates various mathematical concepts.

The role of the integral calculus in determining the area under a curve is explained.

The concept of indefinite integral and its relationship with definite integrals is clarified.

The transcript touches on the use of technology, such as integral calculators, in solving problems.

The importance of practice and reviewing previous problems is stressed for better understanding.

The transcript provides a comprehensive guide for students and enthusiasts interested in integral calculus.

Transcripts

Browse More Related Video

INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 14

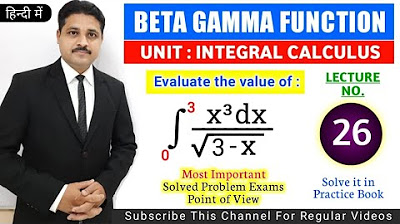
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 26 | BETA GAMMA FUNCTION SOLVED PROBLEM IN HINDI

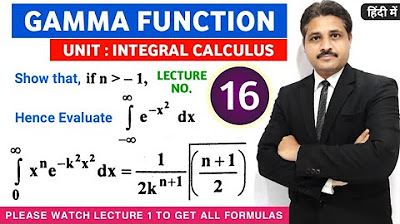
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 16

INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 15

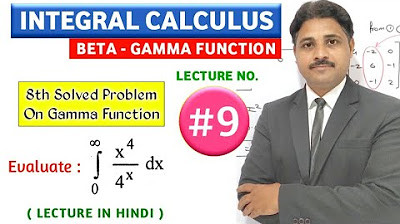
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 9

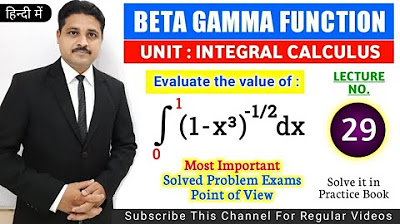
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 29 | BETA GAMMA FUNCTION SOLVED PROBLEM IN HINDI

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
IntegralCalculusMathematicsEducationProblemSolvingFunctionsEducationalContentYouTubeChannelMathTutorialsAdvancedMathOnlineLearning