INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 29 | BETA GAMMA FUNCTION SOLVED PROBLEM IN HINDI

TIKLE'S ACADEMY OF MATHS
24 Jul 202312:36
EducationalLearning
32 Likes 10 Comments

TLDRThe video lecture introduces the 29th lesson on Integral Calculus, focusing on solving a specific problem involving the Beta function. The讲师 begins by converting a given integral into the standard form of the Beta function and then proceeds to solve it step by step. The solution process involves taking the derivative, applying limits, and using the properties of the Beta function. The讲师 emphasizes the importance of understanding the basic concepts of the Beta function and provides a clear explanation of the formula and its application. The video concludes with the讲师 offering a comprehensive solution to the problem, encouraging viewers to practice solving similar problems for better understanding.

Takeaways
  • 📚 The video is a lecture on Integral Calculus, specifically focusing on solving a particular problem involving the Beta function.
  • 🔢 The problem given is to evaluate the integral ∫(1 - x³)^(-1/2) dx, which is part of a series of lectures on Integral Calculus.
  • 🎓 The讲师 begins by converting the given integral into the standard form of the Beta function, which is crucial for solving the problem.
  • 📈 The讲师 then proceeds to differentiate the integral with respect to x, simplifying the expression using the properties of the Beta function.
  • 🌟 The讲师 emphasizes the importance of understanding the basic concept of the Beta function from previous lectures for solving this problem.
  • 📊 The solution process involves finding the limits of x as it approaches 0 and infinity, which are key steps in the evaluation of the integral.
  • 🔍 The讲师 demonstrates the process of converting the integral into the form of the Beta function's standard formula and then solving it.
  • 📝 The讲师 provides a detailed step-by-step solution, including the application of the Beta function's formula and the simplification of the expression.
  • 🎯 The final solution to the problem is derived by applying the properties of the Gamma function and the Beta function in their standard forms.
  • 📌 The讲师 suggests that viewers should practice solving similar problems from previous lectures to better understand the concepts.
  • 🚀 The video concludes with the讲师 encouraging viewers to practice more problems for a better grasp of Integral Calculus, with more problems to be covered in the next lectures.
Q & A

  • What is the main topic of the video?

    -The main topic of the video is the integration of a specific function using the Beta function in calculus.

  • What is the 29th lecture of the Integral Calculus series about?

    -The 29th lecture of the Integral Calculus series is about solving an integral using the Beta function.

  • What is the problem the video aims to solve?

    -The problem the video aims to solve is the evaluation of the integral ∫(1 - x³)^(-1/2) dx.

  • What is the standard form of the Beta function?

    -The standard form of the Beta function is B(x, y) = ∫(0, 1) (1 - xt)^(y - 1)(1 - yt)^(x - 1) dt.

  • How does the video convert the given integral into the standard form of the Beta function?

    -The video converts the given integral by first identifying the integral as a life integral, then converting it into the standard form of the Beta function by applying the formula and making appropriate substitutions.

  • What is the role of differentiation in solving the integral?

    -Differentiation is used to find the derivative of the expressions within the integral, which is crucial for applying the Beta function formula to solve the integral.

  • What are the limits of x in the integral?

    -The lower limit of x in the integral is 0, and the upper limit is 1.

  • How does the video handle the limits of the integral?

    -The video handles the limits by substituting the values of x into the transformed integral expression and evaluating the limits to find the final result.

  • What is the final solution of the integral?

    -The final solution of the integral is expressed in terms of the Beta function with specific parameters derived from the limits and the transformed integral.

  • What is the significance of the Beta function in this context?

    -The Beta function is significant in this context as it provides a method to evaluate integrals of the form (1 - x)^a * (1 + x)^b when a and b are positive real numbers.

  • What advice does the video give for further understanding?

    -The video advises viewers to practice solving similar problems and to review previous lectures for a better understanding of the concepts and to prepare for upcoming problems.

Outlines
00:00
📚 Introduction to Integral Calculus and Problem Solving

The paragraph introduces the topic of Integral Calculus, specifically focusing on the 29th lecture of the series. The speaker welcomes everyone to the video and sets the stage for solving a problem involving the Beta function and Gamma function. The problem involves integrating a function with respect to x, and the speaker emphasizes the importance of understanding the basic concepts of the Beta and Gamma functions before proceeding. The speaker also encourages viewers to review previous lectures for a solid foundation in these concepts.

05:03
🧮 Converting the Integrand to Standard Form

This paragraph delves into the process of converting the given integrand into its standard form. The speaker explains the need to replace the x³ term with a new variable, T, to simplify the integration process. The paragraph outlines the steps to find the derivative of T and how to express the integral in terms of T. The speaker also discusses the importance of understanding the limits of integration and how to apply them to the problem at hand.

10:04
🔢 Solving the Integral Using Beta Function

The speaker continues the problem-solving process by focusing on the Beta function's role in solving the integral. The paragraph explains how to convert the integral into the form of the Beta function and how to apply the formula for the Beta function. The speaker also addresses the need to find the limits of T and how to express the final solution in terms of the original variable, x. The paragraph concludes with the final solution to the integral, demonstrating the application of the Beta and Gamma functions in integral calculus.

Mindmap
Keywords
💡Integral Calculus
Integral Calculus is a branch of mathematics that deals with the study of integrals, which are used to find areas under curves, volumes of solids, and other geometric properties. In the video, the speaker is working on a problem related to integral calculus, specifically focusing on the evaluation of a definite integral involving the Beta function.
💡Beta Function
The Beta function is a generalization of the factorial function to real and complex numbers and is used in various areas of mathematics, including probability theory and combinatorics. In the context of the video, the Beta function is integral to solving the given problem, which involves converting a given integral into a form involving the Beta function and then evaluating it.
💡Standard Form
In mathematics, the standard form refers to a conventional format in which an equation or expression is written to facilitate calculations and understanding. In the video, the speaker converts the given integral into the standard form of the Beta function to simplify the problem and make it easier to solve.
💡Differentiation
Differentiation is a fundamental process in calculus that involves finding the derivative of a function, which represents the rate of change of the function with respect to its variable. In the video, the speaker differentiates the transformed integral expression to apply the properties of the Beta function.
💡Limits
Limits in calculus are used to describe the behavior of a function as the input (or argument) approaches a particular value. In the context of the video, the speaker calculates the limits of the transformed integral expression to find the value of the integral at specific points.
💡Gamma Function
The Gamma function is a generalization of the factorial function to complex numbers and is closely related to the Beta function. It plays a crucial role in various areas of mathematics, including the study of special functions. In the video, the speaker uses the Gamma function in the formula to evaluate the integral involving the Beta function.
💡Evaluation
In mathematics, evaluation refers to the process of determining the value of an expression or function for given input values. The video's main focus is on evaluating a specific integral using the properties of the Beta function and related mathematical techniques.
💡Transformation
Transformation in mathematics involves changing the form or representation of an expression or function to make it easier to work with. In the video, the integral is transformed into a form involving the Beta function, which allows the speaker to apply the relevant formulas and techniques to solve the problem.
💡Series
A series in mathematics is the sum of the terms of a sequence, often used to represent functions or to approximate values. In the context of the video, the speaker may discuss series in relation to the expansion or representation of the Beta function or other related functions.
💡Combinatorics
Combinatorics is a branch of mathematics concerned with counting, arrangement, and combination of objects. The Beta function has applications in combinatorics, as it can be used to calculate certain combinatorial identities and probabilities. Although not directly mentioned in the transcript, the Beta function's relevance to combinatorics is an important aspect of its utility in mathematics.
Highlights

The video welcomes everyone to a lecture on Integral Calculus, focusing on solving a specific problem involving the Beta function.

The problem to be solved is an integral involving the Beta function with a power of -1/2.

The讲师 emphasizes the importance of understanding the basic concept of the Beta function before solving the problem.

The讲师 converts the given integral into the standard form of the Beta function.

The讲师 demonstrates the process of differentiating the integral with respect to the variable x.

The讲师 explains how to find the limits of the variable x, which are crucial for solving the integral.

The讲师 applies the formula for the Beta function to the differentiated integral.

The讲师 simplifies the expression by performing algebraic manipulations.

The讲师 converts the power of x in the integral to a different variable, T, to simplify the process.

The讲师 finds the limits of T to further simplify the integral.

The讲师 replaces the variable x with T in the integral and continues to solve it.

The讲师 explains the process of converting the integral back to the standard form of the Beta function.

The讲师 uses the properties of the Beta function to simplify the final expression.

The讲师 provides the final solution to the integral problem.

The lecturer emphasizes the importance of practicing these problems for a better understanding of Integral Calculus.

The讲师 concludes the lecture by encouraging students to solve more problems for practice.

Transcripts

Browse More Related Video

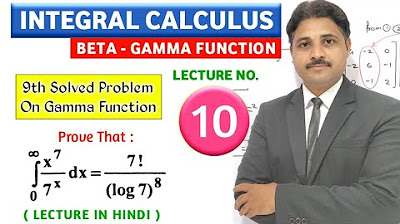
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 10

INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 27 | BETA GAMMA FUNCTION SOLVED PROBLEM IN HINDI

INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 11

INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 28 | BETA GAMMA FUNCTION SOLVED PROBLEM IN HINDI

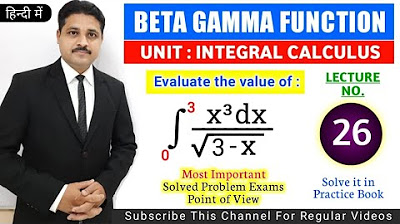
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 26 | BETA GAMMA FUNCTION SOLVED PROBLEM IN HINDI

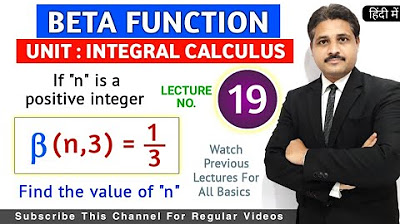
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 19 | BETA FUNCTION SOLVED PROBLEM @TIKLESACADEMY

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
IntegralCalculusLecture29ExponentialFunctionBetaFunctionMathematicsEducationProblemSolvingMathLectureOnlineLearningAdvancedMath