INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 9

TIKLE'S ACADEMY OF MATHS
14 May 202017:19
EducationalLearning
32 Likes 10 Comments

TLDRThe video script discusses the concept of integral calculus, focusing on solving a specific problem involving the integral of a function. It emphasizes the importance of understanding the definition of the integral and its standard form, as well as the technique used to solve the problem, which remains consistent with previous examples. The script also mentions the need to convert the given problem into the proper form and to apply the concept of limits and exponential functions. The video aims to provide a comprehensive practice for viewers by covering a range of problems and topics in integral calculus, encouraging them to invest time in understanding the material and to engage with the content by liking, sharing, and subscribing to the channel for more educational content.

Takeaways
  • 📚 The video is a lecture on Integral Calculus, focusing on solving a specific problem involving integrals.
  • 🔢 The problem discussed involves finding the integral of a given function, with an emphasis on understanding the standard form of the integral.
  • 📈 The lecture introduces the concept of 'Integration by Parts' as a technique to solve the problem, which is a method previously used in the course.
  • 🌐 The script mentions the importance of converting the given problem into a form that matches the standard form of the integral for easy computation.
  • 📊 The video also touches on the concept of 'Integration by Parts' in the context of exponential functions and how to apply it correctly.
  • 🔍 The process of solving the problem involves setting up the integral, identifying the function to integrate, and applying the appropriate formula.
  • 🎓 The lecture emphasizes the need to understand the definition of the function and its standard form to solve the integral correctly.
  • 🤔 The script provides a step-by-step approach to solving the integral, including the use of logarithms and exponential functions.
  • 📋 The video mentions the use of a list of previous problems and topics covered in the course, encouraging viewers to review them for better understanding.
  • 💡 The lecture highlights the importance of practice, suggesting that viewers should solve more problems to gain proficiency in integral calculus.
  • 📝 The video ends with a reminder to like, share, and subscribe for more educational content on similar topics.
Q & A

  • What is the main topic of the lecture?

    -The main topic of the lecture is integral calculus, specifically solving integrals of the form ∫(e^x * sin(x))dx from 0 to infinity.

  • What is the significance of the function's standard form in integral calculus?

    -The standard form of a function is crucial in integral calculus because it allows for the application of established techniques and theorems to evaluate the integral. It is the canonical representation that simplifies the process of finding the integral's value.

  • How does the lecturer approach solving the given integral problem?

    -The lecturer approaches the problem by first ensuring that the function is in the correct form for integration. They then apply the definition of the integral, considering the limits of integration and the properties of the function involved.

  • What is the role of the exponential function in the integral discussed?

    -The exponential function e^x is a part of the product with the sine function in the integral. It is important because it affects the growth rate of the function, which in turn influences the strategy for evaluating the integral.

  • Why is the limit of integration from 0 to infinity significant in this problem?

    -The limits of integration from 0 to infinity indicate that the function is being integrated over the entire real number line starting from zero. This is significant as it often leads to evaluating infinite series or requiring special techniques to handle the infinite limit.

  • How does the lecturer ensure that the integral is set up correctly?

    -The lecturer ensures the integral is set up correctly by defining the function's standard form, checking the limits of integration, and confirming that the function meets the criteria for the integration techniques being used.

  • What is the importance of the definition of the function in solving the integral?

    -The definition of the function is important because it provides the exact form of the function that is being integrated. This is necessary for applying the rules of integration and for evaluating the integral correctly.

  • What is the role of the logarithmic function in the solution?

    -The logarithmic function appears in the solution as part of the process of evaluating the integral. It is used to simplify the expression and to handle the product of the exponential and trigonometric functions within the integral.

  • How does the lecturer address the complexity of the integral?

    -The lecturer addresses the complexity by breaking down the integral into manageable parts, using the properties of the functions involved, and applying the appropriate rules of calculus to simplify and evaluate the expression.

  • What is the final result of the integral as presented in the lecture?

    -The final result of the integral is not explicitly stated in the transcript. However, the lecturer outlines the steps to solve it, which would involve simplifying the expression and evaluating the definite integral using the limits and the properties of the functions involved.

Outlines
00:00
📚 Introduction to Integral Calculus Problem Solving

The video opens with a welcome to viewers on the Stitch Less Academy of Maths YouTube channel, introducing the focus on advancing the topic of integral calculus. The presenter announces that they will solve a specific problem in integral calculus, marking it as not the first lecture on the subject. Previous lectures have covered a number of problems and topics in mathematics. The presenter encourages viewers to check the video description for links to playlists of previous lectures for a comprehensive understanding of the topics covered before diving into today’s problem-solving session.

05:00
🔍 Detailed Problem-Solving Technique

The presenter outlines a step-by-step approach to solving an integral calculus problem. The technique involves converting the given problem into a standard form using known definitions and comparing it to a 'gamma function' standard form. The explanation covers the adjustment of the integral's limits and the manipulation of the function into a format that aligns with the gamma function's definition. This detailed explanation includes the conversion of expressions and the importance of understanding the fundamental definitions and properties of functions involved in the solution process.

10:01
🧩 Converting to Gamma Function Form

This segment focuses on the critical step of transforming the problem into a gamma function format, which involves a series of substitutions and mathematical manipulations. The presenter highlights the importance of accurately setting up the problem according to the gamma function’s definition, emphasizing the role of constants and the need for precision in aligning the problem's form with that of the gamma function. This section meticulously explains how to achieve this alignment and set the stage for solving the problem.

15:03
🎓 Final Steps and Conclusion

The concluding part of the video showcases the final steps in solving the integral calculus problem, leading to the expression in terms of the gamma function. The presenter explains the application of the gamma function formula, providing a clear path to the problem's solution. The segment wraps up with a summary of the process, highlighting the factorial function’s role in the solution. The presenter encourages further practice with similar problems available in the channel's playlists and invites viewers to like, share, and subscribe for more educational content.

Mindmap
Keywords
💡Integral Calculus
Integral Calculus is a branch of mathematics that deals with the study of integrals, which are used to find areas under curves, volumes of solids, and other similar quantities. In the video, the speaker is working on problems related to integral calculus, specifically focusing on the computation and application of definite integrals.
💡Limits
In calculus, limits are a fundamental concept that describes the behavior of a function as the input (or argument) approaches a particular value. Limits are used to define continuity, derivatives, and integrals. In the context of the video, the speaker is likely discussing how to evaluate limits in relation to integral calculus problems.
💡Functions
A function in mathematics is a relation that assigns a single output value to each input value. Functions are central to the study of calculus as they allow us to model and analyze changes. The video likely involves the manipulation and evaluation of functions within the context of integral calculus.
💡Power Series
A power series is an infinite series that represents a function as the sum of a sequence of terms calculated from a power of the variable. Power series are used in calculus to approximate functions and find their derivatives and integrals. The video might be discussing how to work with power series in the context of integral calculus.
💡Exponential Functions
Exponential functions are mathematical functions where the base is a constant and the exponent is the variable. They are crucial in many areas of mathematics, including calculus, where they are used to model growth and decay processes. The video likely involves integrals or derivatives of exponential functions.
💡Definite Integrals
A definite integral represents the signed area under a curve between two points on the x-axis. It is a fundamental concept in integral calculus and is used to calculate quantities such as the area of a region, the displacement of an object, or the total change in a quantity. The video's main focus seems to be on solving problems involving definite integrals.
💡Logarithmic Functions
Logarithmic functions are inverse trigonometric functions that calculate the power to which a base must be raised to produce a given number. They are used in various applications, including solving exponential equations and in financial modeling. The video might be discussing the properties of logarithmic functions and their role in integral calculus.
💡Factorial
The factorial of a non-negative integer n is the product of all positive integers less than or equal to n. It is denoted by n! and is used in combinatorics and probability theory. In the context of the video, factorial might be discussed in relation to functions that are being integrated or differentiated.
💡Infinite Series
An infinite series is a sequence of numbers that adds up to a finite value. It is a fundamental concept in calculus, especially in the study of sequences and series convergence. The video likely involves the convergence or divergence of infinite series and their applications in integral calculus.
💡Differential Equations
Differential equations are equations that involve an unknown function and its derivatives. They are crucial in modeling various phenomena in physics, engineering, and other sciences. The video might be discussing how to solve differential equations using integral calculus techniques.
Highlights

The video introduces integral calculus and its application to solve a problem involving integration.

The problem discussed involves finding the integral of a function with specific conditions.

The method used to solve the problem is based on the standard form of the integral and its properties.

The video emphasizes the importance of understanding the definition of the integral function and its standard form.

The solution process involves converting the given problem into a form that matches the integral function's definition.

The video demonstrates the process of setting up the integral by considering given permissions and constraints.

The integral's value is found by applying the definition of the function and its properties.

The video provides a detailed explanation of how to write the integral in the proper form for solving the problem.

The solution involves comparing the given problem with the integral's standard form to ensure they match.

The video explains the importance of converting the problem into the integral's standard form for easy computation.

The process of solving the integral is demonstrated step by step, with clear explanations at each stage.

The video concludes with the successful solution of the integral problem, providing a clear and correct answer.

The video encourages practice and understanding of integral calculus by suggesting viewers to watch previous videos for better comprehension.

The presenter invites questions and discussions in the comments section for further clarification and learning.

The video ends with an invitation to subscribe to the channel for more educational content on mathematics and other topics.

Transcripts

Browse More Related Video

INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 14

INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 13

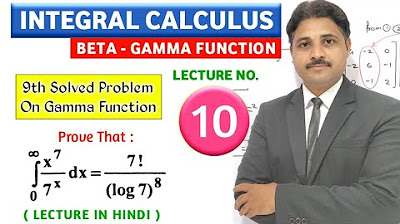
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 10

INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 15

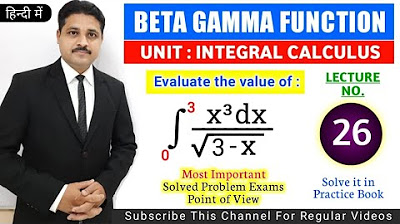
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 26 | BETA GAMMA FUNCTION SOLVED PROBLEM IN HINDI

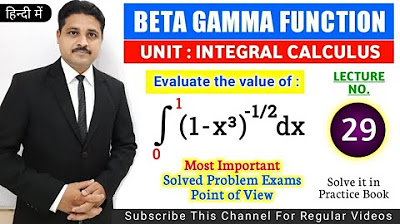
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 29 | BETA GAMMA FUNCTION SOLVED PROBLEM IN HINDI

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
IntegralCalculusExponentialFunctionsLimitsPowerFunctionsMathLectureEducationalContentAdvancedMathOnlineLearningMathematicsProblemSolving