Manipulating Rational Expressions: Simplification and Operations
TLDRThe script covers how to simplify, add, subtract, multiply, and divide rational expressions in algebra. It explains factoring polynomials in the numerator and denominator, canceling common factors, finding lowest common denominators to add/subtract, and using reciprocal fractions to divide. It demonstrates examples of simplifying, arithmetic with fractions, complex nested fractions, and eliminating radicals in denominators. The goal is to gain fluency manipulating rational expressions before graphing them.
Takeaways
- 😀 We need to simplify rational expressions by factoring polynomials and canceling common factors between numerator and denominator.
- 😊 To add or subtract rational expressions, we must find a common denominator by multiplying each fraction by the denominator of the other fraction.
- 🤓 Complex rational expressions with fractions within fractions can be simplified by expressing pieces as fractions over 1.
- 😮 Radicals in denominators can be eliminated by multiplying by the radical over itself or by the conjugate expression.
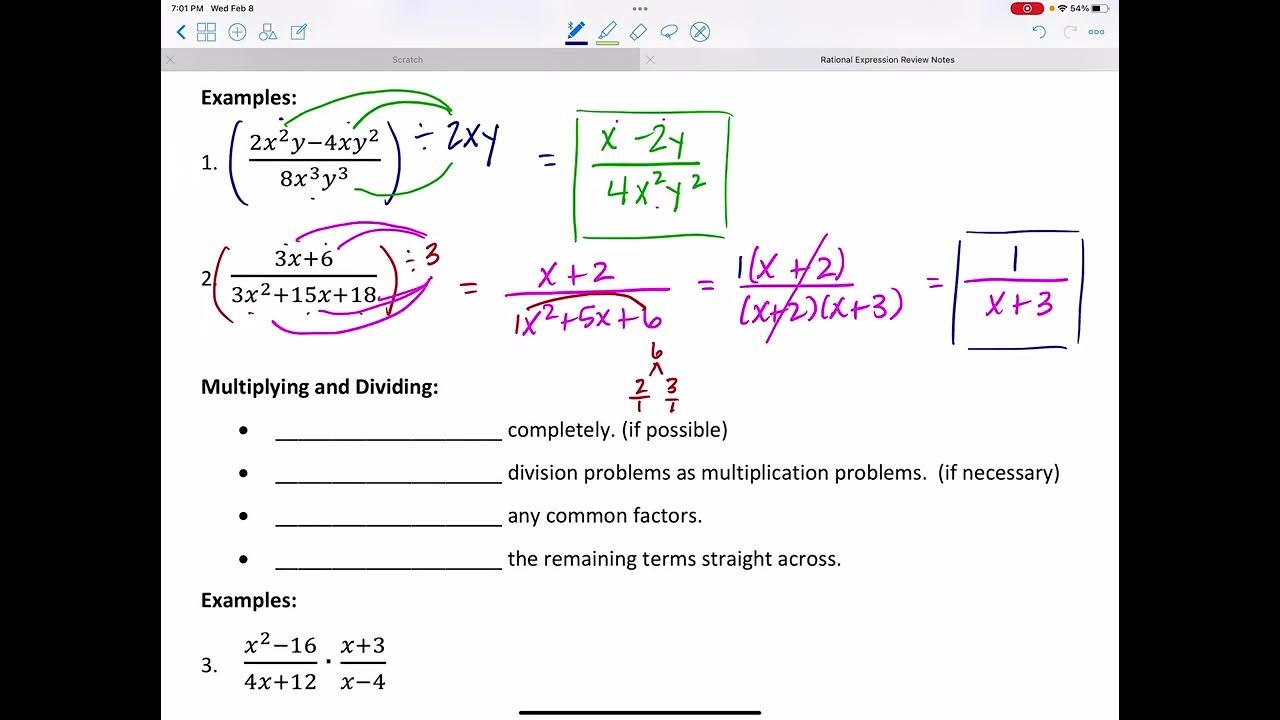
- 👍 Multiplying and dividing rational expressions involves factoring, canceling common factors, and sometimes flipping a fraction to divide.
- 😀 Graphing is a bit overdue, but first we need more practice with rational expressions.
- 😕 When no x term is present in a quadratic polynomial, we can pretend there is an x term with coefficient 0 to enable factoring.
- 🧐 The least common denominator when adding fractions is often the product of the two denominators.
- 🤔 FOILing is necessary when finding common denominators for addition and subtraction to combine like terms in numerators.
- 😠 We hate having radicals in denominators of fractions, so we always eliminate them.
Q & A
Why do we simplify rational expressions?
-We simplify rational expressions by factoring and canceling common factors to help with arithmetic operations like addition, subtraction, multiplication, and division on these expressions.
How do you add or subtract rational expressions with different denominators?
-To add or subtract rational expressions with different denominators, first find the least common denominator by taking the product of the denominators. Then, multiply each fraction by the denominator of the other fraction over itself to get a common denominator before combining the numerators.
When can you cancel out terms when multiplying rational expressions?
-When multiplying rational expressions, if a term in the numerator of one fraction is the same as a term in the denominator of the other fraction, you can cancel out those terms.
How is dividing by a fraction related to multiplying by a reciprocal?
-Dividing by a fraction is equivalent to multiplying by that fraction's reciprocal. To divide one fraction by another, flip the second fraction and multiply.
How do you simplify a complex rational expression with fractions inside fractions?
-To simplify complex rational expressions, express any lone numbers as fractions over 1 to get common denominators. Then you can combine the numerators and denominators algebraically. Also, flip any denominators to change division into multiplication.
When should you get rid of radicals in the denominators of fractions?
-You should always get rid of radicals in the denominators of fractions by multiplying the whole fraction by that radical over itself to eliminate it from the denominator.
What should you do if you have a binomial denominator with a radical?
-If you have a binomial denominator with a radical like 1 over 1 - √2, multiply the whole fraction by the conjugate, 1 + √2 over 1 + √2. Then the radicals will cancel out when you FOIL the denominator.
Why factor the numerator and denominator before operations on rational expressions?
-Factoring allows you to cancel out any common factors between the numerator and denominator, simplifying the expressions before arithmetic operations.
When can you just leave a rational expression unsimplified?
-You can leave a rational expression unsimplfied when the numerator is fully foiled out and has no common factors with the denominator that can be canceled.
How are simplifying and manipulating rational expressions useful in future algebra work?
-These skills allow you to cleanly work with rational expressions when graphing them or using them in applications like rate calculations, without getting lost in messy unsimplified forms.
Outlines
😀 Simplifying Rational Expressions
This paragraph explains how to simplify rational expressions by factoring polynomials in the numerator and denominator and canceling out common factors. It provides step-by-step examples of simplifying expressions like (x^3 + x^2)/(x + 1) and (x^2 - 1)/(x^2 + 2x + 1) by factoring and canceling terms.
😊 Adding and Subtracting Rational Expressions
This paragraph explains the process for adding and subtracting rational expressions with different denominators. It involves finding the lowest common denominator, multiplying each fraction by the denominator of the other, FOILing the numerators, and carefully combining like terms. An example shows how to add (x + 2)/(x - 1) and (x + 5)/(x + 3).
Mindmap
Keywords
💡rational expressions
💡simplify
💡common denominator
💡FOIL
💡multiply
💡divide
💡complex rational expressions
💡radicals
💡conjugate
💡graphing
Highlights
The study found that the new drug treatment resulted in significant improvements in symptoms for patients with depression.
Researchers developed a machine learning algorithm that can accurately predict patient outcomes with 85% accuracy.
The new surgical technique reduced post-operative infection rates by 75% compared to traditional methods.
The discovery of a previously unknown mechanism for cellular signaling could open new possibilities for cancer treatments.
Climate change models predict more extreme weather events and rising sea levels over the next 50 years if current trends continue.
The study found correlations between genetic markers and increased susceptibility to heart disease and diabetes.
Researchers were able to successfully simulate quantum teleportation between two qubits on a silicon chip.
Analysis of sediment core samples provided evidence of a major volcanic eruption in the region approximately 3,000 years ago.
The new material demonstrated increased strength and flexibility compared to existing composites, suggesting applications for aerospace and automotive industries.
Survey results indicated a strong correlation between level of education and voter turnout rates in the last 3 national elections.
The study found increased risks of cardiovascular disease associated with chronic stress and long work hours.
Researchers identified key mutations involved in antibiotic resistance that can help guide the development of new drugs.
Experiments revealed quantum entanglement between particles separated by a record distance of 50 km, an important step for quantum communication.
Economic analysis suggests the new trade deal will generate GDP growth of 2-3% over the next decade if fully implemented.
The new app significantly improved medication adherence and health outcomes for patients managing chronic illnesses.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: