Solving Algebraic Equations With Roots and Exponents

Professor Dave Explains

14 Sept 201705:45

EducationalLearning

32 Likes 10 Comments

TLDRIn this algebra video, Professor Dave teaches how to solve equations involving roots and exponents. He explains that square roots and cube roots can be written as fractional exponents, so you have to undo operations systematically, starting from the outside. He demonstrates solving equations step-by-step, using the rules of exponents and radicals to isolate the variable term. The goal is to methodically apply these rules to remove any roots, exponents, or extraneous terms acting on the variable you aim to solve for. With practice, this becomes an intuitive process of strategically manipulating roots and exponents until only the variable remains.

Takeaways

😀 The transcript covers algebraic equations involving roots and exponents
😊 Square roots and cube roots can be represented using fractional exponents
🤓 To solve equations with square roots, square both sides to eliminate the square root
🧐 Be careful about the order of operations when solving equations with roots
🤔 To eliminate a root, raise both sides to the reciprocal exponent
😎 Use rules for manipulating exponents to simplify expressions with roots
🥸 Equations with square roots have two possible solutions
😲 Eliminate roots before other operations when solving equations
🔍 Apply rules for exponents and roots step-by-step to isolate the variable
🤩 With practice, solving equations with roots and exponents becomes straightforward

Q & A

What is the first step to solve an equation with a square root, like the square root of (X plus 9) equals 5?
-The first step is to square both sides of the equation to eliminate the square root. This leaves you with X + 9 = 25.
Why can't you just subtract 9 from both sides of the square root of (X plus 9) equals 5?
-Because the 9 is inside the square root, subtracting 9 would leave you with the square root of X plus 9 minus 9, which does not simplify the equation.
How do you get rid of an exponent like the three halves power in the equation X + 2 to the three halves power equals 8?
-You can raise both sides of the equation to the reciprocal exponent, in this case two thirds, which will cancel out the original exponent and leave you with just X + 2.
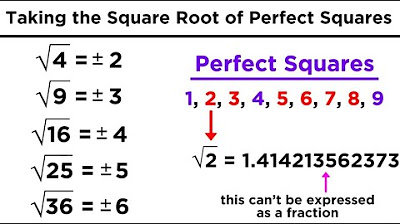
What are the two solutions when you take the square root of both sides of an equation like X squared plus 5 equals 41?
-When you take the square root, you get two solutions - positive 6 and negative 6. Equations with square roots generally have two solutions.
In the last example, 3 root (X - 1) = root (X + 1), why do you have to square both sides first?
-Squaring both sides eliminates the square root radicals. This simplifies the equation to work with.
After squaring both sides in the last example, why do you have to multiply the 3 and the radical term on the left side?
-Because when you square a term with multiple factors, you have to square each factor. So you square the 3 and the radical term separately.
What are some key rules to remember when manipulating roots and exponents to solve equations?
-Some key rules are: square roots and squared terms undo each other; you can eliminate exponents by raising both sides to the reciprocal exponent; be systematic by undoing operations inside-out.
What is a common mistake students make when trying to solve equations with roots?
-A common mistake is trying to break apart terms under a root sign or subtract terms that are under a root. The root applies to the entire quantity.
Why do some equations with squared terms have two solutions?
-Because when you take the square root of a squared term, you get both a positive and negative root. So there are two solutions.
What should you remember about order of operations when solving equations with roots and exponents?
-Remember to go from the inside-out, undoing each operation systematically before moving to undo the next outer operation.

Outlines

00:00

😊 Introducing More Algebra with Roots and Exponents

Paragraph 1 introduces more advanced algebra involving roots like square roots, cube roots, and fractional exponents. It explains how these can be used to solve trickier algebraic equations than before by strategically applying rules for manipulating roots and exponents.

😀 Step-by-Step Solution of a Square Root Equation

Paragraph 2 provides a step-by-step walkthrough of solving the equation: The square root of (X plus nine) equals five. It emphasizes the importance of removing the square root first before attempting to isolate the variable X.

😃 Solving an Equation with a Fractional Exponent

Paragraph 3 demonstrates solving an equation involving a fractional exponent: The quantity X plus two to the three halves power equals eight. It shows how to eliminate the exponent efficiently in one step by raising both sides to the reciprocal power.

😉 Dealing with Two Solutions from Square Roots

Paragraph 4 points out that equations involving square roots have two solutions, using the example: X squared plus five equals forty-one. After simplifying, this gives two solutions: positive six and negative six.

😇 One Final Comprehensive Example Problem

The last paragraph provides one more complex example to reinforce the concepts: Three root (X minus one) equals root (X plus one). It steps through eliminating radicals, distributing, combining like terms, and simplifying to ultimately solve for X.

Mindmap

Keywords

💡algebra

Algebra is a branch of mathematics that uses symbols, usually letters, to represent unknown values or quantities. In the video, algebra is used to set up and solve equations with variables like X. Key algebraic concepts covered include roots, exponents, squaring and cubing equations, etc. These are applied to eliminate terms step-by-step and solve for the unknown variable X.

💡equations

Equations state that two mathematical expressions have equal value. The video teaches how to solve algebraic equations with variables, where the goal is to isolate the variable term (often X) on one side. This involves undoing operations like roots, exponents, addition/subtraction by applying their inverse operations.

💡roots

Roots refer to radical expressions like square roots and cube roots. They signify a number that when multiplied by itself gives the radicand. The video covers manipulating equations with root terms by using fractional exponents and squaring/cubing to eliminate the roots.

💡exponents

Exponents represent repeated multiplication of a number by itself. The video uses rules of exponents to manipulate equations - for example, squaring both sides to eliminate a square root. Fractional exponents like 1/2 also denote roots.

💡squaring

Squaring means raising a quantity to the power of 2. The video shows squaring both sides of an equation with a square root, which cancels out the root and makes the equation simpler to solve.

💡cubing

Cubing refers to raising a number to the 3rd power. Similarly the video uses cubing equations with cube roots to eliminate the root.

💡order of operations

This refers to the sequence in which numerical operations must be performed to evaluate an expression. As explained in the video, proper order is crucial when manipulating equations with multiple terms under roots or exponents.

💡subtracting/adding

After eliminating roots, exponents etc. from equations, basic addition and subtraction is used to isolate the variable term on one side, often by subtracting constants from both sides.

💡dividing

Sometimes terms on both sides need to be divided by a common factor to get the variable X alone on one side. This is done after eliminating roots/exponents and subtracting/adding.

💡solutions

The solutions refer to the value(s) for the variable that satisfy the equation. As noted in the video, equations with squared terms can have two solutions, while others have one.

Highlights

The speaker discusses using deep learning for natural language processing.

Word embeddings are introduced as a way to capture semantic meaning.

The ELMo model is explained as an improvement over word embeddings.

Transformers are presented as a novel neural network architecture.

Attention mechanisms allow transformers to focus on relevant parts.

BERT leverages transformers and attention for bidirectional pretraining.

Fine-tuning is discussed as a way to adapt BERT to new tasks.

RoBERTa is presented as an improvement to the BERT approach.

XLNet aims to overcome limitations of auto-regressive models.

The speaker reflects on progress and remaining challenges.

Ethical considerations around bias are highlighted.

Applications to question answering are discussed.

Sentiment analysis is mentioned as an impactful use case.

Future directions could include multimodal learning.

The speaker concludes by emphasizing responsible AI development.

Transcripts

Browse More Related Video

Square Roots, Cube Roots, and Other Roots

Simplifying Expressions With Roots and Exponents

Algebra Basics: Exponents In Algebra - Math Antics

Algebraic Equations and Their Solutions

Using Substitutions to Solve Equations (Precalculus - College Algebra 23)

First Principles Example: Square Root of x