INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 28 | BETA GAMMA FUNCTION SOLVED PROBLEM IN HINDI

TIKLE'S ACADEMY OF MATHS

13 Jun 202320:55

EducationalLearning

32 Likes 10 Comments

TLDRIn this engaging lecture from the 28th installment of the Integral Calculus series, the focus is on solving a specific problem involving the integral of an exponential function. The video meticulously guides the audience through converting the given integral into the standard form of the beta function, applying various formulas, and simplifying the expression step by step. The lecture emphasizes the importance of understanding the properties and formulas related to beta and gamma functions, and it encourages practice to master the calculations essential for tackling similar problems in exams.

Takeaways

The video is a lecture on integral calculus, specifically focusing on solving an integral involving the beta and gamma functions.
The problem to be solved is an integral of the form ∫(3x^2 / (x^2 + 3))^(1/3) dx.
To solve the integral, the讲师 introduces the concept of the beta function and its standard form, which is crucial for solving the problem.
The讲师 emphasizes the importance of understanding the properties and formulas of the beta and gamma functions before attempting the problem.
The讲师 demonstrates the process of converting the given integral into the standard form of the beta function by changing the limits of integration and the integrand.
The讲师 uses the properties of exponents and roots to simplify the integrand and to find the correct form for the beta function.
The讲师 explains how to find the limits of the integral by setting up the integral in the form of the beta function and then solving for the variable.
The讲师 shows how to apply the formula for the beta function to the integral, which involves the product of the gamma functions of the exponents in the numerator and the denominator.
The讲师 provides a step-by-step solution to the integral, including the simplification of the expression and the calculation of the final result.
The final solution to the integral is given as (48√3) / 5, demonstrating the application of the beta and gamma functions in integral calculus.
The讲师 encourages students to practice solving problems of this type as they often appear in exams and to review previous lectures for a better understanding of the concepts.

Q & A

What is the topic of the video?
-The topic of the video is the 28th lecture on Integral Calculus, specifically solving an integral involving the beta function.
What is the integral problem discussed in the video?
-The integral problem discussed is to evaluate the integral of (1 / (x^2 + 3 - x * sqrt(3))) dx from 0 to 1.
What are the key formulas needed to solve the problem?
-The key formulas needed include the properties and rules of the beta function and gamma function, as well as the standard form of the beta function.
What is the standard form of the beta function?
-The standard form of the beta function is B(x; a, b) = (x^(a-1) * (1-x)^(b-1)) / B(a, b), where B(a, b) is the beta function and is a normalization constant.
How does the video approach the conversion of the given integral to the standard form?
-The video approaches the conversion by first identifying the limits of integration, then applying formulas related to the beta and gamma functions to transform the integral into a form that can be solved.
What is the role of the gamma function in solving the integral?
-The gamma function is used to simplify the expression under the square root in the denominator, which helps in applying the beta function formula for solving the integral.
How does the video handle the limits of integration in the problem?
-The video identifies that the lower limit of integration is 0 and the upper limit is 1, and then proceeds to adjust the expression to match the standard form of the beta function, which requires the limits to be from 0 to 1.
What is the final solution to the integral problem presented in the video?
-The final solution to the integral problem is 48√3 / 5, which is derived after applying the beta and gamma function formulas and simplifying the resulting expression.
What advice does the video give for practicing integral calculus?
-The video advises to practice solving problems like the one presented, especially those involving the beta function, and to review previous lectures that covered related problems for better understanding and practice.
Why is it important to avoid mistakes in calculations like the one discussed in the video?
-Avoiding mistakes in such calculations is crucial because they often lead to incorrect results in exams and can cause students to miss out on important concepts and problem-solving techniques in integral calculus.

Outlines

00:00

📘 Introduction to Integral Calculus - Lecture 28

The paragraph introduces the 28th lecture on integral calculus, focusing on solving a specific problem involving the beta function. It emphasizes the importance of understanding beta and gamma functions' formulas and properties. The problem to be solved is presented, and the video encourages viewers to apply the formulas and rules learned in previous lectures to find the solution.

05:01

📚 Derivative and Limit Concepts in Solving Integrals

This section delves into the process of solving integrals by first differentiating and then finding limits. It explains the need to identify the lower and upper limits for the integral and how to convert the given integral into the standard form of the beta function. The paragraph also discusses the importance of understanding the limits of integration, particularly when they are constants, and how to handle them in the calculation.

10:02

🔢 Applying Formulas to Convert and Solve the Integral

The paragraph outlines the step-by-step process of converting the given integral into a form that can be solved using the formulas of beta and gamma functions. It details the algebraic manipulations required to match the integral with the standard form, including the substitution of variables and the simplification of terms under the radical and in the denominator. The explanation is technical and assumes prior knowledge of calculus concepts.

15:02

📈 Solving the Integral Using Gamma Function Properties

This part of the script focuses on using the properties of the gamma function to solve the integral. It explains how to apply the gamma function formula to the transformed integral and how to simplify the expression by canceling out terms and performing multiplications. The paragraph also touches on the importance of understanding the factorial property of the gamma function and how it simplifies the calculation of the integral.

20:02

🎓 Conclusion and Practice Recommendations

The conclusion summarizes the solution to the integral problem and reiterates the importance of practicing this type of calculation. It encourages students to review previous lectures for a better understanding of the concepts and to solve more problems for practice. The video ends with a reminder that the next video will continue with more problems, emphasizing the importance of consistent practice in integral calculus.

Mindmap

Keywords

💡Integral Calculus

Integral calculus is a branch of mathematics that deals with the study of integrals, which are used to find areas under curves, volumes of solids, and other similar quantities. In the context of the video, integral calculus is the main subject being discussed, with a specific focus on solving integrals involving exponential functions, as indicated by the problem-solving process detailed in the script.

💡Exponential Function

An exponential function is a mathematical function of the form f(x) = a^x, where 'a' is a constant and 'x' is the variable. These functions are crucial in modeling growth and decay processes. In the video, the exponential function is part of the integral that needs to be solved, highlighting the importance of understanding exponential functions when dealing with integral calculus.

💡Beta Function

The Beta function is a generalization of the factorial function to real and complex numbers. It is used in various areas of mathematics, including probability theory and combinatorics. In the video, the Beta function is mentioned as part of the formulas needed to solve the integral, showing its relevance in advanced calculus problems.

💡Gamma Function

The Gamma function is a mathematical function that extends the factorial function to complex numbers. It is used in many areas of mathematics and physics, particularly in the study of special functions. In the context of the video, the Gamma function is used as part of the solution process for the integral, demonstrating its importance in integral calculus.

💡Standard Form

In mathematics, the standard form of an equation or function is a conventional, simplified, and widely accepted format. It is used to make calculations and comparisons easier. In the video, the standard form of the Beta function is discussed, which is necessary to understand and solve the integral problem.

💡Limits

In calculus, limits are used to describe the behavior of a function as the input (or argument) approaches a particular value. They are fundamental to understanding continuity, derivatives, and integrals. The script discusses the limits of the integral from zero to three, which is crucial for solving the given problem.

💡Differentiation

Differentiation is the process of finding the derivative of a function, which represents the rate of change of the function with respect to its variable. It is a key concept in calculus and is used to analyze functions and their behavior. In the video, differentiation is mentioned as a step in solving the integral, showing its importance in the process.

💡Conversion

Conversion in mathematics refers to the process of changing a mathematical expression from one form to another, often to simplify it or to make it easier to solve. In the video, conversion is discussed in the context of transforming the given integral into the standard form of the Beta function.

💡Solving Integrals

Solving integrals involves finding the antiderivative or the function whose derivative equals the given integrand. It is a fundamental process in integral calculus. The video's main focus is on solving a specific integral involving exponential functions, showcasing the steps and techniques required to find the solution.

💡Practice Problems

Practice problems are exercises that are used to help learners apply and reinforce the concepts they have learned. In the context of the video, practice problems involving integral calculus are recommended for viewers to better understand and master the material.

💡Lecture Series

A lecture series is a set of presentations or lessons on a particular topic, often delivered by an expert or educator. In the video, the lecture series is focused on integral calculus, with the current video being the 28th lecture in the series.

Highlights

The video is a part of a lecture series on Integral Calculus, specifically the 28th lecture.

The problem to be solved involves an integral of an exponential function multiplied by a power of x and divided by a root of 3.

The process begins by converting the given integral into the standard form of the beta function.

It is important to understand the properties and formulas related to the beta and gamma functions to solve the problem.

The integral is transformed by applying various formulas and rules, including the limits of integration.

The problem involves changing the limits of integration from 0 to 3 and adjusting the power of x accordingly.

The solution requires factoring out constants and simplifying the terms under the root and in the denominator.

The use of the gamma function formula is crucial in finding the final solution.

The lecture emphasizes the importance of practicing problems of this type for better understanding and application in exams.

The final answer is derived by simplifying the expression and canceling out terms in the numerator and denominator.

The video provides a detailed step-by-step solution to the problem, ensuring clarity and understanding.

The lecture series covers a range of problems and formulas in Integral Calculus, aiming to build a strong foundation for students.

The problem-solving approach demonstrated in the video is applicable to a variety of similar integral calculus problems.

The video is a valuable resource for students preparing for exams that involve Integral Calculus.

The lecture series is designed to help students avoid common mistakes in the calculation of integrals.

The video concludes with an encouragement for students to practice more problems for better mastery of Integral Calculus.

Transcripts

Browse More Related Video

INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 27 | BETA GAMMA FUNCTION SOLVED PROBLEM IN HINDI

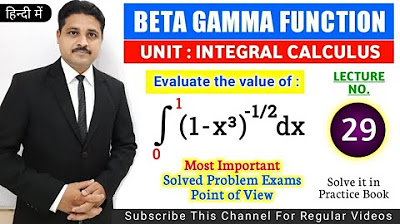
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 29 | BETA GAMMA FUNCTION SOLVED PROBLEM IN HINDI

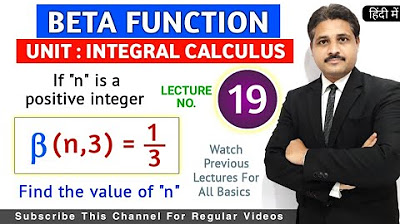
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 19 | BETA FUNCTION SOLVED PROBLEM @TIKLESACADEMY

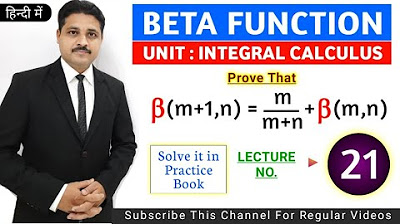
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 21 | BETA FUNCTION SOLVED PROBLEM

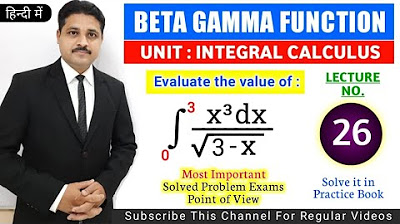
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 26 | BETA GAMMA FUNCTION SOLVED PROBLEM IN HINDI

INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 15

Related Tags

IntegralCalculus BetaFunction GammaFunction MathLecture AdvancedMath Education Mathematics OnlineLearning CalculusSeries MathPractice