INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 16

TIKLE'S ACADEMY OF MATHS
8 Feb 202119:04
EducationalLearning
32 Likes 10 Comments

TLDRThe video script is an in-depth tutorial on integral calculus, focusing on solving a specific problem involving knowledge and functions. The instructor welcomes viewers to the YouTube channel and encourages them to watch previous videos for context. The problem involves complex calculations, including converting the integral to standard form, applying various mathematical techniques, and solving for specific functions. The solution process is methodical, emphasizing the importance of understanding the principles of integral calculus and the application of formulas. The video aims to enhance the viewer's comprehension of advanced mathematical concepts and their practical application.

Takeaways
  • ๐Ÿ“˜ The video is a tutorial on integral calculus, focusing on solving a specific problem involving knowledge and functions.
  • ๐Ÿ”ข The problem given in the question involves calculating a great wall area and is set in the year 202, with specific mathematical expressions.
  • ๐Ÿ“Œ The solution process includes converting the given integral into its standard form, which involves dealing with functions of knowledge and functions.
  • ๐Ÿค” The video emphasizes the importance of understanding the standard form of integral calculus and how to convert expressions into this form for easier problem-solving.
  • ๐Ÿง  The่ฎฒๅธˆ explains the concept of depression in the context of the problem, highlighting the second form of the knowledge function.
  • ๐Ÿ”„ The video demonstrates the use of substitution and rearrangement techniques to simplify the integral and solve the problem.
  • ๐Ÿ“Š The่ฎฒๅธˆ also discusses the concept of limits in integral calculus, showing how to find the lower and upper limits for the integral.
  • ๐Ÿ‘“ The solution involves the use of power rules and the concept of limits, which are crucial for solving advanced calculus problems.
  • ๐Ÿ“ˆ The่ฎฒๅธˆ provides a step-by-step breakdown of the solution, ensuring that viewers can follow along and understand the process.
  • ๐ŸŒŸ The video concludes with the่ฎฒๅธˆ solving the problem and providing the final answer, reinforcing the concepts taught throughout the tutorial.
  • ๐Ÿ’ก The่ฎฒๅธˆ encourages viewers to practice and apply the techniques learned in the video to improve their understanding of integral calculus.
Q & A

  • What is the main topic of the video?

    -The main topic of the video is solving a problem involving integral calculus and functions in a mathematical context.

  • What does the video suggest to do before starting the problem?

    -The video suggests reviewing all previous videos in the series by visiting the description of the current video, where a playlist link will be provided for easy access.

  • How does the video approach the problem-solving process?

    -The video approaches the problem-solving process by first converting the given integral into its standard form, then applying various mathematical techniques to simplify and solve the problem.

  • What is the significance of the 'Should have' and 'Given' expressions mentioned in the script?

    -The 'Should have' and 'Given' expressions refer to the expected and provided integral forms, respectively, which are crucial for converting the problem into a solvable format.

  • What does the term 'Depression' mean in the context of the script?

    -In the context of the script, 'Depression' likely refers to a mathematical function's form, specifically the second form of the integral function that needs to be evaluated.

  • How does the video explain the concept of limits in the context of integral calculus?

    -The video explains limits by discussing how to convert the given problem into a form that allows for easy evaluation of the limits, specifically focusing on the lower and upper limits of the integral function.

  • What is the role of the 'Extra Elephant' in the problem-solving process?

    -The 'Extra Elephant' seems to be a term used in the script to refer to an additional variable or term that is introduced during the problem-solving process, possibly to help in simplifying or evaluating the expression.

  • How does the video address the concept of 'Defensive' in the script?

    -The term 'Defensive' in the script likely refers to a step or technique used to simplify the problem or to address a specific challenge within the integral calculus process.

  • What is the significance of the 'Root' mentioned in the script?

    -The 'Root' mentioned in the script is likely a mathematical concept used to simplify the integral expression or to find the value of the function at a particular point.

  • How does the video conclude the problem-solving process?

    -The video concludes the problem-solving process by verifying that the final result matches the expected outcome, ensuring that all steps have been correctly applied, and providing a clear solution to the problem.

Outlines
00:00
๐Ÿ“˜ Introduction to Integral Calculus

The video begins with a warm welcome to the audience on the YouTube channel, focusing on Integral Calculus. The first problem of knowledge and function is solved, highlighting the process of solving integral calculus. The video encourages viewers to watch previous videos for context and introduces the concept of solving a problem in integral calculus, emphasizing the importance of understanding the standard form of integral and function.

05:01
๐Ÿ”ข Dealing with Integral Limitations

The second paragraph delves into the specifics of handling limitations in integral calculus. It discusses the process of converting the given integral into a standard form and solving it using previously used methods. The paragraph also explains how to deal with the left-hand side of the equation and the concept of limits, providing a detailed walkthrough of the steps involved in solving the integral calculus problem.

10:02
๐Ÿ”„ Integrating and Simplifying Expressions

This section focuses on the integration process, simplifying expressions, and solving complex problems in integral calculus. It explains the technique of replacing certain elements in the equation to simplify the problem and illustrates how to deal with different types of limits, such as lower and upper limits, in the context of integral calculus.

15:03
๐Ÿ“ˆ Finalizing the Integral Calculation

The final paragraph wraps up the integral calculus problem by solving the remaining parts and ensuring that the solution matches the standard form of the knowledge and function. It emphasizes the importance of checking the solution against the given problem and provides a comprehensive review of the entire process, from setting up the integral to simplifying and solving the equation.

Mindmap
Keywords
๐Ÿ’กIntegral Calculus
Integral calculus is a core concept in the field of mathematics that deals with the study of integrals, which are used to find areas under curves, volumes of solids, and other similar quantities. In the video, the author is working on a problem that involves solving an integral calculus equation, indicating the importance of this concept in understanding the content.
๐Ÿ’กFunctions
Functions are mathematical relationships between sets of inputs and outputs. In the context of the video, the author is working with functions to solve a problem, which is a common application in calculus. Functions are essential for modeling real-world situations and are used to represent changes or dependencies between different quantities.
๐Ÿ’กLimits
Limits in calculus refer to the value that a function approaches as the input (or sometimes the output) gets arbitrarily close to a certain point. Limits are fundamental to understanding continuity, derivatives, and integrals. In the video, the author discusses limits in the context of evaluating functions at certain points, which is crucial for solving the given problem.
๐Ÿ’กDepression
In the context of the video, 'Depression' seems to be a term used metaphorically or as part of a mathematical concept, possibly related to a function that represents a 'depressed' or lower value. It is not clear from the transcript how 'Depression' is directly related to the main theme, but it suggests that the author might be using it to describe a specific mathematical condition or scenario.
๐Ÿ’กPower Functions
Power functions are a class of functions that have the form f(x) = x^n, where n is a real number. These functions are important in various areas of mathematics and are used in the video to describe certain mathematical relationships. Power functions are a key concept in calculus as they often appear in the study of derivatives and integrals.
๐Ÿ’กExponential Functions
Exponential functions are mathematical functions of the form f(x) = a^x, where a is a constant and x is the variable. They are widely used in many fields of science and engineering to model growth and decay processes. In the video, exponential functions might be part of the problem being solved, and understanding their properties is crucial for accurate calculations in calculus.
๐Ÿ’กProof
Proof in mathematics is a logical demonstration that a statement is true. It involves a series of steps or arguments that lead from premises to a conclusion. In the video, the author is likely to be proving certain properties or solutions related to the integral calculus problem, which is a standard practice in mathematical demonstrations and explanations.
๐Ÿ’กSubstitution
Substitution is a common mathematical technique used to simplify expressions or solve equations by replacing one value or variable with another. In the context of the video, substitution might be used as a method to simplify the integral calculus problem, allowing the author to solve it more easily by changing the variables or expressions involved.
๐Ÿ’กDifferential
A differential is a concept in calculus that represents the rate of change of a function at a particular point. It is the infinitesimal version of the difference quotient, which is used to approximate the derivative of a function. In the video, the author might be using differentials to find the derivative of a function, which is a fundamental operation in integral calculus.
๐Ÿ’กRoot
In mathematics, a root of an equation is a value of the variable that makes the equation true. For example, in a quadratic equation, the roots are the values that satisfy the equation. In the context of the video, the author might be discussing roots in relation to solving quadratic or other types of equations, which is a common application in calculus and algebra.
Highlights

The video discusses integral calculus and its application to solve a specific problem involving knowledge and functions.

The problem involves calculating the integral of a function and understanding its standard form.

The video demonstrates the process of converting the given integral into its standard form for easier proofreading.

The solution process follows a technique similar to previous processes used in the video series.

The video emphasizes the importance of watching all previous videos for a comprehensive understanding of the topic.

The problem involves the concept of 'Should have a great wall' and the exclusion of certain terms in the function.

The video explains how to deal with the power of functions and their integration in the problem-solving process.

The process includes the use of the integration formula and understanding the limits of integration.

The video provides a detailed explanation of the depression function and its second form.

The solution involves the use of the power rule for integration and the concept of limits.

The video demonstrates how to simplify the integral expression and solve for the function's value.

The video explains the concept of the extra element and how to handle it in the problem-solving process.

The solution process involves the use of the chain rule and the concept of limits for integration.

The video provides a clear example of how to solve the problem by applying the knowledge and function of integral calculus.

The video concludes with a summary of the problem-solving process and the final result.

The video encourages viewers to practice the problem-solving techniques discussed for better understanding and application.

The video ends with a call to action for viewers to like, share, and subscribe for more educational content.

Transcripts

Browse More Related Video

INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 14

INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 13

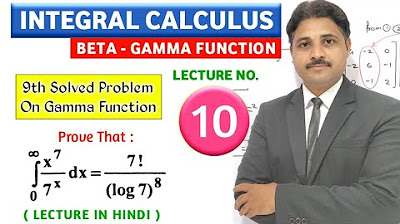
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 10

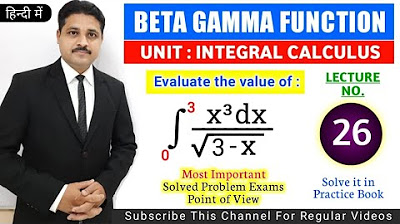
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 26 | BETA GAMMA FUNCTION SOLVED PROBLEM IN HINDI

INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 15

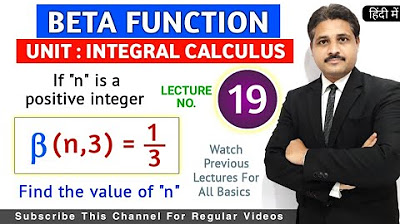
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 19 | BETA FUNCTION SOLVED PROBLEM @TIKLESACADEMY

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
MathematicsIntegral CalculusEducationalProblem SolvingYouTube TutorialMath TipsFunctionsLimitsSeriesEducation