INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 10

TIKLE'S ACADEMY OF MATHS
29 May 202015:47
EducationalLearning
32 Likes 10 Comments

TLDRThe video script discusses the concept of integral calculus, focusing on solving a specific problem involving the integration of a function. The讲师, presumably an educator in mathematics, walks through the process of converting the given integral expression into its exponential form, applying the rules of calculus to find the solution. The video aims to provide a clear understanding of the topic, emphasizing the importance of practice and the application of mathematical concepts to solve real-world problems. The讲师 also encourages viewers to engage with previous videos for a comprehensive understanding of the subject matter.

Takeaways
  • 📘 The video is a lecture on Integral Calculus, specifically focusing on the unit on Integration by Parts and solving a related problem.
  • 🔢 The problem discussed involves integrating a function with respect to x, from negative infinity to positive infinity, with a particular function given in the problem statement.
  • 📚 The lecture builds upon previous lessons, including the basics of integration and function definitions, emphasizing the importance of understanding these concepts.
  • 🧠 The process of solving the problem involves converting the given integral into a form that can be integrated by parts, which requires understanding the function's definition and its properties.
  • 🌐 The video also discusses the concept of limits, particularly lower and upper limits, and how they apply to the integral calculus problem at hand.
  • 📈 The solution to the problem involves identifying and applying the correct formula for the integral, taking into account the specific function and limits provided.
  • 🤔 The lecture emphasizes the importance of practice, encouraging viewers to review previous videos and lessons to solidify their understanding of the material.
  • 📝 The script mentions a link in the video description that directs viewers to all previous lessons on the topic, allowing for easy access to supplementary material.
  • 🎓 The video is part of a series on mathematics, aiming to cover various topics and problems in depth, with an upcoming video promised to cover more columns of numbers.
  • 👍 The video creator encourages viewers to like, share, and subscribe to the channel for more educational content, and to ask questions in the comments section for further clarification.
  • 🔄 The video concludes with a summary of the problem solved and an invitation to the next lecture, reinforcing the key concepts discussed.
Q & A

  • What is the main topic of the video?

    -The main topic of the video is solving a problem related to integral calculus and functions in mathematics.

  • Which specific area of integral calculus is discussed in the video?

    -The video discusses the area of integral calculus involving functions with infinite limits and power functions.

  • What is the integral formula used in the problem?

    -The integral formula used in the problem is ∫nh/12 from ∞ to -x^n/2 * 2dx.

  • What is the significance of the left-hand side and right-hand side in the integral problem?

    -The left-hand side and right-hand side are used to denote the limits of integration in the problem, which are essential for solving the integral.

  • How does the video approach the problem-solving process?

    -The video approaches the problem-solving process by first converting the given integral into its standard form, then applying the function definition, and finally calculating the limits of integration.

  • What is the role of the exponential function in the integral?

    -The exponential function is a part of the integrand, and understanding its behavior is crucial for determining the form of the integral and solving the problem.

  • How does the video ensure the audience understands the problem and its solution?

    -The video ensures understanding by breaking down the problem, explaining each step clearly, and providing a detailed solution to the problem.

  • What is the importance of practicing problems like this in mathematics?

    -Practicing such problems helps in building a strong foundation in calculus, understanding the application of integral formulas, and improving problem-solving skills.

  • What is the next step suggested by the video for further learning?

    -The video suggests practicing all the previous problems and topics covered in the series, and watching the upcoming videos for more complex concepts.

  • How can viewers access the previous videos mentioned in the script?

    -Viewers can access the previous videos by clicking on the link provided in the video description.

Outlines
00:00
📚 Introduction to Integral Calculus

The paragraph introduces the topic of Integral Calculus, welcoming viewers to the Tennis Academy's YouTube channel. It sets the stage for solving a problem involving the integration of a function, emphasizing the importance of understanding the fundamentals of calculus. The speaker also encourages viewers to review previous videos for a better grasp of the concepts discussed.

05:01
🔢 Solving an Integration Problem

This paragraph delves into the process of solving an integration problem, specifically focusing on the function defined as the integral from negative infinity to the power minus x squared, divided by 2dx. The speaker explains the steps to convert the integral into its exponential form, discusses the concept of limits, and how they apply to the problem at hand. The explanation includes the use of mathematical notation and the transformation of expressions to fit the standard form of the exponential function.

10:03
📱 Converting to Phone Format

The speaker describes the process of converting the mathematical problem into a format that can be input into a calculator, emphasizing the importance of understanding the constant and the integration with respect to the function. The paragraph details the steps to convert the integral into a form that can be solved using a calculator, including the handling of limits and the final expression of the integral in terms of the original function.

15:04
🎓 Additional Topics and Practice

The final paragraph touches on additional topics such as the讲师 (lecturer) number 90 and the importance of practicing previous problems. The speaker encourages viewers to like the video, share it with friends, and ask questions in the comments section. The paragraph concludes with a call to action for viewers to subscribe to the channel for more educational content.

Mindmap
Keywords
💡Integral Calculus
Integral calculus is a core concept in mathematics that deals with the study of integrals, which are used to find areas under curves, volumes of solids, and other similar quantities. In the context of the video, integral calculus is the main theme, with the speaker discussing the process of solving problems related to it and the importance of understanding its fundamental concepts and formulas. The video aims to provide a deeper understanding of integral calculus by solving a specific problem and discussing its application.
💡Functions
In mathematics, a function is a relation that pairs each element from a set (the domain) to a unique element in another set (the codomain). Functions are central to the video's content as they are the basis for integral calculus problems. The speaker uses functions to illustrate how integral calculus works, particularly in solving the given problem. Functions are used to define the behavior of variables and are integral to understanding the mathematical relationships and processes being discussed.
💡Limits
Limits in calculus refer to the value that a function or sequence approaches as the input (or index) approaches some value. Limits are fundamental to understanding continuity, derivatives, and integrals. In the video, the concept of limits is crucial for evaluating the integral expressions, especially when dealing with infinity. The speaker emphasizes the importance of calculating limits to solve the integral calculus problem presented.
💡Power Functions
Power functions are a class of functions that have the form f(x) = x^n, where n is a real number. These functions are significant in the video as they appear in the integral calculus problem that the speaker is solving. Understanding the properties and behavior of power functions is essential for comprehending how the integral is evaluated and the techniques used to find the solution.
💡Exponential Functions
Exponential functions are a type of mathematical function that appears in the form f(x) = a^x, where a is a constant and x is the variable. In the context of the video, exponential functions are part of the integral calculus problem. The speaker discusses how to handle these functions when integrating, which is a critical aspect of solving the problem presented in the video.
💡Integration
Integration is the process of finding the integral of a function, which can be thought of as the reverse process of differentiation. It is a fundamental operation in calculus and is the focus of the video. The speaker demonstrates the steps and techniques involved in integrating a given function, which is the core activity of the video and is essential for solving the problem at hand.
💡Infinite Series
An infinite series is the sum of an infinite sequence of numbers. It is a mathematical concept that is relevant in the video, especially when dealing with functions that extend to infinity. The speaker may discuss the convergence or divergence of series, which is crucial for understanding the behavior of certain functions and integrals in calculus.
💡Limits of Integration
The limits of integration refer to the specific intervals over which an integral is computed. In the video, the speaker emphasizes the importance of correctly identifying these limits when setting up and solving integral calculus problems. Understanding the limits is essential for obtaining accurate results from the integration process.
💡Antiderivatives
Antiderivatives, also known as indefinite integrals, are functions that have a derivative equal to a given function. In the context of the video, finding the antiderivative is a key step in solving the integral calculus problem. The speaker likely explains how to find the antiderivative of the functions involved and how this relates to the concept of integration.
💡Evaluation
In mathematics, evaluation refers to the process of determining the value of an expression or function for specific input values. In the video, evaluation is a crucial step in solving the integral calculus problem, as it involves calculating the value of the integral and the antiderivative at the limits of integration. The speaker demonstrates how to evaluate the expressions to find the solution to the problem.
Highlights

Welcome to the Tennis Academy of Mathematics on YouTube, where today's video will delve into the topic of Integral Calculus.

The video begins with a recap of previous problems and concepts, emphasizing the continuity of learning.

An integral calculus problem involving 'Knowledge V' and 'Function' is introduced, setting the stage for today's lesson.

The problem presented involves calculating the integral from a given function, with a specific focus on the left-hand side and the right-hand side of the expression.

The process of solving the integral calculus problem is explained, highlighting the use of previous knowledge and techniques.

The importance of understanding the definition of functions in integral calculus is stressed, as it is key to solving the problem.

The video demonstrates the conversion of the given integral into its exponential form, a crucial step in the problem-solving process.

The concept of limits in integral calculus is discussed, with a focus on how to check and convert them into the required form.

The video provides a clear example of how to convert the integral expression into its final form, using the principles of integral calculus.

The practical application of the integral calculus problem is shown, with a step-by-step guide on how to approach and solve it.

The video emphasizes the importance of practice, providing a link in the description to previous videos for further study.

The presenter encourages viewers to like, share, and subscribe to the channel for more educational content on mathematics.

The video concludes with a summary of the integral calculus problem and an invitation to the next video for further learning.

Transcripts

Browse More Related Video

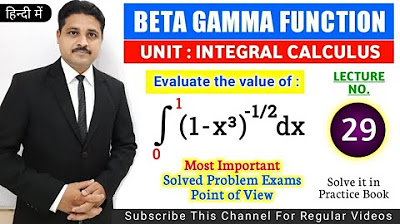
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 29 | BETA GAMMA FUNCTION SOLVED PROBLEM IN HINDI

INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 11

INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 14

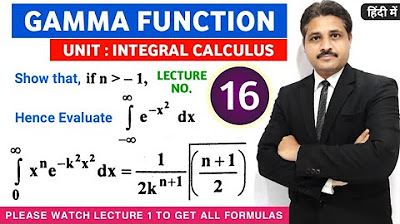
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 16

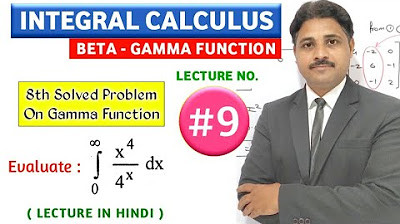
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 9

INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 15

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
IntegralCalculusMathematicsEducationInfiniteSeriesExponentialGrowthYouTubeChannelHelloHelloEveryoneTennisAcademyProblemSolvingMathLecture