EASIEST question on the International Physics Olympiad?

ZPhysics
8 Apr 202203:06
EducationalLearning
32 Likes 10 Comments

TLDRThe video script explores a fundamental concept of quantum mechanics: the minimum energy of a particle trapped in a one-dimensional box with infinite potential barriers. It explains how the particle, behaving as a de Broglie standing wave, has a non-zero minimum energy due to quantum weirdness, unlike classical physics. The script delves into the formula for calculating this energy, which involves Planck's constant, the particle's momentum, and the box's length, emphasizing the intriguing zero-point energy phenomenon.

Takeaways
  • 🌏 The International Physics Olympiad is a prestigious physics competition.
  • πŸ“š The script discusses an easy question from the 2021 Olympiad held in Lithuania.
  • πŸš€ The scenario involves a particle trapped in a one-dimensional box with infinite potential barriers and zero potential inside.
  • 🌌 The particle can be described as a de Broglie standing wave with nodes at the box edges.
  • πŸ”¬ The de Broglie wavelength formula is given by \( \lambda = \frac{h}{p} \), where \( h \) is Planck's constant and \( p \) is the particle's momentum.
  • πŸ“ For the lowest energy state, half of the wavelength is contained within the box, implying \( \lambda = 2l \).
  • πŸ” The momentum of the particle can be rearranged from the wavelength formula to \( p = \frac{h}{2l} \).
  • ⚑ The minimum energy of the particle is purely kinetic, as the potential inside the box is zero.
  • πŸ“‰ The minimum kinetic energy is calculated using the formula \( E = \frac{p^2}{2m} \), where \( m \) is the mass of the particle.
  • 🧲 Substituting the expression for \( p \) into the kinetic energy formula yields \( E_{min} = \frac{h^2}{8ml^2} \).
  • πŸŒ€ Quantum mechanics introduces a concept of zero-point energy, which is different from classical physics where an object could have zero energy.
  • πŸ” The script invites curiosity about why zero-point energy is not observable and suggests further exploration in a related video.
Q & A

  • What is the International Physics Olympiad?

    -The International Physics Olympiad is one of the most challenging and prestigious physics exams in the world, attracting top high school students from various countries to compete in solving complex physics problems.

  • What is the concept of a particle trapped in a one-dimensional box with infinite potential?

    -In quantum mechanics, a particle trapped in a one-dimensional box with infinite potential means that the particle is confined to a specific region with zero potential inside the box and cannot escape because the potential outside the box is infinitely large.

  • How is the De Broglie wavelength of a particle defined?

    -The De Broglie wavelength of a particle is defined by the equation Ξ» = h / p, where Ξ» is the wavelength, h is Planck's constant, and p is the momentum of the particle.

  • What is the significance of the nodes in the De Broglie standing wave?

    -The nodes in the De Broglie standing wave represent points where the wave function is zero, corresponding to the boundaries of the box. These nodes indicate that the particle cannot exist outside the box, thus defining the quantum states of the particle.

  • How is the minimum energy of a particle in a box determined?

    -The minimum energy of a particle in a box is determined by the lowest possible energy state, where half of the De Broglie wavelength fits within the length of the box. This leads to the equation E_min = h^2 / (8 * m * L^2), where E_min is the minimum energy, h is Planck's constant, m is the mass of the particle, and L is the length of the box.

  • Why is the wavelength of the particle equal to 2L in this context?

    -The wavelength of the particle is equal to 2L because, in the lowest energy state, half of the wavelength fits within the length of the box, meaning the full wavelength is twice the length of the box.

  • What role does momentum play in determining the minimum energy of the particle?

    -Momentum plays a crucial role in determining the minimum energy of the particle. By rearranging the De Broglie wavelength equation, the momentum is found to be p = h / (2L), which is then used to calculate the kinetic energy (and thus the minimum energy) of the particle.

  • Why does the kinetic energy formula involve the square of the momentum?

    -The kinetic energy formula involves the square of the momentum because kinetic energy (K) is defined as K = p^2 / (2m), where p is the momentum and m is the mass of the particle. This relationship comes from classical mechanics and is adapted in quantum mechanics to describe the energy of the particle in the box.

  • What does the expression for the minimum energy tell us about quantum mechanics?

    -The expression for the minimum energy, E_min = h^2 / (8 * m * L^2), highlights a fundamental principle of quantum mechanics: even at the lowest energy state, a particle cannot have zero energy. This non-zero minimum energy is a manifestation of the quantum phenomenon known as zero-point energy.

  • Why can't we observe the zero-point energy in classical mechanics?

    -In classical mechanics, an object can theoretically have zero energy, meaning it can be at rest with no kinetic or potential energy. However, in quantum mechanics, due to the wave nature of particles, zero-point energy is always present, and this energy is not directly observable in the classical sense because it is inherent to the quantum state of the system.

Outlines
00:00
πŸ“š Introduction to Quantum Mechanics and the Particle in a Box Problem

The paragraph introduces the International Physics Olympiad and delves into a simplified example from the 2021 competition in Lithuania. It discusses a particle trapped in a one-dimensional box with infinite potential barriers and zero potential inside, which can be modeled as a de Broglie standing wave. The concept of the particle's minimum energy state is explored, using the relationship between wavelength, Planck's constant, and the particle's momentum. The paragraph explains how to derive the minimum energy of the particle using the kinetic energy formula and emphasizes the quantum mechanical phenomenon of zero-point energy, which is different from classical physics where an object could theoretically have zero energy.

Mindmap
Keywords
πŸ’‘International Physics Olympiad
The International Physics Olympiad (IPhO) is a highly prestigious and challenging physics competition for high school students worldwide. It tests deep knowledge and problem-solving skills in various areas of physics. In the context of the video, it is described as one of the most difficult physics exams, indicating the high level of complexity of the problems presented, including the one being discussed from the 2021 Olympiad in Lithuania.
πŸ’‘Particle in a One-Dimensional Box
This is a fundamental problem in quantum mechanics where a particle is confined to move within a one-dimensional box with infinitely high potential walls on either side, meaning the particle cannot escape. The concept is used in the video to explore the quantum mechanical behavior of particles, emphasizing how they differ from classical expectations.
πŸ’‘De Broglie Wavelength
The De Broglie wavelength is a concept in quantum mechanics that describes the wave-like behavior of particles. It is given by the formula Ξ» = h/p, where h is Planck's constant and p is the momentum of the particle. In the video, it is used to describe the standing wave nature of the particle trapped in the one-dimensional box, illustrating the quantum mechanical property of wave-particle duality.
πŸ’‘Standing Wave
A standing wave is a wave that remains stationary within a medium, with specific points (nodes) that do not move. In the context of the video, the particle inside the box is described as a De Broglie standing wave, with nodes at the edges of the box, demonstrating the wave-like properties of particles in quantum mechanics.
πŸ’‘Planck's Constant
Planck's constant (h) is a fundamental physical constant that plays a crucial role in quantum mechanics, particularly in the relationship between energy and frequency of a photon (E = hΞ½) and in defining the De Broglie wavelength. The video uses Planck's constant in calculating the momentum and minimum energy of the particle, highlighting its significance in quantum mechanical equations.
πŸ’‘Momentum
Momentum is a physical quantity that represents the product of a particle's mass and velocity. In the video, momentum is used to derive the minimum energy of the particle in the box, using the De Broglie wavelength to express momentum as p = h/2L. This showcases the interplay between wave properties and momentum in quantum mechanics.
πŸ’‘Minimum Energy
Minimum energy refers to the lowest possible energy state that a quantum system, such as a particle in a box, can occupy. In the video, this is calculated using the derived momentum, resulting in an expression that shows the quantum mechanical nature of particles, where they possess a non-zero energy even in their lowest energy state, unlike classical systems.
πŸ’‘Kinetic Energy
Kinetic energy is the energy a particle possesses due to its motion, calculated as (p^2)/2m, where p is the momentum and m is the mass. The video explains that the particle's energy in the box is purely kinetic, as the potential energy inside the box is zero. This kinetic energy corresponds to the minimum energy state derived in the discussion.
πŸ’‘Zero Point Energy
Zero point energy is the lowest possible energy that a quantum mechanical system can have, even when not in motion. In the video, the concept of zero point energy is used to explain why a particle in a box cannot have zero energy, highlighting the 'quantum weirdness' where even at the lowest energy state, a quantum particle still possesses some energy.
πŸ’‘Quantum Mechanics
Quantum mechanics is the branch of physics that deals with the behavior of particles on very small scales, such as atoms and subatomic particles. It introduces concepts like wave-particle duality, quantization of energy, and the uncertainty principle. The entire video revolves around quantum mechanical principles, illustrating them through the example of a particle in a one-dimensional box.
Highlights

The International Physics Olympiad is one of the most prestigious physics exams globally.

The 2021 Olympiad in Lithuania featured a question about a particle trapped in a one-dimensional box with infinite potential barriers.

The particle can be described as a de Broglie standing wave with nodes at the box edges.

The minimum energy of the particle can be determined using quantum mechanics principles.

De Broglie wavelength is given by the expression Ξ» = h/p, where h is Planck's constant and p is the particle's momentum.

The lowest energy state occurs when half of the wavelength is contained within the box's length, making the wavelength equal to 2l.

Momentum p can be rearranged from the equation 2l = h/p to p = h/2l.

Kinetic energy inside the box is the only form of energy considered, as the potential inside is zero.

The minimum energy E_min is given by the expression p^2/2m, where m is the mass of the particle.

The expression for minimum energy can be derived as E_min = h^2/(8ml^2).

Quantum mechanics introduces the concept of zero-point energy, which is different from classical mechanics.

The video explores why zero-point energy is not observable in everyday life.

The problem demonstrates the quantum weirdness of particles having non-zero energy even at absolute zero temperature.

Understanding the minimum energy of a particle in a box is crucial for grasping quantum mechanics fundamentals.

The question from the Olympiad challenges students to apply quantum principles to solve a physical problem.

The solution process involves mathematical manipulation of quantum formulas to find the minimum energy.

The video serves as an educational resource to explain complex quantum concepts in a simplified manner.

The concept of de Broglie waves is fundamental to understanding quantum behavior of particles.

The video encourages curiosity about the invisible aspects of quantum mechanics, such as zero-point energy.

Transcripts

Browse More Related Video

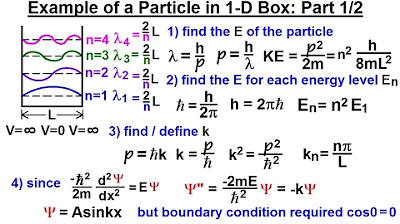
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (19 of 92) Particle in 1-D Box: Example 1/2

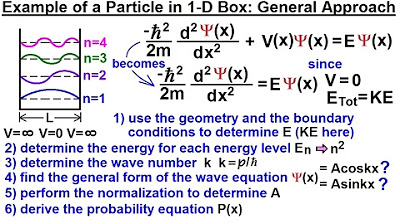
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (18 of 92) Particle in 1-D Box: Gen. Appr.

23. Quantum Mechanics V: Particle in a Box

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (29 of 92) Expect. Value Momentum=? 1-D Box

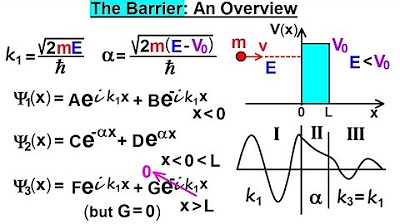
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (77 of 92) The Barrier: An Overview

22. Quantum mechanics IV: Measurement theory, states of definite energy

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Physics OlympiadQuantum MechanicsZero-Point EnergyDe Broglie WavesParticle EnergyInfinite PotentialOne-Dimensional BoxPlanck's ConstantKinetic EnergyEducational ContentScience Enthusiasts