AP Calculus BC Lesson 10.8
TLDRThis video lesson delves into the Ratio Test, a method for determining the convergence or divergence of infinite series with non-zero terms. It explains the test's conditions and its inconclusive nature when the limit equals one. The video also covers the concept of factorials and their simplification, crucial for Ratio Test problems. Several examples illustrate how to apply the test, including series involving exponential and factorial terms. The lesson concludes with a series of questions to test understanding of the Ratio Test and its application to various series.
Takeaways
- 📚 The ratio test is used to determine the convergence or divergence of a series with non-zero terms by examining the limit of the ratio of consecutive terms as n approaches infinity.
- 🎓 If the limit of the ratio is less than one, the series converges; if greater than one, it diverges; and if equal to one, the ratio test is inconclusive.
- 📈 The script provides a detailed explanation of how to handle factorials in ratio test problems, including their definition and calculation using a graphing calculator.
- 🌟 The script demonstrates the simplification of factorial expressions in the context of the ratio test through several examples.
- 📝 The process of evaluating the convergence or divergence of a series involves finding the limit of the ratio of the (n+1)th term to the nth term and applying the ratio test criteria.
- 🔢 The script walks through the evaluation of specific series, such as those involving powers of 5, 4, and 2, and their respective factorials.
- 🚫 The ratio test is not always conclusive; it may be necessary to use other tests, such as the geometric series test or limit comparison, for a definitive answer.
- 🤔 The script emphasizes the importance of simplifying expressions within the absolute value during the ratio test to accurately determine the limit.
- 📊 The script provides a step-by-step approach to solving ratio test problems, including the cancellation of common terms in the numerator and denominator.
- 📋 The script concludes with a series of questions and answers that apply the ratio test to various series, highlighting the test's application and limitations.
Q & A
What does the ratio test state about a series with non-zero terms?
-The ratio test states that for a series with non-zero terms, if the limit as n approaches infinity of the absolute value of the ratio of the (n+1)th term to the nth term is less than 1, the series converges. If this limit is greater than 1, the series diverges. If the limit is equal to 1, the ratio test is inconclusive.
How are factorials related to ratio test problems?
-Factorials often appear in ratio test problems because they represent the product of an integer and all the positive integers below it. They can be evaluated on a graphing calculator and are commonly used in simplifying or evaluating expressions within the context of the ratio test.
What is the significance of the expression 5^(n+1)/n! in the context of the ratio test?
-The expression 5^(n+1)/n! represents the (n+1)th term of a series where the nth term is 5^n/n!. This is used to find the limit as n approaches infinity for the ratio of consecutive terms, which helps determine the convergence or divergence of the series according to the ratio test.
How does the ratio test help in determining the convergence or divergence of a series?
-The ratio test provides a method to determine if a series converges or diverges by comparing the ratio of consecutive terms as the number of terms approaches infinity. If the limit of this ratio is less than 1, the series converges; if it's greater than 1, it diverges; and if it's equal to 1, the test is inconclusive.
What is the role of simplification in applying the ratio test?
-Simplification is crucial in applying the ratio test as it helps in evaluating the limit of the ratio of consecutive terms. By simplifying expressions involving factorials and other mathematical operations, one can more easily identify whether the limit condition for convergence or divergence is met.
How does the ratio test apply to the series ∑(5^n / n!) from n=1 to ∞?
-For the series ∑(5^n / n!), the ratio test involves finding the limit of the absolute value of (5^(n+1) / (n+1)!) / (5^n / n!) as n approaches infinity. The simplification of this expression leads to the limit being less than 1, indicating that the series converges according to the ratio test.
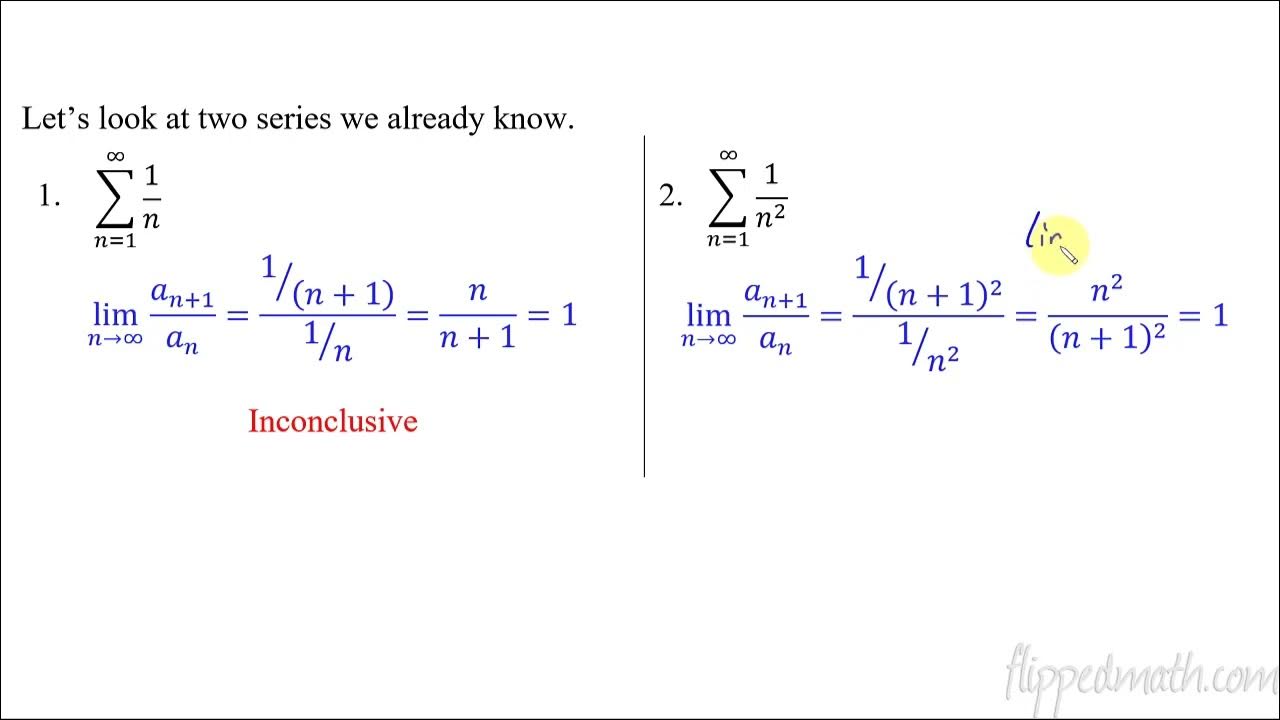
What happens when the limit of the ratio in the ratio test is equal to 1?
-When the limit of the ratio is equal to 1 as n approaches infinity, the ratio test is inconclusive. This means that the test does not provide a definitive answer about whether the series converges or diverges, and another method must be used to determine the series' behavior.
How can one evaluate 5! on a graphing calculator?
-To evaluate 5! on a graphing calculator, you would press the 'math' button, scroll over to 'probability', and then scroll down to or press the button for '4', which corresponds to the factorial function. Then, you would enter the number 5 and the calculator would evaluate 5! for you.
What is the general process for using the ratio test on a series?
-The general process involves identifying the general term a_n of the series, finding the term a_(n+1), and then calculating the limit as n approaches infinity of the absolute value of the ratio (a_(n+1) / a_n). If this limit is less than 1, the series converges; if greater than 1, it diverges; and if equal to 1, the test is inconclusive.
What is the significance of the ratio test being inconclusive?
-An inconclusive ratio test means that the limit of the ratio of consecutive terms as n approaches infinity is equal to 1. This does not provide enough information to determine whether the series converges or diverges, so alternative tests or methods must be used to analyze the series further.
Outlines
📚 Introduction to the Ratio Test
This paragraph introduces the concept of the Ratio Test, a mathematical tool used to determine the convergence or divergence of an infinite series. It explains the basic principle of the test, which involves taking the limit of the ratio of consecutive terms of the series as the term number approaches infinity. The paragraph also notes that the Ratio Test is often applied to series involving factorials and provides a brief explanation of what factorials are, including how to calculate them using a graphing calculator. The importance of simplifying factorials in Ratio Test problems is emphasized.
📈 Simplifying Factorials and Evaluating Ratios
The paragraph delves into the process of simplifying factorial expressions and evaluating the ratios of consecutive terms in a series. It provides examples of how to simplify factorials by canceling out common terms in the numerator and denominator. The paragraph also demonstrates how to evaluate the ratio of terms like 6 factorial over 5 factorial and generalizes this to the case of (n+1) factorial over n factorial. The concept is further extended to more complex examples involving terms like (3n+3) factorial over (3n) factorial, highlighting the cancellation of terms and the simplification process.
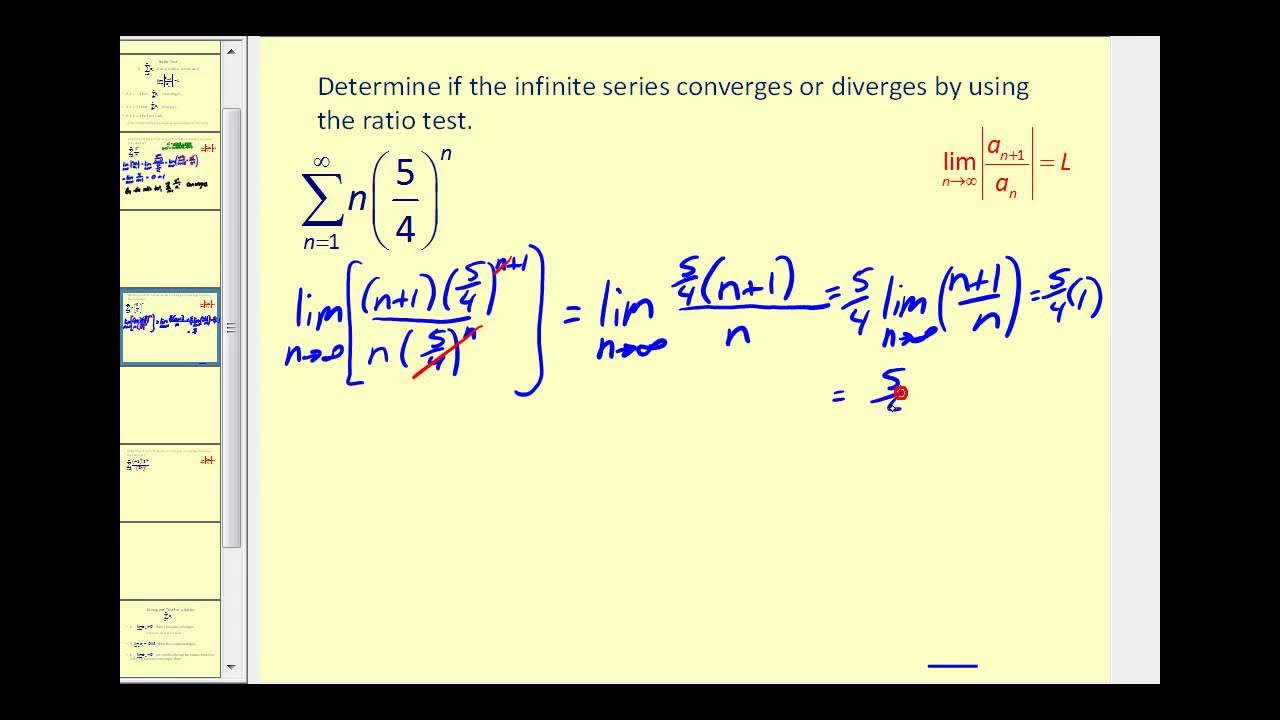
🔢 Applying the Ratio Test to Determine Convergence
This section applies the Ratio Test to specific series to determine their convergence or divergence. It explains the process of finding the limit of the ratio of consecutive terms and how this limit relates to the series' convergence or divergence. The paragraph provides detailed examples of series with different powers of n and factorials in the denominator, showing how to simplify the expressions and evaluate the limit. It concludes with the application of the Ratio Test to series involving exponential functions and how the test's outcome indicates the series' convergence or divergence.
📊 Analyzing Series Convergence with the Ratio Test
The paragraph continues the analysis of series convergence using the Ratio Test. It presents a methodical approach to determining the convergence of series by evaluating the limit of the ratio of consecutive terms. The paragraph includes examples of series with different algebraic expressions and explains how to handle the simplification and limit evaluation process. It also discusses the implications of the limit being greater than one, less than one, or equal to one in the context of the Ratio Test and series convergence.
🧠 Understanding the Limit Comparison and Geometric Series Tests
This paragraph explores alternative methods for determining series convergence beyond the Ratio Test, specifically the Limit Comparison Test and the Geometric Series Test. It explains the conditions under which these tests can be applied and provides examples of series that might be more efficiently analyzed using these methods. The paragraph also compares the behavior of series to established divergent or convergent series to draw conclusions about the original series' convergence properties.
📈 Applying the Ratio Test to Specific Series
The paragraph focuses on applying the Ratio Test to specific series to demonstrate its utility in determining convergence. It presents a series of examples where the test is used to show that certain series converge, while others are shown to diverge. The paragraph also discusses the limitations of the Ratio Test when the limit equals one, making it inconclusive. The examples provided cover a range of scenarios, including series with polynomial and exponential terms, and illustrate the step-by-step process of using the Ratio Test to assess series convergence.
🤔 Evaluating Inequalities for Series Divergence
The final paragraph addresses the application of the Ratio Test to series with specific algebraic expressions, focusing on evaluating inequalities that imply series divergence. It outlines the process of setting up the limit for the ratio of consecutive terms and simplifying the expression to determine the series' behavior. The paragraph concludes by identifying the correct inequality that implies the series diverges based on the established limit conditions of the Ratio Test.
Mindmap
Keywords
💡Ratio Test
💡Factorials
💡Convergence
💡Divergence
💡Infinite Series
💡Limit
💡Exponential Functions
💡Simplification
💡Graphing Calculator
💡Absolute Value
Highlights
Exploring the Ratio Test for series convergence.
Series with non-zero terms, if the limit of (|a_(n+1)/a_n|) < 1, it converges; if > 1, it diverges.
Ratio Test is inconclusive if the limit of (|a_(n+1)/a_n|) = 1.
Ratio Test problems often involve factorials, which are the product of an integer and all positive integers below it.
Example: Simplify 6!/5! to 6, showing how factorials can be canceled out.
Detailed explanation of how to handle factorials in the Ratio Test.
Demonstration of evaluating (n+1)!/n! and its simplification.
Example: Simplification of (3n+3)!/(3n)! leading to 3n+3.
Method to determine the convergence or divergence of a series using the Ratio Test.
Example of a series involving 5^n/n! and its convergence using the Ratio Test.
Explanation of how to handle exponents and factorials in the Ratio Test.
Example of a series with 4^(n+1)/(n! * 3^n) and its convergence.
Rewriting series to simplify the Ratio Test process, such as 2N!/(5^2N).
Series with 2N!/(5^2N) diverges by the Ratio Test.
Using the Ratio Test to compare different series for convergence or divergence.
Example of a series with n!/7^n and its divergence using the Ratio Test.
Explanation of when to use the geometric series test versus the Ratio Test.
Series with 5^(n+2)/4^n may look like a Ratio Test problem but is better solved with the geometric series test.
Demonstration of using limit comparison to determine the behavior of a series.
Example of a series with (rad n)/(n - pi) and its divergence using limit comparison.
The Ratio Test is inconclusive for a p-series with p > 1, even though the series converges.
Series with (n+1)^3/(3^(n+1)) converges by the Ratio Test.
Analysis of a series with 3n * 6^n / e^n and its divergence using the Ratio Test.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: