Ratio Test
TLDRThis video tutorial delves into the Ratio Test, a mathematical method for determining the convergence of infinite series. It explains the fundamental principle that if the limit of the ratio of consecutive terms' absolute values is less than 1, the series converges absolutely. If the limit is greater than 1 or diverges to infinity, the series diverges. A limit equal to 1 results in an inconclusive test, necessitating alternative methods. The script proceeds with several examples, demonstrating the application of the Ratio Test to various series, including those involving exponential and factorial terms, and concludes with scenarios where the test is inconclusive, thus requiring further analysis.
Takeaways
- 📚 The ratio test is a method to determine the convergence of an infinite series by examining the limit of the ratio of consecutive terms.
- 🔍 If the limit l is less than 1, the series is absolutely convergent.
- 🔎 If the limit l is greater than 1 or tends to infinity, the series is divergent.
- 🤔 When the limit l equals 1, the ratio test is inconclusive, and other tests may be needed to determine convergence.
- 🌐 The script provides an example using the series 3^n / n! and shows that it converges absolutely by applying the ratio test.
- 📉 For the series n / 4^n, the ratio test reveals that it converges.
- 📈 In the series 3^{n+2} * n^2 / 4^n, the ratio test indicates absolute convergence.
- 🔮 The series n^n / n! is shown to diverge by using the ratio test and applying L'Hôpital's rule.
- 🤹♂️ The series 2^{4n+1} / n^n is demonstrated to converge through the ratio test, with the limit approaching 0.
- 💭 For the alternating series (-1)^{n+1} * (n+2) / √n, the ratio test is inconclusive, suggesting further analysis is needed.
- 📝 The script emphasizes the importance of simplifying expressions and applying L'Hôpital's rule when necessary to apply the ratio test effectively.
Q & A
What is the basic idea behind the ratio test for series convergence?
-The ratio test involves taking the limit as n approaches infinity of the absolute value of the ratio of the (n+1)th term to the nth term of a series. If this limit l is less than 1, the series is absolutely convergent. If l is greater than 1 or approaches infinity, the series is divergent. If l equals 1, the test is inconclusive.
How is the ratio test applied to the series of 3^n over n factorial?
-The ratio test is applied by calculating the limit of (3^(n+1)/(n+1)!) divided by (3^n/n!) as n approaches infinity. Simplifying this expression leads to the limit of 3/(n+1), which approaches 0, indicating the series is absolutely convergent.
What does the ratio test indicate for the series n over 4^n?
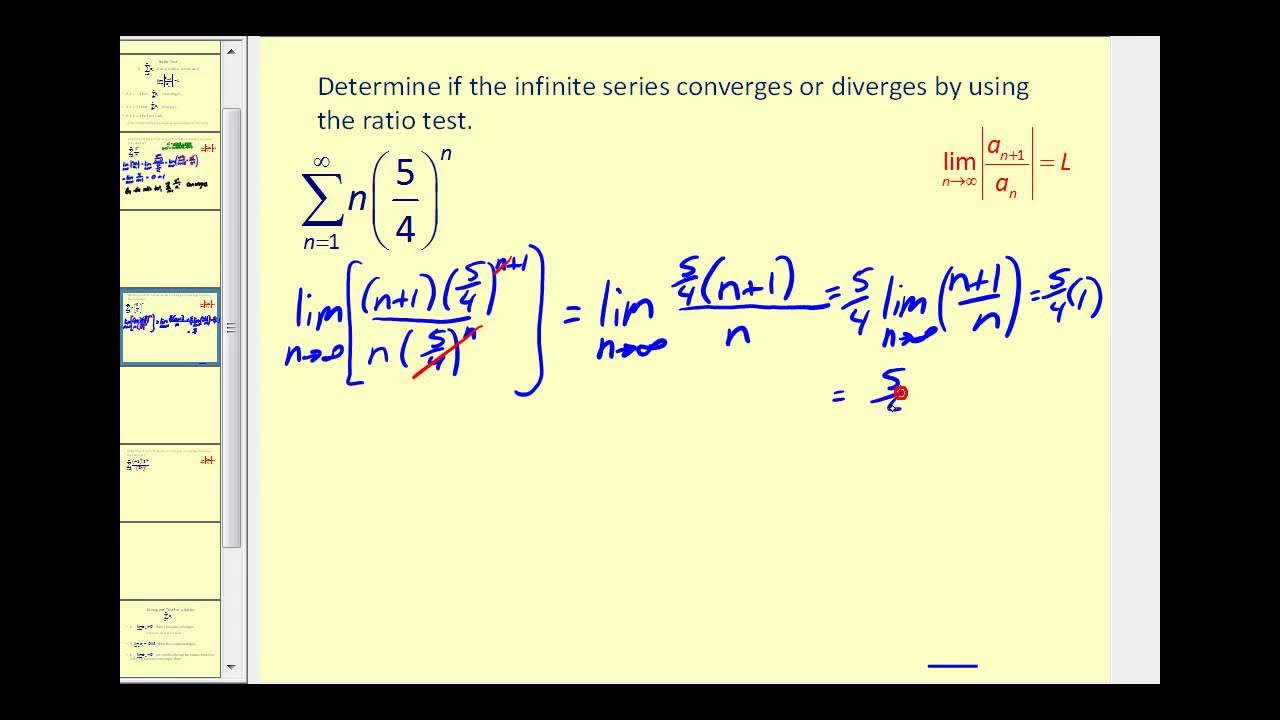
-Applying the ratio test to this series involves finding the limit of ((n+1)/4^(n+1)) divided by (n/4^n) as n approaches infinity. This simplifies to 1/4, which is less than 1, indicating the series converges.
How does the ratio test determine the convergence of the series 3^(n+2) * n^2 over 4^n?
-The ratio test is used by finding the limit of ((3^(n+1) * (n+1)^2) / 4^(n+1)) divided by ((3^n * n^2) / 4^n) as n approaches infinity. After simplification, the limit is 3/4, which is less than 1, indicating the series is absolutely convergent.
What happens when applying the ratio test to the series n^n over n factorial?
-The limit of ((n+1)^(n+1) / (n+1)!) divided by (n^n / n!) as n approaches infinity simplifies to e, which is greater than 1. This means the series diverges according to the ratio test.
How does the ratio test apply to the series 2^(4n+1) over n^n?
-The ratio test is used by calculating the limit of ((n+1)^(n+1) * 2^4) divided by (n^n * 2^(4n)) as n approaches infinity. The limit converges to 16/e, which is less than 1, indicating the series converges.
What is the result of applying the ratio test to the series -1^(n+1) * (n+2) / sqrt(n)?
-Since it's an alternating series, the limit of ((n+3) / sqrt(n+1)) divided by ((n+2) / sqrt(n)) as n approaches infinity is 1. A limit of 1 from the ratio test is inconclusive, so further tests are needed to determine convergence or divergence.
Why does the ratio test sometimes give an inconclusive result?
-The ratio test is inconclusive when the limit of the ratio of successive terms equals 1. In such cases, the test does not provide enough information to determine if the series converges or diverges, and other tests may be required.
What is the significance of the limit being less than 1 in the ratio test?
-If the limit of the ratio of the absolute value of successive terms is less than 1, it indicates that the terms of the series are getting smaller at a rate that ensures the series will sum to a finite value, meaning the series is absolutely convergent.
Can the ratio test be applied to any series, or are there exceptions?
-The ratio test is not universally applicable to all series. It is particularly useful for series where the terms are not necessarily decreasing but where the ratio of successive terms can be evaluated. However, for series that do not fit this pattern, other tests may be more appropriate.
Outlines
📚 Introduction to Ratio Test for Series Convergence
This paragraph introduces the concept of the ratio test for determining the convergence of an infinite series. It explains that if the limit of the absolute value of the ratio of consecutive terms (a_(n+1)/a_n) approaches a value less than 1 as n goes to infinity, the series is absolutely convergent. Conversely, if this limit is greater than 1 or tends towards infinity, the series is divergent. A limit equal to 1 results in an inconclusive test, necessitating alternative methods for convergence assessment. The paragraph sets the stage for working through specific examples to apply the ratio test.
🔍 Applying the Ratio Test to a Series with 3^n/n!
The second paragraph delves into applying the ratio test to the series 3^n/n!. It defines the sequence terms a_n and a_(n+1), then calculates the limit of the ratio a_(n+1)/a_n as n approaches infinity. By simplifying the expression and showing that the limit tends to zero (which is less than 1), the paragraph concludes that the series converges absolutely. This example illustrates the step-by-step process of using the ratio test on a specific series.
📉 Ratio Test on n/4^n Series and Simplification
In this paragraph, the ratio test is applied to the series n/4^n. The process involves rearranging terms and simplifying the expression for the limit of the ratio of consecutive terms. The simplification leads to the conclusion that the limit is 1/4, which is less than 1, indicating that the series converges. The explanation includes a step-by-step simplification process and the rationale behind each step.
🔢 Complex Ratio Test Application with 3^(n+2)n^2/4^n
The paragraph presents a more complex example of the ratio test, using the series 3^(n+2)n^2/4^n. It guides through the process of finding the limit of the ratio of consecutive terms, including separating and simplifying the expression. The limit is eventually shown to be 3/4, which is less than 1, thus indicating the series is absolutely convergent. The explanation highlights the importance of careful simplification and the application of the ratio test in determining convergence.
🌐 Exploring Series Convergence with n^n/n!
This paragraph examines the convergence of the series n^n/n! using the ratio test. It simplifies the expression for the limit of the ratio of consecutive terms and applies l'Hôpital's rule to find that the limit is e, which is greater than 1. Since e is the base of the natural logarithm and exceeds 1, the series is determined to be divergent. The explanation provides a clear demonstration of using l'Hôpital's rule in conjunction with the ratio test for series analysis.
📘 Further Ratio Test Examples and Their Analysis
The final paragraph discusses additional examples of applying the ratio test, including the series 2^(4n+1)/n^n and (-1)^(n+1)(n+2)/√n. It explains the simplification process for each series and determines their convergence or divergence based on the ratio test. The explanation includes the use of limits and the significance of the results in understanding the behavior of the series as n approaches infinity.
Mindmap
Keywords
💡Ratio Test
💡Convergent Series
💡Divergent Series
💡Inconclusive
💡Factorial
💡Limit
💡Absolute Value
💡L'Hôpital's Rule
💡Exponential Function
💡Natural Logarithm
Highlights
The ratio test determines if a series converges absolutely, diverges, or is inconclusive by taking the limit of the absolute value of the ratio of consecutive terms.
If the limit of the ratio test is less than 1, the series converges absolutely.
If the limit of the ratio test is greater than 1 or goes to infinity, the series diverges.
If the limit of the ratio test is equal to 1, the test is inconclusive.
Example: For the series 3^n/n!, the ratio test shows that the series converges absolutely.
Example: For the series n/4^n, the ratio test shows that the series converges.
Example: For the series 3^(n+2) * n^2 / 4^n, the ratio test shows that the series converges absolutely.
Example: For the series n^n / n!, the ratio test shows that the series diverges.
Example: For the series 2^(4n+1) / n^n, the ratio test shows that the series converges.
Example: For the alternating series (-1)^(n+1) * (n+2) / sqrt(n), the ratio test is inconclusive.
The process involves defining a_n and a_(n+1) and then simplifying the ratio a_(n+1) / a_n.
For factorials, use the property n! = n * (n-1)!, which helps in canceling terms.
When simplifying powers, separate and factor terms to cancel and reduce the expression.
For limits involving n, understand that terms like 1/n approach zero as n approaches infinity.
L'Hôpital's rule can be used to resolve limits that result in indeterminate forms.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: