Ratio Test
TLDRThis educational video explores the Ratio Test, a method for determining the convergence of infinite series, particularly those involving factorials. The script begins by explaining the concept of factorials, their combinatorial significance, and their common values. It then introduces the Ratio Test, demonstrating how to apply it to series with factorial components. The video provides examples, including a p-series, to illustrate the test's application, showing when the series converges absolutely, diverges, or when the test is inconclusive. The presenter also discusses the limitations of the Ratio Test and when to use alternative methods, promising further exploration of the topic in subsequent videos.
Takeaways
- 📚 The video introduces the ratio test, a mathematical method used to determine the convergence of a series.
- 🔢 The concept of factorial is discussed, explaining its definition and how to calculate it for different values.
- ❗ The significance of the exclamation point in mathematics, denoting factorial, is clarified for those unfamiliar with it.
- 📉 The video explains the factorial of numbers like 2, 3, 4, and 5, and how to simplify expressions involving factorials.
- 🔄 The cancellation of terms in factorial expressions, such as \( n! / (n+1)! \), is shown to simplify to \( 1/(n+1) \).
- 🎓 The combinatorial meaning of factorial is touched upon, relating it to the number of unique arrangements of items.
- 🧩 The video establishes that 0! equals 1, which is important for understanding factorial in series calculations.
- 🔍 The ratio test is applied to series, especially those involving factorials, with a step-by-step explanation of the process.
- 📉 The outcome of the ratio test is explained: if the limit L is less than 1, the series converges absolutely; if greater than 1, it diverges; and if equal to 1, the test is inconclusive.
- 📈 The ratio test is particularly useful for series that resemble p-series, which are series with polynomial terms in the numerator and denominator.
- 📚 Examples are provided to demonstrate the application of the ratio test, including scenarios where the test is conclusive and inconclusive.
Q & A
What is the ratio test used for in mathematics?
-The ratio test is used to determine whether an infinite series converges or diverges. It involves taking the limit of the absolute value of the ratio of consecutive terms of the series as n approaches infinity.
What is the factorial function denoted by an exclamation point (e.g., 5!)?
-The factorial function, denoted by an exclamation point, is the product of all positive integers up to a given number. For example, 5! = 5 × 4 × 3 × 2 × 1.
What is the significance of 0! in terms of combinatorial meaning?
-According to the script, 0! is significant because it represents the number of ways to arrange zero items, which by definition is one, as there is only one way to arrange nothing.
How is the ratio test applied to a series with factorials in it?
-The ratio test is applied by considering the limit as n approaches infinity of the absolute value of the ratio of the (n+1)th term to the nth term of the series, which should include factorials if the series has them.
What is the result of the ratio test if the limit L is less than one?
-If the limit L is less than one, the series converges absolutely, meaning it sums to a finite value.
What happens if the limit L from the ratio test is greater than one?
-If the limit L is greater than one, the series diverges, which means it does not sum to a finite value.
What does it mean if the limit L from the ratio test is equal to one?
-If the limit L is equal to one, the ratio test is inconclusive, and another test must be used to determine the convergence of the series.
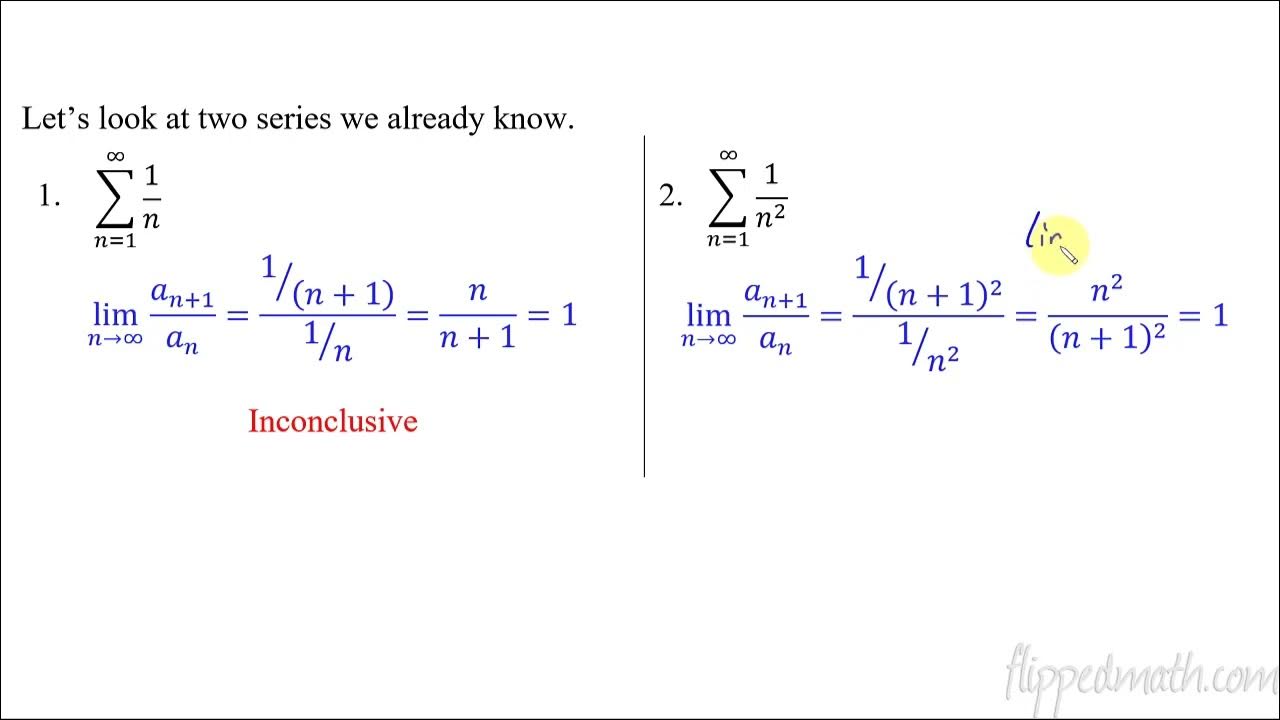
Why might the ratio test be inconclusive for p-series?
-The ratio test might be inconclusive for p-series because these series often have a form similar to 1/n^p, where the limit of the ratio can approach one, making it difficult to determine convergence or divergence.
What is a common scenario where the ratio test is conclusive?
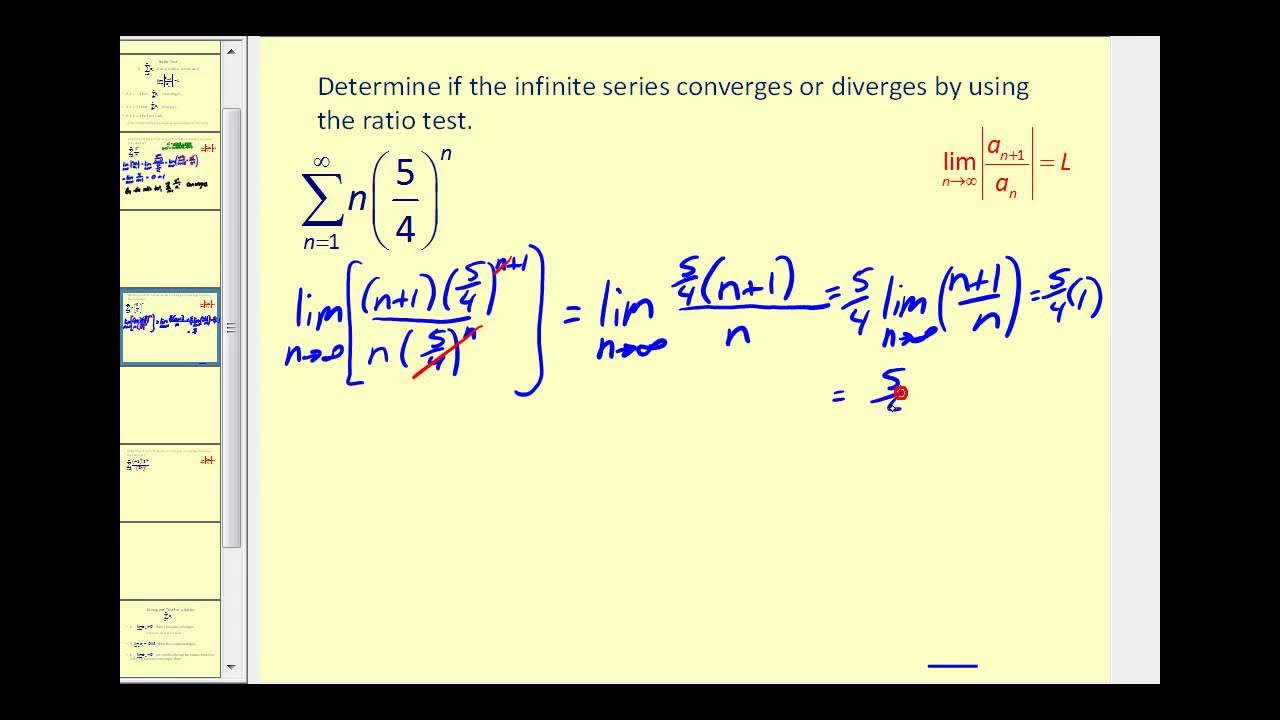
-The ratio test is often conclusive when applied to series that have a mix of factorial and geometric aspects, such as a series with terms involving both factorial and a power of a constant like 5^n.
Can you provide an example of a series where the ratio test shows divergence?
-An example given in the script is a series with terms involving (n+1)! * 5^(n+1) / 5^n / n!. The ratio test shows that this series diverges because the limit of the ratio as n approaches infinity is greater than one.
Can you provide an example of a series where the ratio test shows convergence?
-An example given in the script is a series with terms involving 2^(n+1) / (n+1)! / (n+2)!. The ratio test shows that this series converges absolutely because the limit of the ratio as n approaches infinity is less than one.
Outlines
📚 Introduction to the Ratio Test and Factorials
This paragraph introduces the concept of the Ratio Test, a mathematical test used to determine the convergence of a series. It begins by explaining the factorial notation, which is a key component in the test. The speaker clarifies what factorial means, how to calculate it for numbers like 2, 3, 4, and 5, and how to handle factorial expressions with variables. They also delve into the combinatorial significance of factorials, relating them to the number of unique arrangements of items. The paragraph concludes with a brief mention of the Ratio Test's application to series with factorials in them, setting the stage for a deeper exploration in the subsequent paragraphs.
🔍 Applying the Ratio Test to Series with Factorials
The second paragraph delves into the specifics of applying the Ratio Test to series, particularly those that include factorials. It explains the process of setting up the Ratio Test by taking the limit of the absolute value of the ratio of the (n+1)th term to the nth term of the series as n approaches infinity. The speaker provides a detailed example using a series with terms involving 1/n^2, demonstrating how to simplify the expression and find the limit. The paragraph also touches on the inconclusive nature of the Ratio Test for p-series and series that can be compared to p-series, highlighting the conditions under which the test is conclusive or not.
📉 Examples of Using the Ratio Test to Determine Convergence
In this paragraph, the speaker provides two examples to illustrate how the Ratio Test can be used to determine whether a series converges or diverges. The first example involves a series with terms that include factorials and a geometric aspect, showing that the series diverges as the limit of the ratio is greater than one. The second example features a series with terms that simplify to a limit of zero, indicating absolute convergence. The speaker emphasizes the utility of the Ratio Test for series that combine different types of expressions and provides a clear conclusion for each example, demonstrating the practical application of the test.
Mindmap
Keywords
💡Factorial
💡Ratio Test
💡Combinatorial Meaning
💡n! / (n+1)!
💡P Series
💡Limit
💡Convergence
💡Divergence
💡Inconclusive
💡Power Series
Highlights
Introduction to the ratio test for determining series convergence.
Explanation of factorial notation and its combinatorial meaning.
Calculation of common factorial values like 2!, 3!, 4!, and 5!.
Demonstration of simplifying factorial expressions with variables, such as n!/(n+1)!.
The definition of 0! as 1 and its combinatorial significance.
Introduction of the ratio test formula and its application to series with factorials.
Description of the limit process in the ratio test and its implications for series convergence.
Conditions for series convergence, divergence, and inconclusive results from the ratio test.
Application of the ratio test to p-series and an example with 1/n^2.
Explanation of when the ratio test is inconclusive and the need for alternative tests.
Example of applying the ratio test to a series with factorial and geometric components.
Step-by-step process of simplifying the ratio test expression for a given series.
Determination of series divergence using the ratio test with a limit greater than one.
Application of the ratio test to another series, leading to an absolute convergence conclusion.
General advice on when to use the ratio test based on the series components.
Upcoming discussion on using the ratio test for finding intervals and radii of convergence in the next video.
Conclusion of the video with a summary of the ratio test outcomes for the examples provided.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: