Series Tests - The Ratio Test
TLDRThis video explains the Ratio Test, a method to determine if an infinite series converges or diverges. It highlights the test's utility in cases where series involve factorials or power series. The video demonstrates the test with examples, showing how to calculate the limit of the ratio of consecutive terms and interpret the results to conclude convergence or divergence.
Takeaways
- 📚 The ratio test is a method used to determine if an infinite series converges or diverges and is favored by the speaker.
- 🔢 The test involves calculating 'Rho', which is the limit as n approaches infinity of the absolute value of the ratio of consecutive terms in the series.
- 🌐 Rho's value determines the series' behavior: if Rho < 1, the series converges; if Rho > 1, it diverges; if Rho = 1, the test is inconclusive.
- 🔄 The ratio test is particularly useful for series with factorials in the terms and is commonly used in power series analysis.
- 📉 The test is similar to the geometric series test in terms of convergence criteria, which can help with memorization.
- ❌ The ratio test is not suitable for series that resemble a p-series, where the limit comparison test is recommended instead.
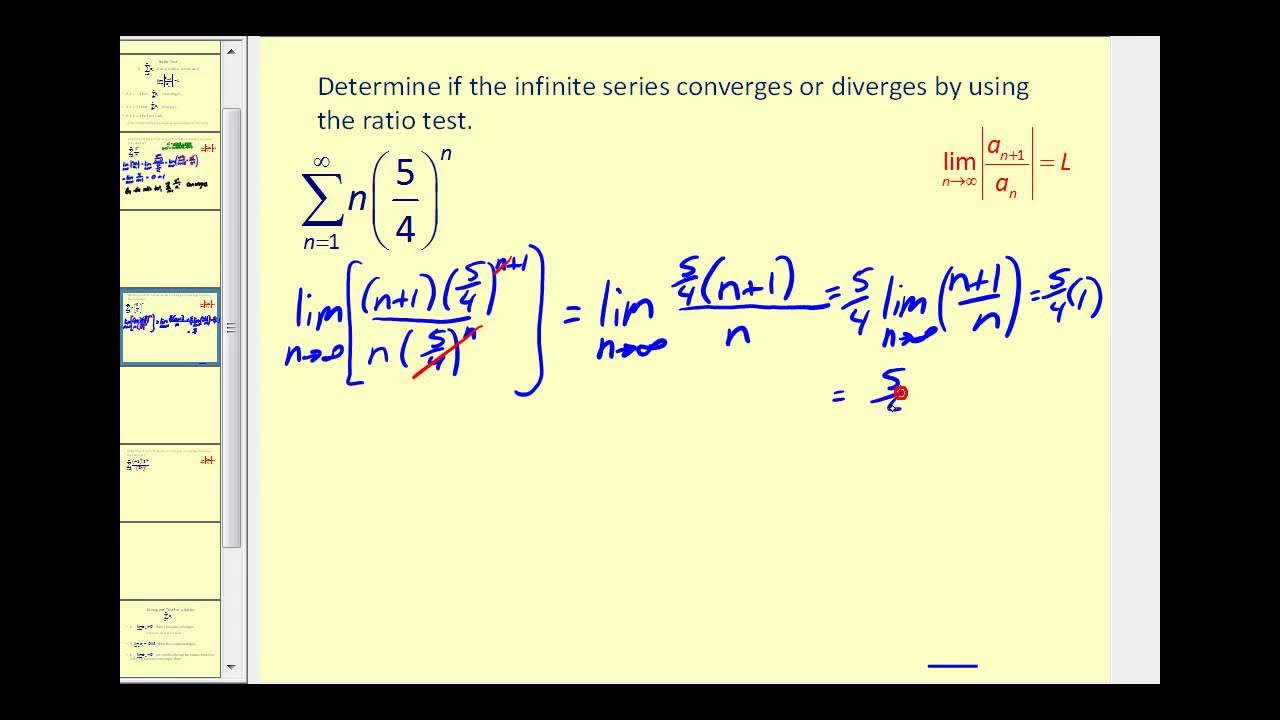
- 📝 The first example provided is the series summing n times (2/3)^n, which converges by the ratio test as Rho is calculated to be 2/3.
- 📉 The second example is the series summing n^n over 2^n times n factorial, which diverges as Rho is greater than 1 after simplification.
- 🧩 Simplification techniques such as canceling out terms and using reciprocals can make the calculation of Rho more manageable.
- 🔑 Memorizing the limit of (1 + 1/n)^n as n approaches infinity being equal to 'e' is crucial for solving ratio test problems.
- 📚 The video script is intended to be educational, providing examples and explanations to help viewers understand and apply the ratio test.
Q & A
What is the ratio test used for?
-The ratio test is used to determine if an infinite series converges or diverges.
What does the Greek letter Rho represent in the context of the ratio test?
-Rho represents the limit as n approaches infinity of the absolute value of the ratio of the (n+1)th term to the nth term of the series.
Why is the absolute value important in the ratio test?
-The absolute value is important because it allows the ratio test to be used on series that do not necessarily have positive terms, unlike many other tests.
What are the three possible outcomes when calculating Rho in the ratio test?
-The three possible outcomes are: Rho is less than 1 (series converges), Rho is greater than 1 (series diverges), and Rho equals 1 (test is inconclusive, and a different test must be used).
Why is the ratio test often used for series with factorials in the terms?
-The ratio test is often used for series with factorials because it simplifies the process of determining convergence, especially in cases involving power series and their intervals of convergence.
What is the significance of the ratio test being inconclusive when Rho equals 1?
-When Rho equals 1, the test is inconclusive, indicating that the series may not follow the same convergence rules as those with Rho less than or greater than 1, and an alternative test should be used.
Why might the ratio test be inconclusive for a p-series?
-The ratio test might be inconclusive for a p-series because these types of series often require the limit comparison test for a definitive convergence or divergence conclusion.
In the first example given in the script, what is the series and what does the ratio test conclude about its convergence?
-The series is the sum of n times 2/3 to the power of n. The ratio test concludes that since Rho is 2/3, which is less than 1, the series converges.
In the second example, what is the series and what does the ratio test conclude about its divergence?
-The series is the sum of n to the power of n over 2 to the power of n times n factorial. The ratio test concludes that since Rho is y over 2, which is greater than 1, the series diverges.
What is the significance of the limit as n approaches infinity of (1 + 1/n)^n being equal to e in the context of the ratio test?
-This limit is significant because it often appears in the ratio test calculations, especially when simplifying terms raised to the power of n, and it is a well-known mathematical constant that represents the base of the natural logarithm.
Outlines
📚 Introduction to the Ratio Test
The video introduces the Ratio Test, a method for determining the convergence or divergence of an infinite series. The presenter emphasizes its utility, especially when dealing with series that include factorials or power series. The test involves calculating the limit of the absolute value of the ratio of consecutive terms as n approaches infinity, denoted by Rho. If Rho is less than 1, the series converges; if greater than 1, it diverges; and if equal to 1, the test is inconclusive, prompting the use of an alternative test. The presenter also notes the similarity between the Ratio Test and the geometric series test, and provides a practical example involving a series with a factorial term.
🔍 Applying the Ratio Test with Examples
The presenter provides two examples to illustrate the application of the Ratio Test. The first example involves a series with a factorial term, where the calculation of Rho simplifies to a well-known limit, leading to the conclusion that the series diverges. The second example features a series with a term structure that, upon simplification, results in a ratio test calculation yielding a value less than 1, indicating convergence. The presenter highlights the importance of recognizing when to apply the Ratio Test and the significance of the limit of (1 + 1/n)^n as n approaches infinity, which is equal to e, a fundamental constant in mathematics. The video concludes with a reminder of the Ratio Test's usefulness in various mathematical contexts.
Mindmap
Keywords
💡Ratio Test
💡Convergence
💡Divergence
💡Rho (ρ)
💡Inconclusive
💡Factorials
💡Power Series
💡Limit
💡Geometric Series Test
💡Limit Comparison Test
💡E (Euler's Number)
Highlights
The ratio test is a method to determine if an infinite series converges or diverges.
The ratio test is often referred to as the best test ever for series convergence.
Rho (ρ) is defined as the limit as n approaches infinity of the absolute value of a sub n+1 over a sub n.
The absolute value in the definition of Rho allows for series with non-positive terms.
If Rho is less than 1, the series converges.
If Rho is greater than 1, the series diverges.
If Rho equals 1, the test is inconclusive and a different test must be used.
The ratio test is similar to the geometric series test in terms of convergence criteria.
The ratio test is often used when the series has factorials in the terms.
The ratio test is frequently used in power series to find the interval and radius of convergence.
The first example involves the sum of n times 2/3 to the power of n.
In the first example, Rho is calculated to be 2/3, indicating convergence by the ratio test.
The second example involves the sum of n to the power of n over 2 to the power of n times n factorial.
In the second example, Rho is calculated to be y over 2, indicating divergence by the ratio test.
The limit as n approaches infinity of (1 + 1/n)^n is a famous limit equal to e.
Memorizing the limit of (1 + 1/n)^n as e is useful for ratio test problems.
The ratio test is a valuable tool in mathematical analysis, especially for series involving factorials and power series.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: