Calculus BC – 10.8 Ratio Test for Convergence

The Algebros
10 Jan 202209:36
EducationalLearning
32 Likes 10 Comments

TLDRIn this calculus lesson, Mr. Bean introduces the Ratio Test for determining the convergence of a series with positive terms. He explains the concept of factorials and exponential simplification strategies before diving into the test itself. The video demonstrates the test's application on various series, including harmonic and p-series, and highlights its limitations when the ratio equals one. Mr. Bean emphasizes the importance of the Ratio Test as an additional tool in analyzing series convergence, offering step-by-step examples to solidify understanding.

Takeaways
  • 📚 The video is a calculus lesson focused on the Ratio Test for convergence of series.
  • 🔢 Mr. Bean starts by reviewing factorials, explaining that \( n! \) is the product of all positive integers up to \( n \).
  • ✂️ He demonstrates how to simplify expressions involving factorials by canceling out terms.
  • 📈 The lesson introduces the Ratio Test, which involves taking the limit of the ratio of consecutive terms of a series as \( n \) approaches infinity.
  • ⏩ The test states that if the limit is less than 1, the series converges; if greater than 1, it diverges; and if equal to 1, the test is inconclusive.
  • 🚫 Mr. Bean clarifies that the Ratio Test is not suitable for alternating series or series that are purely negative.
  • 🔍 The instructor uses the Ratio Test on a harmonic series and a \( p \)-series, finding the test inconclusive for both due to the limit equaling 1.
  • 📉 The video emphasizes that for \( p \)-series, the Ratio Test is not useful and understanding the degree of the series is more important.
  • 📚 Mr. Bean provides examples of series where the Ratio Test is conclusive, showing how to simplify and apply the test to determine convergence or divergence.
  • 📝 He stresses the importance of writing down \( a_{n+1} \) and \( a_n \) before setting up the limit for the Ratio Test.
  • 🔑 The lesson concludes with a reminder that the Ratio Test is an additional tool for analyzing series, not a replacement for other tests or understanding of series behavior.
Q & A

  • What is the main topic of the lesson presented by Mr. Bean in the transcript?

    -The main topic of the lesson is the Ratio Test for convergence in calculus.

  • What is a factorial and how is it represented mathematically?

    -A factorial of a non-negative integer n, denoted as n!, is the product of all positive integers less than or equal to n. For example, 5! = 5 × 4 × 3 × 2 × 1.

  • What is the significance of the ratio test in determining the convergence of a series?

    -The ratio test helps in determining if a series converges or diverges by examining the limit of the ratio of the (n+1)th term to the nth term as n approaches infinity.

  • How does the ratio test work for a series with positive terms?

    -For a series with positive terms, if the limit of the ratio of consecutive terms is less than 1 as n approaches infinity, the series converges. If the limit is greater than 1, the series diverges. If the limit equals 1, the test is inconclusive and another test must be used.

  • What does it mean if the ratio test result is inconclusive?

    -If the ratio test result is inconclusive, it means that the limit of the ratio equals 1, and the test does not provide enough information to determine whether the series converges or diverges. In such cases, another test must be applied.

  • Why is the ratio test not suitable for p-series?

    -The ratio test is not suitable for p-series because it will always be inconclusive. The ratio of consecutive terms in a p-series will always equal 1, which does not help in determining convergence or divergence.

  • What is the result of the ratio test for a harmonic series?

    -The ratio test for a harmonic series is inconclusive because the limit of the ratio of consecutive terms equals 1, which does not provide enough information to determine convergence or divergence.

  • Can you provide an example of a series where the ratio test indicates convergence?

    -An example of a series where the ratio test indicates convergence is a series where the limit of the ratio of the (n+1)th term to the nth term is less than 1 as n approaches infinity, such as a geometric series with a common ratio less than 1.

  • What is the result of the ratio test for a series with a factorial in the numerator?

    -If the ratio test is applied to a series with a factorial in the numerator, and the limit of the ratio of consecutive terms approaches 0 as n approaches infinity, the series converges.

  • How does the ratio test handle series with alternating signs?

    -The ratio test as described in the transcript is specifically for series with positive terms. For series with alternating signs, a different approach or test may be required to determine convergence or divergence.

  • What is the conclusion of the lesson regarding the use of the ratio test?

    -The conclusion of the lesson is that the ratio test is another tool in the toolbox for analyzing series. It is not always the first choice, especially for p-series, but it can be useful in certain situations where the limit of the ratio of consecutive terms is clearly less than or greater than 1.

Outlines
00:00
📚 Introduction to the Ratio Test in Calculus

In this calculus lesson, Mr. Bean introduces the concept of the Ratio Test for series convergence. He starts by reminding students about factorials and exponentials, explaining how to rewrite expressions involving these concepts for simplification. The Ratio Test is then defined for series with positive terms, where the ratio of the (n+1)th term to the nth term is considered. If the limit of this ratio as n approaches infinity is less than one, the series converges; if greater than one, it diverges; and if equal to one, the test is inconclusive, requiring further analysis. The instructor uses examples of harmonic and p-series to illustrate the application of the Ratio Test, noting that it is inconclusive for p-series.

05:01
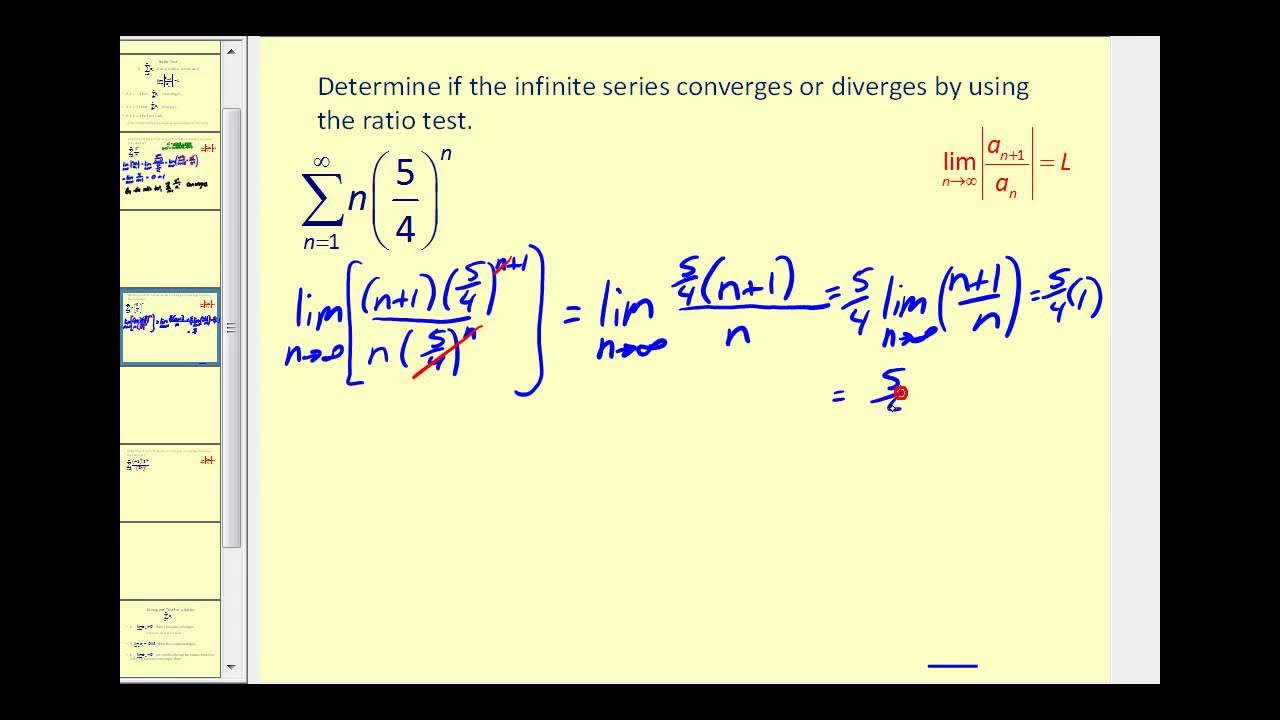
🔍 Applying the Ratio Test to Series with Exponents and Factorials

The second paragraph delves deeper into applying the Ratio Test to series, starting with a series involving exponential terms. The instructor demonstrates the process of setting up the limit for the ratio of consecutive terms and simplifying using exponent rules. This results in a simplified expression that allows for the determination of convergence based on the limit's value relative to one. The lesson continues with examples involving factorials, showing how to rewrite and simplify expressions to find the limit of the ratio. The instructor emphasizes the importance of writing down the nth and (n+1)th terms clearly before setting up the limit. The examples provided show how the Ratio Test can be conclusive when the limit is not equal to one, leading to a determination of convergence or divergence. However, for series where the limit equals one, the test is inconclusive, and other methods must be used. The paragraph concludes with a complex example involving factorials, demonstrating the cancellation process and the final determination that the series diverges because the limit approaches infinity, indicating a ratio greater than one.

Mindmap
Keywords
💡Calculus
Calculus is a branch of mathematics that deals with the study of rates of change and accumulation. In the video, calculus is the overarching subject, with the focus on a specific test within the field. The video aims to teach students about the ratio test for convergence, which is a concept in calculus used to determine the behavior of infinite series.
💡Ratio Test
The ratio test is a method used to determine the convergence or divergence of an infinite series. It involves taking the limit of the ratio of consecutive terms of the series as the number of terms approaches infinity. The video's main theme revolves around explaining and applying the ratio test to various series to understand their convergence properties.
💡Factorial
A factorial, denoted by an exclamation mark (e.g., 5!), is the product of all positive integers up to a given number. In the script, the concept of factorial is introduced as a fundamental operation necessary for understanding series that involve factorials, such as in the ratio test calculations.
💡Exponential
Exponential functions are mathematical functions where the variable is in the exponent. In the context of the video, the teacher simplifies expressions involving exponential terms by separating the exponents, which is crucial for applying the ratio test to series with exponential components.
💡Convergence
Convergence in the context of series refers to the property where the sum of the series approaches a finite limit as the number of terms increases indefinitely. The video discusses the ratio test as a tool to determine if a series converges, with the test indicating convergence if the limit of the ratio is less than one.
💡Divergence
Divergence is the opposite of convergence; it refers to a series where the sum does not approach a finite limit as the number of terms increases. The video explains that if the ratio test's limit is greater than one, the series is divergent.
💡Horizontal Asymptote
A horizontal asymptote is a horizontal line that a function approaches as the input (or the variable) approaches infinity. In the script, the teacher uses the concept of a horizontal asymptote to describe the limit of the ratio in the ratio test, which helps determine the behavior of the series.
💡Harmonic Series
The harmonic series is a specific type of infinite series where the terms are the reciprocals of the natural numbers. The video uses the harmonic series as an example to demonstrate the application of the ratio test, noting that the test is inconclusive for this series as it equals one.
💡P-Series
A p-series is an infinite series of the form 1/n^p, where p is a real number. The video mentions p-series as a type of series for which the ratio test is inconclusive, and it suggests using other methods to determine the convergence of such series.
💡Leading Coefficients
In the context of polynomial expressions, leading coefficients are the coefficients of the term with the highest power. The video explains that when applying the ratio test, one should consider the leading coefficients of the terms in the series to simplify the limit expression and determine the series' behavior.
Highlights

Introduction to the ratio test for series convergence.

Review of factorial definition and its properties.

Explanation of how to rewrite factorial expressions for simplification.

Introduction of exponential properties and how to simplify exponents.

The formula for the ratio test and its conditions for series convergence.

Application of the ratio test to a harmonic series and its inconclusive result.

Use of the ratio test on a p-series and its limitations.

Demonstration of the ratio test on a series with exponential terms.

Simplification of the ratio test expression using exponent rules.

Conclusion that the series converges when the ratio test result is less than one.

Introduction of factorials in the ratio test and their simplification.

Cancellation technique in factorial expressions during the ratio test.

Application of the ratio test to a series with factorials in the numerator.

Conclusion of series convergence when the ratio test limit equals zero.

Complex example with 2n factorial and its ratio test application.

Analysis of a series that diverges based on the ratio test result being greater than one.

Summary of the ratio test's utility in the toolbox of series analysis.

Final remarks and sign-off from Mr. Bean.

Transcripts

Browse More Related Video

AP Calculus BC Lesson 10.8

Calculus BC – 10.13 Radius and Interval of Convergence of Power Series

The Ratio Test

Ratio Test

Calculus BC – 10.6 Comparison Tests for Convergence

Calculus BC – 10.9 Determining Absolute or Conditional Convergence

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
CalculusConvergenceRatio TestFactorialsExponentsSeries AnalysisEducationalMath StrategyInfinite LimitsMr. BeanTeaching