Tensor Calculus 19: Covariant Derivative (Intrinsic) and Geodesics

eigenchris

27 Oct 201822:49

EducationalLearning

32 Likes 10 Comments

TLDRThis video delves into the intrinsic definition of the covariant derivative in curved spaces, building upon previous discussions on flat and curved space derivatives. It explains the concept without reference to an external space, focusing on the metric tensor and Christoffel symbols. The video also touches on geodesics, defining them as curves resulting from parallel transporting a vector along itself, and introduces the geodesic equations. Aimed at viewers with a keen interest in differential geometry and its applications in fields like general relativity.

Takeaways

📚 The video discusses the intrinsic definition of the covariant derivative, continuing from previous videos on flat and curved space definitions.
🔗 It suggests watching related videos for a comprehensive understanding, specifically mentioning geodesics covered in videos 15 and 16.
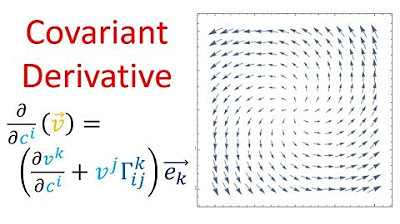
📉 The covariant derivative on a curved surface is explained as the rate of change of a vector field with the normal component of the rate of change subtracted.
📍 Christoffel symbols are introduced as part of the formula for the covariant derivative, which are crucial for understanding parallel transport of vectors.
🌐 The video contrasts extrinsic and intrinsic approaches to curved spaces, highlighting the absence of an external reference frame in intrinsic geometry.
📈 The intrinsic formula for Christoffel symbols is derived without relying on external coordinates, using the metric tensor and its properties.
🔍 The importance of intrinsic geometry is emphasized, particularly its application in general relativity where space-time is considered intrinsically curved.
📘 The video demonstrates how to compute the Christoffel symbols for a sphere using the intrinsic method and confirms it matches the extrinsic method.
🔄 The concept of parallel transport is reiterated, showing how it applies to both intrinsic and extrinsic spaces, with examples of vector transport on a sphere.
🛤️ A new definition of geodesics is introduced using the covariant derivative, describing them as curves resulting from parallel transporting a vector along itself.
🔮 The video concludes with a preview of the next video, which will cover the abstract definition of the covariant derivative for general tensor fields.

Q & A

What is the main topic discussed in the video?
-The main topic discussed in the video is the intrinsic definition of the covariant derivative in the context of curved spaces, as well as a brief discussion on geodesics.
Why is it important to understand both extrinsic and intrinsic approaches to curved spaces?
-Understanding both approaches is important because extrinsic geometry uses an embedding space for calculations, while intrinsic geometry operates solely within the space itself, which is crucial for theories like general relativity where there is no 'outside' space to refer to.
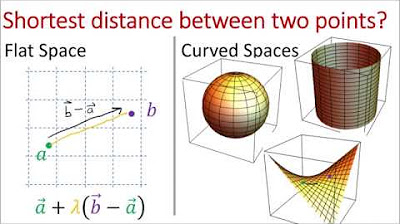
What are geodesics in the context of curved spaces?
-Geodesics are the shortest paths or 'straightest' curves within a curved space, and they can be defined using the covariant derivative by setting the covariant derivative of a vector in the direction of itself to zero.
What is the significance of the covariant derivative being zero in the context of parallel transport?
-A covariant derivative of zero indicates that a vector is being parallel transported along a curve, meaning the vector maintains its direction and magnitude relative to the local frame of reference.
How does the formula for the covariant derivative change when moving from extrinsic to intrinsic geometry?
-In intrinsic geometry, the formula for the covariant derivative remains the same, but the computation of Christoffel symbols, which serve as coefficients in the expansion of basis vector derivatives, becomes more challenging due to the absence of an external reference frame.
What role do Christoffel symbols play in the computation of the covariant derivative?
-Christoffel symbols are used to express the derivative of a basis vector in terms of other basis vectors. They act as coefficients in the expansion and are essential for calculating the covariant derivative in both intrinsic and extrinsic geometries.
Why is the metric tensor important in intrinsic geometry?
-The metric tensor is important in intrinsic geometry because it provides a way to measure distances and angles within the space without referring to an external coordinate system. It is also used in the computation of Christoffel symbols.
What is the relationship between the covariant derivative and the geodesic equations?
-The geodesic equations can be derived from the condition that the covariant derivative of a vector field in the direction of itself is zero. This condition describes the path of a particle moving in a 'straight line' on a curved space.
How does the video script differentiate between the covariant derivative in flat and curved spaces?
-The script explains that in flat spaces, the covariant derivative is simply the ordinary derivative, while in curved spaces, it involves additional terms to account for the curvature, specifically the Christoffel symbols.
What is the significance of the Einstein summation convention used in the script?
-The Einstein summation convention is a notational shorthand used in the script to indicate that when an index variable appears twice in a single term and is not otherwise defined, it implies that we are summing over all of its possible values.
How does the script explain the concept of 'walking forward' on a curved space?
-The script explains 'walking forward' on a curved space as the process of parallel transporting a vector along itself, which mathematically translates to setting the covariant derivative of a vector in the direction of itself to zero.

Outlines

00:00

📚 Introduction to the Intrinsic Definition of Covariant Derivative

This paragraph introduces the intrinsic definition of the covariant derivative, which is a continuation of previous discussions on flat and curved space definitions. The covariant derivative on a curved surface is explained as the rate of change of a vector field with the normal component subtracted. The paragraph also mentions the importance of understanding geodesics and the intrinsic approach to curved spaces, where one only works with coordinate lines on the surface without reference to an external 3D space. The Christoffel symbols, which are crucial for the covariant derivative, are highlighted as needing a different approach in intrinsic geometry due to the absence of an external coordinate system.

05:01

🔍 Exploring the Intrinsic Geometry and Christoffel Symbols

The second paragraph delves into the computation of the covariant derivative in intrinsically curved spaces, emphasizing the absence of normal vectors and the reliance on the metric tensor. It explains that the metric tensor components are symmetric and that the derivative of the basis vectors can be expressed using Christoffel symbols. The paragraph introduces a new strategy for computing Christoffel symbols intrinsically, utilizing the symmetry of the metric tensor and the fact that the basis vectors are partial derivative operators. It also discusses the importance of intrinsic geometry in the context of general relativity and Einstein's concept of a four-dimensional curved space-time without an external space.

10:01

📘 Deriving the Intrinsic Formula for Christoffel Symbols

This paragraph focuses on deriving the intrinsic formula for Christoffel symbols by taking the derivative of the metric tensor components and using the metric compatibility property. It explains how to replace basis vector derivatives with Christoffel symbols and how to use the symmetry of the metric tensor components and the Christoffel symbols to solve for the symbols. The paragraph provides a detailed mathematical process that leads to a new formula for Christoffel symbols, which is essential for defining the covariant derivative in intrinsically curved spaces.

15:02

🌐 Verifying the Intrinsic Formula with an Example

The fourth paragraph verifies the derived intrinsic formula for Christoffel symbols using the example of a sphere. It recomputes the Christoffel symbols for the sphere using the new formula and compares the results with the previously computed extrinsic formula. The paragraph demonstrates that the intrinsic formula yields the same results as the extrinsic formula, confirming the validity of the intrinsic approach. It also discusses the concept of parallel transport and how it applies to both intrinsic and extrinsic spaces.

20:03

🛤️ Geodesics and the Abstract Definition of Covariant Derivative

The final paragraph introduces the concept of geodesics as curves resulting from walking straight forward in a curved space, defined using the covariant derivative. It explains the geodesic equations and how they describe curves that satisfy the condition of parallel transporting a vector along itself. The paragraph also provides a brief overview of the upcoming content, which will cover the abstract definition of the covariant derivative for general tensor fields, not just vector fields.

Mindmap

Keywords

💡Covariant Derivative

The covariant derivative is a concept in differential geometry that generalizes the directional derivative to curved spaces. It allows for the measurement of the rate of change of a vector field along a curve on a manifold, taking into account the curvature of the space. In the video, the covariant derivative is discussed in the context of both flat and curved spaces, with a focus on its intrinsic definition for curved spaces, which is essential for understanding phenomena in general relativity and other areas of physics.

💡Flat Space vs. Curved Space

Flat space and curved space refer to the geometrical properties of the space being studied. Flat space is characterized by Euclidean geometry, where the shortest distance between two points is a straight line. Curved space, on the other hand, follows non-Euclidean geometry, where the shortest path between two points is a curve, as seen on the surface of a sphere. The video script discusses the covariant derivative in both contexts, highlighting the differences in how it is defined and computed.

💡Geodesics

Geodesics are the shortest paths between two points in curved space, analogous to straight lines in flat space. They are significant in the study of general relativity, where they represent the paths followed by objects moving under the influence of gravity alone. The script mentions that the main videos on geodesics are in videos 15 and 16, and it also introduces a new way to define geodesics using the covariant derivative.

💡Christoffel Symbols

Christoffel symbols are used in differential geometry to express the covariant derivative in terms of the metric tensor of the space. They encode the 'bending' of the space and are essential in the computation of the covariant derivative on a curved manifold. The video explains how to compute these symbols intrinsically, without reference to an extrinsic coordinate system.

💡Intrinsic Geometry

Intrinsic geometry is the study of geometric properties that are defined solely by the properties of the space itself, without reference to an external space. It is contrasted with extrinsic geometry, which considers a space embedded in a higher-dimensional space. The video emphasizes the intrinsic approach to define the covariant derivative and other concepts, which is crucial for understanding general relativity.

💡Extrinsic Geometry

Extrinsic geometry involves studying a geometric object while considering its embedding in a higher-dimensional space. For example, studying a sphere by considering its position and orientation in three-dimensional space. The script contrasts extrinsic geometry with intrinsic geometry, particularly when discussing how basis vectors and the covariant derivative are defined differently in each approach.

💡Metric Tensor

The metric tensor is a fundamental concept in differential geometry that provides a way to measure distances and angles within a space. It is particularly important in intrinsic geometry, as it defines the space's curvature. The video discusses how the metric tensor is used in the computation of Christoffel symbols and the covariant derivative within the intrinsic framework.

💡Parallel Transport

Parallel transport is a concept that describes how a vector is moved along a path in a curved space in such a way that it remains parallel to its original direction. It is related to the covariant derivative being zero. The script explains that parallel transport can be visualized on a sphere and is a key concept in understanding the behavior of vectors in curved spaces.

💡Basis Vectors

Basis vectors are vectors that form a basis for a vector space, allowing any vector in that space to be expressed as a linear combination of the basis vectors. In the context of the video, basis vectors in intrinsic geometry are represented by partial derivative operators, as opposed to the tangent vectors along a curve in extrinsic geometry.

💡Einstein Field Equations

The Einstein field equations are the fundamental equations of general relativity that describe the fundamental interaction of gravitation as a result of space-time being curved by mass and energy. The script mentions these equations in the context of deriving the metric tensor, which is essential for understanding the intrinsic geometry of space-time.

💡Tangent Vector Space

The tangent vector space at a point on a manifold is the set of all vectors that are tangent to the manifold at that point. It is a key concept in differential geometry and is central to the intrinsic definition of the covariant derivative. The script explains that each point on a curved space has its tangent vector space, which is used to define basis vectors intrinsically.

Highlights

Introduction to the intrinsic definition of the covariant derivative as a continuation of previous videos on flat and curved space definitions.

Brief discussion on geodesics and reference to main videos for detailed understanding.

Explanation of the covariant derivative on a curved surface as the rate of change of a vector field with the normal component subtracted.

Christoffel symbols are introduced as part of the formula for the covariant derivative in curved spaces.

Parallel transport of a vector along a curve is defined when the covariant derivative is zero.

The intrinsic approach to curved spaces is contrasted with the extrinsic approach, emphasizing living on the surface without external reference.

Importance of intrinsic geometry in general relativity and Einstein's concept of space-time as intrinsically curved.

Basis vectors in intrinsic geometry are represented by partial derivative operators, differing from the extrinsic approach.

The covariant derivative formula remains the same in intrinsic geometry, but the computation of Christoffel symbols becomes more challenging.

Metric tensor components are symmetric due to the commutative property of the dot product in intrinsic spaces.

Derivation of the intrinsic formula for Christoffel symbols without reliance on extrinsic coordinates.

The metric compatibility property is introduced as crucial for the intrinsic definition of the covariant derivative.

Verification of the intrinsic formula for Christoffel symbols using the example of a sphere, matching the extrinsic calculation.

Geodesics are redefined using the covariant derivative as curves resulting from parallel transporting a vector along itself.

Introduction to the geodesic equations as a set of differential equations derived from the covariant derivative.

Upcoming coverage of the abstract definition of the covariant derivative for general tensor fields in the next video.

Transcripts

Browse More Related Video

Tensor Calculus 18: Covariant Derivative (extrinsic) and Parallel Transport

Tensor Calculus 17: The Covariant Derivative (flat space)

Tensor Calculus 15: Geodesics and Christoffel Symbols (extrinsic geometry)

General Relativity Lecture 4

General Relativity Lecture 2

Video 29 - Christoffel Symbol Transformation

Related Tags

Covariant Derivative Curved Spaces Intrinsic Geometry General Relativity Geodesics Metric Tensor Christoffel Symbols Parallel Transport Space-Time Differential Geometry