Calculus grade 12 Optimisation: Exam
TLDRIn the video script, the presenter guides students through solving an optimization problem involving the area of a stage, which is a combination of a rectangle and a semicircle. The presenter emphasizes a common approach to such problems: finding a formula for the area, using the given perimeter to eliminate one variable, and then differentiating the area formula with respect to the remaining variable to find the maximum area. The specific example provided involves calculating the value of 'r' that maximizes the stage area, resulting in a solution of 8.40 meters for 'r'.
Takeaways
- 📚 The speaker is discussing the process of solving optimization problems, specifically finding the maximum area of a stage that consists of a semicircle and a rectangle.
- 🔍 The speaker dismisses a question they dislike, indicating a preference for focusing on the method rather than the specific question.
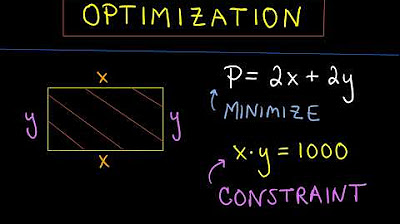
- 📐 The formula for the area of the stage is derived by combining the area of a rectangle (length times breadth) and a semicircle (half of pi times radius squared).
- 🔢 The speaker emphasizes the importance of understanding the 'recipe' for optimization problems, which involves finding a formula for the quantity of interest and then finding its maximum or minimum.
- 📈 The method to find the maximum or minimum involves taking the first derivative of the area formula and setting it to zero, which corresponds to finding turning points on a graph.
- 🔗 The problem provides additional information about the perimeter of the stage, which is used to relate the two variables, the breadth (b) and the radius (r), of the stage.
- 🧩 The perimeter equation is manipulated to express one variable in terms of the other, which simplifies the area formula to a single variable function.
- 📝 The speaker makes a mistake in simplifying the area formula but corrects it, emphasizing the importance of careful calculation in such problems.
- ✂️ The area formula is simplified further to a form that allows for the application of calculus, specifically differentiation.
- 📉 The first derivative of the area with respect to the variable r is calculated to find the critical points that could represent the maximum area.
- 📍 The solution to the optimization problem is found by setting the first derivative equal to zero and solving for r, which yields the value of r that maximizes the area of the stage.
Q & A
What is the main topic of the video script?
-The main topic of the video script is solving an optimization problem related to finding the maximum area of a stage that consists of a semicircle and a rectangle.
What is the formula for the area of the rectangle part of the stage?
-The formula for the area of the rectangle part of the stage is the length times the breadth, which is represented as "b × 2r" in the script.
What is the formula for the area of the semicircle part of the stage?
-The formula for the area of the semicircle part of the stage is half the area of a full circle, which is given by "(1/2) π r^2".
What method is suggested in the script to find the maximum area?
-The script suggests using calculus to find the maximum area by taking the first derivative of the area formula with respect to the variable and setting it to zero.
What is the given perimeter of the stage and how is it used in the problem?
-The given perimeter of the stage is 60 units. It is used to create an equation relating the variables "b" and "r" to find an expression for one variable in terms of the other.
How does the script simplify the equation for the perimeter of the stage?
-The script simplifies the equation for the perimeter by combining terms and expressing "b" in terms of "r" as "30 - r - (π r / 2)".
What is the final expression for the area 'a' in terms of 'r' after substituting the expression for 'b'?
-The final expression for the area 'a' in terms of 'r' is "60r - 2r^2 - 0.5π r^2 + (1/2)π r^2", which simplifies to "60r - 2r^2 - 0.5π r^2".
Why is the first derivative of the area formula taken?
-The first derivative of the area formula is taken to find the maximum or minimum value of the area, as setting the first derivative equal to zero helps identify turning points on a graph.
What is the final step in finding the value of 'r' that maximizes the area?
-The final step is to solve the equation obtained by setting the first derivative equal to zero for the variable 'r', which results in "r = 60 / (4 + π)", approximately 8.40 meters.
Why does the script mention that the question is 'difficult' and then decide to 'get rid of it'?
-The script mentions the question is 'difficult' because it involves a common type of problem that is often asked in exams, but the script decides to 'get rid of it' because the steps required to solve it are already being covered in the explanation, making the question redundant.
Outlines
📚 Optimization Problem Introduction
The speaker introduces an optimization problem related to maximizing the area of a stage, which is a combination of a semicircle and a rectangle. They express a dislike for a particular question but proceed to demonstrate the problem-solving process. The key steps include identifying the need to find a formula for the area, understanding the components of the stage's shape, and using the given perimeter to establish a relationship between the variables involved.
🔍 Deriving the Area Formula and Setting Up the Problem
The speaker explains how to derive the formula for the area of the stage by considering both the rectangle and the semicircle. They simplify the problem by expressing the rectangle's area in terms of its breadth and the semicircle's area as half of pi times the radius squared. The speaker then uses the given perimeter to eliminate one of the variables, setting up the problem for optimization by expressing one variable in terms of the other.
📉 Finding the Maximum Area Through Derivatives
The speaker proceeds to find the maximum area by taking the first derivative of the area formula with respect to the variable r. They simplify the expression for the area, combining like terms, and then calculate the derivative. The goal is to set the derivative equal to zero to find the critical points, which are potential maxima or minima. The speaker then solves for r, the variable representing the radius, to find the value that maximizes the area of the stage.
Mindmap
Keywords
💡Optimization
💡Area
💡Derivative
💡Perimeter
💡Rectangle
💡Semicircle
💡First Derivative
💡Variable
💡Formula
💡Maximum
💡Calculus
Highlights
Introduction to optimization problems with a focus on finding the maximum area.
The question requires finding the area of a stage composed of a semicircle and a rectangle.
Key strategy: Focus on what the question is asking—finding the area, ignoring unnecessary details at the start.
Formula for area derived as the sum of the area of a rectangle and half of a circle.
Explanation of how to maximize or minimize a function by finding the turning point using the first derivative.
Identifying the problem of having two variables (b and r) and the need to eliminate one by substituting with given information.
Using the perimeter of the stage (60 units) to find an expression for one of the variables (b) in terms of the other (r).
Detailed steps to simplify the equation for the perimeter and solve for b.
Substituting the expression for b back into the area formula to get an area function in terms of a single variable (r).
Simplification of the area formula to a function of r, preparing it for differentiation.
Taking the first derivative of the area function with respect to r.
Setting the first derivative equal to zero to find the value of r that maximizes the area.
Calculation of r using the equation derived from setting the derivative equal to zero.
Final result: The value of r that maximizes the area is approximately 8.40 meters.
Reiteration of the importance of understanding the optimization process and the commonalities across similar problems.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: