Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (29 of 92) Expect. Value Momentum=? 1-D Box

Michel van Biezen
29 Nov 201706:04
EducationalLearning
32 Likes 10 Comments

TLDRIn this educational video, the concept of the expectation value of momentum for a particle within a one-dimensional box is explored. The video explains that despite particles moving back and forth, the average momentum is zero due to symmetry in motion. This is derived through integrating the product of the wave function, its complex conjugate, and the momentum operator over the box's length. The conclusion is that for any energy level, the expectation value of momentum in such a system is always zero, highlighting the particle's equal motion to the right and left, which cancels out to yield an average momentum of zero.

Takeaways
  • ๐Ÿ“ฆ The topic is the expectation value of the momentum of a particle in a one-dimensional box.
  • ๐ŸŽฏ The expectation value represents the average value of a physical quantity, in this case, the momentum of a particle.
  • ๐ŸŒŸ The wave function for a particle in a one-dimensional box and its complex conjugate are discussed, with the complex conjugate being the same as the original wave function when there's no imaginary part.
  • ๐Ÿ”„ The momentum operator is introduced, and its definition involves the derivative of the wave function with respect to position.
  • ๐Ÿงฎ The calculation of the expectation value involves an integral of the product of the wave function, the momentum operator, and the complex conjugate of the wave function over the box's extent from 0 to L.
  • ๐ŸŒ The integral is evaluated by first removing constant factors, then integrating the product of sine and cosine functions.
  • ๐Ÿ“ˆ The integral simplifies to a form involving the sine squared of (n PI x / L) evaluated from 0 to L, which results in zero due to symmetry considerations.
  • ๐Ÿšซ The conclusion is that the expectation value of the momentum of a particle in a one-dimensional box is zero for any energy level, indicating a symmetry in the particle's motion.
  • ๐Ÿ”„ The average momentum is zero because the particle's motion to the right is mirrored by its motion to the left, leading to a cancellation effect.
  • ๐ŸŒ The script suggests looking beyond the expectation value to eigenvalues, which might provide more insight into the actual momentum of particles in a single direction.
Q & A

  • What is the expectation value of momentum for a particle in a one-dimensional box?

    -The expectation value of the momentum of a particle in a one-dimensional box is always 0 for any energy level of the particle.

  • What does the expectation value represent in this context?

    -The expectation value represents the average value of a physical quantity, in this case, the momentum of a particle.

  • How is the complex conjugate of the wave function defined in this script?

    -In this script, the complex conjugate of the wave function is the same as the original wave function since there is no imaginary unit 'i' involved.

  • What is the role of the momentum operator in this calculation?

    -The momentum operator is used in the integral expression to calculate the expectation value of the momentum of the particle in the box.

  • Why does the expectation value of momentum turn out to be zero?

    -The expectation value of momentum is zero because the positive and negative momentum values of the particle in the box cancel each other out due to the particle's symmetrical motion to the right and left.

  • What is the significance of the symmetry in the particle's motion?

    -The symmetry in the particle's motion ensures that the average momentum is zero, as the particle's movement to the right is balanced by its movement to the left.

  • How does the boundary condition of the one-dimensional box affect the calculation?

    -The boundary condition of the one-dimensional box (from 0 to L) limits the integration range and affects the evaluation of the integral, leading to the conclusion that the expectation value of momentum is zero.

  • What is the relationship between the wave function and its derivative in this context?

    -The derivative of the wave function with respect to x is used in the calculation of the expectation value of momentum, as it is part of the integrand when the momentum operator is applied.

  • What happens when we integrate the product of sine and cosine functions as seen in the script?

    -When integrating the product of sine and cosine functions, the result is (1/2)a times the sine squared of Ax, where 'a' is the constant in front of 'x' in the wave function.

  • Why is the expectation value of momentum not very informative about the particle's actual motion?

    -The expectation value of momentum being zero does not provide information about the actual motion of the particle, as it only reflects the average value over all possible states, which happens to be zero due to symmetry.

  • What alternative property of momentum can be explored for more insight?

    -Instead of the expectation value, one can explore the eigenvalues of momentum, which represent the values of momentum that do not depend on direction and might provide more insight into the particle's motion.

Outlines
00:00
๐ŸŒŸ Understanding Expectation Value of Momentum in a One-Dimensional Box

This paragraph introduces the concept of the expectation value of momentum for a particle within a one-dimensional box. It explains that the expectation value represents the average momentum of the particle. The discussion revolves around the wave function of the particle and its complex conjugate, as well as the derivative of the wave function with respect to X. The paragraph delves into the mathematical process of calculating the expectation value using the momentum operator and integral calculus, leading to the conclusion that the expectation value of momentum is zero for any energy level of the particle. This surprising result is explained by the symmetry of particle motion in both positive and negative directions, which cancels out to an average of zero.

05:01
๐Ÿ”„ Symmetry and Average Momentum in Quantum Systems

The second paragraph expands on the concept of symmetry in particle motion and its implications for the average momentum. It clarifies that even though particles move back and forth, the positive and negative motions are symmetrical, leading to a net average momentum of zero. The paragraph then suggests shifting the focus from the expectation value of momentum to finding the eigenvalues of momentum, which are independent of direction. This approach aims to provide a better understanding of the actual momentum in a single direction or its magnitude, offering more insight into the particle's behavior than just the expectation value.

Mindmap
Keywords
๐Ÿ’กExpectation Value
The expectation value is a fundamental concept in quantum mechanics that represents the average value of a physical quantity for a system in a particular state. In the context of this video, it specifically refers to the average momentum of a particle within a one-dimensional box. The expectation value is calculated by integrating the product of the wave function and the momentum operator over all space. The video explains that for a particle in a one-dimensional box, the expectation value of momentum is always zero, regardless of the energy level of the particle.
๐Ÿ’กMomentum
Momentum is a physical quantity in physics that represents the motion of an object and is defined as the product of an object's mass and its velocity. In quantum mechanics, momentum is an operator in the Schrรถdinger equation, and the expectation value of momentum is used to understand the average motion of particles. The video discusses the expectation value of momentum for a particle in a one-dimensional box scenario, highlighting that the average momentum is zero due to symmetry in the particle's motion.
๐Ÿ’กOne-Dimensional Box
A one-dimensional box, also known as an infinite potential well or a particle in a box, is a thought experiment in quantum mechanics that involves a particle confined within a box with infinite potential energy walls. This model is used to illustrate the quantization of energy levels and wave-like behavior of particles. The video uses this model to explain how the expectation value of momentum is calculated and why it results in zero for any energy level of the particle.
๐Ÿ’กWave Function
In quantum mechanics, the wave function is a mathematical description of the probability amplitude of a particle's location. It is a fundamental concept that provides insight into the behavior of quantum systems. The wave function's square gives the probability density of finding the particle at a particular point in space. The video discusses the wave function for a particle in a one-dimensional box and its role in calculating the expectation value of momentum.
๐Ÿ’กComplex Conjugate
The complex conjugate of a complex number is obtained by changing the sign of its imaginary part. In the context of quantum mechanics, the complex conjugate of a wave function is used in the calculation of expectation values. The video explains that for the one-dimensional box model, the complex conjugate of the wave function is identical to the original wave function since there is no imaginary part in the wave function, which simplifies the calculation of the expectation value of momentum.
๐Ÿ’กMomentum Operator
The momentum operator is a differential operator used in quantum mechanics to represent the momentum of a particle. It is defined as the negative of the gradient operator (โˆ‡) times the reduced Planck constant (ฤง). The expectation value of momentum is calculated by integrating the product of the wave function and the momentum operator over all space. In the one-dimensional box model, the momentum operator is used to find the average momentum of the particle, which is found to be zero.
๐Ÿ’กIntegral
In mathematics, an integral represents the accumulation of a quantity over a range or region. In physics and specifically in quantum mechanics, integrals are used to calculate expectation values by integrating products of wave functions and operators over all possible states or positions. The video demonstrates the use of integrals to find the expectation value of momentum for a particle in a one-dimensional box, which results in zero due to the symmetry of the particle's motion.
๐Ÿ’กQuantum Mechanics
Quantum mechanics is a fundamental theory in physics that describes the behavior of matter and energy at very small scales, such as atomic and subatomic particles. It introduces concepts like wave functions, quantization of energy levels, and operators to explain phenomena that cannot be understood by classical mechanics. The video is about a specific problem in quantum mechanics, the expectation value of momentum for a particle in a one-dimensional box, which illustrates key quantum mechanical concepts.
๐Ÿ’กEnergy Levels
In quantum mechanics, energy levels refer to the quantized, discrete amounts of energy that a particle can have in a given state. The video discusses the expectation value of momentum for a particle in a one-dimensional box at different energy levels, showing that the average momentum is zero for any energy level due to the symmetrical motion of the particle within the box.
๐Ÿ’กSine and Cosine Functions
Sine and cosine are trigonometric functions that describe periodic phenomena and are used extensively in mathematics and physics. In the context of the video, sine and cosine functions appear in the mathematical expression of the wave function for a particle in a one-dimensional box. These functions are essential for calculating the expectation value of momentum, as they are part of the integrand that is integrated over the spatial domain.
๐Ÿ’กSymmetry
Symmetry in physics often refers to the balanced or mirror-like properties of a system. In the video, symmetry is used to explain why the expectation value of momentum is zero. The particle's motion to the right is mirrored by its motion to the left, leading to a cancellation of positive and negative momenta, resulting in a net momentum of zero.
Highlights

The topic is finding the expectation value of the momentum of a particle in a one-dimensional box.

Expectation value refers to the average value of a physical quantity.

The wave function and its complex conjugate for any energy level n are discussed.

The momentum operator is defined and used in the calculation.

The expectation value of momentum is calculated as an integral from 0 to L.

The integral involves the wave function, momentum operator, and complex conjugate.

The derivative of the wave function with respect to X is utilized.

Constants are factored out of the integral for simplification.

The integral involves the sine and cosine functions of n PI x over L.

The integral of sine times cosine results in a formula involving sine squared.

The expectation value of momentum is found to be zero for any energy level n.

The average momentum is zero due to symmetry in the particle's motion to the right and left.

The video suggests looking at eigenvalues for more insight into the particle's momentum.

Eigenvalues represent the magnitude of momentum in a single direction.

The process of calculating the expectation value of momentum is described in detail.

The video provides a comprehensive explanation of quantum mechanics concepts.

Transcripts

Browse More Related Video

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (30 of 92) Momentum Eigenvalue=? n=1

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (27 of 92) Expectation Value=? 1-D Box n=1

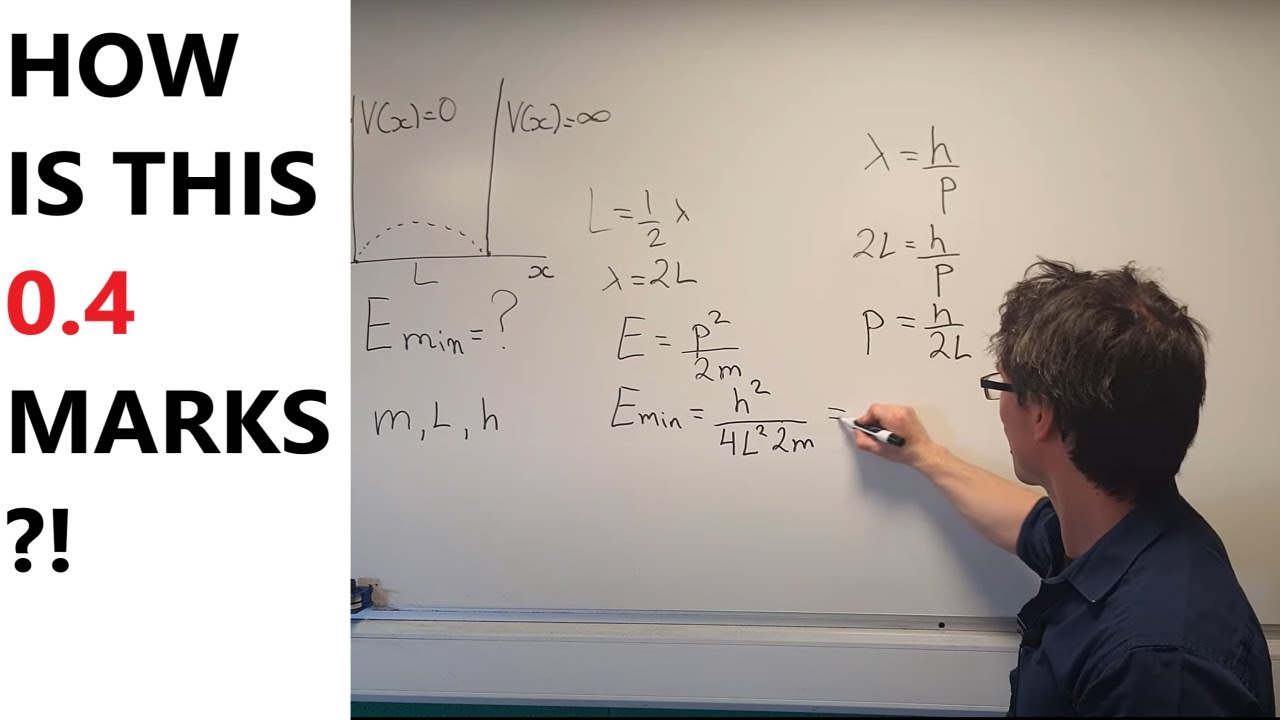
EASIEST question on the International Physics Olympiad?

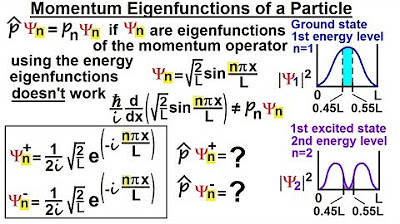
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (31 of 92) Momentum Eigenfunction Particle

21. Quantum Mechanics III

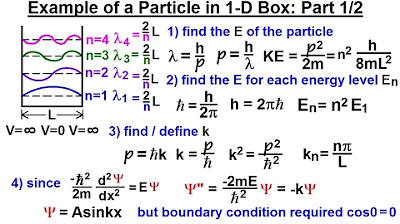
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (19 of 92) Particle in 1-D Box: Example 1/2

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
QuantumPhysicsExpectationValueMomentumOperatorWaveFunctionOneDimensionalBoxZeroMomentumSymmetryEigenvaluesQuantumMechanicsParticlePhysics