AP Physics B Kinematics Presentation #48
TLDRThis script explains the process of finding the direction of the resultant vector C from two given vectors, A and B. Vector A is 8 units north, and vector B is 4.5 units east. Using geometry, the script demonstrates two methods to determine the angle θ between the resultant vector C and the x-axis. The first method involves using the tangent of θ as the opposite over the adjacent side, resulting in θ being 60 degrees. The second method finds θ by considering the angle between A and C, also yielding a 60-degree angle with the x-axis. Both methods confirm that the resultant vector makes a 60-degree angle with respect to the x-axis.
Takeaways
- 📍 The script discusses the direction of the resultant vector when adding two vectors, A and B.
- 🔍 Vector A is described as having a magnitude of eight units and pointing north.
- 📏 Vector B is described as having a magnitude of four point five units and pointing east.
- 📈 The tail-to-tip method is used to add the vectors, resulting in a new vector C.
- 🧭 The magnitude of the resultant vector C was previously calculated, but the focus here is on its direction.
- 📐 The direction of C with respect to the x-axis is sought using trigonometric methods.
- 📈 The angle between the vectors is determined using the tangent of the angle, which equals the opposite side over the adjacent side in a right triangle.
- 📊 The first method involves finding the angle between vector A and the x-axis by using the inverse tangent of the ratio of A's magnitude to B's magnitude.
- 📉 The second method involves finding the angle between vector B and vector A, and then subtracting it from 90 degrees to find the angle with the x-axis.
- 🔢 The calculations result in an angle of 60 degrees for the direction of the resultant vector C with respect to the x-axis.
- 🔚 The script concludes that the resultant vector makes an angle of 60 degrees with the x-axis, confirmed by two different methods.
Q & A
What are vectors A and B in the given scenario?
-Vector A is eight units north, and vector B is four point five units east.
How is vector A represented in the script?
-Vector A is represented in red and has a magnitude of eight units in the north direction.
What is the method used to find the resultant vector C?
-The tail-to-tip method is used to add vectors A and B to find the resultant vector C.
What is the magnitude of the resultant vector C?
-The magnitude of C is not explicitly stated in the script, but it can be calculated using the Pythagorean theorem from the components of A and B.
What is the purpose of finding the direction of the resultant vector C?
-The direction of the resultant vector C is found to determine the angle it makes with respect to the x-axis.
How is the angle θ related to vectors A and B?
-The angle θ is found using the tangent function, where θ equals the inverse tangent of the ratio of the magnitudes of A and B.
What are the two methods described in the script to find the angle of the resultant vector C with respect to the x-axis?
-The first method uses the geometric property that the angles between the vectors are equal. The second method involves finding the angle between vector A and the resultant vector C, and then subtracting it from 90 degrees.
What is the calculated angle θ using the first method?
-Using the first method, the angle θ is found to be 60 degrees using the inverse tangent of 8 units over 4.5 units.
What is the calculated angle θ using the second method?
-Using the second method, the angle θ is initially found to be approximately 30 degrees, and then adjusted to 60 degrees by subtracting from 90 degrees.
Why are two different methods used to verify the angle of the resultant vector C?
-Two different methods are used to provide verification and ensure the accuracy of the result, showing that the angle of the resultant vector C with respect to the x-axis is indeed 60 degrees.
What is the significance of the angle between the x-axis and the resultant vector C?
-The angle between the x-axis and the resultant vector C is significant as it provides the direction of the combined effect of vectors A and B in the context of a coordinate system.
Outlines
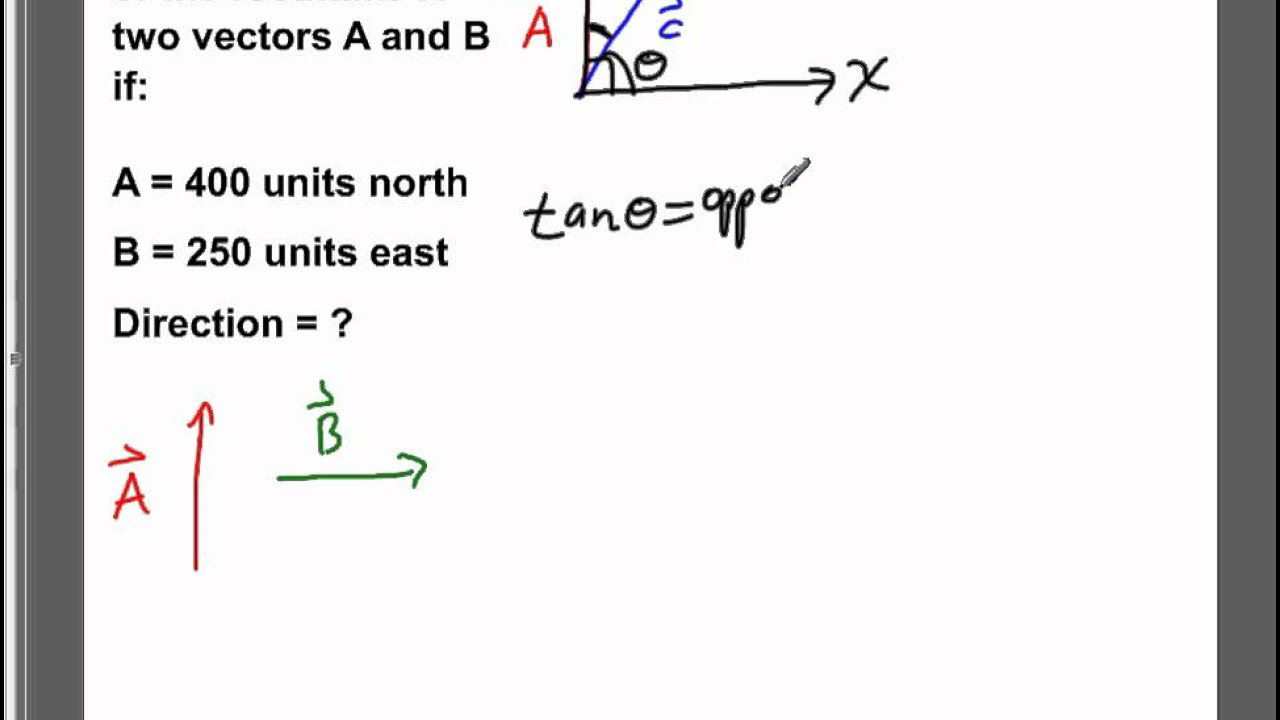
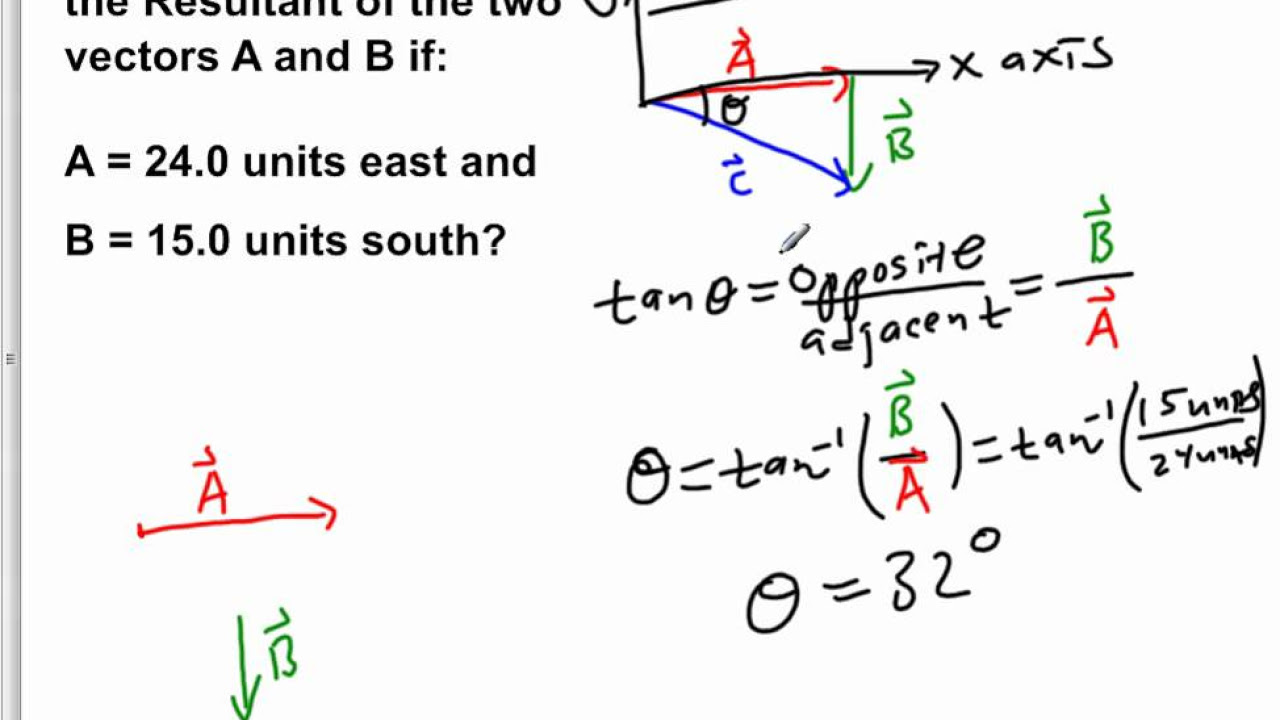
📚 Vector Addition and Resultant Direction
This paragraph explains the process of adding two vectors, A and B, where A is 8 units north and B is 4.5 units east. The script describes drawing these vectors using the tail-to-tip method and finding the resultant vector C. It then discusses two methods to find the direction of C with respect to the x-axis. The first method involves using the geometry properties of the angles formed by the vectors, while the second method involves calculating the angle between vector A and C. Both methods conclude that the angle of the resultant vector C with respect to the x-axis is 60 degrees.
🔍 Determining the Resultant Vector's Angle with the X-axis
In this paragraph, the focus is on determining the angle that the resultant vector C makes with the x-axis. It clarifies that since the y-axis is perpendicular to the x-axis, and vector A is parallel to the y-axis, the angle between A and C is 30 degrees. By subtracting this from 90 degrees, the angle between C and the x-axis is found to be 60 degrees. This paragraph reinforces the conclusion from the previous paragraph, confirming that the resultant vector C makes an angle of 60 degrees with the x-axis.
Mindmap
Keywords
💡Resultant Vector
💡Tail-to-Tip Method
💡Magnitude
💡Direction
💡Tangent
💡Inverse Tangent
💡Trigonometry
💡Cartesian Coordinate System
💡Perpendicular
💡Right Angle
Highlights
Introduction to the problem of finding the direction of the resultant vector of two given vectors.
Vector A is defined as eight units north.
Vector B is defined as four point five units east.
Illustration of vector addition using the tail-to-tip method.
Resultant vector C is introduced as the sum of vectors A and B.
Magnitude of resultant vector C was previously calculated.
Current focus is on determining the direction of vector C with respect to the x-axis.
Explanation of using geometric properties to find the angle between vectors.
Method 1 involves finding the angle between vector A and the x-axis.
Method 2 involves finding the angle between vector B and the x-axis.
Use of the tangent function to calculate the angle theta.
Calculation of theta as the arctan of the ratio of vector A to vector B.
Theta is found to be 60 degrees using the first method.
Theta is calculated to be 30 degrees using the second method.
Explanation of the relationship between the angles to find the resultant's direction.
Final conclusion that the resultant vector makes a 60-degree angle with the x-axis.
Demonstration of two different methods arriving at the same conclusion.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: