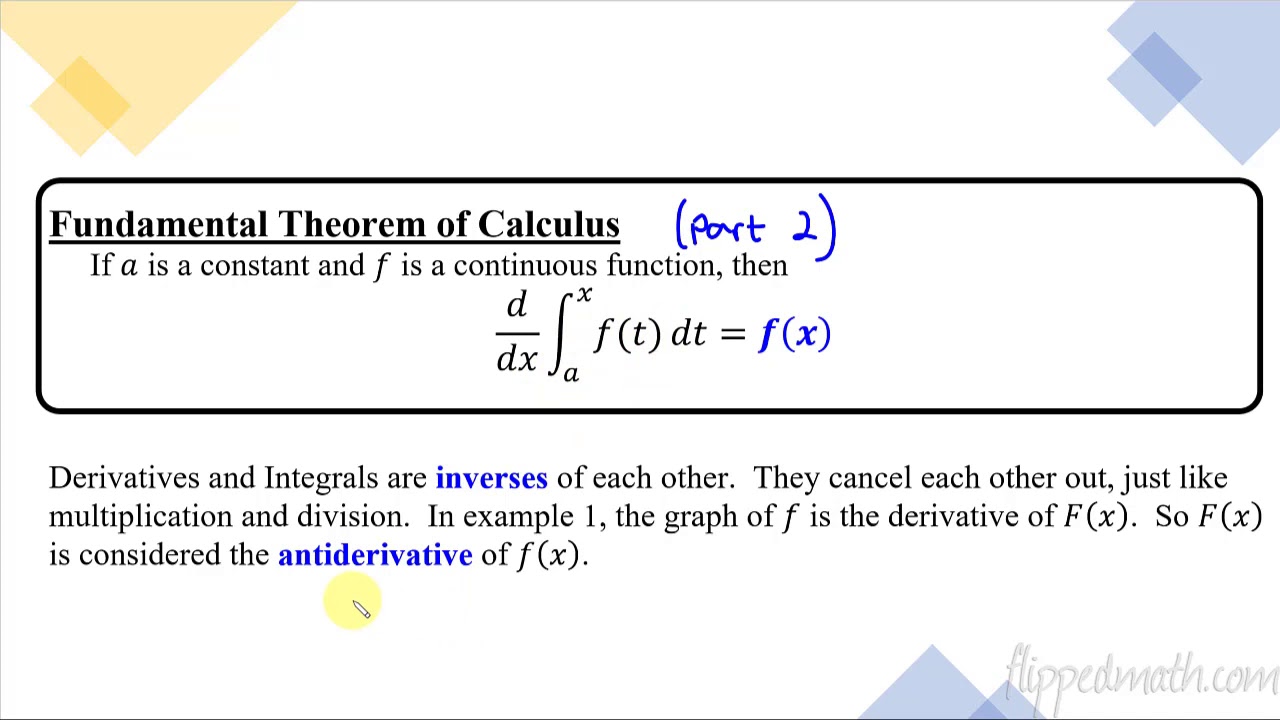
FTC #2
TLDRThis video script delves into the Second Fundamental Theorem of Calculus, illustrating how to differentiate integrals with ease. It demonstrates the process of 'working inside out' to find derivatives of integrals, highlighting the inverse relationship between these operations. The script uses examples to clarify the concept, including the application of the chain rule when the upper limit of integration is a function of the variable. It also discusses how to identify relative maxima, minima, and points of inflection, using the derivative to analyze the behavior of functions defined by integrals, which is a common topic in AP Calculus exams.
Takeaways
- 🔍 The second fundamental theorem of calculus (FTC Part 2) involves taking derivatives of integrals.
- 📈 Functions defined by integrals can be analyzed for increasing or decreasing behavior and points of inflection.
- 🧩 To take the derivative of an integral, replace the variable in the integrand with the upper limit of integration.
- 🚀 Derivatives and integrals are inverse operations, with the derivative of an integral essentially canceling out the integral.
- 🔄 The shortcut for taking the derivative of an integral involves replacing the variable in the function with the upper limit of integration and multiplying by the derivative of the upper limit if it's a function of x.
- ⚠️ If the lower limit of the integral is a function of x, the result is negated.
- 🔗 The chain rule is used when the upper limit of integration is a function of x, requiring multiplication by the derivative of that function.
- 🔧 The second fundamental theorem of calculus states that the derivative of the integral from a constant to a function of x of f(t) dt is f(g(x)) times the derivative of g(x).
- 📊 In applied problems, such as on the AP Calculus exam, understanding the behavior of functions defined by integrals is crucial for finding values, extrema, and points of inflection.
- 📝 Critical points and inflection points are determined by examining the behavior of the function's derivative, which may involve analyzing changes in sign or direction.
Q & A
What is the main focus of the video script?
-The video script focuses on explaining the Second Fundamental Theorem of Calculus, demonstrating how to take the derivative of an integral and applying this concept to various examples.
Why is it necessary to take the derivative of an integral in calculus?
-Taking the derivative of an integral is necessary to analyze functions defined by integrals, such as determining when the function is increasing or decreasing, or finding points of inflection.
What does the script mean by 'working inside out'?
-'Working inside out' refers to the process of first evaluating the integral and then taking the derivative at the end, which is a method demonstrated in the script to simplify the calculation.
Can you explain the concept of 'replacing T with X' as mentioned in the script?
-The concept of 'replacing T with X' comes from the Fundamental Theorem of Calculus, which states that when you take the derivative of an integral, the variable of integration (T) is replaced by the variable of the function (X).
What is the significance of the derivative and integral being inverse functions of each other as per the script?
-The significance is that the derivative and integral are inverse operations that cancel each other out when applied sequentially, leaving only the substitution of the variable of integration with the variable of the function.
How does the script illustrate the concept of the chain rule in the context of the Second Fundamental Theorem of Calculus?
-The script illustrates the chain rule by showing that when the upper limit of integration is a function of X, the derivative of that function must be multiplied to the result of the integral after replacing the variable of integration with X.
What is the purpose of the example where the integral has a variable on the bottom, as opposed to the top?
-The purpose is to demonstrate that when the variable is on the bottom, the integral can be flipped, and the process involves negating the integral and understanding that the derivative of a constant is zero.
Why is it said that the derivative of a number is zero, as mentioned in the script?
-The derivative of a number is zero because the derivative measures the rate of change, and a constant number does not change; hence, its rate of change is zero.
What is the application of the Second Fundamental Theorem of Calculus in the context of the AP Calculus exam?
-The application includes solving free-response questions that require understanding the relationship between derivatives and integrals, such as finding the maximum and minimum values of a function defined by an integral or determining points of inflection.
How does the script use the Second Fundamental Theorem of Calculus to find the relative maximum of a function G defined by an integral?
-The script finds the relative maximum by taking the derivative of G, which is equal to the function F inside the integral, and identifying where this derivative crosses the x-axis from positive to negative, indicating a relative maximum.
What is the significance of the absolute minimum value of G and the value at which it occurs, as discussed in the script?
-The significance lies in understanding the behavior of the function G over its domain. By finding the absolute minimum value and the point at which it occurs, one can analyze the overall trend and performance of the function, which is crucial for optimization problems.
Outlines
📚 Introduction to the Second Fundamental Theorem of Calculus
The script begins with an introduction to the second fundamental theorem of calculus, often referred to as FTC part two. The presenter aims to explore the concept of taking the derivative of an integral, a process that might seem counterintuitive but is essential for analyzing functions defined by integrals. The main goal is to understand the behavior of these functions, such as their increasing or decreasing nature and points of inflection, by differentiating them. The explanation includes a step-by-step demonstration using specific integrals and emphasizes the 'working inside out' approach, which simplifies the process by replacing the variable of integration with the upper limit of integration and then taking the derivative.
🔍 Applying the Second Fundamental Theorem with the Chain Rule
This paragraph delves into the application of the second fundamental theorem of calculus in conjunction with the chain rule. The presenter illustrates how to handle integrals where the upper limit is a function of the variable, emphasizing the importance of multiplying by the derivative of that function. Several examples are provided to demonstrate the process, showing how the derivative and integral operations cancel each other out, leaving only the substitution of the variable and the application of the chain rule where necessary. The explanation clarifies that the lower limit of the integral does not affect the derivative and that the process simplifies to a straightforward substitution once the concept is grasped.
📉 Analyzing the Graph and Calculating Function Values
The script continues with an analysis of a graph representing a function 'f' and defines another function 'G' as an integral from 0 to 'X' of 'f' with respect to 'T'. The presenter calculates specific values of 'G' at different points, such as 'G' of 0, 'G' of -1, and 'G' of 2, interpreting these as areas under the curve. The explanation covers how to handle negative limits by flipping the integral and understanding the geometric representation of these areas. The presenter also discusses the behavior of 'G' as it increases and decreases, providing a detailed account of the signed areas contributing to the function's value at various points.
🔍 Finding Extremes and Points of Inflection
In this section, the presenter focuses on finding the relative maximum and absolute minimum values of the function 'G', as well as the points of inflection. The process involves understanding the derivative of 'G', which is equal to 'f', and identifying where this derivative crosses the x-axis, indicating a relative maximum. The absolute minimum is found by considering endpoints and critical points, with the presenter using a candidate test to list values and determine the smallest one. Points of inflection are then discussed, which occur where the second derivative, or the derivative of 'G', changes sign, indicating a change in the direction of the slope of 'G'. The presenter argues the direction changes at specific points and provides justification for these points being points of inflection.
Mindmap
Keywords
💡Second Fundamental Theorem of Calculus
💡Derivative
💡Integral
💡Antiderivative
💡Chain Rule
💡Function of X
💡Relative Maximum
💡Absolute Minimum
💡Point of Inflection
💡Net Signed Area
Highlights
Introduction to the Second Fundamental Theorem of Calculus (FTC).
Exploring the concept of taking the derivative of an integral.
Understanding when a function defined by an integral is increasing or decreasing.
Demonstration of integrating T squared from one to X and then taking the derivative.
Explanation of replacing T with X in the integral.
Derivative of x cubed over 3 resulting in x squared.
Integration of cosine and its derivative.
Derivative of sine resulting in cosine.
Insight on derivatives and integrals being inverse functions.
Shortcut for taking the derivative of an integral from a to X.
Handling integrals with a variable lower limit.
Application of the chain rule in the context of the Second Fundamental Theorem of Calculus.
Example of using the chain rule with a function of X as the upper limit.
Free response question example on the AP calculus exam.
Finding values of G for different X values.
Determining the relative maximum of G.
Calculating the absolute minimum value of G.
Identifying points of inflection for the graph of G.
Transcripts
Browse More Related Video
Calculus AB/BC – 6.4 The Fundamental Theorem of Calculus and Accumulation Functions
Fundamental Theorem of Calculus FRQs

Examples of Using the Second Fundamental Theorem of Calculus (2nd FTC)

Derivatives of Integrals (w/ Chain Rule)

The Second Fundamental Theorem of Calculus
Fundamental Theorem of Calculus Part 1
5.0 / 5 (0 votes)
Thanks for rating: