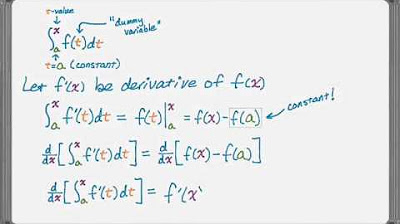Examples of Using the Second Fundamental Theorem of Calculus (2nd FTC)
TLDRThe video script provides a comprehensive walkthrough of the Second Fundamental Theorem of Calculus through several examples. The presenter assumes prior knowledge of the theorem and focuses on applying it to find derivatives of integrals. The examples illustrate how to replace the variable in the integrand with a function of 'X' when the upper bound is a function of 'X', and then apply the chain rule to multiply by the derivative of that bounding function. The script emphasizes the ease of using the Second Fundamental Theorem over the First, as it avoids the need to find antiderivatives. The examples cover a range of scenarios, including when the upper bound is not a function of 'X', requiring the bounds to be swapped and the sign changed. The presenter's approach is practical, aiming to give viewers a clear understanding of the theorem's application in calculus.
Takeaways
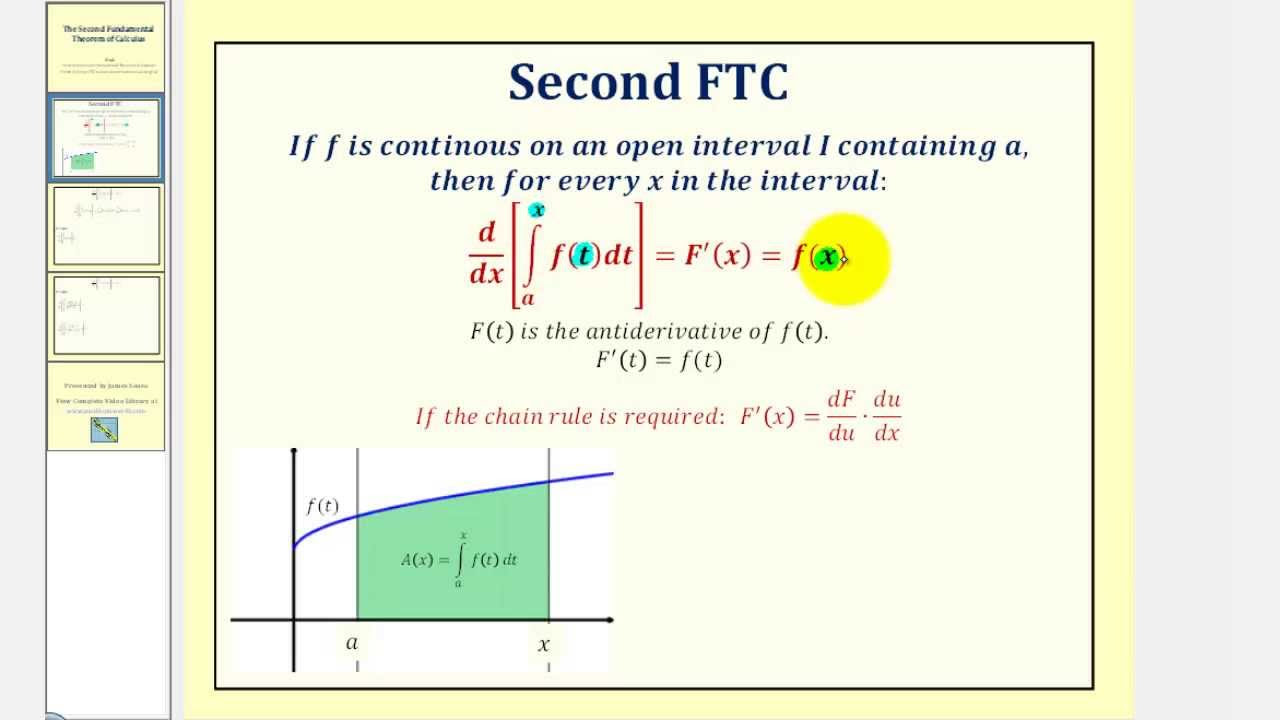
- 📚 The second fundamental theorem of calculus is used to find the derivative of a definite integral where the bounds include a variable.
- 🔑 When applying the theorem, ensure that one of the bounds of the integral is a constant, and the other is a function of the variable with respect to which you're differentiating.
- 📐 The dummy variable (often 't') in the integrand is replaced with the variable representing the upper bound of integration.
- 🔄 The chain rule is applied by multiplying the integrand by the derivative of the upper bounding function.
- 🧮 For the integral from a constant to a function of 'x', the derivative of the integral is simply the function evaluated at 'x', multiplied by its derivative.
- 🔢 If the upper bound is not a function of 'x', use properties of integrals to rewrite the integral with the bounds swapped and change the sign accordingly.
- ⛓ When the lower bound is a function, swap the bounds and adjust the sign of the integral before applying the second fundamental theorem.
- 🌟 The derivative of e^(x^n) is e^(x^n) * n * x^(n-1), which is used when the upper bound is an exponential function of 'x'.
- 📉 If the upper bound is a constant, like in the example with the integral from 'x' to 4, you can rewrite the integral to have the variable as the lower bound and apply the theorem.
- 🎓 The second fundamental theorem of calculus is considered easier to use than the first because it doesn't require finding antiderivatives.
- 📈 Understanding the process of applying the second fundamental theorem of calculus helps in grasping the concept of differentiation under the integral sign.
Q & A
What is the Second Fundamental Theorem of Calculus?
-The Second Fundamental Theorem of Calculus establishes the relationship between integration and differentiation. It states that if a function is integrable on the closed interval [a, b] and F is an antiderivative of f on [a, b], then the definite integral from a to b of f(x) dx is F(b) - F(a).
What is a dummy variable in the context of integration?
-A dummy variable is a temporary variable used in the integrand when dealing with definite integrals. It does not affect the final result and is often represented by t, u, or v.
How does the chain rule apply to the Second Fundamental Theorem of Calculus?
-The chain rule is applied when differentiating the integrand with respect to x, where the upper bound of integration is a function of x. It allows us to find the derivative of the integral by multiplying the derivative of the upper bounding function by the integrand evaluated at that function.
Why is it necessary for the lower bound of integration to be constant?
-The lower bound of integration needs to be constant because the Second Fundamental Theorem of Calculus deals with differentiation with respect to a single variable, which is x in this case. If the lower bound were a function of x, it would complicate the differentiation process.
What happens when the upper bound of integration is not a function of x?
-If the upper bound of integration is not a function of x, you can often manipulate the integral by swapping the bounds and changing the sign of the integral, as demonstrated in the script with the example involving the integral from x to 4.
What is the derivative with respect to X of the integral from 5 to X of cosine of 2t dt?
-The derivative with respect to X of the integral from 5 to X of cosine of 2t dt is the cosine of 2x, as per the Second Fundamental Theorem of Calculus and the application of the chain rule.
How do you find the derivative of the integral from 0 to x squared of cosine of 2t dt?
-You replace the variable t with the function x squared in the integrand, then multiply by the derivative of the upper bounding function (which is 2x), resulting in 2x * cosine of 2x squared.
What is the derivative with respect to X of the integral from negative 1 to e to the X cubed of cosine of 2t dt?
-The derivative is found by replacing t with e to the X cubed in the integrand, then multiplying by the derivative of the upper bounding function (which is 3x squared times e to the X cubed), resulting in 3x squared * e to the X cubed * cosine of 2e to the X cubed.
Why is the Second Fundamental Theorem of Calculus considered easier to use than the First?
-The Second Fundamental Theorem of Calculus is considered easier to use because it does not require finding antiderivatives. Instead, it directly provides a method to find the derivative of a definite integral by applying the chain rule and differentiating the integrand with respect to the variable of interest.
What is the final example's derivative with respect to X of the integral from x to 4 of cosine of 2t dt?
-The final example's derivative is found by swapping the bounds and changing the sign, resulting in the negative cosine of 2x, and then multiplying by the derivative of the upper bounding function, which is 1, so the final answer is -cosine of 2x.
Why is it important to understand the Second Fundamental Theorem of Calculus?
-Understanding the Second Fundamental Theorem of Calculus is important because it provides a powerful tool for finding derivatives of integrals, which is a common task in calculus and its applications, including physics and engineering.
Outlines
📚 Introduction to the Second Fundamental Theorem of Calculus
This paragraph introduces the topic of the video, which is to work through examples of the Second Fundamental Theorem of Calculus. The speaker assumes that the audience has prior knowledge of the theorem and will focus on solving examples while explaining the process. The emphasis is on the use of dummy variables and the application of the chain rule in finding derivatives with respect to X of integral functions.
Mindmap
Keywords
💡Second Fundamental Theorem of Calculus
💡Derivative
💡Integral
💡Dummy Variable
💡Chain Rule
💡Cosine Function
💡Constant Lower Bound
💡Function of X
💡Exponential Function
💡Antiderivative
💡Definite Integrals
Highlights
Introduction to the second fundamental theorem of calculus with examples.
Assumption that the viewer is already familiar with the fundamental theorem of calculus.
Derivative with respect to X of the integral from 5 to X of cosine of 2t DT is demonstrated.
Use of dummy variables in the integrand and their replacement with a function of X.
Application of the chain rule to multiply by the derivative of the upper bounding function.
Final answer for the first example is the cosine of 2x.
Second example involves the integral from 0 to x squared of cosine of 2t DT.
Emphasis on the lower bound being a constant for the application of the theorem.
Derivative of the upper bounding function is multiplied by the integrand in the chain rule.
Third example with the integral from negative 1 to e to the X cubed of cosine of 2t DT.
Explanation of replacing the variable in the integrand with the upper bounding function.
Derivative of e to the X cubed is e to the X cubed times 3x squared.
Fourth example with the integral from x to 4 of cosine of 2t DT and the need to swap bounds.
Use of the property of definite integrals to swap bounds and change the sign.
Solution involves negative cosine of 2x multiplied by the derivative of the upper bounding function.
Discussion on the ease of using the second fundamental theorem of calculus compared to the first.
Mention of not needing to find antiderivatives when using the second fundamental theorem.
Conclusion and encouragement for viewers to apply the theorem effectively.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: