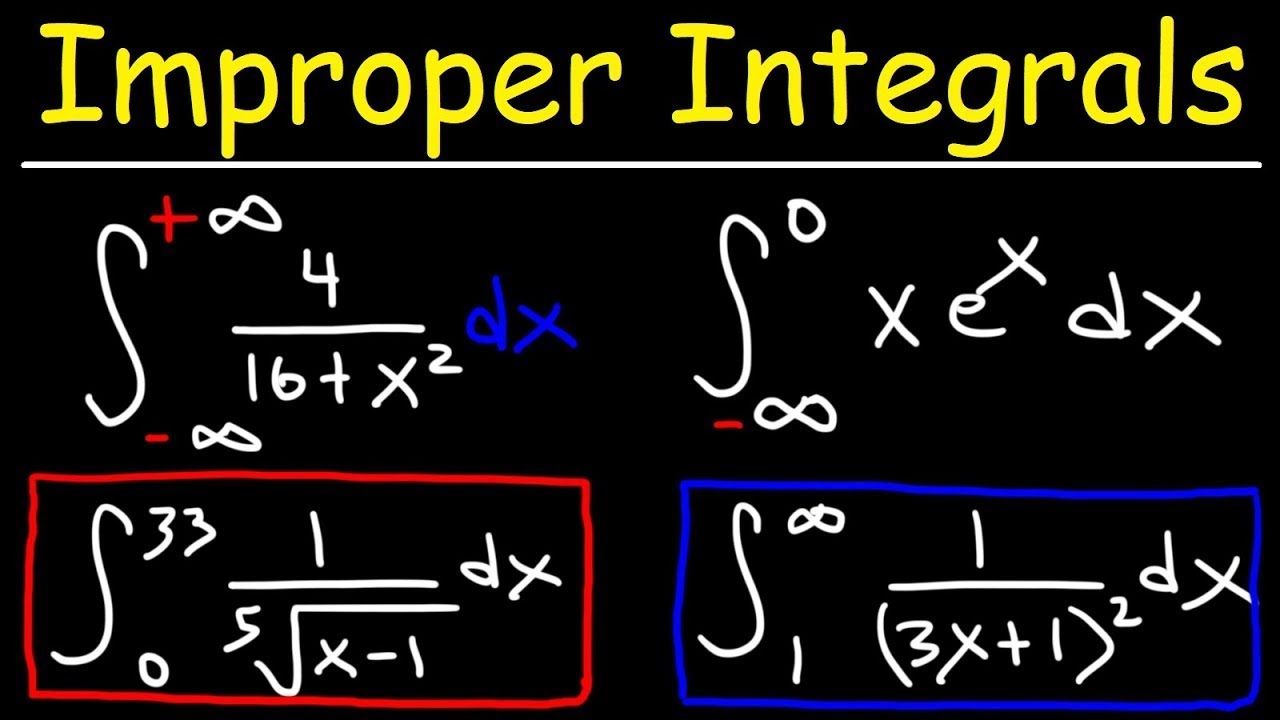
Improper Integrals - Discontinuity at a Boundary

turksvids
8 Jun 201403:55
EducationalLearning
32 Likes 10 Comments

TLDRThis video script delves into the concept of improper integrals, outlining three main types that arise from discontinuities at the limits of integration or within the interval itself. The fourth, more complex scenario, involves a combination of these issues. The script provides step-by-step examples of the first type, demonstrating how to evaluate improper integrals by taking limits and applying the fundamental theorem of calculus. The examples illustrate convergence to a finite value and divergence to infinity, offering a clear understanding of how to handle these integrals in calculus.

Takeaways
  • 📚 There are three types of improper integrals, with a fourth being a combination of the others, which is particularly complex.
  • 🔍 Improper integrals arise when the function is discontinuous at the limits of integration, or when the limits themselves are infinite.
  • 📉 The first type of improper integral involves a function that is discontinuous at either the lower limit 'a' or the upper limit 'b'.
  • 🔄 To handle improper integrals, limits are used to evaluate the integral by approaching the point of discontinuity.
  • 🌐 An example given is the integral from 0 to 1 of 1/√x dx, which is improper because the function is discontinuous at x=0.
  • 📝 The integral is rewritten using the limit as 'a' approaches zero from the right, and the anti-derivative is found using the fundamental theorem of calculus.
  • 🔢 The solution to the first example converges to the value of 2, indicating that the integral has a finite value.
  • 🚫 A second example involves the integral from 0 to 5 of (5 - x^3)^(-1), which is improper due to discontinuity at x=5.
  • 📉 The integral is evaluated using the limit as 'b' approaches 5 from the left, and the chain rule is applied to find the anti-derivative.
  • 💥 The second example diverges to infinity, meaning the integral is unbounded and grows without limit.
  • 🔄 The video promises to cover the second type of improper integral in a follow-up video, suggesting a series of educational content.
Q & A

  • What are the three types of improper integrals discussed in the script?

    -The script discusses three types of improper integrals: 1) The function 'f(x)' could be discontinuous at either the lower limit 'A' or the upper limit 'B'. 2) 'f(x)' could be discontinuous at some value 'C' between 'A' and 'B'. 3) Either 'A' or 'B' or both could be infinite, meaning the integral could range from negative infinity to positive infinity.

  • What is the fourth case scenario of improper integrals mentioned in the script?

    -The fourth case scenario is a combination of the first three, which is considered particularly challenging because it involves multiple issues of discontinuity and infinite limits.

  • How does the script suggest dealing with improper integrals?

    -The script suggests dealing with improper integrals by taking limits. This involves evaluating the limit as the variable approaches the point of discontinuity or infinity.

  • What is the integral in the first example provided in the script?

    -The first example integral is from 0 to 1 of √x dx, where the function is discontinuous at x=0.

  • Why is the function in the first example discontinuous at x=0?

    -The function 1/√x is discontinuous at x=0 because the square root of zero is defined, but as x approaches zero from the right, the function's value grows without bound.

  • How is the integral in the first example rewritten to make taking the anti-derivative easier?

    -The integral is rewritten as x^(1/2) to simplify the process of finding the anti-derivative.

  • What is the result of the first improper integral example in the script?

    -The result of the first improper integral example is 2, indicating that it converges to the value of two.

  • What is the integral in the second example provided in the script?

    -The second example integral is from 0 to 5 of (5 - x^3) or 1/(5 - x^3) dx, where the function is discontinuous at x=5.

  • Why is the function in the second example discontinuous at x=5?

    -The function 1/(5 - x^3) is discontinuous at x=5 because the denominator becomes zero, which makes the function undefined at that point.

  • What is the result of the second improper integral example in the script?

    -The result of the second improper integral example is that it diverges to infinity, meaning it is unbounded and keeps growing.

  • What does the script suggest will be covered in the next video?

    -The script suggests that in the next video, the second type of improper integral will be discussed.

Outlines
00:00
📚 Introduction to Improper Integrals

This paragraph introduces the concept of improper integrals, explaining that they can occur in three main scenarios: discontinuity at the limits of integration (A or B), discontinuity within the interval (at some point C), or when the limits of integration are infinite (from negative to positive infinity). The fourth scenario is a combination of these issues, which is particularly complex. The paragraph sets the stage for a deeper exploration of improper integrals through examples.

🔍 Analyzing the First Type of Improper Integral

The speaker delves into the first type of improper integral, where the function may be discontinuous at the limits of integration. Two examples are provided to illustrate the concept. The first example involves the integral from 0 to 1 of 1/√x dx, where the function is discontinuous at x=0. The solution involves taking the limit as x approaches 0 from the right and simplifying the integral to find that it converges to the value of 2. The second example is the integral from 0 to 5 of (5 - x^3)/5 - x^2 dx, which has a discontinuity at x=5. The solution process involves taking the limit as x approaches 5 from the left and applying the chain rule and reverse power rule to find that this integral diverges to infinity. The paragraph concludes with a promise to discuss the second type of improper integral in a subsequent video.

Mindmap
Keywords
💡Improper Integrals
Improper integrals are a type of definite integral that cannot be computed using the standard techniques due to the presence of an infinite limit of integration or a discontinuity within the interval of integration. In the video, the concept is introduced as having three types, with a fourth being a combination of these, which is considered particularly challenging. The script discusses these types and provides examples to illustrate how to approach them using limits.
💡Discontinuous
A function is said to be discontinuous at a point if it does not have a limit there. In the context of the video, discontinuity is one of the reasons that can make an integral improper. The script mentions that a function could be discontinuous at the limits of integration (A or B) or at some point C within the interval [A, B].
💡Limits
In calculus, limits are a fundamental concept used to define the behavior of a function as its input approaches a certain value. In the video, limits are used to evaluate improper integrals by taking the limit as the variable approaches the point of discontinuity or infinity. For example, the script describes taking the limit as 'a' approaches zero from the right for the integral of 1/√x from 0 to 1.
💡Fundamental Theorem of Calculus
The Fundamental Theorem of Calculus is a key result that links the concept of integration with differentiation. It is used in the video to evaluate definite integrals by finding antiderivatives. The script mentions using this theorem to find the antiderivative of the function 1/√x, which simplifies the process of evaluating the improper integral.
💡Antiderivatives
An antiderivative is a function whose derivative is the original function. In the context of the video, antiderivatives are found to evaluate improper integrals by using the Fundamental Theorem of Calculus. The script provides the process of finding the antiderivative of √x, which is used to simplify the evaluation of the integral from 0 to 1 of √x dx.
💡Convergence
In the context of improper integrals, convergence refers to the property of an integral where the value approaches a finite limit. The video discusses how some improper integrals converge to a finite value, such as the integral of √x from 0 to 1, which converges to 2.
💡Divergence
Divergence, in contrast to convergence, is when an integral does not approach a finite value but instead grows without bound. The video provides an example of an improper integral that diverges to infinity, such as the integral of 1/(5 - x)^(3/2) from 0 to 5, which becomes unbounded as x approaches 5 from the left.
💡Chain Rule
The Chain Rule is a fundamental principle in calculus for differentiating composite functions. In the video, the Chain Rule is mentioned in the context of finding the antiderivative of a function, which involves differentiating a function of another function, such as in the integral from 0 to 5 of 1/(5 - x)^(3/2) dx.
💡Power Rule
The Power Rule is a basic principle in calculus for differentiating functions of the form x^n, where n is a constant. In the video, the script refers to the Power Rule in the context of simplifying the integral and finding the antiderivative of a function, such as in the integral from 0 to 5 of 1/(5 - x)^(3/2) dx.
💡Reciprocal
The reciprocal of a number is 1 divided by that number. In the video, the reciprocal is used in the process of evaluating improper integrals, particularly when dealing with functions that have a discontinuity at the limits of integration. An example from the script is the integral from 0 to 1 of 1/√x dx, where the reciprocal is used to simplify the expression before taking the limit.
Highlights

Introduction to improper integrals and their three types.

Definite integrals can be improper based on the relationship between a, b, and f(x).

First type of improper integral: f(x) could be discontinuous at either A or B.

Second type of improper integral: f(x) could be discontinuous at some value C between a and b.

Third type of improper integral: A or B or both could be infinite (e.g., negative or positive infinity).

Fourth type of improper integral: a combination of the first three issues.

Example of the first type: integral from 0 to 1 of √x dx.

Dealing with improper integrals by taking limits, specifically approaching zero from the right in the first example.

Rewriting the function as x^(1/2) to simplify the anti-derivative process.

Using the fundamental theorem of calculus to evaluate the improper integral.

Result of the first example: the improper integral converges to the value of 2.

Second example: integral from 0 to 5 of (5 - x^3) or 1/(5 - x^3).

Discontinuity at x = 5 in the second example, approaching from the left.

Rewriting the function and applying the chain rule and reverse power rule.

Evaluating the limit as B approaches 5 from the left.

Result of the second example: the integral diverges to infinity, indicating unbounded growth.

Discussion of the first type of improper integral with examples and their outcomes.

Promise to cover the second type of improper integral in a follow-up video.

Transcripts

Browse More Related Video

Improper Integrals - Convergent or Divergent (Made Easy)

AP Calculus BC Lesson 6.13

Improper Integrals

Improper Integrals - Convergence and Divergence - Calculus 2

Calculus Chapter 3 Lecture 27 Improper Integrals

Improper Integrals BC Calc

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Improper IntegralsMathematicsCalculusDiscontinuityLimitsIntegralsEducationalCalculus TutorialMath AnalysisInfinite Limits