Calculus Chapter 3 Lecture 27 Improper Integrals

Penn Online Learning

23 Jun 201616:32

EducationalLearning

32 Likes 10 Comments

TLDRIn Lecture 27 on calculus, Professor Greist explores the limitations of the fundamental theorem of integral calculus, focusing on improper integrals. He explains the challenges posed by discontinuous integrands and infinite intervals, introducing the technique of taking limits to evaluate these integrals. The lecture delves into 'P integrals', highlighting when they converge or diverge, and uses them to assess the convergence of more complex integrals. The importance of understanding improper integrals for solving real-world problems is emphasized, with practical examples provided.

Takeaways

📚 The fundamental theorem of calculus has limitations, particularly regarding the continuity of the integrand and the finiteness of the interval.
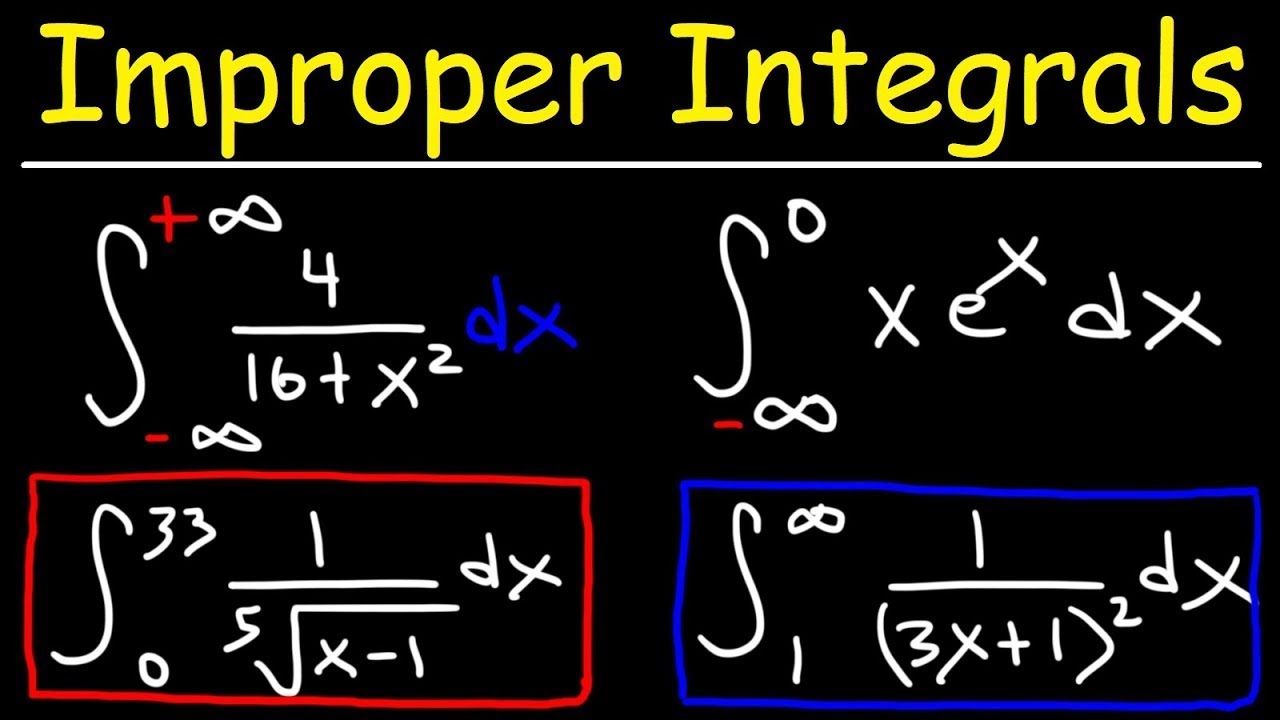
⚠️ Discontinuous integrands can cause problems, as demonstrated by the integral of 1/x^2 from -1 to 1, which is not defined at x=0.
🔍 The improper integrals, such as those with infinite limits or undefined points, require the technique of taking limits to evaluate.
📉 There are two main cases of improper integrals: 'blow up' at an endpoint and 'tail' where the integration domain extends to infinity.
🌐 The 'p' integrals, integrals of 1/x^p, are crucial for understanding convergence and divergence of improper integrals.
🔑 For 'p' integrals, convergence depends on the value of 'p': converges for p > 1 with a tail singularity, diverges for p ≤ 1; reverse for a blow-up singularity.
📈 The integral of 1/√(x^2 + x) from 0 to 1 converges because the leading order term is a 'p' integral with p = 1/2, which is convergent.
📉 The same integrand diverges as x goes to infinity because the leading order term becomes a 'p' integral with p = 1, indicating divergence.
🤔 Memorizing the behavior of 'p' integrals is essential for determining the convergence or divergence of other integrals without easily computable anti-derivatives.
❗ Double limits in integrals over the entire real number line require careful consideration and separate evaluation of each limit.
📚 The lecture emphasizes the importance of practice in understanding improper integrals, which are often challenging for students.

Q & A

What is the fundamental theorem of integral calculus, and what are its limitations?
-The fundamental theorem of integral calculus relates the derivative and the integral, allowing for the calculation of definite integrals by finding antiderivatives. Its limitations include the requirement that the integrand must be continuous on the interval from A to B and that the interval must be finite.
Why can't we use the fundamental theorem directly on the integral of 1/x^2 from -1 to 1?
-The fundamental theorem cannot be directly applied because the integrand, 1/x^2, is not continuous over the interval, specifically it is undefined at x=0, leading to a contradiction when evaluating the integral as a Riemann sum.
What is the correct approach to evaluate an improper integral with an infinite interval?
-For improper integrals with infinite intervals, the correct approach is to take a limit by integrating over a finite domain up to a constant T and then taking the limit as T approaches infinity.
What are the two types of improper integrals discussed in the script?
-The two types of improper integrals are 'blow up', where the integrand is not well defined at an endpoint, and 'tail', where the integration domain extends to infinity.
How do you determine the convergence of a p-integral of the form 1/x^p?
-The convergence of a p-integral is determined by evaluating the limit as T approaches infinity for a tail singularity or as T approaches 0 for a blow-up singularity. It converges when p > 1 for a tail and when p < 1 for a blow-up, and it diverges otherwise, especially when p equals 1.
What is the antiderivative of 1/(1 + x^2)dx over a symmetric domain?
-The antiderivative of 1/(1 + x^2)dx is the natural logarithm of the absolute value of (1 + x^2), and when evaluated over a symmetric domain around the origin, the result is 0 due to the odd symmetry of the integrand.
Why does the integral of dx/√(x^2 + x) from 0 to 1 converge?
-The integral converges because the leading order behavior near the blow-up singularity at x=0 is x^(-1/2), which is a convergent p-integral for p=1/2.
What is the behavior of the integral of dx/√(x^2 + x) as x approaches infinity?
-As x approaches infinity, the leading order term becomes 1/x, indicating a divergent integral because it resembles a p-integral with p=1, which always diverges.
What is the issue with evaluating double limits for integrals over the entire real number line?
-Evaluating double limits for integrals over the entire real number line can lead to incorrect conclusions. One must take two independent limits, one for the lower bound going to negative infinity and one for the upper bound going to positive infinity, to properly assess convergence.
Why does the integral of 1/x over the entire real number line not converge to 0?
-The integral of 1/x over the entire real number line diverges because the leading order term for large x is 1/x, which is a p-integral with p=1, indicating divergence.
What is the importance of understanding the convergence or divergence of improper integrals?
-Understanding the convergence or divergence of improper integrals is crucial for determining the validity of the integral's result. It helps in solving real-world problems where the function may not be well-behaved over the entire domain of interest.

Outlines

00:00

📚 Introduction to Improper Integrals

Professor Greist introduces the concept of improper integrals, discussing the limitations of the fundamental theorem of integral calculus. He highlights the importance of the integrand being continuous over a finite interval from A to B. The lecture provides examples of improper integrals, such as the integral of 1/x^2 from -1 to 1, and explains why it results in a negative value, which contradicts the definition of a definite integral. The professor also discusses the case of infinite intervals, using the integral of 2x/(1+x^2) from negative to positive infinity as an example, and explains how to handle these cases by taking limits.

05:04

🔍 Analyzing P-Integrals and Their Convergence

The professor delves into the specifics of P-integrals, which are integrals of the form 1/x^p. He examines two types of singularities: 'blow-up' at an endpoint and 'tail' at infinity. For each case, he explains how to evaluate the integral by taking limits as the variable approaches the singularity or infinity. The lecture provides a comprehensive analysis of when these integrals converge or diverge, depending on the value of p. The professor emphasizes the importance of understanding these cases to determine the convergence or divergence of other, more complex integrals.

10:05

🔬 Applying Binomial Series to Improper Integrals

In this segment, Professor Greist applies the binomial series to analyze the behavior of improper integrals near singular points. He uses the example of the integral of 1/√(x^2+x) from 0 to 1, which has a singularity at x=0. By factoring and applying the binomial theorem, he simplifies the integrand to a form where the leading order term is a P-integral, allowing him to determine the convergence of the integral. The professor also discusses the behavior of the integral as x approaches infinity and the importance of considering the leading order term in such cases.

15:07

📉 The Challenge of Double Limits in Improper Integrals

The final paragraph addresses the complexity of double limits in improper integrals, particularly when integrating over the entire real number line. The professor cautions against the common mistake of incorrectly evaluating such integrals by taking a single limit. Using the integral of 1/(1+x^2) over all real numbers as an example, he demonstrates the correct approach of taking two independent limits and explains why the integral diverges. The lecture concludes with a reminder of the importance of practicing with improper integrals and a preview of the next lesson, which will focus on trigonometric integrals.

Mindmap

Keywords

💡Improper Integrals

Improper integrals are integrals that do not have a finite limit of integration or have an integrand that is not continuous over the interval of integration. In the video, the theme revolves around understanding and evaluating such integrals, which are a challenge due to their nature of extending to infinity or having discontinuities. For example, the script discusses the integral of 1 over x squared from negative 1 to 1, which is improper due to the discontinuity at x equals 0.

💡Fundamental Theorem of Integral Calculus

The Fundamental Theorem of Integral Calculus is a key concept in calculus that relates the derivative and the integral. It allows for the computation of definite integrals by finding antiderivatives. In the script, it is mentioned that while this theorem is powerful, it has limitations, particularly when dealing with improper integrals where the conditions of continuity and finite limits are not met.

💡Continuity

Continuity in calculus refers to a function being unbroken and without any gaps or jumps. The script emphasizes that the fundamental theorem of calculus assumes the integrand is continuous on the interval from A to B. An example of a discontinuous integrand is given as 1 over x squared, which is undefined at x equals 0, leading to issues when applying the fundamental theorem.

💡Riemann Sum

A Riemann Sum is a method for approximating the definite integral of a function by summing the areas of rectangles. It is foundational in understanding the definition of the definite integral. In the script, the Riemann Sum is contrasted with the result obtained by applying the fundamental theorem to a discontinuous integrand, highlighting the inconsistency that arises.

💡Antiderivative

An antiderivative is a function that represents the reverse process of differentiation. It is used to evaluate definite integrals. The script discusses finding antiderivatives for improper integrals, such as the log of (1 + x squared), and the complications that arise when evaluating these at infinite limits.

💡Blow-Up

In the context of improper integrals, a 'blow-up' refers to a singularity where the function becomes infinite at one of the endpoints. The script uses this term to describe a situation where the integrand is not well defined at a point, such as at x equals 0, and explains how to handle such cases by taking a limit as the variable approaches the singularity.

💡Tail Singularity

A tail singularity occurs when the interval of integration extends to infinity. The script discusses this in the context of improper integrals, explaining how to evaluate them by integrating over a finite domain up to a constant T and then taking the limit as T approaches infinity.

💡P-Integrals

P-Integrals refer to the integrals of the form 1 over x to the power of p. They are crucial in understanding the convergence or divergence of improper integrals. The script provides a detailed analysis of P-integrals, explaining how their convergence or divergence depends on the value of p, and how they can be used to determine the behavior of other integrals.

💡Convergence

Convergence in the context of improper integrals means that the integral has a finite value. The script explains the conditions under which P-integrals converge or diverge, such as when the value of p is greater than 1 leading to convergence, and when p is less than or equal to 1 leading to divergence.

💡Divergence

Divergence in improper integrals indicates that the integral does not have a finite value. The script discusses divergence in relation to P-integrals and other integrals, such as the integral of 1 over the square root of x squared plus x, which diverges because one part of the integral has a leading term that leads to a P-integral with p equals 1, which is always divergent.

💡Taylor Series

A Taylor Series is a representation of a function as an infinite sum of terms calculated from the values of the function's derivatives at a single point. The script mentions the Taylor Series to explain the leading order behavior of an integrand near a singularity, which is useful for determining the convergence of an improper integral.

💡Binomial Series

The Binomial Series is a specific type of Taylor Series used for expanding expressions of the form (1 + x)^n. In the script, the Binomial Series is used to simplify and understand the behavior of the integrand as x approaches zero, helping to identify the leading order term in the integral and its implications for convergence.

💡Double Limits

Double limits refer to the situation where both the lower and upper limits of integration approach infinity or negative infinity. The script warns against the common mistake of taking a single limit for double limits, using the example of integrating over the entire real number line, and explains the correct approach of taking two independent limits.

Highlights

Introduction to improper integrals and their limitations in relation to the fundamental theorem of integral calculus.

The importance of continuity in the integrand for the fundamental theorem to hold.

Example of an improper integral with a discontinuous integrand: 1/x^2 from -1 to 1.

The issue of non-convergence in the Riemann sum for discontinuous integrands.

The concept of finite intervals for the fundamental theorem and the problem of infinite limits.

Example of an improper integral with infinite limits: 2x/(1+x^2) from -∞ to ∞.

The technique of taking limits to evaluate improper integrals.

Two cases of improper integrals: 'blow up' at an endpoint and 'tail' extending to infinity.

The significance of 'p-integrals' in understanding convergence and divergence of improper integrals.

Convergence criteria for 'p-integrals' with a tail singularity.

Divergence of 'p-integrals' when p is less than or equal to 1, regardless of the direction of integration.

Convergence criteria for 'p-integrals' with a blow-up singularity at 0.

The use of Taylor series to determine the leading order behavior of an integrand near a singularity.

Example of applying the binomial series to determine convergence of an improper integral.

The importance of recognizing when an improper integral diverges due to one part of the integral.

The problem of double limits in improper integrals over the entire real number line.

The incorrect assumption of convergence in double limits and the need for careful evaluation.

The general behavior of improper integrals and the importance of practice in understanding them.

Upcoming lesson on broader techniques for evaluating improper integrals and their application to trigonometric integrals.

Transcripts

Browse More Related Video

Unit VI: Lec 4 | MIT Calculus Revisited: Single Variable Calculus

Calculus BC – 6.13 Evaluating Improper Integrals

Improper Integrals - Convergent or Divergent (Made Easy)

Improper Integrals

Improper Integrals - Discontinuity at a Boundary

Improper Integrals - Convergence and Divergence - Calculus 2