9.3 Additional Gas Laws | Dalton's Law and Graham's Law | High School Chemistry
TLDRThis chemistry lesson covers essential gas laws including Dalton's Law of Partial Pressures, Graham's Law of Effusion, and the concept of gas density. It explains how the total pressure in a container is the sum of the partial pressures of individual gases, the volume of one mole of gas at standard temperature and pressure (STP), and how gas density can be calculated. The lesson also humorously illustrates Graham's Law with a practical example involving cologne and flatulence.
Takeaways
- 📚 The lesson covers additional gas laws including Dalton's Law of Partial Pressures, Graham's Law of Effusion, and the concept of gas density at Standard Temperature and Pressure (STP).
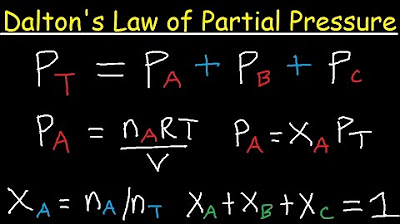
- 🌌 Dalton's Law of Partial Pressures states that the total pressure of a gas mixture is equal to the sum of the partial pressures of the individual gases, which are dependent on the number of moles of each gas.
- 📊 Partial pressure can be calculated by multiplying the mole fraction of a gas by the total pressure. For example, nitrogen with half the moles in a container contributes to half the total pressure.
- 🔍 At STP, which is 273 Kelvin and 1 atmosphere, one mole of any ideal gas occupies a volume of 22.4 liters, regardless of the gas's identity.
- 🧪 An example demonstrates the application of stoichiometry and the volume of gas produced at STP, using the reaction of magnesium with hydrochloric acid to produce hydrogen gas.
- 🔢 The density of a gas can be calculated by dividing its mass by volume, or more conveniently using the derived formula: density = (pressure × molar mass) / (RT), where R is the gas constant and T is the temperature.
- 🚀 Graham's Law of Effusion describes the relative rates at which gases effuse through a small opening, with lighter gases moving faster due to their higher average velocity at the same kinetic energy.
- 🌀 The rate of effusion of a gas is inversely proportional to the square root of its molar mass, which can be used to compare the effusion rates of different gases.
- 🎈 A humorous anecdote illustrates the concept of effusion and diffusion, suggesting that lighter gases, like methane in flatulence, will reach a person's nose faster than heavier molecules in cheap perfume.
- 📈 The lesson aims to provide a comprehensive understanding of gas laws, with practical examples and calculations to aid in the learning process for high school chemistry.
- 🔗 The instructor promotes a premium course on chatsprep.com for further study materials and practice problems related to high school chemistry.
Q & A
What is Dalton's Law of Partial Pressures?
-Dalton's Law of Partial Pressures states that the total pressure of a gaseous mixture is equal to the sum of the partial pressures of the individual gases in the mixture. The partial pressure of each gas is proportional to its mole fraction in the mixture.
How is partial pressure related to the mole fraction of a gas?
-Partial pressure is the pressure that a specific gas in a mixture would exert if it were to occupy the entire volume by itself. It is calculated as the mole fraction of the gas multiplied by the total pressure of the mixture.
What is the volume of one mole of an ideal gas at Standard Temperature and Pressure (STP)?
-At STP, which is 0°C (273.15 K) and 1 atmosphere of pressure, the volume of one mole of any ideal gas is approximately 22.4 liters.
What is the significance of the number 22.4 liters in the context of STP?
-The volume of 22.4 liters is the molar volume of an ideal gas at STP. This value is often memorized because it is a convenient reference for calculating the volume of gases under standard conditions.
How can you calculate the density of a gas?
-The density of a gas can be calculated by dividing the mass of the gas by its volume. Alternatively, a derived formula from the ideal gas law, which is the density equals the pressure times the molar mass divided by the product of the gas constant and temperature, can be used.
What is Graham's Law of Effusion and how does it relate to the molar mass of gases?
-Graham's Law of Effusion states that the rate of effusion of a gas is inversely proportional to the square root of its molar mass. This means that lighter gases will effuse faster than heavier ones at the same temperature and pressure.
What is the practical application of Graham's Law of Effusion in everyday life?
-Graham's Law of Effusion can be observed in everyday phenomena such as the diffusion of scents. Lighter molecules, such as those in perfumes or gases, will diffuse more quickly and reach areas faster than heavier molecules.
How does the molar mass affect the rate of effusion of a gas?
-According to Graham's Law, the rate of effusion is inversely proportional to the square root of the molar mass of the gas. Therefore, gases with lower molar masses will effuse at a faster rate compared to those with higher molar masses.
What is the ideal gas law and how is it used in the context of the video script?
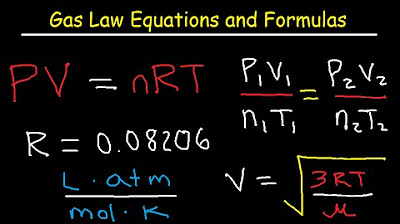
-The ideal gas law is given by the equation PV = nRT, where P is the pressure, V is the volume, n is the number of moles, R is the gas constant, and T is the temperature in Kelvin. In the script, it is used to derive expressions for partial pressures, molar volume at STP, and the density of a gas.
Can you provide an example of how to use the ideal gas law to calculate the volume of hydrogen gas produced in a chemical reaction?
-In the script, the example given is the reaction of magnesium with hydrochloric acid to produce hydrogen gas. By knowing the mass of magnesium and its molar mass, you can calculate the moles of magnesium and, by stoichiometry, the moles of hydrogen produced. Using the ideal gas law or the molar volume at STP (22.4 L/mol), you can then calculate the volume of hydrogen gas at STP.
Outlines
🔬 Dalton's Law of Partial Pressures
This paragraph introduces Dalton's Law of Partial Pressures, which states that the total pressure of a gas mixture is equal to the sum of the partial pressures of its individual gases. The concept of partial pressure is explained as the pressure exerted by a single gas in a mixture, which depends on the number of moles of that gas. The example provided involves a rigid container with 10 moles of N2, 8 moles of O2, and 2 moles of CO2, totaling a pressure of 50 atmospheres. The calculation of partial pressures for each gas is demonstrated, showing how nitrogen, being the most abundant, contributes the most to the total pressure.
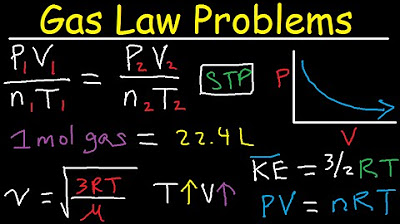
🌡️ Standard Temperature and Pressure (STP) and Gas Volume
This paragraph discusses the concept of Standard Temperature and Pressure (STP), defined as a temperature of 273 Kelvin (0°C) and a pressure of one atmosphere. Under these conditions, the volume of one mole of any ideal gas is consistently 22.4 liters, regardless of the gas's identity. The script uses the ideal gas law (PV = nRT) to derive this volume, emphasizing the importance of memorizing this value for ease in calculations. An example involving the reaction of magnesium with hydrochloric acid to produce hydrogen gas is given, demonstrating how to calculate the volume of hydrogen gas produced at STP based on the moles of magnesium reacted.
💭 Gas Density Calculation
This paragraph explores the calculation of gas density, defined as mass per unit volume. The script derives a formula for gas density from the ideal gas law, showing that density can be expressed as the product of pressure, molar mass, and the inverse of the gas constant and temperature. An example is provided where the density of helium gas at 2 atmospheres and 273 Kelvin is calculated. The process involves determining the volume of one mole of helium using the ideal gas law and then calculating its density based on its mass and volume.
🏃♂️ Graham's Law of Effusion
This paragraph introduces Graham's Law of Effusion, which describes the relative rates at which gases effuse (escape through a small opening). The law states that the rate of effusion of a gas is inversely proportional to the square root of its molar mass. The script uses the example of hydrogen and oxygen gases to illustrate how hydrogen, being lighter, will effuse faster. The calculation involves comparing the square roots of the molar masses of the two gases to determine the relative rates of effusion. The concept is also humorously applied to a scenario involving the diffusion of perfume and methane gas (from flatulence), showing that lighter gases will diffuse faster.
📚 High School Chemistry Playlist and Resources
In the final paragraph, the script promotes the high school chemistry playlist available on the channel, which will be updated weekly throughout the 2020-21 school year. The speaker encourages viewers to subscribe and enable notifications to stay updated on new lessons. Additionally, resources such as study guides and practice problems for high school chemistry are mentioned, directing interested viewers to a premium course on chatsprep.com for further learning materials.
Mindmap
Keywords
💡Dalton's Law of Partial Pressures
💡Mole Fraction
💡Standard Temperature and Pressure (STP)
💡Ideal Gas Law
💡Graham's Law of Effusion
💡Effusion
💡Density
💡Stoichiometry
💡Molar Mass
💡Chemical Reaction
Highlights
Introduction to additional gas laws beyond the ideal and combined gas laws.
Explanation of Dalton's Law of Partial Pressures and its application in calculating partial pressures in a mixture.
Total pressure is the sum of partial pressures of individual gases in a mixture.
Partial pressure equals the mole fraction of a gas times the total pressure.
Example calculation of partial pressures for nitrogen, oxygen, and carbon dioxide in a container.
Discussion on the volume of one mole of gas at Standard Temperature and Pressure (STP).
STP conditions defined as 273 Kelvin and one atmosphere, resulting in a volume of 22.4 liters per mole of gas.
Derivation of the volume of one mole of gas at STP using the ideal gas law.
Application of STP volume in calculating the volume of hydrogen gas produced from a chemical reaction.
Conversion of moles of gas to volume at STP using the memorized volume of 22.4 liters per mole.
Introduction to gas density and its calculation.
Derivation of an expression for gas density using the ideal gas law.
Example calculation of the density of helium gas at 2 atmospheres and 273 Kelvin.
Introduction to Graham's Law of Effusion and its relation to the movement of gases.
Explanation of effusion as the movement of gas through a narrow slit or hole.
Graham's Law of Effusion states that the rate of effusion is inversely proportional to the square root of the molar mass.
Example calculation showing hydrogen gas escaping four times faster than oxygen gas due to its lower molar mass.
Application of Graham's Law of Effusion to diffusion, explaining why lighter gases diffuse faster.
Humorous example applying effusion principles to the diffusion of cologne and methane gas.
Transcripts
Browse More Related Video

Introduction to partial pressure | Gases and kinetic molecular theory | Chemistry | Khan Academy
Gas Laws - Equations and Formulas
Dalton's Law of Partial Pressure Problems & Examples - Chemistry
Gas Law Problems Combined & Ideal - Density, Molar Mass, Mole Fraction, Partial Pressure, Effusion

Daltons Law | Partial Pressures

Partial pressure example | Chemistry | Khan Academy
5.0 / 5 (0 votes)
Thanks for rating: