Gas Law Problems Combined & Ideal - Density, Molar Mass, Mole Fraction, Partial Pressure, Effusion
TLDRThe video script delves into the principles of gas laws, offering a comprehensive guide on solving problems related to the ideal gas law, combined gas law, and other fundamental concepts in chemistry. It begins with the definition of pressure and its relation to force and area, then transitions into the importance of unit conversion for pressure. The script meticulously explains the ideal gas law equation, PV=nRT, and its components, emphasizing the significance of using consistent units. It further breaks down into individual gas laws, such as Boyle's law highlighting the inverse relationship between pressure and volume, Charles's law showing the direct correlation between temperature and volume, and Avogadro's law connecting volume and the number of moles. The script also explores Dalton's Law of Partial Pressure, providing insights into mole fractions and total pressure calculations. It concludes with applications of these laws, including the calculation of gas density, root mean square velocity, and the behavior of gases in different conditions, adhering to the kinetic molecular theory for ideal gases.
Takeaways
- 📐 **Understanding Pressure**: Pressure is force per unit area, measured in Newtons per square meter (N/m²) or Pascals (Pa), with 1 atm equal to 101.3 kPa or 760 mmHg.
- 💥 **Gas Particle Collisions**: The pressure exerted by a gas is due to the force from gas molecule collisions with the container walls, with more molecules leading to more collisions and higher pressure.
- 🔄 **Unit Conversion for Pressure**: Conversion between different pressure units (e.g., atm, mmHg, kPa) is essential, using specific conversion factors like 760 mmHg to 1 atm.
- 📉 **Boyle's Law**: Describes the inverse relationship between pressure and volume at constant temperature, with the formula P1V1 = P2V2.
- 📈 **Charles's Law**: Relates temperature and volume when pressure and the amount of gas are constant, using the equation V1/T1 = V2/T2, showing a direct relationship.
- 🌡️ **Gay-Lussac's Law**: Illustrates the direct relationship between pressure and temperature for a given volume and amount of gas, with the formula P1/T1 = P2/T2.
- ⚖️ **Avogadro's Law**: States that the volume of a gas is proportional to the number of moles at constant temperature and pressure, given by V1/N1 = V2/N2.
- 🔗 **Combined Gas Law**: A composite of Boyle's, Charles's, and Gay-Lussac's laws, it accounts for changes in pressure, volume, and temperature with the formula (P1V1/T1) = (P2V2/T2).
- 🔎 **Ideal Gas Law**: The fundamental equation PV = nRT is used to calculate various properties of an ideal gas, where P is pressure, V is volume, n is the number of moles, R is the gas constant, and T is temperature in Kelvin.
- 🧪 **Graham's Law of Diffusion**: Describes the rate of effusion of gases, which is proportional to the square root of the molar mass inversely (lighter gases diffuse faster).
- 🤝 **Dalton's Law of Partial Pressures**: The total pressure in a mixture of non-reacting gases is the sum of the partial pressures of the individual gases.
Q & A
What is the ideal gas law equation mentioned in the video?
-The ideal gas law equation mentioned in the video is PV = nRT, where P stands for pressure, V is volume, n is moles, R is the gas constant, and T is temperature.
How can you convert from Torr to atmospheres (atm) according to the explanation in the video?
-To convert from Torr to atmospheres, you use the conversion factor where 760 Torr is equal to 1 atm. For example, to convert 500 Torr to atm, you calculate 500 / 760 which equals approximately 0.658 atm.
What is Boyle's law and how is it described in the video?
-Boyle's law shows the relationship between pressure and volume, keeping moles and temperature constant. It states that pressure and volume are inversely related; as volume increases, pressure decreases. The equation for Boyle's law is P1V1 = P2V2.
What does the video describe as the primary reason for pressure decrease when the volume of a gas increases?
-The primary reason for the decrease in pressure when the volume increases, as described in the video, is due to fewer collisions of gas molecules with the walls of the container. This reduction in collisions results in less force being exerted on the walls, thereby lowering the pressure.
According to the video, how does temperature affect the kinetic energy of gas molecules?
-The video explains that as the temperature increases, the kinetic energy of gas molecules also increases. This is because the molecules move faster at higher temperatures, leading to more frequent collisions with the container walls, thus increasing the pressure.
Can you explain Charles' Law as mentioned in the video?
-Charles' Law describes the direct relationship between the volume and temperature of a gas, provided pressure and moles remain constant. As temperature increases, volume also increases. The law is mathematically expressed as V1/T1 = V2/T2.
What conversion factor does the video suggest for converting kilopascals to atmospheres?
-The video suggests using the conversion factor where 101.3 kilopascals is equivalent to 1 atmosphere. This factor is used to convert measurements of pressure between these two units.
Why is the ideal gas law particularly useful in chemistry, as outlined in the video?
-The ideal gas law is particularly useful in chemistry because it provides a simple way to relate the pressure, volume, temperature, and number of moles of a gas. This law allows chemists to predict the behavior of gases under different conditions, facilitating calculations and experiments involving gases.
What does the video say about the units for the gas constant (R) and its implication on pressure units?
-The video explains that the choice of the gas constant R affects the units used for pressure in the ideal gas law. For instance, if R = 0.08206 L atm/mol K, pressure must be in atmospheres, while if R = 8.3145 J/mol K, pressure should be in pascals or kilopascals. Matching the units of R with those of pressure is crucial for accurate calculations.
How is Avogadro's Law presented in the video, and what does it state about the relationship between moles and volume?
-Avogadro's Law, as presented in the video, states that the volume of a gas is directly proportional to the number of moles of gas when the pressure and temperature are held constant. The law is mathematically expressed as V1/N1 = V2/N2, indicating that if the number of moles increases, the volume increases proportionally.
Outlines
📚 Introduction to Gas Laws
This paragraph introduces the topic of gas laws, emphasizing the importance of understanding how to solve problems related to the ideal gas law and combined gas law. It mentions various laws such as Boyle's, Charles's, Avogadro's, and Graham's law of diffusion, and highlights concepts like gas density, molar mass, partial pressure, and mole fraction. The explanation begins with the definition of pressure and its units, such as Pascal and ATM, and explains the relationship between the number of gas molecule collisions and the pressure exerted.
🔄 Isothermal and Combined Gas Laws
The second paragraph delves into the ideal gas law equation (PV=nRT), discussing how pressure, volume, moles of gas, and temperature are interrelated. It explains the isothermal condition where temperature is constant and how it leads to Boyle's Law (P1V1=P2V2), indicating the inverse relationship between pressure and volume. The paragraph also covers the combined gas law, which allows for the calculation of gas properties under varying conditions of pressure, volume, and temperature, and introduces Charles's Law (V1/T1=V2/T2) and Avogadro's Law (V1/N1=V2/N2) as specific cases of the combined gas law.
🔆 Gay-Lussac's Law and Temperature's Effect on Gas Pressure
This section focuses on Gay-Lussac's Law, which describes the direct relationship between the pressure of a gas and its temperature, assuming constant volume and moles of gas. It explains that as temperature increases, the kinetic energy of gas molecules also increases, leading to more frequent collisions and thus higher pressure. The paragraph uses the example of heating a pot of water to illustrate the point and emphasizes the linear relationship between temperature and pressure.
🌡️ Charles's Law and the Effect of Temperature on Gas Volume
The fourth paragraph discusses Charles's Law, which shows the direct relationship between the volume of a gas and its temperature when pressure and the number of moles are held constant. It explains that increasing the temperature of a gas in an expandable container will lead to an increase in volume. The molecular explanation involves gas particles moving faster at higher temperatures, causing the gas to expand against the external pressure until equilibrium is reached.
📈 Avogadro's Law and the Relationship Between Volume and Moles
This paragraph explores Avogadro's Law, which states that the volume of a gas is directly proportional to the number of moles of gas, provided that pressure and temperature are constant. It uses the example of blowing air into a balloon to illustrate how increasing the moles of gas increases the volume. The relationship is linear, and the concept is fundamental for understanding how gas particles occupy space.
🔩 Dalton's Law of Partial Pressure and Mole Fractions
The sixth paragraph introduces Dalton's Law of Partial Pressure, which states that the total pressure of a gas mixture is equal to the sum of the partial pressures of the individual gases in the mixture. It explains the concept of mole fractions and how they are used to calculate the partial pressure of each gas in the mixture. The mole fraction is the ratio of the moles of a particular gas to the total moles of all gases present.
🔍 Ideal Gas Law and Calculating Gas Properties
The seventh paragraph focuses on the ideal gas law equation (PV=nRT) and its application in calculating various properties of a gas, such as pressure, volume, and the number of moles, given the other variables. It provides a step-by-step approach to solving for pressure when other parameters like volume, moles, and temperature are known, and also discusses how to calculate the mass of a gas using molar mass.
🚀 Kinetic Molecular Theory and Gas Behavior
The final paragraph outlines the Kinetic Molecular Theory, which describes the behavior of ideal gases. It highlights that gas molecules are assumed to have negligible volume, move in continuous random motion, and experience perfectly elastic collisions. The theory also assumes that intermolecular forces are negligible. The paragraph differentiates between polar and non-polar gases, noting that non-polar gases like helium behave more ideally due to weaker intermolecular forces. It concludes with the conditions under which a real gas will behave more like an ideal gas, which is at high temperatures and low pressures.
Mindmap
Keywords
💡Ideal Gas Law
💡Boyle's Law
💡Charles's Law
💡Gay-Lussac's Law
💡Avogadro's Law
💡Dalton's Law of Partial Pressure
💡Mole Fraction
💡Root Mean Square Velocity
💡Kinetic Molecular Theory
💡Effusion and Diffusion
💡Vapor Pressure
Highlights
The video focuses on gas laws, specifically ideal gas law and combined gas law problems.
Gas density, molar mass, partial pressure, and mole fraction are discussed in relation to gas laws.
Boyle's Law, Charles's Law, Gay-Lussac's Law, Avogadro's Law, and Graham's Law are covered.
Pressure is defined as force per unit area, with common units being Newtons, Pascals, and atmospheres (ATM).
The relationship between the number of gas molecule collisions and pressure is explained.
Conversion between different units of pressure, such as Torr and ATM, is demonstrated.
The ideal gas law equation PV = nRT is introduced, with considerations for unit consistency.
Liters to milliliters conversion is explained for volume measurements.
Boyle's Law is described, illustrating the inverse relationship between pressure and volume at constant temperature.
Gay-Lussac's Law shows the direct relationship between pressure and temperature at constant volume.
Charles's Law is derived, indicating the direct relationship between volume and temperature at constant pressure.
Avogadro's Law is discussed, showing volume is directly proportional to the number of moles at constant pressure and temperature.
The concept of Dalton's Law of Partial Pressure is introduced for mixtures of gases.
Mole fraction is defined and its calculation is demonstrated in the context of gas mixtures.
The identity of an unknown gas can be determined by calculating its molar mass from density and pressure measurements.
Root mean square velocity of gas particles is derived and calculated for a given temperature.
The kinetic molecular theory (KMT) for ideal gases is summarized, including assumptions and behaviors.
Conditions under which a real gas behaves more like an ideal gas are discussed, emphasizing high temperature and low pressure.
Transcripts
Browse More Related Video
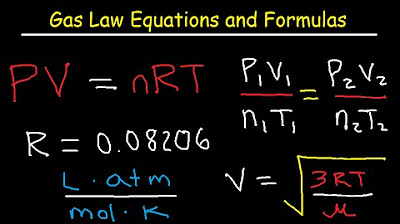
Gas Laws - Equations and Formulas
9.2 Gas Laws including the Ideal Gas Law | High School Chemistry
Ideal Gas Problems: Crash Course Chemistry #13
Kinetic Molecular Theory of Gases - Practice Problems

9.3 Additional Gas Laws | Dalton's Law and Graham's Law | High School Chemistry

Introduction to partial pressure | Gases and kinetic molecular theory | Chemistry | Khan Academy
5.0 / 5 (0 votes)
Thanks for rating: