Ratio Test for Convergence | MIT 18.01SC Single Variable Calculus, Fall 2010
TLDRThis educational video delves into the Ratio Test, a method for assessing the convergence of series, particularly useful for understanding Taylor series. The script explains the test's application, stating that if the limit of the ratio of consecutive terms is less than 1, the series converges; if greater than 1, it diverges; and if equal to 1, the test is inconclusive. The presenter provides examples to illustrate the test's utility, including series where conclusions can and cannot be drawn, and hints at the test's importance in determining the radius of convergence for Taylor series in a forthcoming video.
Takeaways
- 📚 The video introduces the Ratio Test, a method for determining the convergence of series.
- 🔍 The Ratio Test involves calculating the limit of the ratio of consecutive terms of a series as n approaches infinity.
- 📉 If the limit L is less than 1, the series converges.
- 📈 If the limit L is greater than 1, the series diverges.
- ⏳ When the limit L equals 1, the test is inconclusive, and the series may either converge or diverge.
- 🔢 The test is applicable to series with positive terms and can also be used for series with non-positive terms by considering their absolute values.
- 📝 The script provides a detailed explanation of why a series converges when L < 1, using the comparison test with a geometric series.
- 🔑 The video uses examples to illustrate the test, including series where the limit L equals 1, showing that the test cannot determine convergence or divergence in such cases.
- 📉 An example of a series with L > 1 is given, demonstrating the divergence of the series.
- 📈 An example of a series with L < 1 is provided, showing that the series converges.
- 🔄 The script mentions that the Ratio Test can be useful in situations where other tests like the Integral Test are more complex to apply.
- 🔮 The video promises to show how the Ratio Test can be used to determine the radius of convergence for Taylor series in a future video.
Q & A
What is the ratio test for series convergence?
-The ratio test is a method used to determine the convergence of an infinite series. It involves taking the limit of the ratio of the (n+1)th term to the nth term of the series as n approaches infinity. If the limit L is less than 1, the series converges; if L is greater than 1, it diverges; and if L equals 1, the test is inconclusive.
What is the significance of the term 'a sub n' in the context of the ratio test?
-In the ratio test, 'a sub n' represents the nth term of the series being evaluated for convergence. The test involves examining the behavior of this term as n approaches infinity.
Why can we assume all terms of the series to be positive when applying the ratio test?
-Assuming all terms to be positive simplifies the application of the ratio test. If the terms are not positive, taking the absolute value of the terms still allows for conclusions about absolute convergence without affecting the test's outcome.
What happens if the limit in the ratio test does not exist?
-If the limit does not exist, the ratio test cannot be used to determine the convergence of the series. The test requires a definite limit to make a conclusion about the series.
How does the ratio test relate to Taylor series, as mentioned in the script?
-The ratio test can be used to determine the radius of convergence for Taylor series. This application will be discussed in a subsequent video, as the test provides a method to evaluate the behavior of series terms in relation to infinity.
What is the conclusion if the limit L in the ratio test is less than 1?
-If the limit L is less than 1, the series is guaranteed to converge. This is because the terms of the series are getting smaller at a rate that ensures the sum of the series will not diverge.
What is the conclusion if the limit L in the ratio test is greater than 1?
-If the limit L is greater than 1, the series is guaranteed to diverge. The terms of the series are increasing at a rate that will cause the sum of the series to become unbounded.
Why is the ratio test inconclusive when the limit L equals 1?
-When L equals 1, the ratio test does not provide enough information to determine whether the series converges or diverges. The behavior of the terms as n approaches infinity does not clearly indicate convergence or divergence in this case.
Can you provide an example from the script where the ratio test was inconclusive?
-Yes, the script provides the example of the series 1/n^2, where the limit L equals 1. The ratio test was inconclusive for this series, but we know from other tests that it actually converges.
How does the script explain the logic behind the ratio test when L is less than 1?
-The script explains that when L is less than 1, you can choose a number r between L and 1. For large n, the ratio of the (n+1)th term to the nth term is less than r. This implies that the terms of the series are decreasing and can be bounded by a geometric series with a ratio less than 1, which is known to converge.
What is the geometric series, and how does it relate to the ratio test?
-A geometric series is an infinite series where each term is a constant multiple of the previous term. In the context of the ratio test, if the ratio of terms in the series being tested forms a geometric series with a ratio less than 1, the original series is guaranteed to converge.
Can you provide an example from the script where the ratio test was conclusive and indicated divergence?
-Yes, the script provides the example of the series 4^n / (n * 3^n). The limit L for this series was calculated to be 4/3, which is greater than 1, indicating that the series diverges.
What is the importance of the ratio test in evaluating the convergence of series?
-The ratio test is a powerful tool in mathematical analysis for determining the convergence of series. It provides a quick and often conclusive method for assessing the behavior of series terms as they approach infinity, which is crucial in understanding the sum of infinite series.
How does the script suggest the ratio test can be used for series where the terms are not positive?
-The script suggests that even if the terms of the series are not positive, you can still apply the ratio test by taking the absolute value of all the terms. This allows you to make conclusions about absolute convergence.
Outlines
📚 Introduction to the Ratio Test
This paragraph introduces the concept of the ratio test, a method for determining the convergence of a series. The test involves examining the limit of the ratio of consecutive terms as n approaches infinity. If the limit, denoted as L, is less than 1, the series converges; if L is greater than 1, it diverges; and if L equals 1, the test is inconclusive. The paragraph also emphasizes the importance of dealing only with positive terms for simplicity, though the test can be applied to series with non-positive terms by considering their absolute values. The explanation is set to prepare the viewer for a deeper understanding of Taylor series in subsequent videos.
🔍 Applying the Ratio Test with Examples
The second paragraph delves into applying the ratio test with various examples. It explains the logic behind the test when L is less than 1, illustrating how the terms of the series become smaller and can be bounded by a geometric series, which is known to converge. The paragraph also discusses the implications when L is greater than 1, hinting at the divergence of the series by choosing an appropriate value of r. It provides examples, including the harmonic series (1/n), which is known to diverge, and the series 1/n^2, which converges, to demonstrate the test's inconclusiveness when L equals 1. The explanation aims to clarify the test's application and its limitations.
📉 Further Examples and the Ratio Test's Limitations
This paragraph continues the discussion with more examples to illustrate the ratio test's utility and its limitations. It examines series such as 4^n/(n*3^n) and n^10/10^n, calculating their respective limits to determine convergence or divergence. The examples show that the test can provide clear conclusions in some cases, such as the divergence of the first series and the convergence of the second. The paragraph also touches on the integral test as an alternative method for series where the ratio test is inconclusive. Lastly, it sets the stage for the next video, which will explore the ratio test's application in determining the radius of convergence for Taylor series.
Mindmap
Keywords
💡Recitation
💡Convergence Test
💡Series
💡Ratio Test
💡Limit
💡Absolute Convergence
💡Geometric Series
💡Diverges
💡Comparison Test
💡Taylor Series
💡Radius of Convergence
Highlights
Introduction to the Ratio Test for series convergence, a lesser-known but helpful test.
Explanation of the Ratio Test formula: the limit of a_(n+1) / a_n as n approaches infinity.
Clarification that the series terms can be assumed positive for simplicity, with absolute values for non-positive terms.
Three possible conclusions from the Ratio Test based on the value of L (limit): convergence if L < 1, divergence if L > 1, and inconclusive if L = 1.
Demonstration of why a series converges when L < 1 using a comparison with a geometric series.
The importance of choosing a value r between L and 1 for the test's logic and comparison tests.
Example of applying the Ratio Test to the harmonic series (1/n), which is known to diverge, and the test result of L = 1.
Contrasting example with the series 1/n^2, which converges, also yielding L = 1 and showing the test's inconclusive nature in such cases.
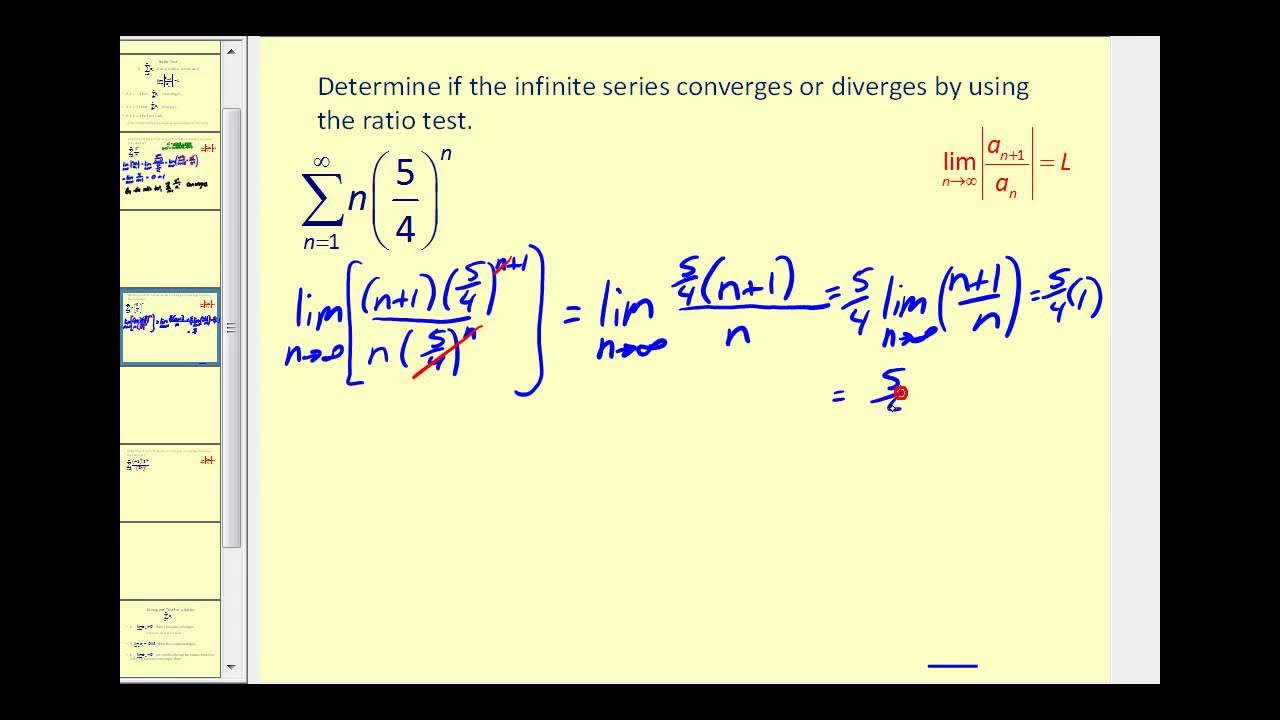
Application of the Ratio Test to a series with terms 4^n / (n * 3^n), resulting in divergence due to L > 1.
Exploration of a series with terms n^10 / (10^n), where the Ratio Test shows convergence with L < 1.
Discussion on the limitations of the Ratio Test when L = 1, and the need for additional tests like the Integral Test.
The Ratio Test's utility in quickly determining the convergence of certain series, especially when compared to the Integral Test.
Preview of the next video's content, which will cover the application of the Ratio Test to determine the radius of convergence for Taylor series.
Emphasis on the Ratio Test's role in understanding the behavior of series at infinity and its practical applications.
The test's significance in mathematical analysis, particularly in the study of series and their convergence properties.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: