❖ The Mean Value Theorem ❖
TLDRThis video script delves into the Mean Value Theorem, explaining its conditions and implications through a visual demonstration. The presenter illustrates the theorem by discussing a function that is continuous on a closed interval and differentiable on an open interval, showing that there exists at least one point where the derivative equals the slope of the line connecting the endpoints. A practical example using the function f(x) = 1 + cube root of (x - 1) is worked through, demonstrating the theorem's application and the process of verifying the function's continuity and differentiability over a given interval.
Takeaways
- 📚 The Mean Value Theorem (MVT) states that if a function is continuous on a closed interval and differentiable on an open interval, there exists at least one point where the derivative equals the slope of the line connecting the endpoints.
- 📈 The conditions for applying MVT are: the function must be continuous on [a, b] and differentiable on (a, b).
- 📉 Continuity implies the function can be drawn without lifting the pen, while differentiability requires the graph to be smooth without sharp points, cusps, or kinks.
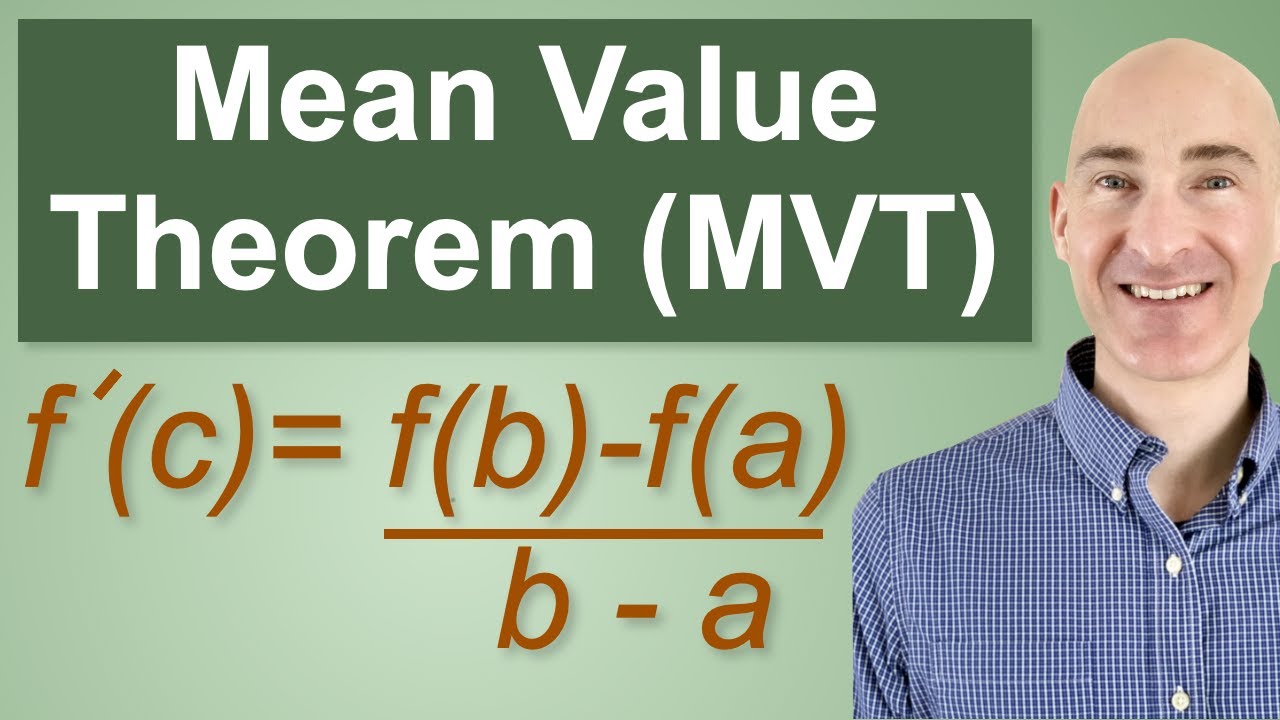
- 📝 The MVT is illustrated by finding a point 'c' in the interval (a, b) where f'(c) = (f(b) - f(a)) / (b - a), which represents the slope of the secant line.
- 🔍 An example function, f(x) = 1 + (x - 1)^(1/3), is used to demonstrate the application of MVT on the interval [2, 9].
- 🌟 The function's continuity is assured as root functions are continuous on their domain, which is all real numbers for the cube root function.
- 🔧 Differentiability is confirmed by finding the domain of the derivative, f'(x) = 2/3 / ((x - 1)^(2/3)), which is all real numbers except x = 1.
- 📌 The derivative at any point 'c' in the interval (2, 9) should equal the slope of the line connecting (2, f(2)) and (9, f(9)).
- 🧩 The calculation involves finding f(9) and f(2), and then using these values to determine the required slope for the MVT to hold.
- 🔄 Solving for 'c' involves algebraic manipulation to isolate 'x' in the equation derived from setting the derivative equal to the slope.
- 📐 The final step is to verify that the solution for 'c' falls within the interval [2, 9], ensuring it meets the conditions of the MVT.
Q & A
What is the Mean Value Theorem?
-The Mean Value Theorem states that if a function f(x) is continuous on the closed interval [a, b] and differentiable on the open interval (a, b), then there exists at least one point c in the interval (a, b) such that the derivative at that point, f'(c), equals the average rate of change of the function over the interval, which is (f(b) - f(a)) / (b - a).
What are the conditions for a function to satisfy the Mean Value Theorem?
-A function must be continuous on the closed interval [a, b] and differentiable on the open interval (a, b) to satisfy the Mean Value Theorem.
What does it mean for a function to be differentiable?
-A function is differentiable at a point if it has a derivative at that point, implying the function is smooth and has no sharp points, cusps, or kinks at that location.
How is the slope of the line connecting the endpoints of a function's graph calculated?
-The slope of the line connecting the endpoints is calculated as the change in y-coordinates (f(b) - f(a)) divided by the change in x-coordinates (b - a).
What is the significance of the Mean Value Theorem in understanding a function's behavior?
-The Mean Value Theorem helps in understanding that there is at least one point in the interval where the instantaneous rate of change (the derivative) equals the average rate of change, providing insight into the function's behavior within that interval.
How does the video script demonstrate the Mean Value Theorem with an example?
-The script uses the function f(x) = 1 + (x - 1)^(1/3) on the interval [2, 9], showing that it is continuous and differentiable over this interval, and then calculates the derivative to find a point where it equals the slope of the line connecting the endpoints.
What is the domain of the function f(x) = 1 + (x - 1)^(1/3)?
-The domain of the function is all real numbers, as any real number can be placed under a cube root, and there are no restrictions that would make the function undefined.
Why is it necessary to find the domain of the derivative function?
-Finding the domain of the derivative function is necessary to determine where the original function is differentiable, which is a requirement for the Mean Value Theorem.
How does the script find the derivative of the given function?
-The script uses the chain rule to find the derivative of f(x) = 1 + (x - 1)^(1/3), resulting in f'(x) = 2/3 / ((x - 1)^(2/3)).
What is the significance of finding where the derivative is undefined?
-Identifying where the derivative is undefined helps in determining the points where the function is not differentiable, which is crucial for applying the Mean Value Theorem correctly.
How does the script solve for the point 'c' where the derivative equals the slope of the line connecting the endpoints?
-The script sets the calculated slope equal to the derivative, rearranges the equation algebraically, and solves for 'c' within the interval [2, 9], ensuring the solution is valid according to the Mean Value Theorem.
Outlines
📚 Introduction to the Mean Value Theorem
This paragraph introduces the Mean Value Theorem (MVT), a fundamental concept in calculus. The theorem states that if a function 'f(x)' is continuous on a closed interval [A, B] and differentiable on the open interval (A, B), then there exists at least one point 'c' in the interval (A, B) where the derivative of the function at 'c' equals the slope of the secant line connecting the endpoints (A, f(A)) and (B, f(B)). The explanation is accompanied by a visual representation, emphasizing the conditions of continuity and differentiability, which are necessary for the theorem to apply. The paragraph also hints at the intuitive idea behind the theorem, suggesting that the slope of the tangent at some point on the graph should match the slope of the line connecting the endpoints.
🔍 Analyzing Continuity and Differentiability for MVT Application
The second paragraph delves deeper into the conditions required for the Mean Value Theorem to be applicable. It discusses the necessity for a function to be continuous on its domain and differentiable everywhere except possibly at a finite number of points. The video script provides an example function, f(x) = 1 + (x - 1)^(1/3), and demonstrates that it is continuous on its entire domain, which is all real numbers. The differentiability of the function is then explored by finding its derivative, which is shown to be undefined only at x = 1. Since the interval of interest is from 2 to 9, the function is differentiable over this interval, satisfying the conditions for the MVT. The process of finding the derivative using the chain rule is explained, and the domain of the derivative function is determined to ensure the function's differentiability within the specified interval.
📉 Applying the Mean Value Theorem to a Specific Function
The final paragraph presents an application of the Mean Value Theorem to the function f(x) = 1 + (x - 1)^(1/3) over the interval [2, 9]. The script calculates the slope of the line connecting the endpoints of the interval, which is found by evaluating the function at x = 9 and x = 2, and then taking the difference divided by the interval length (9 - 2). The result is a slope of 1/7. According to the MVT, there must be at least one point 'c' within the interval where the derivative of the function equals this slope. The script then sets up an equation to solve for 'c', using the previously found derivative of the function. After some algebraic manipulation, the solution for 'c' is found to be 1 + (7/3)^(3/2), which falls within the interval [2, 9]. This confirms the existence of such a point 'c', thus demonstrating the Mean Value Theorem in action.
Mindmap
Keywords
💡Mean Value Theorem
💡Continuous
💡Differentiable
💡Closed Interval
💡Open Interval
💡Derivative
💡Slope
💡Endpoint
💡Cube Root
💡Domain
💡Chain Rule
Highlights
Introduction to the Mean Value Theorem and its conditions for a function to be continuous on a closed interval and differentiable on an open interval.
Explanation of the Mean Value Theorem's implication that there exists at least one point where the derivative equals the slope of the line connecting the endpoints.
Visual representation of the theorem with a graph illustrating the continuous and differentiable function.
Clarification on the necessity of a function being smooth for differentiability, avoiding sharp points, cusps, or kinks.
Demonstration of calculating the slope of the line connecting endpoints as the change in Y over change in X.
Illustration of the intuitive idea behind the theorem using a generic graph.
Procedure to justify the use of the Mean Value Theorem by confirming a function's continuity and differentiability over an interval.
Example function f(x) = 1 + cube root of (x - 1) with an interval from 2 to 9 for applying the Mean Value Theorem.
Verification of the function's continuity on its domain, ensuring its applicability for the theorem.
Differentiability of the function, except at x = 1, allowing the use of the Mean Value Theorem within the interval [2, 9].
Derivation of the function's derivative using the chain rule.
Identification of the point where the derivative is undefined, which is x = 1.
Calculation of the value for the Mean Value Theorem's condition using the example function and interval.
Solution for the point C in the interval [2, 9] where the derivative equals the slope of the endpoints line.
Algebraic manipulation to solve for C, including raising both sides to the power of 3/2.
Verification of the solution to ensure it falls within the specified interval [2, 9].
Conclusion of the example problem, confirming the existence of a point satisfying the Mean Value Theorem's condition.
Encouragement for viewers to engage with comments and seek clarification if needed.
Transcripts
Browse More Related Video

Calculus 1 Lecture 3.2: A BRIEF Discussion of Rolle's Theorem and Mean-Value Theorem.
Mean Value Theorem with Example
The MEAN Value Theorem is Actually Very Nice

AP Calculus Review Three Theorems You Must Know (EVT, IVT, MVT)
The Mean Value Theorem For Integrals: Average Value of a Function

2011 Calculus AB free response #4d | AP Calculus AB | Khan Academy
5.0 / 5 (0 votes)
Thanks for rating: