AP Calculus Review Three Theorems You Must Know (EVT, IVT, MVT)

turksvids
14 Apr 201809:47
EducationalLearning
32 Likes 10 Comments

TLDRThe video script discusses three crucial theorems for the AP exam: the Extreme Value Theorem (EVT), the Intermediate Value Theorem (IVT), and the Mean Value Theorem (MVT). The EVT states that a continuous function on a closed interval must attain a maximum and minimum, emphasizing the importance of continuity over differentiability. The IVT asserts that a continuous function on a closed interval, taking on values at the endpoints, must also take on any value between those at some point within the interval. Lastly, the MVT posits that for a function that is continuous on a closed interval and differentiable on an open interval, there exists at least one point where the derivative equals the average rate of change over that interval. The video emphasizes the practical application of these theorems in solving problems and advises students to be well-versed in them for the AP exam, suggesting practice with multiple-choice and free-response questions.

Takeaways
  • 📌 The Extreme Value Theorem (EVT) states that a continuous function on a closed interval attains a maximum and a minimum on that interval.
  • 📌 The Intermediate Value Theorem (IVT) asserts that a continuous function on a closed interval takes every value between its endpoints at some point within the interval.
  • 📌 The Mean Value Theorem (MVT) posits that for a function that is continuous on a closed interval and differentiable on the open interval, there exists at least one point where the derivative equals the average rate of change over the interval.
  • 📌 Continuity is a requirement for EVT and IVT, while MVT additionally requires differentiability.
  • 📌 Differentiability implies continuity, which is crucial for applying these theorems when a function is described as differentiable.
  • 📌 EVT is often used as a justification in multiple-choice questions without being explicitly named.
  • 📌 IVT is commonly used to prove equalities and is frequently applied in table problems and compositions.
  • 📌 MVT is used to move between levels of a function, such as using the function to prove something about its derivative or vice versa.
  • 📌 Rolle's Theorem is a special case of MVT where the function has the same value at the endpoints, implying the existence of a point with a derivative of zero.
  • 📌 When applying MVT, it is important to state the conditions (continuity and differentiability) and show that they are met.
  • 📌 Practice with a variety of problems, including multiple-choice and free-response questions, is essential for mastering these theorems for the AP exam.
Q & A

  • What are the three theorems discussed in the video that are likely to appear on the AP exam?

    -The three theorems discussed are the Extreme Value Theorem (EVT), the Intermediate Value Theorem (IVT), and the Mean Value Theorem.

  • What is the Extreme Value Theorem (EVT) and what does it state?

    -The Extreme Value Theorem (EVT) states that if a function is continuous on a closed interval from A to B, then the function must attain a maximum and a minimum on that interval at least once.

  • Why is it important to remember that differentiability implies continuity?

    -It is important because often you are told a function is differentiable, and from this, you can infer that it is also continuous. This allows you to apply theorems like EVT that require continuity.

  • How is the Intermediate Value Theorem (IVT) applied in the context of the AP exam?

    -The IVT is used to prove that a function must be equal to a certain value within a given interval if it is continuous and takes specific values at the endpoints of the interval.

  • What does the Mean Value Theorem state and what are the conditions required for its application?

    -The Mean Value Theorem states that if a function is continuous on a closed interval and differentiable on an open interval, then there exists at least one point in the open interval where the derivative of the function equals the average rate of change over the interval. The conditions are that the function must be continuous on the closed interval and differentiable on the open interval.

  • How does the Mean Value Theorem allow you to move between different levels of a function?

    -The Mean Value Theorem allows you to use information about the function (F) to prove things about its derivative (F'), and vice versa. It bridges the gap between different levels of the function.

  • What is Rolle's Theorem and how does it relate to the Mean Value Theorem?

    -Rolle's Theorem is a special case of the Mean Value Theorem where the function takes the same value at the endpoints of the interval. It allows you to find a point where the derivative of the function is zero.

  • How can the Mean Value Theorem be used to prove that a function is increasing or decreasing on a certain interval?

    -By showing that the derivative of the function (F') is positive (for increasing) or negative (for decreasing) on the interval, using the Mean Value Theorem to find a point where this condition holds.

  • What are some common pitfalls or misconceptions regarding the application of these theorems on the AP exam?

    -Common pitfalls include assuming a function is differentiable when it is only stated to be continuous, or overlooking the fact that the Mean Value Theorem requires both continuity and differentiability on specific intervals.

  • Why is it recommended to practice with a variety of Free Response Questions (FRQs) when studying for the AP exam?

    -Practicing with FRQs helps to solidify understanding of the theorems and their applications, as these questions often require a deep understanding and the ability to apply the theorems in different contexts.

  • What is the significance of the Mean Value Theorem in calculus and why is it important for students to master it?

    -The Mean Value Theorem is significant because it connects the concepts of derivatives and slopes of tangent lines to the average rate of change over an interval. Mastering it is important as it is widely used in calculus to solve a variety of problems and is a key topic on the AP exam.

  • How can a student ensure they are correctly applying the theorems discussed in the video?

    -Students should ensure they understand the conditions required for each theorem, state these conditions when applying the theorem in their work, and practice problems that test these theorems to reinforce their understanding.

Outlines
00:00
📚 Introduction to Theorems for AP Exam

The video introduces three key theorems that are crucial for the AP Calculus exam: the Extreme Value Theorem (EVT), the Intermediate Value Theorem (IVT), and the Mean Value Theorem (MVT). The presenter emphasizes that while the EVT may not be explicitly mentioned, it is fundamental for understanding and justifying solutions without naming it. The IVT and MVT are more commonly used and require continuity and differentiability, respectively. The video focuses on conceptual understanding rather than problem-solving, aiming to ensure that viewers are familiar with these theorems for the exam.

05:01
🔍 Deep Dive into Calculus Theorems

This paragraph delves deeper into the application and importance of the Mean Value Theorem (MVT) in calculus. It highlights the conditions necessary for using the MVT, which include a function being continuous on a closed interval and differentiable on an open interval. The presenter advises to always state these conditions when applying the theorem in Free Response Questions (FRQs). The MVT is used to relate the behavior of a function to its derivatives, allowing for proofs about the function's rate of change (F prime). The video also discusses strategies for using the MVT, such as finding points where the function's rate of change is zero (Rolle's Theorem), and provides examples of how the theorem might appear in multiple-choice and FRQ formats on the AP exam.

Mindmap
Keywords
💡Extreme Value Theorem (EVT)
The Extreme Value Theorem is a fundamental concept in calculus stating that if a function is continuous on a closed interval, it must attain both a maximum and a minimum value within that interval. In the context of the video, this theorem is crucial for understanding the behavior of functions on specific intervals and is often used as a justification in AP exam problems without being explicitly mentioned by name. An example from the script is the mention of a function being continuous, which implies it must have an absolute minimum and maximum.
💡Intermediate Value Theorem (IVT)
The Intermediate Value Theorem asserts that if a function is continuous on a closed interval and takes on values F(a) and F(b) at the endpoints, then it must also take on any value between F(a) and F(b) at some point within the interval. This theorem is frequently used in the video to demonstrate that a function must equal a certain value within a given range and is applicable to both the function and its derivative. For instance, the script mentions using IVT to prove that f(x) equals a specific value like 12.
💡Mean Value Theorem (MVT)
The Mean Value Theorem posits that if a function is continuous on a closed interval and differentiable on the open interval within that range, there exists at least one point 'C' where the derivative of the function at 'C' is equal to the average rate of change of the function over the interval. This theorem is central to the video's discussion on calculus and is used to connect different levels of a function, such as using the value of a function to prove something about its derivative. An example given is that if f is continuous and differentiable on an interval and f(3) equals f(7), then by MVT, there exists a 'C' such that f'(C) equals zero.
💡Continuity
Continuity of a function is a property that implies there are no breaks, jumps, or asymptotes in the graph of the function. In the video, continuity is a prerequisite for applying both the EVT and IVT, making it a key concept for understanding the behavior of functions on closed intervals. The script emphasizes that while differentiability implies continuity, one must be aware of when a function is only stated to be differentiable to ensure the continuity condition is met.
💡Differentiability
A function is differentiable at a point if it has a derivative at that point, meaning the rate at which the function changes can be calculated. In the context of the video, differentiability is important because it is a stronger condition than continuity but often implies it. The Mean Value Theorem specifically requires differentiability, which is highlighted in the video when discussing the conditions under which one can apply the theorem.
💡Rolle's Theorem
Rolle's Theorem is a corollary of the Mean Value Theorem and is mentioned in the video as a special case where a function has the same value at the endpoints of an interval. It states that under these conditions, there is at least one point in the interval where the derivative of the function is zero. The script uses Rolle's Theorem to illustrate a situation where the slope of the secant line is zero, indicating a flat tangent line at some point within the interval.
💡Slope of Secant Line
The slope of the secant line is the average rate of change of a function between two points. It is a concept used in the video to explain the Mean Value Theorem, where the slope of the tangent line at a point 'C' is shown to be equal to the slope of the secant line between the endpoints of the interval. The script uses this concept to demonstrate how one can find a point where the derivative of the function equals a certain value.
💡Free Response Questions (FRQs)
Free Response Questions are a type of question found on the AP exam that require students to write out their solutions and reasoning. In the video, FRQs are discussed in the context of applying the theorems to various problems, such as proving that a function is increasing or decreasing at a point or finding a value of 'C' that satisfies a certain condition. The script emphasizes the importance of stating the conditions of the theorems when using them to solve FRQs.
💡Multiple Choice Questions
Multiple Choice Questions are a standard part of the AP exam format where students select the correct answer from a list of options. The video script discusses how the theorems are often used as justifications in these types of questions, where understanding the theorems can help students discern which options are true or false. An example given is a scenario where a function's continuity is used to determine the truth of a statement about the function's behavior.
💡Table Problems
Table Problems are a specific type of question on the AP exam that present a table of values and ask students to analyze and draw conclusions based on that data. In the video, table problems are mentioned as a common context where the Intermediate Value Theorem and Mean Value Theorem can be applied, such as when determining the behavior of a function at specific points or proving certain properties about the function.
💡Practice
The importance of practice is emphasized throughout the video as a key strategy for mastering the theorems and successfully applying them to AP exam questions. The script suggests that students engage in a lot of practice with FRQs and multiple-choice questions to become familiar with how the theorems are used in different contexts and to improve their problem-solving skills.
Highlights

The extreme value theorem (EVT) states that if a function is continuous on a closed interval [A, B], it must attain a maximum and minimum on that interval.

EVT is the least commonly mentioned of the three theorems on the AP exam, but it's important to know as it often comes up as a justification in multiple-choice questions.

The intermediate value theorem (IVT) states that if a function is continuous on a closed interval [A, B] and takes values f(A) and f(B), it also takes any value between f(A) and f(B) at some point within the interval.

IVT is used to prove equalities, such as f(x) = 12, and is commonly used on effort cues and table problems.

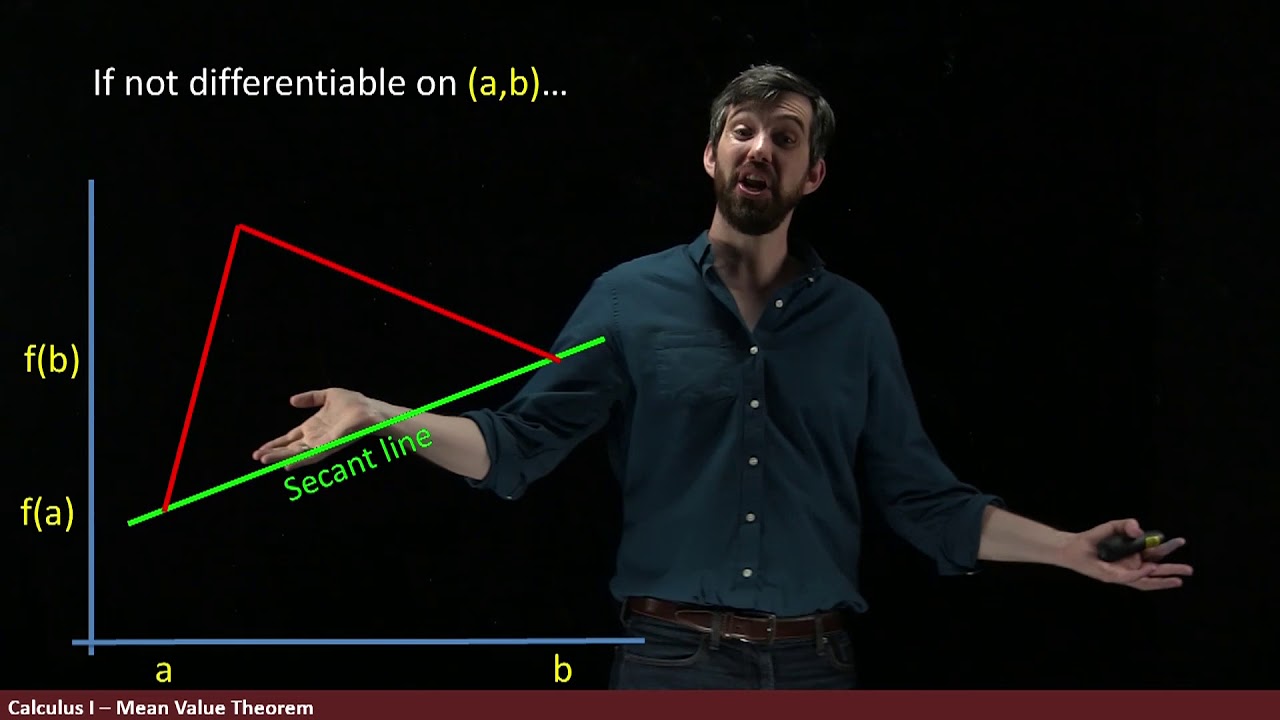
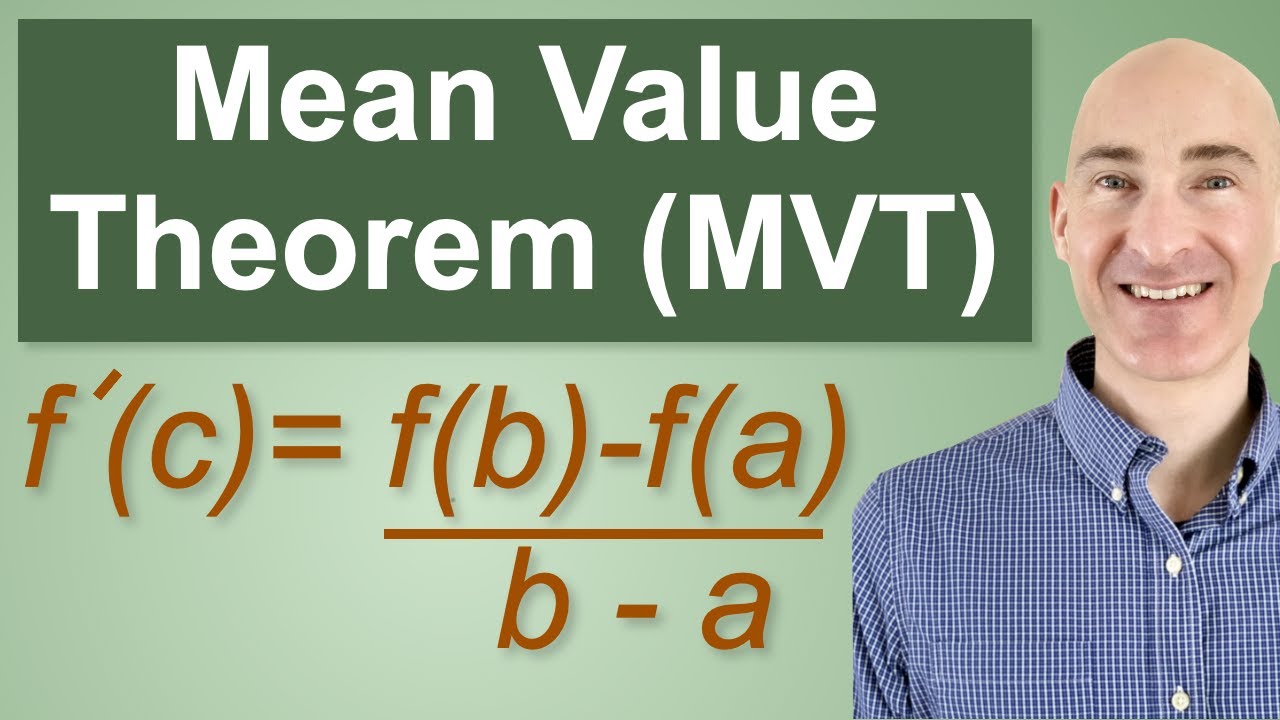
Mean value theorem (MVT) states that if a function f is continuous on [a, b] and differentiable on (a, b), there exists a point c in (a, b) such that f'(c) = (f(b) - f(a)) / (b - a).

MVT is the most calculus-based of the three theorems and is used to move between levels, e.g. using f to prove things about f' and vice versa.

To use MVT, you need to verify that the function is continuous on the closed interval and differentiable on the open interval.

Rolle's theorem is a special case of MVT where f(a) = f(b), which implies that there exists a point c where f'(c) = 0.

MVT is commonly used on multiple-choice questions to find a value of c, determine the number of guaranteed c values, or show that no c value exists.

On free-response questions, MVT is frequently used to prove that a function is increasing/decreasing on an interval or to show that f' is not always positive/negative on an interval.

MVT is often used to show that f'(c) equals a specific value, which is the most common application on table problems.

It's important to practice using MVT on a variety of problem types, as it comes up frequently on the AP exam, especially on table problems.

Differentiability implies continuity, so if a function is given as differentiable, it is also continuous and the continuity-based theorems (EVT, IVT) can be applied.

When using a theorem on a multiple-choice question, be sure to check if the function is continuous or differentiable as required by the theorem.

Be cautious of multiple-choice questions that try to trick you with a function that is only continuous but not differentiable.

If a function is given as twice differentiable, it means both f and f' are continuous and differentiable on the required intervals.

When using MVT to prove a statement about f' or f'', make sure to state the continuity and differentiability conditions that you are assuming.

In summary, know the extreme value theorem, intermediate value theorem, and mean value theorem well and practice applying them on a variety of AP exam problems.

Transcripts

Browse More Related Video

Using the Mean Value Theorem with a Table of Values

Mean Value Theorem and Rolle's Theorem

The MEAN Value Theorem is Actually Very Nice

Calculus 1 Lecture 3.2: A BRIEF Discussion of Rolle's Theorem and Mean-Value Theorem.

Mean value theorem | Existence theorems | AP Calculus AB | Khan Academy

Mean Value Theorem with Example

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
AP CalculusTheoremsExtreme ValueIntermediate ValueMean ValueExam PrepContinuous FunctionsDifferentiabilityMathematical AnalysisEducational VideoTheorem ApplicationCalculus Exam