One-way ANOVA & Post-Hoc Analysis in Excel

Dr. Jacob Goodin
6 Nov 202010:20
EducationalLearning
32 Likes 10 Comments

TLDRThis instructional video demonstrates how to perform a one-way ANOVA in Excel to determine if there are significant differences in means among multiple groups. It uses a dataset of athletes' isometric peak force across three sports. After explaining the process and significance of ANOVA, the video guides viewers through post-hoc Bonferroni adjusted pairwise comparisons to identify specific group differences, ensuring a clear understanding of statistical analysis in sports science.

Takeaways
  • 📊 The video demonstrates how to perform a one-way ANOVA (Analysis of Variance) in Excel to determine if there are differences in means among multiple groups.
  • 🔍 The context of the analysis involves comparing the isometric peak force (IPF) among three groups of athletes: tennis, football, and basketball players.
  • 📈 The video explains that a one-way ANOVA is used when there are more than two groups and the goal is to assess whether the groups come from the same population.
  • 🤔 It's important to note that ANOVA does not specify which groups are different; it only indicates if there is a difference somewhere among the groups.
  • 📝 The script provides a step-by-step guide on how to arrange the data in Excel, highlighting the need to separate data by groups due to Excel's limitations.
  • 📉 The descriptive statistics table generated by Excel is used to compare the average IPF among the different sports groups, with football players showing the highest average.
  • 📊 The ANOVA table in Excel provides the sum of squares, degrees of freedom, mean squares, and the F value, which is used to determine statistical significance.
  • 🔑 A significant F value and a small p-value from the ANOVA indicate that there is a difference among the groups, but further analysis is needed to pinpoint which groups differ.
  • 🔍 Post-hoc analysis, specifically pairwise comparisons with a Bonferroni adjustment, is recommended to control for family-wise error when multiple comparisons are made.
  • 📐 The Bonferroni correction is applied by dividing the alpha level (0.05) by the number of comparisons to reduce the chance of a Type I error.
  • 📝 The video concludes with the results of the pairwise comparisons, showing significant differences in IPF among all three groups of athletes even after applying the Bonferroni adjustment.
Q & A

  • What statistical test is being discussed in the video?

    -The video discusses how to run a one-way Analysis of Variance (ANOVA) in Excel.

  • What is the purpose of a one-way ANOVA?

    -A one-way ANOVA is used to determine if there is a statistically significant difference in means among three or more groups.

  • Why is a post-hoc analysis necessary after a one-way ANOVA?

    -A post-hoc analysis is necessary to identify which specific groups differ from each other, as ANOVA only tells us if there is a difference somewhere among the groups.

  • What type of athletes are included in the dataset used in the video?

    -The dataset includes tennis, football, and basketball athletes.

  • What does IPF stand for in the context of the video?

    -IPF stands for Isometric Peak Force, a measure of the maximum force that athletes can generate isometrically.

  • How is isometric peak force measured in the video's dataset?

    -Isometric peak force is measured in newtons, not in pounds or kilograms.

  • What Excel tool is used to perform a one-way ANOVA?

    -The 'Data Analysis Tool Pack' in Excel is used to perform a one-way ANOVA.

  • What is the significance of the F value in ANOVA?

    -The F value is the ratio of the between-group mean squares to the within-group mean squares, and it is used to determine if the variance between groups is significantly different from the variance within groups.

  • What is the Bonferroni adjustment used for in post-hoc analysis?

    -The Bonferroni adjustment is used to reduce the risk of type I error (false positives) when performing multiple pairwise comparisons by dividing the alpha level by the number of comparisons.

  • How many pairwise comparisons are made in the video's example?

    -Three pairwise comparisons are made: tennis to football, tennis to basketball, and football to basketball.

  • What is the new alpha level after applying the Bonferroni correction in the video?

    -The new alpha level is 0.05 divided by the number of comparisons, which is 3, resulting in an alpha level of approximately 0.0167.

Outlines
00:00
📊 Running a One-Way ANOVA in Excel

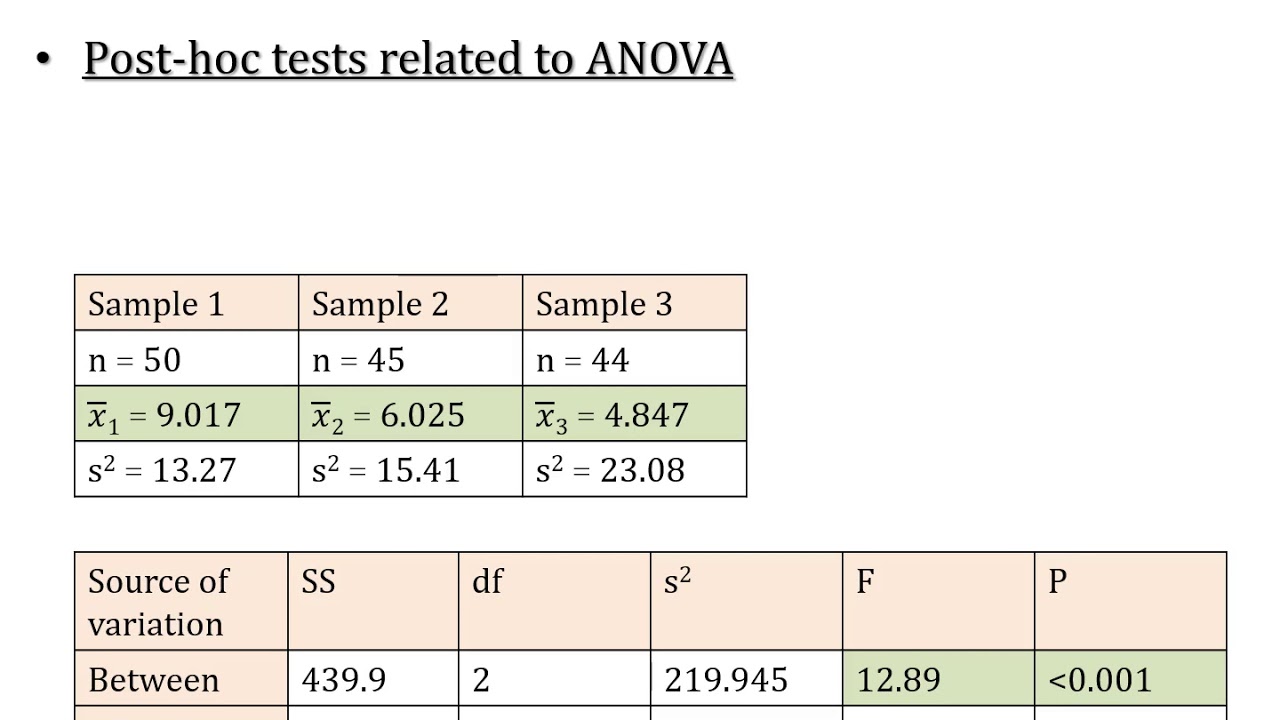
This paragraph introduces a tutorial video on how to conduct a one-way ANOVA in Excel, which is used to analyze if there are significant differences in means between three or more groups. The video will also cover a post-hoc follow-up with a Bonferroni adjustment to determine which groups differ significantly from each other. The example uses data from athletes of different sports, measuring their isometric peak force, and explains the process of setting up the data in Excel, running the ANOVA test, and interpreting the results.

05:01
🔍 Post-Hoc Analysis with Bonferroni Adjustment

The second paragraph delves into the necessity of post-hoc analysis when a significant result is obtained from the ANOVA test. It explains the Bonferroni adjustment method for pairwise comparisons to control the family-wise error rate when conducting multiple tests. The video demonstrates how to perform t-tests for each pair of groups and then apply the Bonferroni correction to determine the significance of the differences between them. The results indicate significant differences in isometric peak force among the groups of athletes from different sports.

10:03
📚 Conclusion and Further Learning

The final paragraph of the script is incomplete, but it seems to be an introduction to another segment of the video or a continuation of the tutorial. It suggests that the presenter will continue to show how to perform a one-way ANOVA and possibly address more complex statistical analyses in future videos. The paragraph encourages viewers to explore further topics in kinesiology and statistics through the provided playlists.

Mindmap
Keywords
💡One-Way ANOVA
One-Way ANOVA, or Analysis of Variance, is a statistical method used to determine if there are any differences between the means of three or more independent groups. In the video, the presenter uses One-Way ANOVA to analyze the differences in isometric peak force among three groups of athletes: tennis, football, and basketball players. The video demonstrates how to perform this analysis in Excel to test the hypothesis that all groups come from the same population.
💡Post-Hoc Analysis
Post-Hoc Analysis is conducted after an ANOVA test to determine which specific groups differ from each other when the ANOVA indicates a significant overall effect. In the context of the video, post-hoc analysis is necessary because the initial ANOVA test shows that there is a difference among the groups, but it doesn't specify which groups are different. The presenter explains how to perform pairwise comparisons with a Bonferroni adjustment in Excel to identify these differences.
💡Bonferroni Adjustment
The Bonferroni Adjustment is a method used to control the familywise error rate in multiple comparisons. In the video, the presenter uses this adjustment to reduce the chance of Type I errors when conducting multiple pairwise comparisons. By dividing the alpha level (0.05) by the number of comparisons, the Bonferroni method ensures that the significance level is adjusted to account for multiple tests, as seen when comparing the isometric peak force of tennis, football, and basketball athletes.
💡Isometric Peak Force (IPF)
Isometric Peak Force, abbreviated as IPF, is a measure of the maximum force that can be generated by an athlete without changing joint angles. It is a common metric in sports science and kinesiology to assess an athlete's maximum strength. In the video, IPF is the dependent variable being analyzed to determine if there are differences in strength among different types of athletes.
💡Dependent Variable
A dependent variable is the variable that is being measured or tested in an experiment and is expected to change as a result of the manipulation of the independent variable. In the video, IPF is the dependent variable, as it is the measure that changes based on the type of sport the athletes play.
💡Independent Variable
An independent variable is the variable that researchers manipulate in an experiment to observe its effect on the dependent variable. In the context of the video, the type of sport (tennis, football, basketball) is the independent variable, as it is used to categorize the athletes and analyze its effect on their IPF.
💡Descriptive Statistics
Descriptive Statistics are used to summarize and describe the characteristics of a data set. In the video, the presenter refers to a descriptive table generated by Excel's ANOVA tool, which includes averages for each group. These descriptive statistics provide an initial understanding of the data, such as the average IPF for each type of athlete.
💡Sum of Squares
Sum of Squares is a statistical measure that represents the sum of the squared differences between observed values and their mean. In the video, the presenter discusses the sum of squares between groups and within groups as part of the ANOVA table. These values are used to calculate the mean squares, which are crucial for determining the F-value.
💡Degrees of Freedom
Degrees of Freedom in statistics is a measure of the number of values that are free to vary in a calculation. In the context of ANOVA, the degrees of freedom for the between groups and within groups are mentioned in the video. These values are important for determining the critical F-value and for understanding the distribution of the test statistic.
💡T-Test
A T-Test is a statistical method used to determine if there is a significant difference between the means of two groups. In the video, the presenter uses T-Tests to perform pairwise comparisons between the groups of athletes after the initial ANOVA indicates a significant overall effect. The T-Tests are conducted assuming equal variances, which is a common assumption when the sample sizes are similar.
Highlights

The video demonstrates how to conduct a one-way ANOVA in Excel with a post-hoc follow-up.

One-way ANOVAs are used when there are multiple groups to determine if there is a difference in means.

The analysis does not specify which groups differ but indicates if any groups differ from the rest.

A significant p-value and large F-value from ANOVA necessitate a post hoc analysis.

Excel is used to perform the analysis with a Bonferroni adjustment to reduce family-wise error.

The dataset consists of three groups of athletes: tennis, football, and basketball players.

IPF, or isometric peak force, is the dependent variable measured in newtons.

IPF measures the maximum force generated isometrically without changing joint angles.

The aim is to determine if there are differences in IPF among the three types of athletes.

Data must be arranged in separate columns in Excel for the ANOVA analysis.

Excel's Data Analysis Toolpak is used to perform the Single Factor ANOVA.

Descriptive statistics and averages from each group are provided by the ANOVA summary table.

The F-value and p-value from the ANOVA table indicate a statistically significant difference among the groups.

Post hoc analysis involves pairwise comparisons using t-tests with Bonferroni adjustments.

Bonferroni adjustments reduce the alpha level to decrease the chance of type 1 errors.

T-tests are conducted for each pair of athlete groups to identify specific differences.

Even with the Bonferroni adjustment, all pairwise comparisons show significant differences.

The process can be applied to datasets with more than three groups and a single independent factor.

The video will cover more complex ANOVA and post hoc analysis in future content.

Transcripts

Browse More Related Video

Performing a One-way ANOVA in Excel with post-hoc t-tests

Fisher's LSD Explained

Perform ANOVA Post-Hoc Tests (Bonferroni Correction) In Excel

Understanding Analysis of Variance (ANOVA) including Excel - Statistics Help

One-Way ANOVA with LSD (Least Significant Difference) Post Hoc Test in Excel

LSD; Least Significant Difference; Post Hoc Test of ANOVA; Comparison of Means (Part A)

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
ANOVA TutorialExcel AnalysisPost-Hoc TestsBonferroni CorrectionStatistical SignificanceSports ScienceIsometric Peak ForceData InterpretationAthlete StrengthStatistical Methods