Elementary Stats Lesson #7
TLDRIn this week four lesson, the focus is on introducing probability, covering its basic concepts and rules. The instructor outlines the importance of understanding probability for statistical procedures and preparing for the upcoming test in week five. The lesson covers the definition of probability, types of events, sample spaces, and probability assignments. It also introduces the law of large numbers, the empirical and theoretical approaches to probability, and the addition rule for combining events. Key takeaways include understanding unusual events and the rule of complements, all essential for advancing in statistical studies.
Takeaways
- 📚 This is the fourth week of the semester, focusing on lessons seven and eight, which introduce the concept of probability.
- 🔮 Next week is crucial as it's week five with the first test scheduled for the A and B groups on Tuesday and Thursday, respectively.
- 📈 Probability is defined as the science of chance behavior, providing a measure of the likelihood of an event's occurrence.
- 🎯 A probability experiment is a process with uncertain results that can be repeated, allowing for the study of long-term relative frequency of events.
- 🎲 The sample space is the set of all possible outcomes of an experiment, and it's important to determine if outcomes are equally likely for probability assignments.
- 👉 An event can be a single outcome (simple event) or a collection of outcomes (compound event), differentiated by lowercase and uppercase letters, respectively.
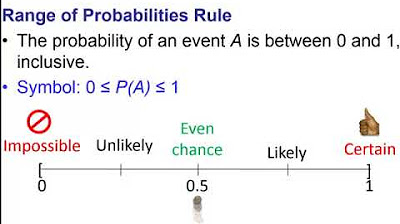
- 📝 Basic rules of probability state that probabilities must be between 0 and 1, and the sum of probabilities of all outcomes must equal one.
- 🤔 The law of large numbers supports the idea that as the number of trials increases, the observed relative frequency of an event will approach its true probability.
- 📉 Probability assignments can be made using the empirical method, which relies on actual data and relative frequencies, or the classical approach, which assumes equally likely outcomes.
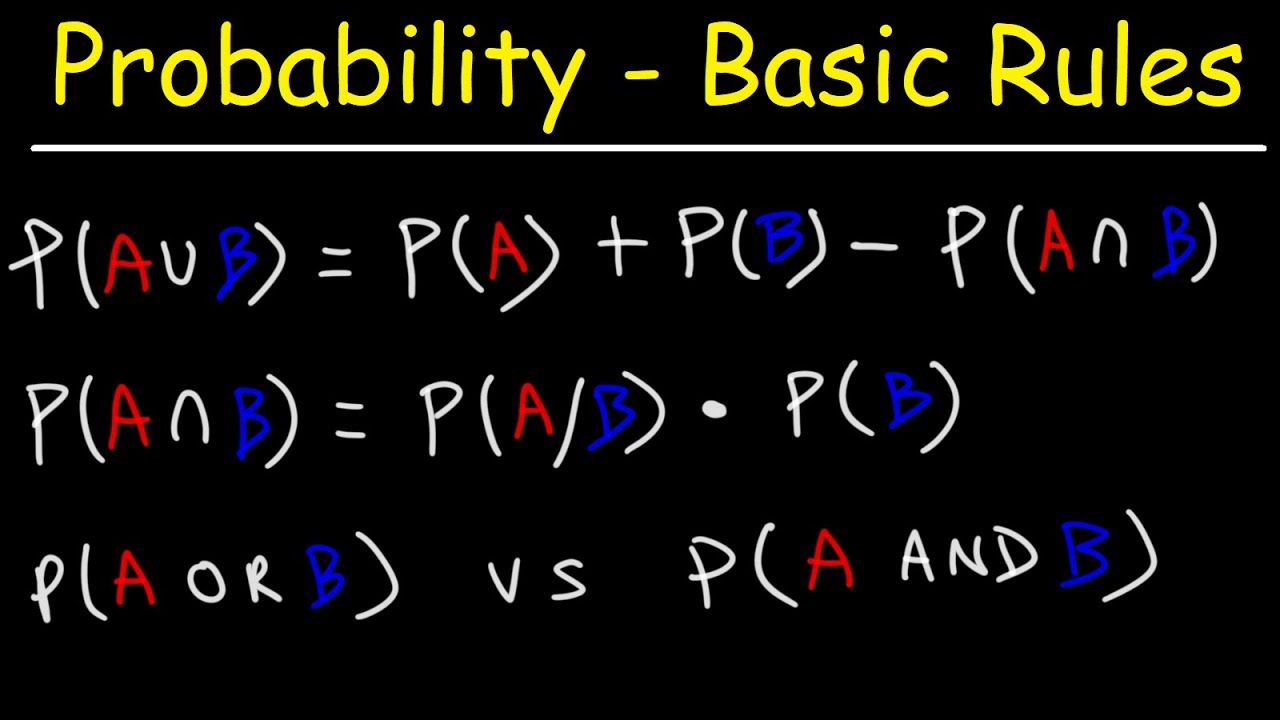
- 📊 The addition rule for probabilities allows for the calculation of the probability of two events occurring together, considering whether they are mutually exclusive or not.
- 🚫 The rule of complements states that the probability of an event not occurring is one minus the probability of the event occurring, providing a way to calculate the opposite outcome.
Q & A
What is the main topic of Chapter 5?
-The main topic of Chapter 5 is an introduction to probability.
When is the first test scheduled, and how are the groups divided?
-The first test is scheduled for week number five. The A group will take their exam on Tuesday, and the B group will take their exam on Thursday.
What is the definition of probability as mentioned in the lesson?
-Probability is defined as the science of chance behavior, and it measures the likelihood of the occurrence of an event of interest.
What is a probability experiment?
-A probability experiment is any process that has uncertain results and can be repeated. It is also referred to as a random experiment.
How are simple and compound events notated differently?
-Simple events are notated with lowercase letters like e1, e2, and compound events are notated with capital letters like E1, E2, or capital letters like A, B.
What are the two key characteristics of a sample space?
-The two key characteristics of a sample space are the size of the sample space (the number of outcomes) and whether the outcomes are equally likely to occur.
What is the law of large numbers?
-The law of large numbers states that as the number of trials of a random experiment increases, the proportion with which an event occurs will approach the true probability of that event.
What is a binomial experiment?
-A binomial experiment is one that has only two possible outcomes. For example, in the context of shooting a free throw, the outcomes are either success (S) or failure (F).
How do you assign probabilities using the empirical method?
-In the empirical method, probabilities are assigned by running the random experiment many times and calculating the long-term relative frequencies of the simple outcomes.
What is the rule of complements?
-The rule of complements states that the probability of an event not occurring is 1 minus the probability of the event occurring. Mathematically, P(E') = 1 - P(E).
Outlines
📚 Introduction to Week 4 and Probability Lessons
The instructor welcomes everyone back for the fourth week of the semester, outlining the goals for the week, which include covering lessons seven and eight, focusing on Chapter Five about probability. The instructor previews that week five will be crucial as it includes the first test for the course. The importance of probability in understanding uncertainty and its foundational role in statistical procedures is emphasized.
🔢 Understanding Probability and its Basics
Probability is introduced as the science of chance behavior, described simply as a measure of the likelihood of an event occurring. The instructor explains that probability is a number representing the long-term relative frequency of an event. The concept of a probability experiment is introduced, defined as any repeatable process with uncertain results, and the importance of these experiments in measuring the likelihood of events is discussed.
🎲 Examples of Probability Experiments and Sample Spaces
Various probability experiments are discussed, including rolling dice and observing outcomes, shooting a free throw, and drawing cards from a deck. The concept of a sample space is defined as the list of all possible outcomes of an experiment. The instructor explains how to determine the size of the sample space and assess whether the outcomes are equally likely. Examples illustrate how to list and count possible outcomes in simple probability experiments.
📊 Probability Assignments and Basic Rules
The process of assigning probabilities to outcomes within a sample space is outlined, with a focus on ensuring that probabilities are between 0 and 1 and that the sum of all probabilities equals 1. The instructor draws parallels between these rules and those for relative frequency distributions. The main idea is that the probability of an outcome is the long-term relative frequency, requiring a large number of trials to ensure accuracy.
🧮 Calculating Probabilities and the Law of Large Numbers
The Law of Large Numbers is introduced, explaining that as the number of trials increases, the proportion of times an event occurs will approach its true probability. The relevance of this law to probability calculations is discussed. The importance of probability in statistical sampling and its role in determining confidence measures is emphasized.
🔍 Developing a Probability Model
The instructor explains how to construct a full probability model, which includes defining the experiment, identifying the sample space, and assigning probabilities to each outcome. The importance of following basic rules to ensure the sum of probabilities equals one and that each probability is between 0 and 1 is reiterated. Examples are provided to illustrate how to combine outcomes to form compound events and calculate their probabilities.
💡 Understanding Unusual Events and Probability Models
The concept of unusual events is defined as those with a low probability (less than 0.05). The significance of identifying unusual events in probability models is highlighted. The instructor introduces the empirical and theoretical approaches to assigning probabilities, noting the importance of equally likely outcomes for the theoretical approach and the reliance on actual data for the empirical approach.
📏 Empirical and Theoretical Probability Models
The difference between empirical and theoretical probability models is elaborated. The empirical approach involves calculating long-term relative frequencies from actual data, suitable for non-equally likely outcomes. The theoretical approach, applicable when outcomes are equally likely, simplifies probability assignment to one divided by the number of outcomes. Examples illustrate how to apply these methods in practice.
🧮 Addition Rule and General Addition Rule for Probabilities
The instructor introduces the addition rule for calculating the probability of either of two events occurring. If events are disjoint (mutually exclusive), their probabilities are simply added. For non-disjoint events, the probability of their intersection must be subtracted to avoid double counting. Examples involving simple and compound events are provided to illustrate these concepts.
🔄 Rule of Complements and Calculation Examples
The rule of complements is explained, which states that the probability of an event not occurring is one minus the probability of the event occurring. This rule simplifies many probability calculations. Examples demonstrate how to use the rule of complements and the addition rule to calculate probabilities in various scenarios, reinforcing the practical application of these rules in probability theory.
📊 Venn Diagrams and Probability Calculations
The use of Venn diagrams to visualize sample spaces and probabilities is discussed. Examples illustrate how to list outcomes and calculate probabilities for events and their complements using Venn diagrams. The importance of understanding intersections and unions of events for accurate probability calculations is emphasized.
🔢 Counting Techniques and Probability Assignments
The instructor wraps up the lesson by introducing counting techniques that will be covered in the next session. These techniques will help in dealing with larger sample spaces and more complex probability calculations. The connection between counting techniques and probability assignments is highlighted as a crucial aspect of understanding and applying probability theory.
📚 Preview of Upcoming Topics and First Test Preparation
A preview of upcoming topics is provided, including advanced probability models and counting techniques. The instructor encourages students to stay safe and keep up with the course material as they prepare for the first test, which will cover the initial five chapters. The importance of mastering these foundational concepts for future lessons is emphasized.
Mindmap
Keywords
💡Probability
💡Sample Space
💡Event
💡Relative Frequency
💡Binomial Experiment
💡Empirical Method
💡Theoretical Approach
💡Addition Rule
💡Rule of Complements
💡Unusual Event
Highlights
Introduction to probability in Week 4, focusing on lessons 7 and 8 covering Chapter 5.
Next week is crucial with Test 1 for groups A and B scheduled in Week 5.
Definition of probability as the science of chance behavior.
Probability is a measure of the likelihood of the occurrence of an event.
Long-term relative frequency is key to understanding probability.
Definition and distinction between simple and compound events in probability.
Introduction to the concept of a sample space as a list of all possible outcomes.
Example of determining the sample space and its size using dice rolls.
Importance of identifying whether outcomes in a sample space are equally likely.
Explanation of probability assignment using relative frequency from long-term data.
Law of large numbers: as the number of trials increases, the observed proportion approaches the true probability.
Using simple random sampling from a population as a probability experiment with equally likely outcomes.
Probability calculations are essential for determining measures of confidence in statistical results.
General addition rule for combining probabilities of events, particularly when they are not mutually exclusive.
Introduction to the rule of complements for calculating the probability of an event not occurring.
Use of Venn diagrams to visually represent sample spaces and probabilities.
Empirical vs. theoretical approaches to probability assignment based on equally likely outcomes.
Example of probability calculation using a single fair die and identifying event probabilities.
Summary of upcoming topics and the importance of understanding probability for future statistical procedures.
Transcripts
Browse More Related Video

Elementary Stats Lesson #8
Chapter 4 Probability Part 1

4.1.0 Basics of Probability - Lesson Overview, Key Concepts and Learning Outcomes

What is probability | Expected Values, Frequency Distribution, Complement

AP Stats Test Quick Review: Probability
Multiplication & Addition Rule - Probability - Mutually Exclusive & Independent Events
5.0 / 5 (0 votes)
Thanks for rating: