What is probability | Expected Values, Frequency Distribution, Complement

365 Data Science

12 Feb 201920:45

EducationalLearning

32 Likes 10 Comments

TLDRThis script delves into the concept of probability, essential for decision-making in business and life. It explains the basics of probability, including events, outcomes, and their numerical expression. The video illustrates how to calculate probabilities for simple and complex events, introduces the idea of expected values, and discusses experimental probabilities. It also covers probability distributions, complements of events, and the importance of the sum of probabilities equaling one, providing a comprehensive foundation for understanding statistical predictions and outcomes.

Takeaways

🧩 Life is full of uncertain events and decisions often require an understanding of probabilities to assess risk.
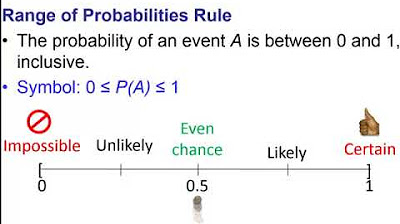
📊 Probability is a measure of the likelihood of an event occurring, expressed as a real number between 0 and 1.
🎲 An event in probability is a specific outcome or a combination of outcomes, like getting heads in a coin flip.
📉 Probabilities are numerically expressed to measure and compare the likelihood of different events.
🔢 A probability of '1' indicates certainty of an event occurring, while '0' indicates certainty of it not occurring.
🎯 The probability of an event is calculated by dividing the number of favorable outcomes by the total number of possible outcomes.
👉 The concept of 'sample space' includes all possible outcomes of an experiment, synonymous with 'favourable outcomes'.
🎯 The probability of independent events occurring together is found by multiplying their individual probabilities.
🔮 Expected value is used to predict the average outcome of an experiment when repeated many times.
📊 Experimental probability is determined through conducting experiments and recording outcomes, which approximates the theoretical probability.
📈 Expected values and probability distributions help in making predictions and understanding the likelihood of various outcomes.

Q & A

What is the basic concept of probability?
-Probability is the measure of the likelihood of an event occurring, expressed as a real number between 0 and 1, where 0 indicates the event will not occur and 1 indicates absolute certainty of the event's occurrence.
What is an event in the context of probability?
-An event is a specific outcome or a combination of outcomes in a probabilistic scenario. It can be something like getting heads when flipping a coin or rolling a specific number on a die.
How are probabilities numerically expressed?
-Probabilities are numerically expressed as real numbers between 0 and 1, often in decimal form rather than percentages or fractions, to indicate the likelihood of an event occurring.
What does a probability of '1' signify?
-A probability of '1' signifies absolute certainty that the event will occur.
What is the meaning of a probability of '0'?
-A probability of '0' signifies absolute certainty that the event will not occur.
How is the probability of an event calculated?
-The probability of an event is calculated by dividing the number of favorable outcomes by the total number of possible outcomes.
What is the concept of expected value in probability?
-The expected value of an event is the average outcome we expect to occur if we run an experiment many times. It is calculated by multiplying each outcome by its probability and summing these products.
What is the difference between experimental and theoretical probabilities?
-Experimental probabilities are those derived from conducting experiments and recording outcomes, whereas theoretical probabilities are calculated based on the mathematical understanding of the event and its outcomes.
How do you calculate the probability of the complement of an event?
-The probability of the complement of an event is calculated by subtracting the probability of the event itself from 1.
What is a probability frequency distribution?
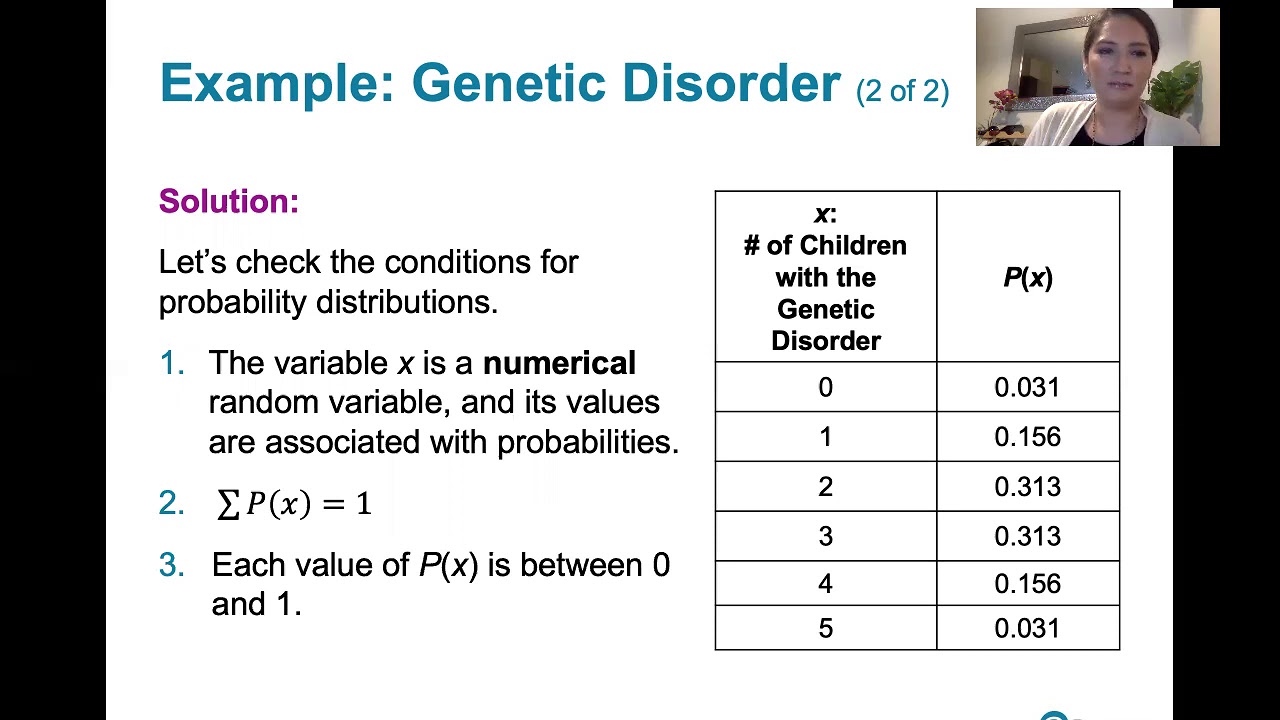
-A probability frequency distribution is a representation of the probabilities of each possible outcome in an experiment, often presented in a table or graph, showing how likely each outcome is.
Why is the sum of probabilities of all possible outcomes equal to 1?
-The sum of probabilities of all possible outcomes equals 1 because it represents certainty that one of the outcomes will occur, covering the entire sample space without any overlap.

Outlines

00:00

📊 Understanding Probability and Its Applications

The first paragraph introduces the concept of probability, its importance in decision-making, especially for CEOs in business scenarios like R&D investments or mergers. It defines probability as the likelihood of an event occurring and distinguishes between simple and complex events using coin flips and dice rolls as examples. The paragraph also explains how probabilities are numerically expressed, ranging from 0 to 1, with interpretations for these values. It further illustrates the calculation of probabilities by dividing the number of favorable outcomes by the total possible outcomes, using coin flipping and die rolling as examples again.

05:01

🎲 Probabilities of Combined Events and Expected Values

This paragraph delves into the probabilities of combined events, such as rolling a die and getting a number divisible by 3, and explains how to calculate these probabilities by considering the number of favorable outcomes over the total outcomes. It introduces the concept of independent events and how their probabilities can be multiplied together. The paragraph then shifts to discuss expected values, which predict the outcome of an event based on repeated trials, and differentiates between experimental and theoretical probabilities. It also explains how to calculate experimental probabilities and expected values for categorical and numerical outcomes, using card drawing and archery as examples.

10:02

🏹 Expected Values in Predictions and Complements of Events

The third paragraph continues the discussion on expected values, emphasizing their use in making predictions about future events, often presented as intervals due to inherent uncertainties. It uses weather forecasting as an example to illustrate this point. The paragraph also touches on the concept of numerical outcomes and how to calculate expected values by multiplying each outcome's value by its probability and summing these products. The importance of understanding the most probable outcomes and the use of probability frequency distribution is highlighted, along with an example of rolling two dice and the resulting sums.

15:02

📊 Probability Frequency Distribution and Complements

This paragraph introduces the concept of a probability frequency distribution, which shows the probabilities of each possible outcome for an event. It explains how to construct such a distribution using a table or graph and emphasizes the importance of the expected value in predicting the most likely outcomes. The paragraph also discusses the complement of an event, which represents all outcomes that the event is not, and explains how to calculate the probability of the complement by subtracting the event's probability from 1. It uses the example of rolling a die and getting an even number to illustrate the concept of complements.

20:03

🎯 Complements and the Sum of Probabilities

The final paragraph focuses on the characteristics of complements and the sum of probabilities. It explains that the sum of probabilities of all possible outcomes must equal 1, reflecting the certainty of all outcomes occurring. The paragraph discusses the implications of having a sum greater than or less than 1, indicating either double-counting of outcomes or the omission of some outcomes, respectively. It concludes with the concept that the complement of a complement returns to the original event, and uses the example of rolling a die to illustrate how the probability of not getting a certain number is calculated and relates to the sum of probabilities of other outcomes.

Mindmap

Keywords

💡Probability

Probability is the central theme of the video, defined as the likelihood or chance of an event occurring. It is expressed numerically as a real number between 0 and 1, where 0 indicates impossibility and 1 indicates certainty. The script uses probability to discuss how CEOs and others can make informed decisions by predicting outcomes. For example, the probability of getting heads when flipping a coin is 0.5, illustrating a 50/50 chance.

💡Event

In the context of the video, an event refers to a specific outcome or a combination of outcomes in a probabilistic scenario. It is a fundamental concept for understanding probability as it represents what we are measuring the likelihood of. The script gives examples such as getting heads in a coin flip, rolling a 4 on a die, which are events with specific probabilities.

💡Sample Space

The sample space encompasses all possible outcomes of an experiment. It is used to calculate probabilities, as the probability of an event is the ratio of the number of favorable outcomes to the total number of outcomes in the sample space. The script mentions the sample space when explaining how to calculate the probability of rolling a 4 on a six-sided die, which has 6 possible outcomes.

💡Favorable Outcomes

Favorable outcomes are those outcomes that satisfy the condition of interest in a probabilistic event. The script explains that the probability of an event is calculated by dividing the number of favorable outcomes by the total number of possible outcomes in the sample space, such as getting a head in a coin flip, which is one favorable outcome out of two possible outcomes.

💡Experimental Probability

Experimental probability is the probability determined through conducting experiments and observing the frequency of outcomes. It serves as an approximation of the theoretical probability when the true probability is unknown or difficult to calculate. The script contrasts experimental probability with theoretical probability, using the example of estimating the probability of getting heads in a coin flip by tossing the coin multiple times.

💡Expected Value

The expected value is the average outcome we expect from an experiment if it were repeated many times. It is calculated by multiplying each outcome by its probability and summing these products. The video uses expected value to predict outcomes, such as estimating how many times one might get a spade in 20 card draws, which has an expected value of 5 spades.

💡Complement

The complement of an event is everything that the event is not, effectively representing the remaining part of the sample space. The script explains that the probability of the complement is crucial for understanding the total probability of 1, as the sum of the probabilities of an event and its complement must equal 1, ensuring all possible outcomes are accounted for.

💡Independence

Independence refers to the property of two events where the occurrence of one does not affect the probability of the other. The script touches on this concept when discussing the probability of two independent events occurring simultaneously, which is the product of their individual probabilities, such as getting an Ace and a spade in a card draw.

💡Frequency Distribution

A frequency distribution is a way to organize and display the frequency of each outcome in a set of data. The script describes constructing a frequency distribution table for the sums of rolling two dice, which helps in understanding the most probable outcomes and their frequencies.

💡Theoretical Probability

Theoretical probability is the calculated probability of an event based on logical reasoning and knowledge of the sample space, without the need for experimentation. The video contrasts this with experimental probability, explaining that theoretical probability is often used when the outcomes and their probabilities can be precisely determined, such as the probability of rolling a 4 on a fair six-sided die, which is one-sixth.

💡Risk Management

Risk management, while not explicitly labeled as such in the script, is an underlying concept related to the use of probability in decision-making. The script discusses how CEOs use probability and statistical data to predict outcomes and make informed decisions, which is a fundamental aspect of risk management in business.

Highlights

Life is filled with uncertain events and the importance of considering possible outcomes before making decisions.

CEOs utilize probability and statistical data to make informed decisions in business strategies like R&D investments or mergers.

Probability is defined as the likelihood of an event occurring, with events being specific outcomes or combinations of outcomes.

Flipping a coin exemplifies a simple probability scenario with two possible outcomes: heads or tails.

Probabilities are numerically expressed using real numbers between 0 and 1, with 0 indicating impossibility and 1 indicating certainty.

The concept of 'sample space' represents all possible outcomes of an event.

The probability of an event is calculated as the ratio of preferred outcomes to the total number of possible outcomes.

Example given: the probability of rolling a 4 on a six-sided die is one-sixth, illustrating the calculation of single-outcome probabilities.

Complex events, such as rolling a number divisible by 3 on a die, involve multiple preferred outcomes.

The probability of independent events occurring simultaneously is found by multiplying the probabilities of the individual events.

Expected values are predictions of outcomes based on running an experiment multiple times, contrasting with theoretical probabilities.

Experimental probabilities are approximations derived from conducting experiments, such as estimating coin flip outcomes.

Expected values for categorical outcomes, like drawing a spade from a deck of cards, are calculated using theoretical probabilities.

For numerical outcomes, expected values are calculated by summing the products of each outcome's value and its probability.

Expected values help make predictions about the future based on past data, despite not guaranteeing exact outcomes.

Probability frequency distribution is a method to understand the likelihood of each possible outcome, visualized through graphs or tables.

The concept of 'complement' in probability theory refers to the opposite of an event, completing the rest of the sample space.

The sum of probabilities of all possible outcomes must equal 1, reflecting the certainty of at least one outcome occurring.

The probability of the complement of an event is calculated as 1 minus the probability of the event itself.

Transcripts

Browse More Related Video

Chapter 4 Probability Part 1

Discrete Random Variables

Chapter 4 Probability Part 2

Math 119 Chapter 4 Part 1

5.1.2 Discrete Probability Distributions - Probability Distributions and Probability Histograms

Statistics Lecture 4.2: Introduction to Probability

Related Tags

Probability Theory Decision Making Risk Assessment Statistical Data CEO Insights Event Likelihood Outcome Analysis Experimental Probabilities Expected Values Complement Events