The Law of Cosines
TLDRThe law of cosines relates the squares of the sides of any triangle to the cosine of the angle between two known sides to determine an unknown third side. While the law of sines solves for side-angle-angle, angle-side-angle and side-side-angle triangles, the law of cosines can solve side-angle-side triangles. The law of cosines can also solve side-side-side triangles by using the longest side to calculate an angle, then applying the law of sines. Both the laws of sines and cosines have straightforward applications for solving triangles.
Takeaways
- 😀 The law of cosines relates the squares of the side lengths of any triangle to the cosine of the angle between those sides.
- 📐 The law of cosines can be used to find the third side of a triangle when you know the other two sides and the angle between them.
- ➗ It allows you to solve side-angle-side triangles, unlike the law of sines.
- 🔢 You can also use the law of cosines to find an unknown angle between two known sides.
- 🤔 It works by setting up an equation equating the square of one side to the sum of the squares of the other sides, minus two times one side times the other side times the cosine of the included angle.
- 📒 You can use both the law of sines and law of cosines to solve triangles if you know all three side lengths.
- 🔭 First use the law of cosines to find one angle, then use the law of sines to find another angle.
- ❓ Subtract those angles from 180 degrees to get the third angle and fully solve the triangle.
- ✅ The law of cosines, combined with the law of sines, allows you to solve any triangle with three known quantities - sides or angles.
- 👍 Both laws provide straightforward methods to find missing sides and angles in oblique triangles.
Q & A
What is the law of cosines and how is it different from the Pythagorean theorem?
-The law of cosines relates the lengths of the sides of any triangle to the cosine of one of its angles. Unlike the Pythagorean theorem, it can be used for non-right triangles.
What information do you need to use the law of cosines to find a missing side length?
-You need to know the lengths of two of the sides and the measure of the angle between those sides.
How can you use the law of cosines to find a missing angle in a triangle if you know all three side lengths?
-You can plug the three known side lengths into the law of cosines formula and solve for the cosine of one of the angles. Then take the inverse cosine to find the measure of that angle.
What kind of triangles can be solved using only the law of sines?
-The law of sines alone can be used to solve side-angle-angle, angle-side-angle, and side-side-angle triangles.
What does the law of cosines allow us to solve that the law of sines does not?
-The law of cosines allows us to solve side-angle-side triangles, which cannot be solved using only the law of sines.
If you know two angles of a triangle, can you use the law of cosines to find the third angle?
-No, the law of cosines requires knowing at least two side lengths and the included angle between those sides. It does not work with only angle measurements.
What is the easiest way to find an angle using the law of cosines if you know all three side lengths?
-Solve for the angle opposite the longest side by plugging the known sides into the law of cosines formula and solving for the cosine of that angle.
After using the law of cosines, how can you find the remaining angles of a triangle?
-After finding one angle with the law of cosines, you can use the law of sines to find a second angle. Then subtract those two angles from 180 degrees to get the measure of the third angle.
Why is the law of cosines useful in triangle trigonometry?
-The law of cosines allows us to solve a broader range of triangles than the Pythagorean theorem or law of sines alone. It is an important tool for finding missing sides and angles.
What other information can you calculate once you have found the three angles of a triangle using the laws of sines and cosines?
-Once you know all three angles, you can use them to calculate the area of the triangle or find the lengths of any remaining unknown sides.
Outlines
😀 Law of Cosines Formula and Usage
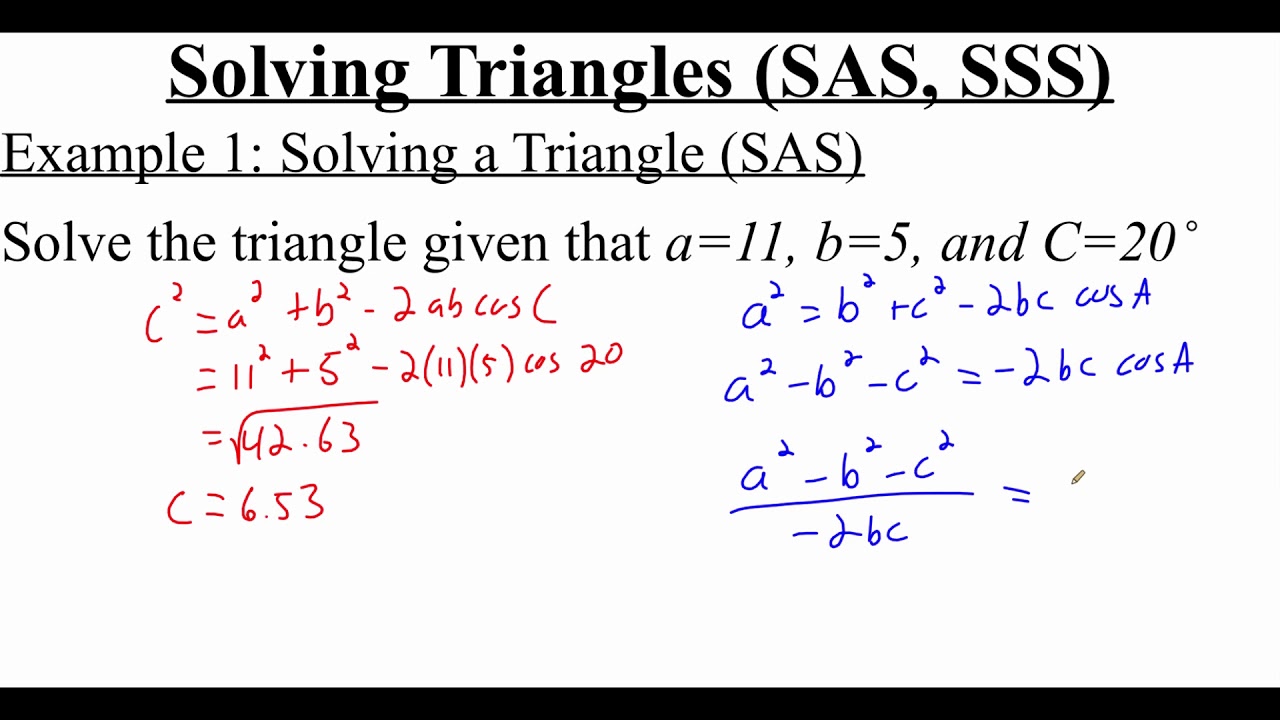
This paragraph introduces the law of cosines, which relates the squares of the side lengths of any triangle to the cosine of one of its angles. It can be used to find missing side lengths or angles. The formula is provided and an example is shown of using the law of cosines to find the third side of a triangle when two sides and the included angle are known.
😃 Solving Triangles with the Law of Cosines
This paragraph demonstrates using the law of cosines to solve two types of triangles - side-angle-side triangles and side-side-side triangles. An example solves for a missing side length given two known sides and the included angle. Another example solves for a missing angle given all three side lengths, by plugging the values into the law of cosines formula.
😊 Combining Laws of Sines and Cosines
This final paragraph notes that the laws of sines and cosines can be combined to fully solve triangles. Once one missing side or angle is found via the law of cosines, the law of sines can then be used to find any remaining missing pieces of information.
Mindmap
Keywords
💡law of cosines
💡Pythagorean theorem
💡oblique triangle
💡side angle side triangle
💡side side side triangle
💡solve
💡law of sines
💡opposite
💡solve for
💡application
Highlights
The law of cosines relates the squares of the lengths of a triangle's sides for any triangle, not just right triangles like the Pythagorean theorem.
The law of cosines allows you to calculate the third side of a triangle if you know the lengths of two sides and the angle between them.
The law of cosines can be used to solve side-angle-side triangles, unlike the law of sines which requires different information.
The law of cosines allows you to calculate an unknown angle if you know all three side lengths of a triangle.
To use the law of cosines to find an unknown angle, solve for the angle opposite the longest side.
The law of cosines and law of sines can be used together to fully solve an oblique triangle when only some information is known initially.
The law of cosines relates the square of one side length to the squares of the other two side lengths and the cosine of the angle between those two sides.
An example shows step-by-step how to use the law of cosines to find a missing third side length when two side lengths and the included angle are known.
After finding one angle with the law of cosines, the law of sines can be used to find a second angle, and then subtracting the known angles from 180 degrees gives the third angle.
The law of cosines can also be rearranged to directly calculate an unknown angle between two known sides.
An example shows step-by-step how to use the law of cosines to calculate an unknown angle given all three side lengths.
Unlike the law of sines, the law of cosines does not require a corresponding side and angle - any two sides and their included angle can be used.
Both the law of sines and law of cosines provide straightforward ways to solve oblique triangles.
Together, the law of sines and law of cosines can be used to solve any oblique triangle, no matter what information is initially known.
Transcripts
Browse More Related Video
The Law of Sines

How to Use the Law of Cosines in Trigonometry (Precalculus - Trigonometry 33)
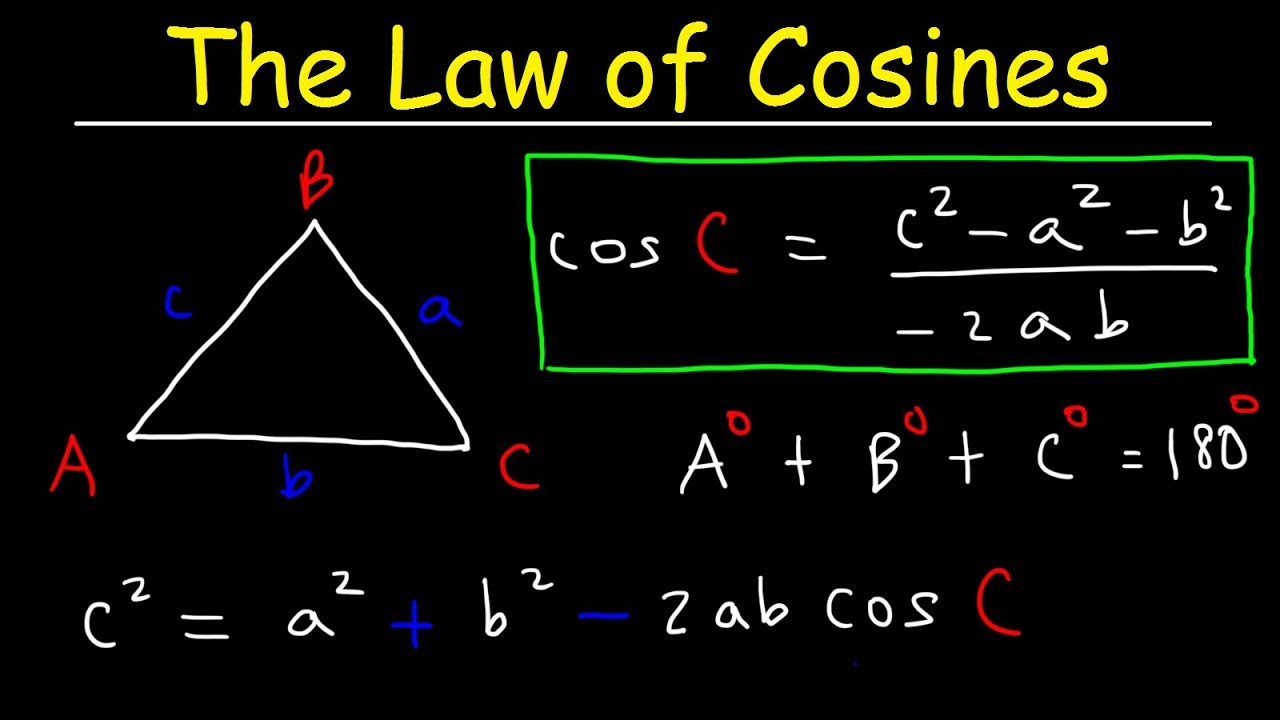
Law of Cosines, Finding Angles & Sides, SSS & SAS Triangles - Trigonometry
How to Use the Law of Sines in Trigonometry (Precalculus - Trigonometry 32)
Law of Sines, Basic Introduction, AAS & SSA - One Solution, Two Solutions vs No Solution, Trigonomet
5.6.1 Law of Cosines: Solving Triangles (SAS, SSS)
5.0 / 5 (0 votes)
Thanks for rating: