How to Use the Law of Cosines in Trigonometry (Precalculus - Trigonometry 33)
TLDRThis video delves into the Law of Cosines, a fundamental principle for solving triangles when the Law of Sines is inapplicable. The presenter explains that the Law of Cosines is especially useful for oblique triangles, where it can determine a missing side when two sides and the included angle are known, or calculate a missing angle when all three sides are known. The video clarifies why the Law of Sines fails in certain cases, emphasizing the straightforward nature of the Law of Cosines, which doesn't require dealing with the ambiguity of two potential solutions. The script provides a step-by-step guide on how to apply the Law of Cosines, including rearrangements of the formula for different scenarios. It also touches on the special case where the Law of Cosines intersects with the Pythagorean theorem. Practical examples, including a real-life scenario of a pilot correcting a flight path, are used to illustrate the application of the Law of Cosines. The video concludes with a teaser for the next topic: calculating the area of a triangle using the Law of Cosines.
Takeaways
- 📐 **Law of Cosines Application**: The law of cosines is used to solve oblique triangles when the law of sines is not applicable, particularly in cases involving two sides and the angle between them or when all three sides are known.
- ❌ **Limitations of Law of Sines**: The law of sines cannot be used in cases where an angle is not between the two known sides, which limits its application compared to the law of cosines.
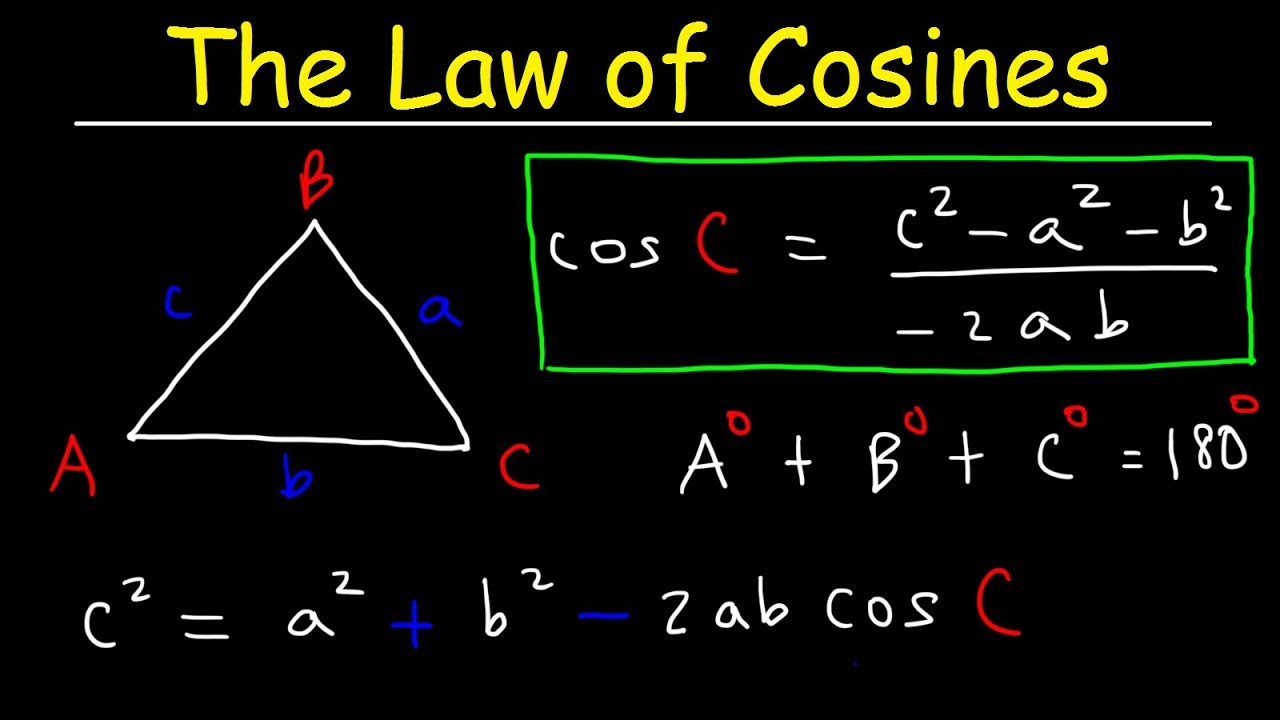
- 🔍 **Law of Cosines Formula**: The formula for the law of cosines is `a² = b² + c² - 2bc * cos(A)`, where `a`, `b`, and `c` are the sides of the triangle and `A` is the angle opposite side `a`.
- 👉 **Finding Missing Sides**: When you know two sides and the included angle, the law of cosines can be used to find the length of the third side.
- 🧮 **Solving for Angles**: Once all three sides of a triangle are known, the law of cosines can be used to find an unknown angle by rearranging the formula to solve for cosine of the angle.
- 🔢 **Avoiding Rounding Errors**: It's important to avoid rounding numbers until the final step to prevent compounding errors in calculations.
- 📉 **Law of Sines Ambiguity**: When using the law of sines, there can be two possible angles for a given side and angle, which requires additional checking to ensure the correct solution is chosen.
- 🎯 **Accuracy with Law of Cosines**: The law of cosines often provides a more straightforward and accurate method for solving triangles, especially when avoiding the need to deal with the ambiguity of the law of sines.
- 🛫 **Real-world Application**: The law of cosines can be applied to real-world scenarios, such as calculating course corrections and speeds for navigation.
- 🔄 **Checking Work**: Using the law of cosines to find one angle and then using the interior angle sum to find another can serve as a check for the accuracy of your work.
- 🚫 **Pythagorean Theorem Limitation**: The Pythagorean theorem cannot be used if the triangle is not a right triangle, emphasizing the importance of the law of cosines for all types of triangles.
Q & A
What is the Law of Cosines and when is it used?
-The Law of Cosines is a formula used in geometry to find a side of a triangle when two sides and the angle between them are known. It is also used when all three sides of a triangle are known to find the angles. It's particularly useful for oblique triangles where the Law of Sines does not apply.
How does the Law of Cosines formula look like?
-The Law of Cosines formula is expressed as: c² = a² + b² - 2ab * cos(γ), where c is the side opposite the angle γ, and a and b are the other two sides of the triangle.
Why doesn't the Law of Sines work for some oblique triangles?
-The Law of Sines does not work for oblique triangles when the angle given is not between the two known sides (case 3 and 4 as mentioned in the script). It requires the angle to be between the two sides for which you have length measurements.
What happens if the angle in the Law of Cosines formula is 90 degrees?
-If the angle is 90 degrees, the cosine of the angle is zero. The Law of Cosines formula then simplifies to a² + b² = c², which is the Pythagorean theorem. This means the Law of Cosines is a generalization of the Pythagorean theorem.
How can you find the angles of a triangle if you know all three sides?
-If you know all three sides of a triangle, you can use the Law of Cosines to find each angle. You would set up an equation for each angle using the Law of Cosines and then take the inverse cosine (cos⁻¹) of the resulting expression to find the measure of the angle.
What is the relationship between the Law of Sines and the Law of Cosines when it comes to solving for angles?
-The Law of Sines is used when you have one angle and its opposite side known, or two sides and an angle not between those sides known. The Law of Cosines is used when you have two sides and the included angle known or all three sides known to find an angle. They are both trigonometric tools, but they apply to different scenarios in triangle solving.
Why is it important to avoid rounding numbers when using the Law of Cosines or Law of Sines?
-Rounding numbers in the Law of Cosines or Law of Sines can introduce approximation errors into the calculations. These errors can compound, especially when using inverse trigonometric functions, leading to less accurate results for the angles or sides of the triangle.
How can you use the Law of Sines to find an angle when you have the largest angle and the largest side of a triangle?
-You can use the Law of Sines to find an angle opposite the smallest side when you know the largest angle and the largest side. Since the smallest side is opposite an acute angle, there will be no ambiguity in the solution (i.e., no need to consider 180 minus the angle), thus avoiding the two solutions issue that can arise with the Law of Sines.
What is the application of the Law of Cosines in real-life scenarios, such as navigation?
-The Law of Cosines can be used in navigation to find the distance to a destination or to calculate the necessary course correction when off track. For instance, if a pilot realizes they have been off course and knows the speed and time flown, the Law of Cosines can help find out how far off course they are and what correction is needed.
How does the Law of Cosines help in finding the remaining angles of a triangle after finding one angle?
-After finding one angle using the Law of Cosines or Law of Sines, you can use the Interior Angle Sum property of triangles (which states that the sum of angles in a triangle is 180 degrees) to find the remaining angles. Alternatively, you can continue to use the Law of Cosines to find each angle one by one.
What is the main takeaway from the video regarding the use of the Law of Cosines and Law of Sines?
-The main takeaway is that the Law of Cosines is a versatile tool for solving triangles when you know two sides and the included angle, or all three sides to find the angles. It is often preferred over the Law of Sines to avoid dealing with the ambiguity of two potential solutions for an angle and the potential for rounding errors. The video emphasizes the importance of understanding when and how to apply each law for accurate triangle solving.
Outlines
📚 Introduction to Law of Cosines
The video begins with an introduction to the Law of Cosines, which is used to solve oblique triangles when the Law of Sines is inapplicable. The presenter explains that the Law of Cosines is more straightforward and can be used in two scenarios: when two sides and the included angle are known, or when all three sides of the triangle are known. The formula for the Law of Cosines is introduced, and the presenter emphasizes its utility in finding missing sides or angles in a triangle.
🔍 Law of Cosines vs. Law of Sines
The presenter contrasts the Law of Cosines with the Law of Sines, explaining why the latter does not apply in cases three and four of oblique triangles. The video illustrates that the Law of Cosines is the appropriate tool when the angle is between the two known sides, and it can also be rearranged to solve for different sides or angles. The special case when an angle is 90 degrees, turning the Law of Cosines into the Pythagorean theorem, is also discussed.
🧮 Applying Law of Cosines - Example 1
An example is provided to demonstrate the application of the Law of Cosines. The presenter labels the triangle's sides and angles and chooses to find the side opposite the known angle first. By applying the Law of Cosines formula, the missing side is calculated. The video then shows how to find the remaining angles using either the Law of Sines or the Law of Cosines, emphasizing the importance of accurate calculations and avoiding rounding errors.
🔢 Law of Cosines in Real-life Scenarios
The Law of Cosines is applied to a real-life scenario where a pilot flying from City A to City B discovers an off-course deviation. The presenter uses the Law of Cosines to calculate the necessary course correction and the speed adjustment needed to reach City B within a specific timeframe. This practical application shows the utility of the Law of Cosines in navigation and problem-solving.
🤔 Choosing Between Law of Sines and Cosines
The presenter discusses the decision process for choosing between the Law of Sines and the Law of Cosines. They advise that while the Law of Sines can be used if one angle and its opposite side are known, the Law of Cosines is often preferred due to its precision and the avoidance of ambiguity. The video also covers the use of interior angle sum for finding angles once a side has been determined using the Law of Cosines.
🛫 Final Application and Summary
The video concludes with a summary of the Law of Cosines and its applications. The presenter reiterates the importance of using the Law of Cosines for precision, especially when dealing with rounded numbers. They also provide a final application example, reinforcing the concepts learned throughout the video and encouraging viewers to practice using the Law of Cosines for various triangle problems.
Mindmap
Keywords
💡Law of Cosines
💡Law of Sines
💡Oblique Triangles
💡Trigonometry
💡Pythagorean Theorem
💡Angle of Inclusion
💡Hypotenuse
💡Interior Angle Sum
💡Cosine
💡Sine
Highlights
Introduction to the Law of Cosines, a formula used for solving oblique triangles when the Law of Sines is inapplicable.
Explanation of why the Law of Sines fails in certain cases, specifically when dealing with side-side-angle configurations.
The Law of Cosines is applicable when you know two sides and the included angle, or all three sides of a triangle.
Derivation and explanation of the Law of Cosines formula, which involves squaring sides and using cosine of the included angle.
Illustration of how to apply the Law of Cosines formula to find a missing side of a triangle.
Clarification that the Law of Cosines simplifies to the Pythagorean theorem when one of the angles is 90 degrees.
Method to find the remaining angles of a triangle once all sides are known, using the Law of Cosines.
Strategy for solving for an angle when two sides and the angle between them are known, using the Law of Cosines.
Discussion on the limitations of using the Law of Sines and when it is not applicable, such as when an angle is not between two known sides.
Demonstration of how to label a triangle for easier application of the Law of Cosines and solving for missing parts.
Use of the Law of Sines to find an angle after determining all side lengths, avoiding the need for multiple Law of Cosines applications.
Real-world application of the Law of Cosines in a scenario involving an airplane's course correction.
Calculation of the required speed adjustment for the airplane to reach its destination within a specific timeframe.
Explanation of how to avoid rounding errors when using trigonometric functions like sine and cosine by plugging in exact expressions.
Comparison of the accuracy between using the Law of Sines and the Law of Cosines, emphasizing the importance of exact calculations.
Advice on using the Law of Cosines for checking work due to its straightforward nature and lack of multiple solution ambiguities.
Summary of when to use the Law of Sines versus the Law of Cosines based on the information available and the need for accuracy.
Transcripts
Browse More Related Video
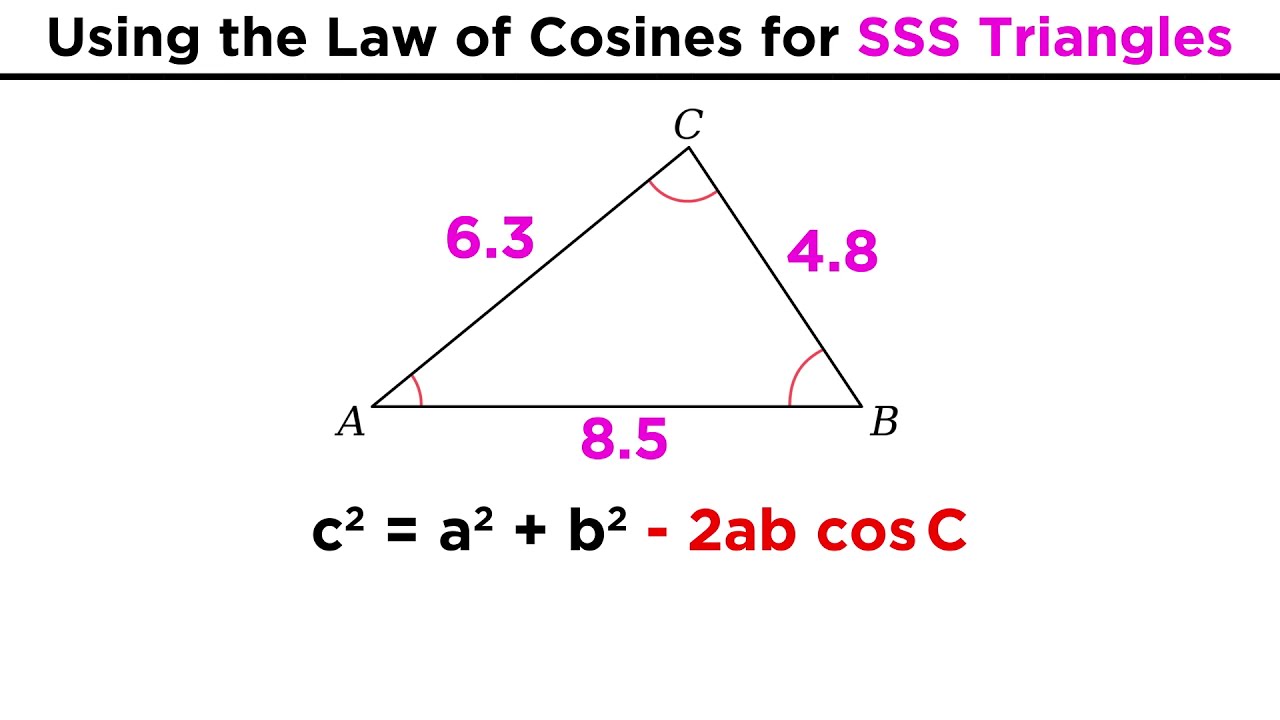
The Law of Cosines
Law of Cosines, Finding Angles & Sides, SSS & SAS Triangles - Trigonometry
How to Use the Law of Sines in Trigonometry (Precalculus - Trigonometry 32)
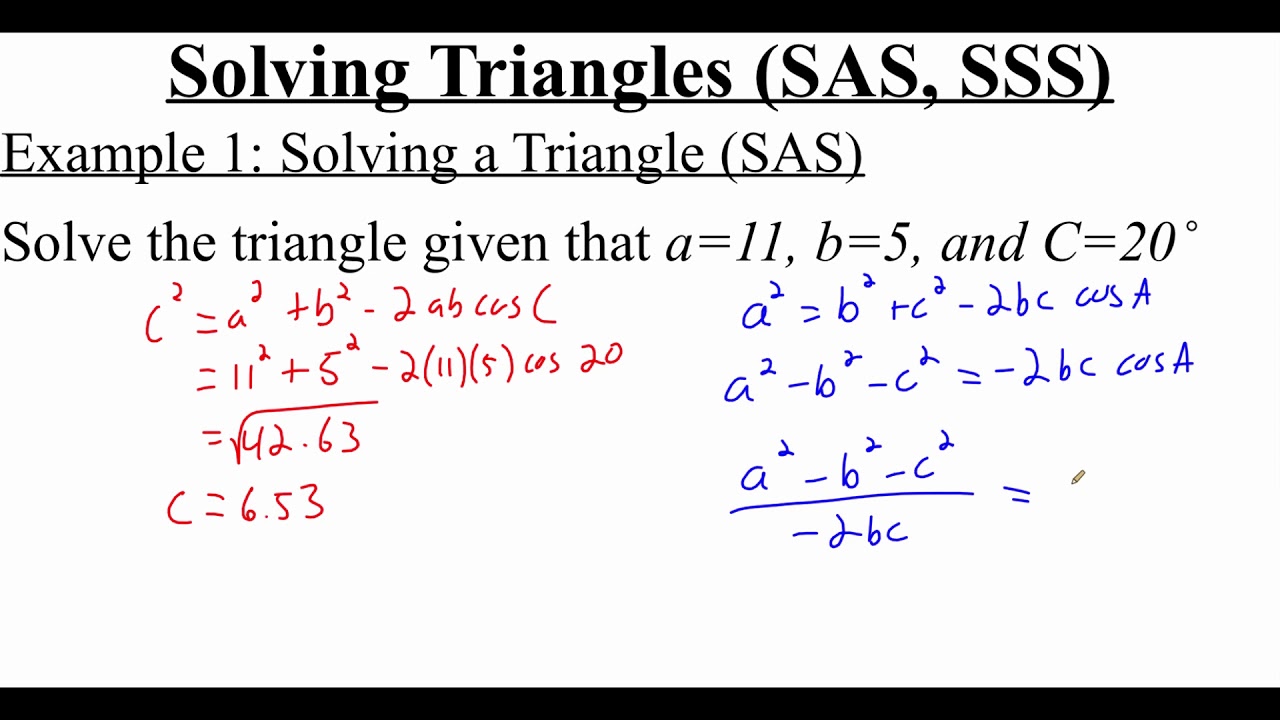
5.6.1 Law of Cosines: Solving Triangles (SAS, SSS)
How To Calculate The Missing Angle In a Triangle

How To Calculate The Missing Side Length of a Triangle
5.0 / 5 (0 votes)
Thanks for rating: