Mean, Median, and Mode: Measures of Central Tendency: Crash Course Statistics #3

CrashCourse
7 Feb 201811:23
EducationalLearning
32 Likes 10 Comments

TLDRThis video explores measures of central tendency - mean, median, and mode - which describe the center or typical value of a dataset. It explains how to calculate these values, noting that the mean uses all data points while the median focuses on the middle value. The mode is the most frequent value. The relationship between these measures indicates the shape and skew of the data distribution. The video stresses that while statistics like averages can precisely describe data, they can also mislead, so we must consider the questions we want to answer and use common sense when interpreting statistics.

Takeaways
  • 😀 Measures of central tendency like mean, median and mode describe the middle or average of a dataset and provide a useful summary.
  • 📊 The mean or average is calculated by summing all data points and dividing by the number of data points.
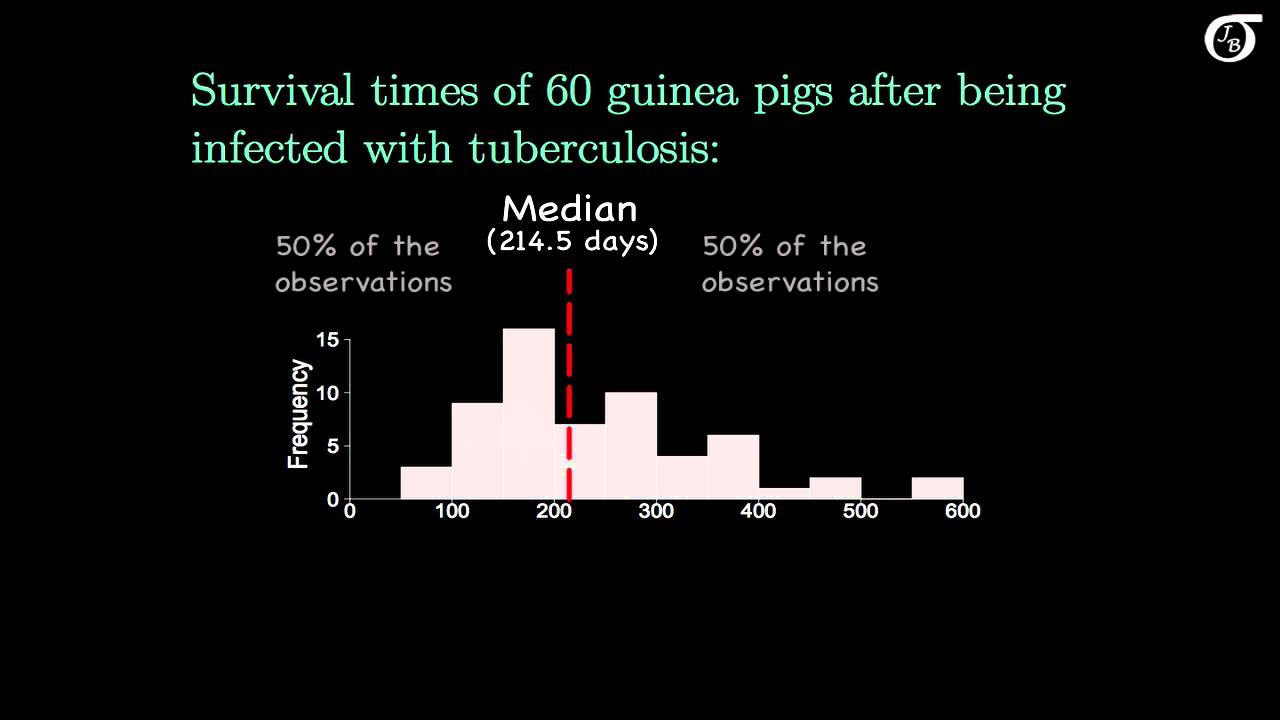
  • 🎯 The median is the middle number when data points are arranged from lowest to highest.
  • 🔢 The mode is the value that occurs most frequently in a dataset.
  • 🤔 The mean can be misleading if there are outliers that skew the data, while median is more robust.
  • ❗ The relationship between mean, median and mode reveals information about the shape and distribution of data.
  • 💰 Statistics like average income can seem positive while hiding a falling median income.
  • 😕 Statistics can be true but also misleading if not considering the right questions.
  • 📈 Normal distributions have mean, median and mode all equal with symmetric bell shape.
  • ↔ Skewed distributions have unequal mean and median due to extreme values on one side.
Q & A

  • What is a measure of central tendency and why is it useful?

    -A measure of central tendency summarizes the center or middle of a dataset. It is useful for getting a sense of a typical value or an overview of the data.

  • What are the three main measures of central tendency discussed?

    -The three main measures discussed are the mean or average, the median, and the mode.

  • How is the mean calculated and what does it tell you?

    -The mean is calculated by adding up all the values and dividing by the number of values. It tells you the average value but can be misleading if there are extreme outliers.

  • What is the median and when would you use it instead of the mean?

    -The median is the middle number when a dataset is ordered from smallest to largest. It is less influenced by outliers so is used over the mean when there are extreme values that skew the distribution.

  • What does a normal distribution look like and why does it matter?

    -A normal distribution is symmetric and bell-shaped, with the mean, median and mode all being equal. This tells you there is an even spread of data with no skew.

  • What is bimodal and multimodal data?

    -Bimodal data has two peaks or most common values. Multimodal data has more than one peak - indicating multiple underlying groups in the full dataset.

  • What does skew tell you about a distribution?

    -Skew describes if a distribution leans one way or another, based on extreme high or low values. If the mean and median differ significantly, the distribution is skewed.

  • Why can statistics be simultaneously true and deceptive?

    -Statistics reflect an aggregate view of data. An average can go up while the median goes down, hiding the real situation for most data points. You have to look deeper to avoid deception.

  • What is the mode and when is it most useful?

    -The mode is the most frequent value in a dataset. It is useful when you have a large sample size to identify the most popular response.

  • How can understanding measures of central tendency help us make decisions?

    -By summarizing the typical or average response, measures of central tendency help us set expectations and make estimates or guesses about future data based on past trends.

Outlines
00:00
📺 Introducing Statistics Crash Course

The host Adriene Hill welcomes viewers to Crash Course Statistics. She refers to previous discussions of large and small numbers and says they will now talk about middle, average numbers like means, medians and modes. These are important for understanding data even if less exciting. The average number of feet per person is used as an silly example.

05:01
📊 Understanding Measures of Central Tendency

The mean, median and mode are explained as measures of central tendency that describe the center or middle of a data set. Examples are used to demonstrate how they are calculated. Limitations are noted, like how the mean can be misleading if data is skewed by outliers. The median is introduced as an alternative. A normal distribution is defined in relation to central tendency.

10:02
😿 Interpreting Statistics Thoughtfully

An example explores how average income rose from 2010-2013 while median income fell. This shows how statistics can be true but misleading. Understanding context and asking probing questions is important. Statistics inform decisions but should be met with healthy skepticism.

Mindmap
Keywords
💡Mean
The mean, or average, is a measure of central tendency calculated by summing all data points and dividing by the number of data points. It gives an indication of the middle or expected value. In the video it's used in examples like the average number of puppies per litter and the average income at a dinner party before and after Elon Musk arrives.
💡Median
The median is the middle number or value when the data is arranged in numerical order. It is less influenced by outliers or extreme values. In the video, median is used in examples like finding the middle number of cats owned when a few people own over a dozen cats.
💡Mode
The mode refers to the value that occurs most frequently in a dataset. In the video it's used to analyze the reviews for a controversial book that had bimodal distributions of 1-star and 5-star reviews.
💡Normal distribution
A normal distribution, also called Gaussian distribution, is a symmetrical bell-shaped pattern where values cluster around the mean/average. The video explains how in a normal distribution, the mean, median and mode converge to the same central value.
💡Skew
Skew refers to an asymmetric distribution where extreme values pull the mean away from the median and mode. Positive skew has extreme high values while negative skew has extreme low values. This indicates the data is not normally distributed.
💡Outlier
Outliers are data points that fall a long way outside the overall pattern of a distribution. In the example with macrame artists, Elon Musk's income is an outlier that greatly shifts the mean even though the median stays the same.
💡Frequency distribution
A frequency distribution shows how often each value in a dataset occurs, which helps visualizepatterns. This is useful for seeing things like bimodal distributions with peaks around lunchtime and dinnertime customers.
💡Descriptive statistics
Measures of central tendency like mean, median and mode are descriptive statistics that summarize large datasets with single representative values. But while descriptive statistics can reveal useful information, they don't tell the whole story and can sometimes be misleading if interpreted incorrectly.
💡Infant mortality
The video explains how high infant mortality rates in the Middle Ages skewed life expectancy figures, since many people still lived into their 50s and 60s. This demonstrates how averages can misrepresent reality if subgroups within data are not considered.
💡Misleading statistics
While statistics like averages can precisely quantify information, they can also mislead if underlying contexts and interpretations are ignored. The video advises using common sense and skepticism when working with statistics to avoid deception.
Highlights

Measures of central tendency summarize data and give a good example of the whole data set

The mean, median, and mode are common measures of central tendency referred to as averages

The mean is calculated by summing all data points and dividing by the number of data points

The median is the middle number when data is ordered from smallest to largest

The mode is the value that appears most frequently in a data set

The mean can be distorted by outliers, but the median is more robust

Skewed distributions have extreme values that pull the mean away from the median and mode

Normal distributions have the mean, median, and mode all equal with no skew

Statistics can be true but still misleading if misinterpreted or misrepresented

Understanding the right questions is key to properly interpreting statistics

Using common sense and skepticism allows proper interpretation of statistics

The relationship between mean, median, and mode gives insight into the data distribution

The mean income rose while the median income fell after the 2008 financial crisis, showing the distribution was skewed by high top incomes

Bimodal distributions have two modes from two underlying groups in the data set

The mode is most useful for categorical data like favorite color where mean and median don't apply

Transcripts

Browse More Related Video

Analyzing Sets of Data: Range, Mean, Median, and Mode

Mean, Median and Mode - Measures of Central Tendency

Finding mean, median, and mode | Descriptive statistics | Probability and Statistics | Khan Academy

An Average Video | Mean, Median, Mode, and Range

Measures of Central Tendency

Mean, Median and Mode in Statistics | Statistics Tutorial | MarinStatsLectures

Rate This

5.0 / 5 (0 votes)

Thanks for rating: