Geometric Distributions and The Birthday Paradox: Crash Course Statistics #16

CrashCourse
16 May 201810:19
EducationalLearning
32 Likes 10 Comments

TLDRThis video explains how geometric probability distributions can be used to calculate the likelihood of an event occurring after a certain number of trials. It provides the formula for geometric probability and walks through examples like guessing how many jelly beans you can eat before getting a vomit flavor. The script also covers the birthday paradox and how probabilities can help make decisions, like whether to buy Pokemon cards based on the chance of getting a certain card. Overall, it explores how probabilities assign numbers to intuition and allow us to quantify waiting times.

Takeaways
  • 😊 Probabilities help estimate wait times for events like getting certain poker hands or winning the lottery.
  • 😮 The geometric probability formula calculates the likelihood of an event happening on the nth attempt.
  • 🤔 A geometric distribution graph shows the probability of each attempt being the first success.
  • 🧐 You can calculate the probability of a success within a certain number of tries using a cumulative distribution.
  • 😲 The geometric distribution's mean tells the average tries needed before a success.
  • 😃 Cumulative probabilities help decide if something is worth the wait, like buying Pokemon cards for a rare card.
  • 😯 The birthday paradox shows a counterintutive result - in a group as small as 23 people, there's over 50% chance two share a birthday.
  • 🤓 Probabilities quantify intuitions about likelihood and wait times.
  • 😀 Small probabilities can equate to very long wait times, like winning the lottery.
  • 🥳 Probabilities help make informed decisions about what's worth your time and money.
Q & A

  • What formula can be used to calculate the probability of something happening on the nth try?

    -The Geometric Probability Formula can calculate the probability of an event happening on the nth try. It calculates the probability of the kth try being the first success.

  • How does the geometric probability distribution differ from the binomial distribution?

    -The geometric probability distribution calculates the probability that the kth try will be the first success. The binomial distribution calculates the probability of a certain number of successes out of a given number of trials.

  • What does the mean of a geometric distribution represent?

    -The mean of a geometric distribution represents the average number of trials needed to achieve the first success.

  • What is the birthday paradox?

    -The birthday paradox explores the counterintuitive probability that even small groups of people are likely to have two members who share the same birthday. With just 70 people, there is a 99.9% chance of a shared birthday.

  • How can cumulative probabilities of a geometric distribution be useful?

    -The cumulative probabilities of a geometric distribution allow you to calculate the likelihood that success is achieved by the nth trial or earlier. This is helpful for deciding if something is worth waiting for.

  • What influences the mean number of trials in a geometric distribution?

    -The lower the probability of success, the higher the average number of trials will be before achieving that first success. So the mean depends on the likelihood of the event occurring.

  • How did probabilities help quantify things that were previously just intuition?

    -Probabilities take intuitive feelings and common sense notions of likelihood and assign numerical values to them. This allows for precise calculations rather than just gut feelings.

  • What everyday decisions could probabilities help inform?

    -Probabilities could help decide things like whether it's worth spending time/money trying to win a prize from a crane machine based on the likelihood of success before your food order arrives.

  • What is an example application of geometric probabilities from the script?

    -The script examines the probability of pulling a rare Pikachu Pokemon card from a pack to help decide between buying more card packs or a Star Wars Blu-Ray movie instead.

  • How unlikely is it that you would win the California lottery?

    -On average, you would expect to have to play over 15 million times before winning the California lottery, making it extremely unlikely to win.

Outlines
00:00
🍬 Probability of Getting Gross Jelly Beans Before a Good Flavor

This paragraph discusses Bertie Bott's Every Flavor Jelly Beans, which come in good and bad flavors. It introduces the geometric probability formula, which calculates the probability of a certain outcome happening on the nth trial. This is applied to find the chance of getting 4 good jelly beans before encountering a vomit-flavored one, assuming the probability of vomit flavor is 5%.

05:01
🏀 Probability of Making a Free Throw within 10 Tries

This paragraph provides another example using geometric probability. It calculates the chance of making 1 free throw within 10 tries, assuming a 20% probability of making each shot. It also shows how to find the average number of shots needed using the geometric distribution's mean.

Mindmap
Keywords
💡probability
The video focuses a lot on probability, which is the likelihood that an event will occur. Probabilities are numbers between 0 and 1 that quantify uncertainty. The video discusses different probability formulas like the geometric probability formula, which calculates the chance that an event happens on a certain attempt.
💡distribution
A probability distribution shows all the possible outcomes of a probabilistic event and the probabilities associated with each outcome. The video introduces the geometric distribution, which gives the probability that a certain trial is the first time a success occurs.
💡mean
The mean, or average, gives a central value that summarizes a distribution. For the geometric distribution, the mean tells us the average number of trials needed to get a first success.
💡cumulative
A cumulative distribution sums up the probabilities as you move along the distribution. The video uses a cumulative geometric distribution to find the chance of getting a success by a certain trial.
💡probability formula
Formulas in probability theory let you calculate chances, averages, and other useful values. The geometric probability formula introduced in the video computes the probability that the kth trial is the first success.
💡success
In probability, a success represents the outcome you are interested in or waiting for. It does not necessarily mean something good - in the jellybean example, success was finding a vomit flavored bean.
💡Bayes' theorem
Bayes' theorem, named after Reverend Thomas Bayes, flips conditional probability around to do reverse inference. It allows you to compute the probability of A given B, if you know the probability of B given A. Bayes theorem is not directly mentioned but some examples use its logic.
💡expected value
Expected value is the average value you would expect if you repeated an experiment many times. Although not called out by name, the video discusses expected values like the mean number of trials to success in the geometric distribution.
💡likelihood
Likelihood measures how probable something is. It is often used informally as a synonym for probability. The narrator refers to using probability to guess or estimate the likelihood of various events.
💡birthday problem
The birthday problem (or paradox) asks for the probability that two people in a group share the same birthday. Despite intuition suggesting otherwise, the probability is surprisingly high - with just 23 people there's a 50-50 chance of a shared birthday.
Highlights

Probabilities can help you guess how long it might take for something to happen, like getting a full house in poker, having your first daughter, or winning the lottery.

Geometric probabilities tell you the probability that your first success will be on your nth try.

The Geometric Probability Formula says that the probability of the kth try being your first success, is the probability of failure to the k-1 power, times the probability of success to the first power.

As k increases, the probability of that being your first success gets incredibly low, mostly because you’ll probably have found the flavor that shall not be named already.

The mean number of shots we’d need to take before we made our first would be 1/0.2, which is 1/(1/5) or 5. And this makes some sense. The smaller the probability of success is, the more tries we’ll need--on average--before we get a success.

The mean and cumulative frequency of the geometric distribution can help you weigh your options.

There are 365 birthdays that anyone can have, we’re not going to count leap days-- to keep things simple. So let’s say there are 20 people in your classroom. What’s the likelihood that there would be any shared birthdays?

Once you get up to a group of 70 there’s a 99.9% chance of someone will be sharing a birthday with someone else.

Probabilities are important. They’re things we use all the time.

Geometric probabilities, and probabilities in general, allow you to guess how long you’ll have to wait for something, so you can decide whether it’s worth it.

Probability “is basically just common sense reduced to calculus; it makes one appreciate with exactness that which accurate minds feel with a sort of instinct, often without being able to account for it.” In other words, it allows us to quantify things we already feel.

Probability assigns numbers to common sense. And we all need a little more of that.

You can use them to figure out that on average, you’ll probably have to wait a looooong time before you win the California State Lottery , like a really long time, on average it should take you about 15 million tries. That’s a lot o’ lotto tickets.

When you’re at your local diner and see those super cool Crane machines, you might estimate the probability that you can snag that sweet stuffed otter by the time your food arrives and whether it’s worth your time and money to even try.

This is one of those instances where statistics trumps my own intuition. And a good reason to be prepared for the possibility of double cupcakes if you have a big class.

Transcripts

Browse More Related Video

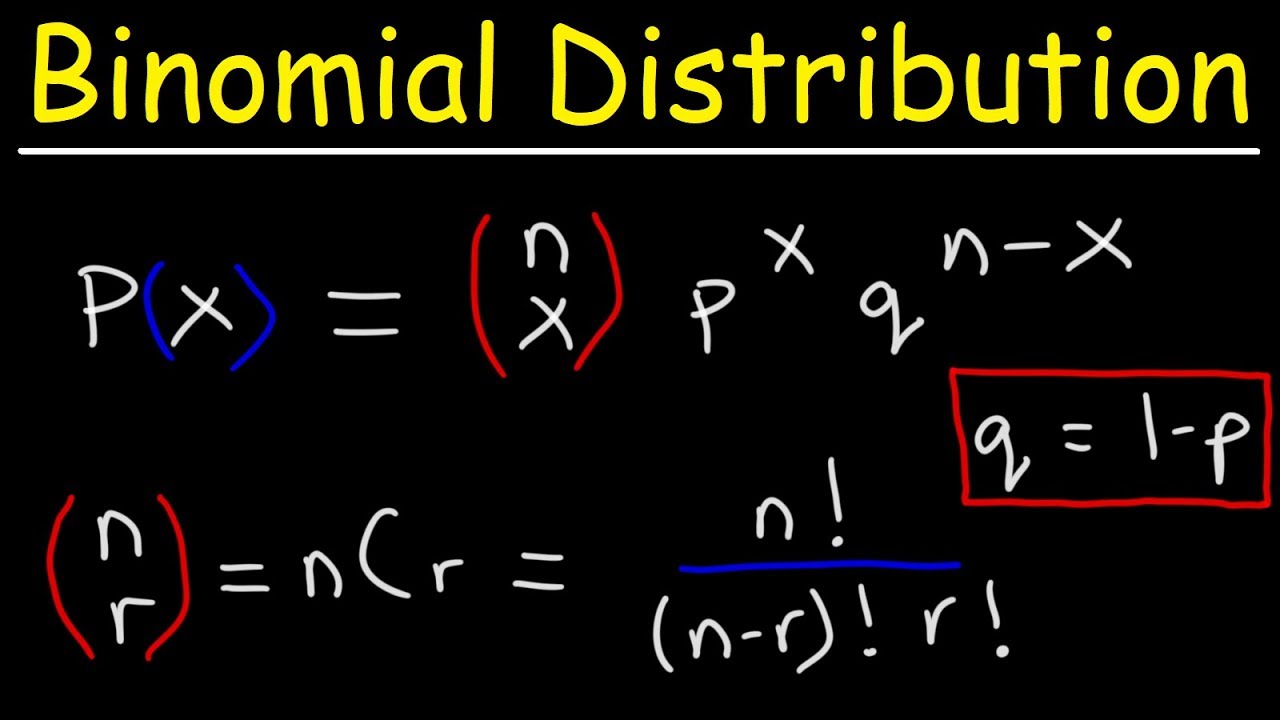
Finding The Probability of a Binomial Distribution Plus Mean & Standard Deviation

The Binomial Distribution: Crash Course Statistics #15

5.2.5 Binomial Probability Distributions - Using Probabilities to Determine Significance

Probability Part 2: Updating Your Beliefs with Bayes: Crash Course Statistics #14

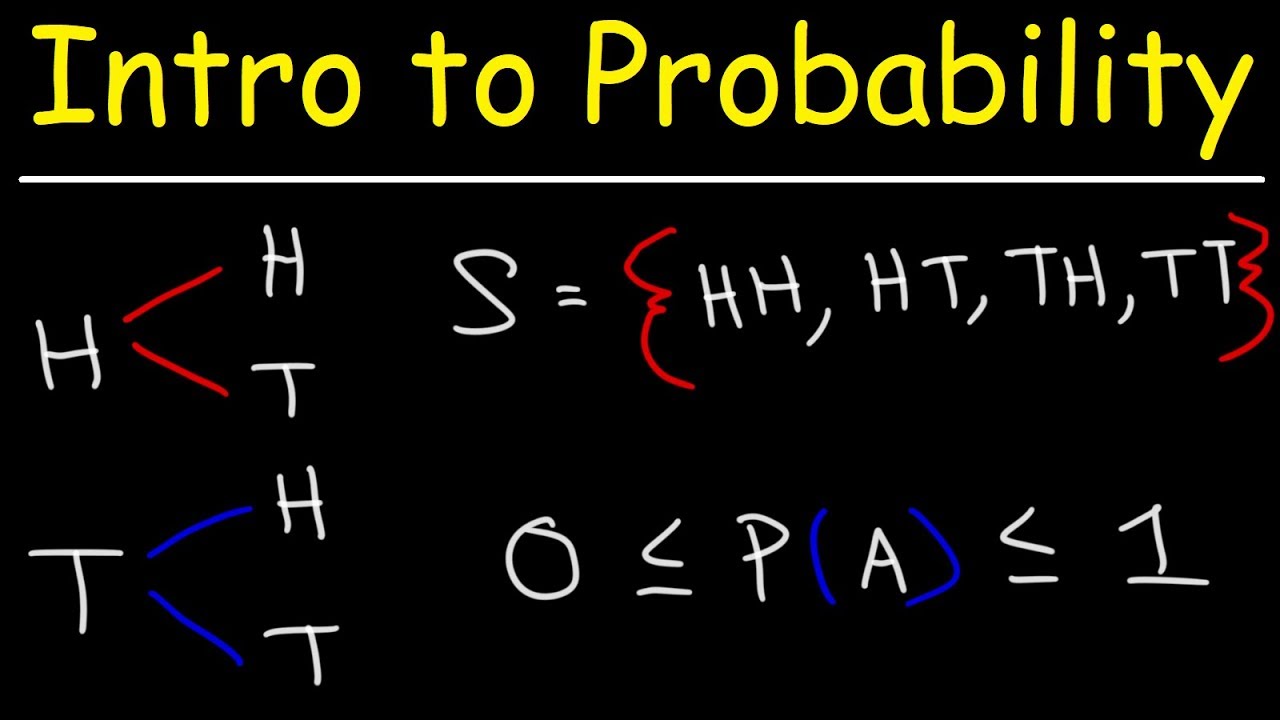
Introduction to Probability, Basic Overview - Sample Space, & Tree Diagrams

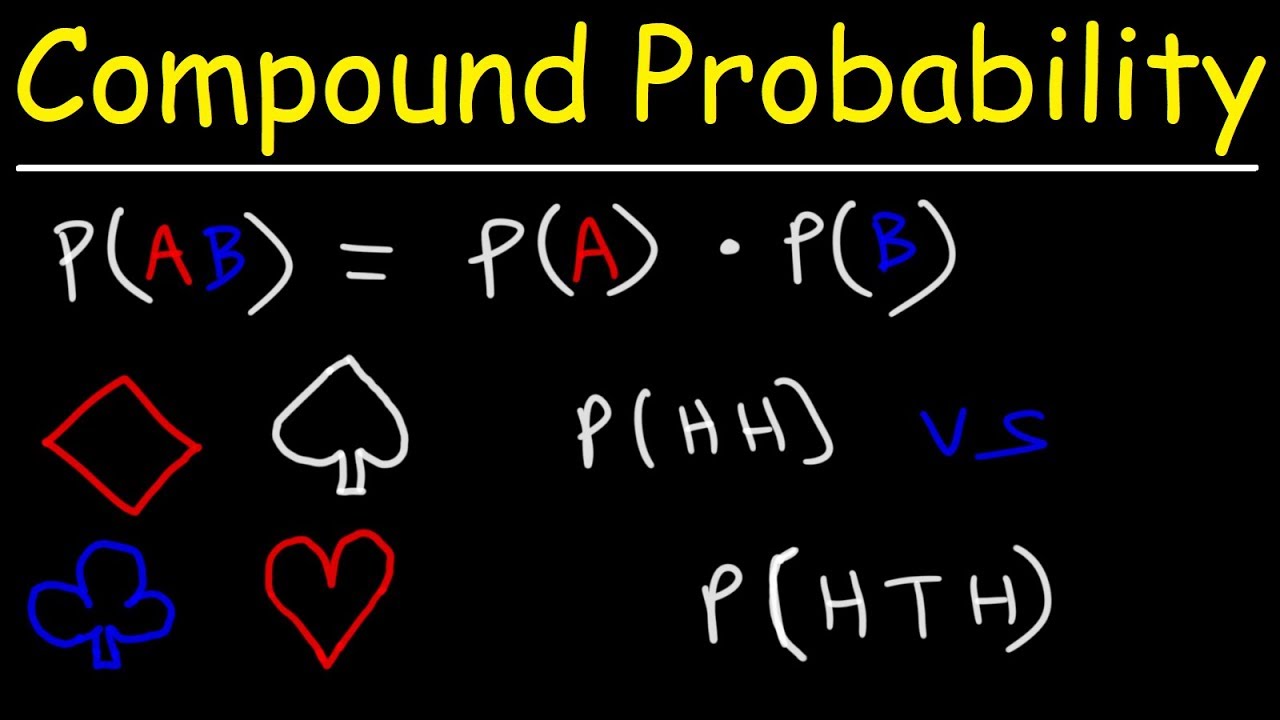
Compound Probability of Independent Events - Coins & 52 Playing Cards

Rate This

5.0 / 5 (0 votes)

Thanks for rating: