How to Use the Law of Sines in Trigonometry (Precalculus - Trigonometry 32)
TLDRThis video script delves into the application of the Law of Sines, a fundamental concept in trigonometry for solving non-right triangles. The presenter clarifies that while right triangle trigonometry is sufficient when dealing with triangles that include a 90-degree angle, the Law of Sines becomes essential for oblique triangles that lack a right angle. The script outlines various scenarios where the Law of Sines is applicable, such as when two angles and one side of a triangle are known, or when two sides and a non-adjacent angle are given. The presenter also addresses the potential for multiple solutions in certain cases, highlighting the 'ambiguous case' where knowing two sides and a non-adjacent angle can lead to one, two, or no possible triangles. The video educates viewers on how to set up proportions using the Law of Sines to find missing angles or sides and emphasizes the importance of checking solutions to ensure they result in a valid triangle. Practical applications, such as calculating the height of a volcano by measuring angles from different distances, are also discussed, demonstrating how the Law of Sines can be used in real-world scenarios. The summary underscores the Law of Sines as a powerful tool for solving a wider array of trigonometric problems beyond the scope of right triangle trigonometry.
Takeaways
- 📐 The Law of Sines is used for non-right triangles to find missing sides or angles when certain conditions are met.
- ⚙️ Right triangle trigonometry doesn't apply to oblique triangles, necessitating the use of the Law of Sines or Law of Cosines.
- 🔍 There are four cases for solving triangles with the Law of Sines: two angles and one side known, two sides and one non-included angle known, all three sides known, or one angle and two sides known with the angle being between the two sides.
- 🧩 For the Law of Sines to work, you need to know three things to find a fourth (e.g., two angles and one side, or two sides and one non-included angle).
- 📊 The Law of Sines creates proportions based on the relationship between angles and their opposite sides, allowing for the cross-multiplication method to solve for missing parts of the triangle.
- 🔢 When using the Law of Sines, it's crucial to avoid using rounded values in further calculations to prevent compounding errors.
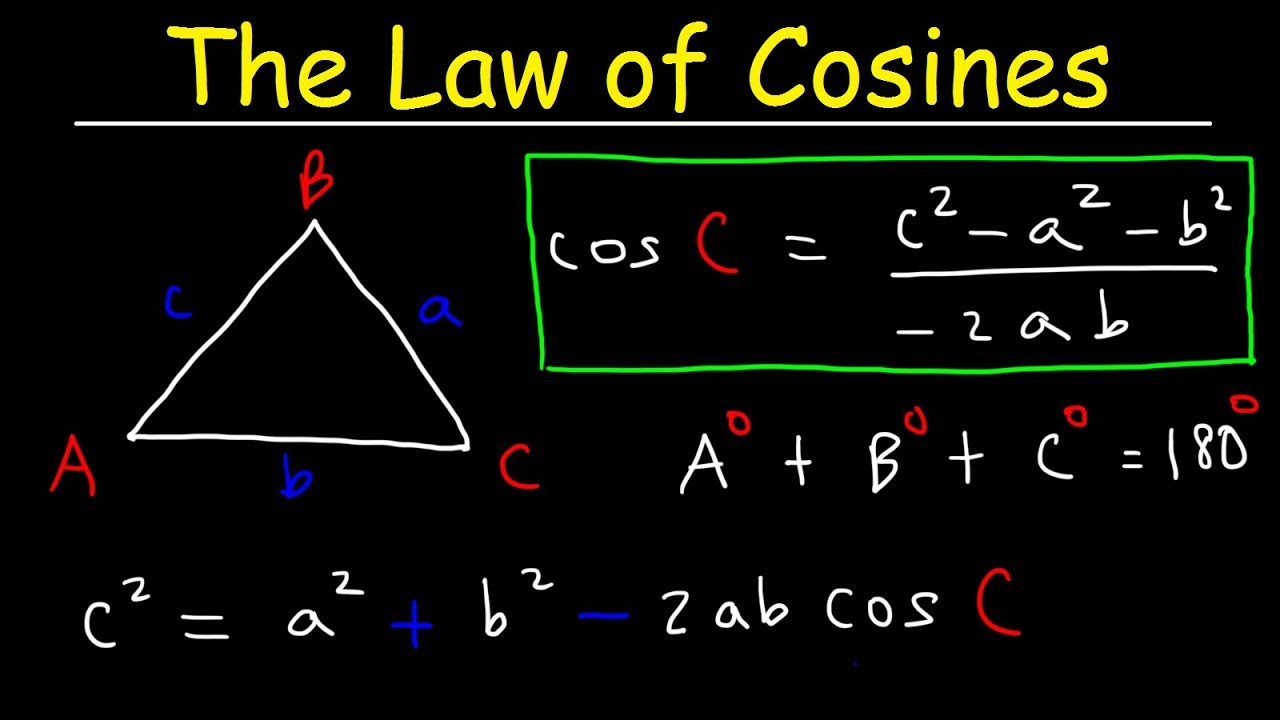
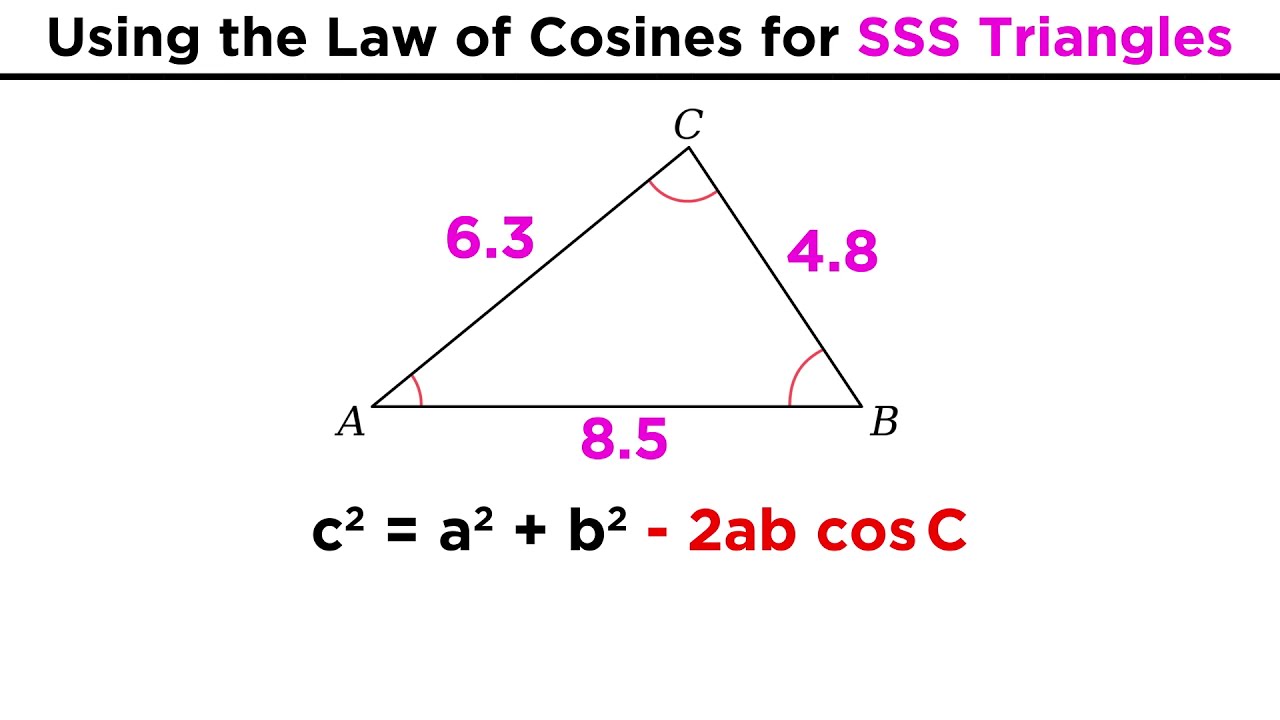
- 🆚 The Law of Cosines is used in different scenarios than the Law of Sines, such as when you know two sides and an angle between them or all three sides of the triangle.
- 🍃 An ambiguous case in the Law of Sines occurs when you have two sides and an angle not between them, which can result in zero, one, or two possible triangles based on the length of the unknown side.
- 🔄 The sine function's properties are essential in understanding the ambiguous case, as it can yield two angles with the same y-coordinate on the unit circle, leading to potential multiple solutions.
- 🏞️ The interior angle sum (180 degrees for a triangle) is a fundamental check to determine the validity of a triangle's angles and is used in conjunction with the Law of Sines.
- 📐 The Law of Sines can also be applied to right triangles, but it's generally unnecessary as right triangle trigonometry suffices.
Q & A
What is the Law of Sines and why is it used?
-The Law of Sines is a trigonometric formula used to solve for missing sides and angles in non-right triangles. It is used when you have either two angles and one side, or two sides and an angle not between them, allowing you to find the remaining parts of the triangle.
What are the different types of triangles mentioned in the script?
-The script mentions three types of triangles: right triangles, acute triangles, and obtuse triangles. Right triangles have one right angle, acute triangles have all angles less than 90 degrees, and obtuse triangles have one angle greater than 90 degrees.
How does the Law of Sines help in solving ambiguous cases?
-The Law of Sines helps in solving ambiguous cases by determining whether a triangle can be formed with the given measurements. It can yield no solution, one solution (forming a right triangle), or two solutions (forming either an acute or an obtuse triangle), depending on the lengths of the sides relative to each other.
What is the formula for the Law of Sines?
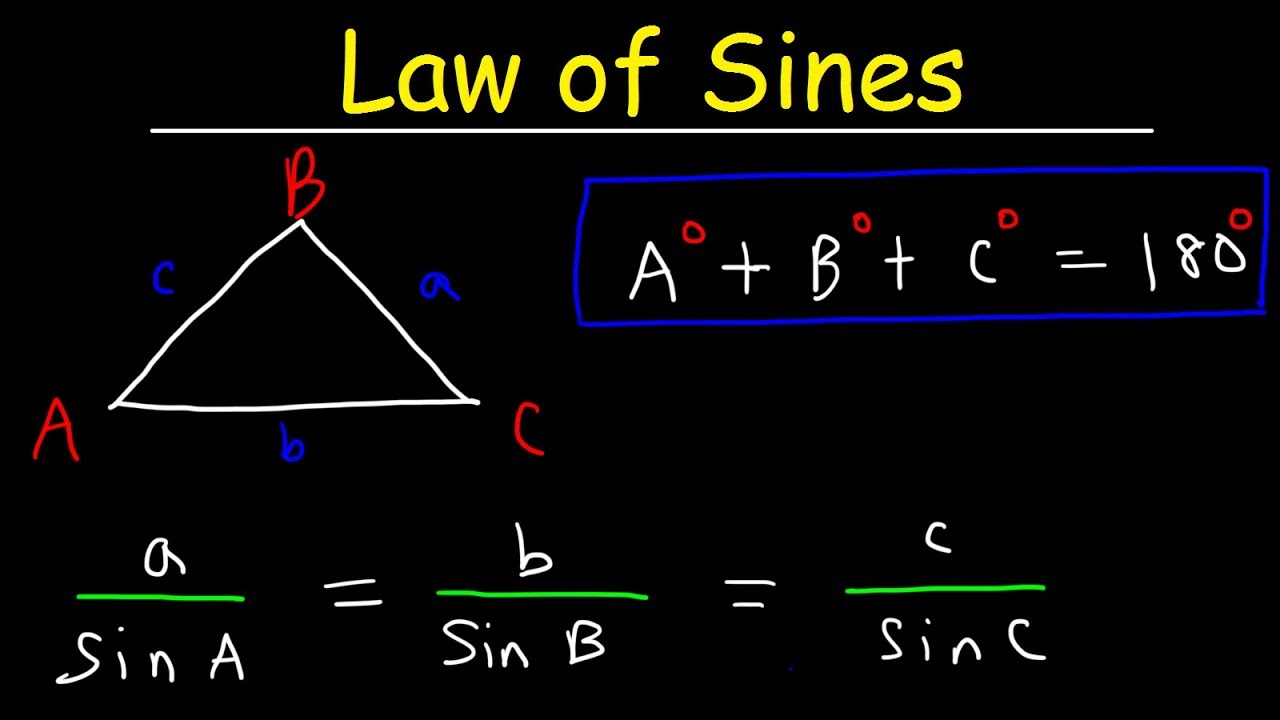
-The Law of Sines formula states that the ratio of the length of a side of a triangle to the sine of its opposite angle is the same for all three sides of the triangle. Mathematically, it is written as (a/sinA) = (b/sinB) = (c/sinC), where a, b, and c are the side lengths, and A, B, and C are the opposite angles.
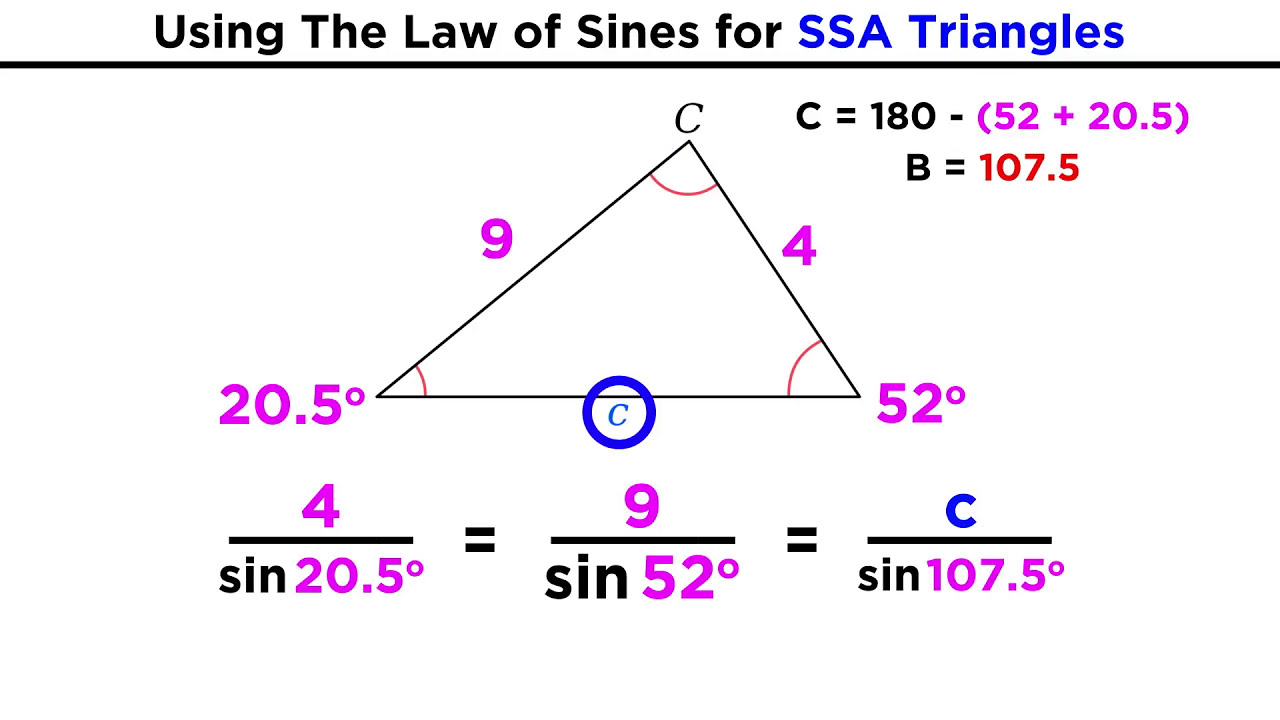
How does the Law of Sines handle the situation when you know two sides and an angle not between them?
-When you know two sides and an angle not between them, the Law of Sines sets up a proportion using one of the known sides and its opposite angle to find the unknown side. It can result in one solution, no solution, or two solutions depending on the lengths of the known sides.
What is the interior angle sum theorem and how is it used in the context of the Law of Sines?
-The interior angle sum theorem states that the sum of the interior angles of a triangle is always 180 degrees. It is used in conjunction with the Law of Sines to find a missing angle when two angles and one side of a triangle are known.
How does the Law of Sines apply to right triangles?
-While the Law of Sines is primarily used for non-right triangles, it can also be applied to right triangles. However, in a right triangle, since one angle is always 90 degrees, the Law of Sines would simplify to a form of right triangle trigonometry, making it unnecessary to use the full Law of Sines.
What are the steps to solve a triangle using the Law of Sines?
-The steps to solve a triangle using the Law of Sines are: 1) Identify if the Law of Sines is applicable (two angles and one side, or two sides and an angle not between them are known). 2) Set up a proportion using the Law of Sines formula. 3) Cross-multiply and solve for the unknown side or angle. 4) Check for possible ambiguous cases and determine the number of solutions.
What is the difference between the Law of Sines and the Law of Cosines?
-The Law of Sines is used when you have two angles and one side, or two sides and an angle not between them of a non-right triangle. The Law of Cosines, on the other hand, is used when you know three sides of a triangle (or two sides and an angle between them) and need to find an angle, or when you have one side and two angles and need to find the remaining side.
How can you check if your solution to a triangle using the Law of Sines is correct?
-You can check your solution by ensuring that the sum of the angles is 180 degrees and that the larger angles are opposite the longer sides. Additionally, if you have found a side, you can use the interior angle sum theorem to verify that the remaining angles can exist with the given side lengths.
What is an example of a real-world application of the Law of Sines?
-An example of a real-world application of the Law of Sines is in surveying, where you might need to find the height of a building or a mountain without direct access to its base. By measuring angles from different known distances, you can use the Law of Sines to calculate the height.
Outlines
📚 Introduction to the Law of Sines
The paragraph introduces the concept of the Law of Sines and its relevance in trigonometry, especially when dealing with non-right triangles. It explains the need for the Law of Sines and Cosines when right triangle trigonometry is not applicable. The speaker sets the foundation for the discussion by differentiating between right triangles and oblique triangles, and explains the categories of triangles, including acute and obtuse triangles. The importance of knowing two angles and a side to find a missing side or two sides and an angle to find a missing angle is highlighted, setting up the context for the Law of Sines.
🔍 When to Apply the Law of Sines
This paragraph delves into the specific scenarios where the Law of Sines can be applied. It outlines four cases of oblique triangles that can be solved using the Law of Sines and Law of Cosines. The paragraph clarifies the difference between right triangle trigonometry and the Law of Sines, emphasizing that three known elements are needed to find a fourth in non-right triangles. It also introduces the concept of ambiguous cases, where knowing two sides and a non-adjacent angle can lead to multiple solutions or no solution at all. The speaker explains the potential outcomes, including no triangle, a right triangle, or two possible obtuse or acute triangles.
📐 Law of Sines: Proportions and Applications
The paragraph explains the Law of Sines in detail, focusing on how it creates proportions to solve for unknown sides or angles in a triangle. The Law of Sines is expressed as a proportion where the sine of an angle over its opposite side is equal to the sine of another angle over its opposite side. The speaker clarifies that this is not about solving the entire equation at once but rather using one proportion at a time. The paragraph emphasizes the importance of identifying whether the problem fits the Law of Sines and the process of setting up and solving proportions to find missing elements in a triangle.
🤔 Error Avoidance in Law of Sines Calculations
The speaker advises on the importance of avoiding rounding errors when using the Law of Sines. They stress that while it's sometimes necessary to use rounded numbers, it's best to minimize their use to prevent compounding errors. The paragraph also addresses the misuse of the Pythagorean theorem in non-right triangle scenarios and the potential for rounding errors when approximating side lengths. The speaker provides a clear warning against using rounded values in future calculations and emphasizes the need for precision in applying the Law of Sines.
🧩 Solving Triangles Using the Law of Sines
The paragraph walks through the process of solving triangles using the Law of Sines. It covers the step-by-step approach to identifying whether a problem can be solved with the Law of Sines, setting up the correct proportions, and solving for the unknowns. The speaker provides examples of different scenarios, including when to use the interior angle sum and how to handle cases with multiple solutions. They also discuss the importance of checking work using the interior angle sum to ensure the validity of the solutions and to confirm that no negative angles are present, as only positive angles are possible in a triangle.
🌋 Real-world Application: Estimating Distances
The paragraph presents a real-world application of the Law of Sines, demonstrating how it can be used to estimate distances or heights, such as the height of a volcano, without direct measurement. The speaker explains a two-step process involving initial angle measurement, moving to a known distance to take a second measurement, and then using the Law of Sines to find the missing side. They then apply right triangle trigonometry to calculate the height of the volcano. The example illustrates the practical use of trigonometry and the Law of Sines in solving real-world problems.
🚫 No Solution Scenario with Law of Sines
The speaker discusses a scenario where the Law of Sines does not yield a solution. They explain that if the calculated sine of an angle exceeds the possible range of sine values (-1 to 1), the situation is impossible and no solution exists. The speaker emphasizes the importance of understanding why an error occurs and how to identify that no solution is possible. This scenario is important for understanding the limitations of the Law of Sines and the necessity of checking the validity of the solutions obtained through trigonometric methods.
Mindmap
Keywords
💡Law of Sines
💡Oblique Triangle
💡Right Triangle Trigonometry
💡Interior Angle Sum
💡Sine Function
💡Pythagorean Theorem
💡Law of Cosines
💡Trigonometry
💡Acute Triangle
💡Obtuse Triangle
💡Ambiguous Case
Highlights
The Law of Sines is essential for solving non-right triangles, where right triangle trigonometry is not applicable.
Oblique triangles, which lack a right angle, can be further categorized into acute and obtuse triangles.
The Law of Sines can solve four cases of oblique triangles: two angles and one side known, two sides and one non-adjacent angle known, all three sides known, or two sides and an angle between them known.
In right triangle trigonometry, knowing two sides or an angle and a side allows you to find a third element, whereas with the Law of Sines, you need to know three elements to find the fourth.
The Law of Sines states that the ratio of the length of a side of a triangle to the sine of its opposite angle is constant for all three sides.
When using the Law of Sines, you may encounter an ambiguous case that can yield no solution, one solution (forming a right triangle), or two solutions (forming either an acute or obtuse triangle).
The sine function can reveal whether a triangle is possible and how many solutions to expect when applying the Law of Sines.
For side-angle-side (SAS) triangles, finding the first angle using the Law of Sines can result in two possible angles due to the properties of the sine function on a unit circle.
When using the Law of Sines to find a missing side, you create a proportion using two known angles and their opposite sides to solve for the unknown side.
The Law of Sines can be used to approximate side lengths, but it's important to avoid using rounded values in subsequent calculations to prevent compounding errors.
In cases where the Law of Sines yields multiple solutions, each valid angle must be checked against the interior angle sum theorem to determine if it forms a valid triangle.
The concept of 'angle-side-angle' (ASA) and 'side-angle-side' (SAS) is crucial in determining the correct application of the Law of Sines and the expected number of solutions.
The Law of Sines is particularly useful in real-world applications, such as surveying or calculating the height of an object when the distance to the object is unknown.
When applying the Law of Sines to find the height of an object, like a volcano, you can use the known angles and a measured distance to calculate the unknown side lengths and subsequently the height.
It's important to remember to add your eye level or the height from which you're measuring when calculating the total height of an object using trigonometric methods.
The Law of Sines is a powerful tool for solving a variety of triangle problems, especially when dealing with non-right triangles and limited information.
Transcripts
Browse More Related Video
Law of Sines, Basic Introduction, AAS & SSA - One Solution, Two Solutions vs No Solution, Trigonomet

How to Use the Law of Cosines in Trigonometry (Precalculus - Trigonometry 33)
The Law of Sines

Law of Sines - Basic Introduction
Law of Cosines, Finding Angles & Sides, SSS & SAS Triangles - Trigonometry
The Law of Cosines
5.0 / 5 (0 votes)
Thanks for rating: