The Law of Sines

Professor Dave Explains
31 Jan 201805:13
EducationalLearning
32 Likes 10 Comments

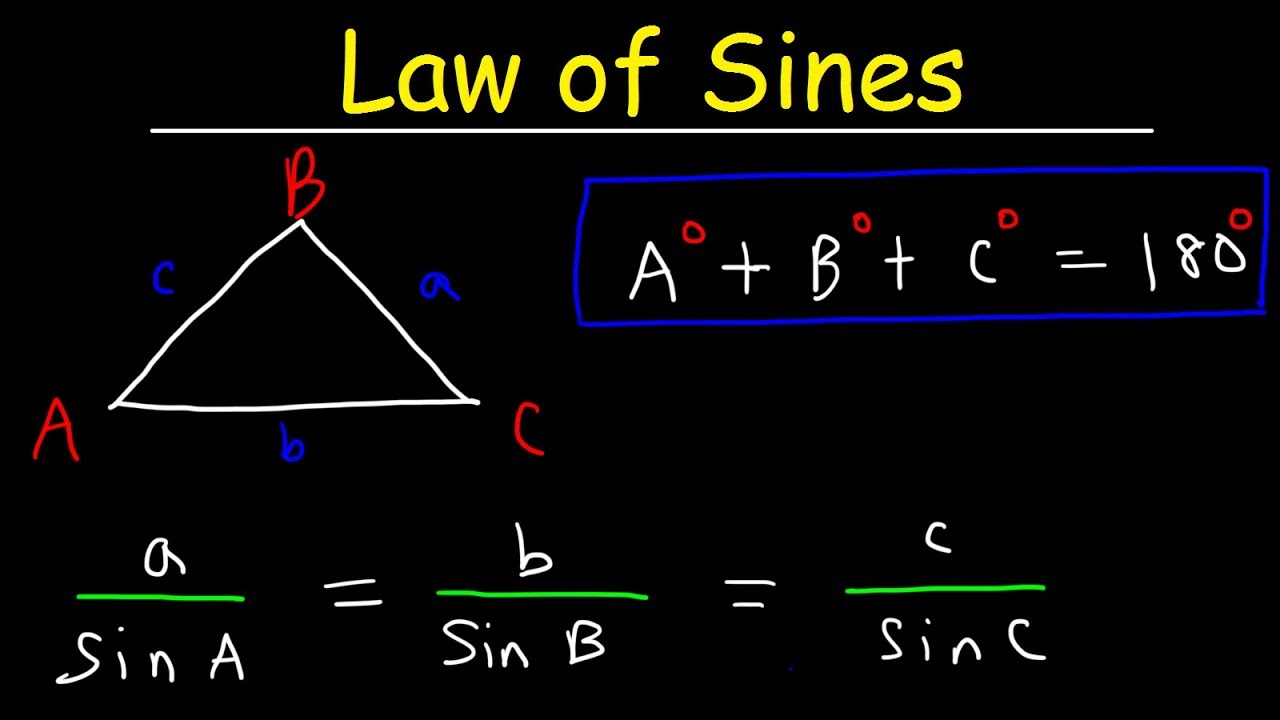
TLDRThe law of sines is used to find sides and angles of oblique triangles, those without a right angle. It states that for any triangle with angles A, B, C and opposite sides a, b, c; a/sin(A) = b/sin(B) = c/sin(C). Given some knowns, we can use these ratios to calculate unknowns. We may know: side-angle-angle, angle-side-angle, or side-side-angle. The last case may show a triangle can't exist. We can also find area as (1/2)ab(sinC). This covers using law of sines for oblique triangles.

Takeaways
  • 😀 The law of sines allows us to find missing sides and angles in oblique triangles using the ratios of sides to sines of opposite angles
  • 👉 Oblique triangles don't have a 90 degree angle, so SOHCAHTOA doesn't apply
  • 🔼 The law states: a/sin(A) = b/sin(B) = c/sin(C) for a triangle with sides a, b, c and angles A, B, C
  • 📐 If we know two angles and one side (side angle angle), we can use the law to find the other sides
  • ⛓ If we know two sides and one angle (side side angle), we can use the law to find the other angles and remaining side
  • ❌ In SSA triangles, sometimes no triangle is possible if sin values end up > 1
  • 🔀 SSA triangles can also result in two valid solutions for the third angle
  • 👍 The law of sines works for any oblique triangle with one known side/angle pair
  • 📏 The area of an oblique triangle equals 1⁄2 x b x h, where b and h are any two side lengths and the sine of the angle between them
  • 🙌 There are three ways to get the area per triangle using the law of sines formula
Q & A

  • What is the law of sines?

    -The law of sines is a relationship between the sides and angles of oblique triangles (triangles without a 90 degree angle). It states that the ratio of any side of the triangle to the sine of its opposite angle is equal to the other such ratios in the triangle.

  • When can we use the law of sines?

    -We can use the law of sines when we have an oblique triangle, meaning one without a 90 degree angle. It allows us to solve for missing sides and angles when we know at least some of that information.

  • What information do we need to apply the law of sines?

    -To apply the law of sines, we need to know at least two angles and one side (ASA or AAS) or at least two sides and one angle (SSA). This gives us enough information to solve for the remaining sides and angles.

  • What is a side-angle-angle (SAA) triangle?

    -A side-angle-angle triangle means we know one side length of the triangle and two of the angle measures. This is enough information to use the law of sines to solve for the remaining sides and third angle.

  • What is an angle-side-angle (ASA) triangle?

    -An angle-side-angle triangle means we know two angles of the triangle and the side length opposite one of those known angles. This also provides enough information to use the law of sines.

  • What happens with SSA triangles sometimes?

    -With side-side-angle (SSA) triangles, sometimes using the information leads to no possible triangle, or two potential triangle solutions. This occurs when solving for a sine value that is not possible or corresponds to two angles.

  • How do we find the third angle, given two known angles?

    -If we know two angles of a triangle, we can always calculate the third angle by subtracting the sum of the known angles from 180 degrees.

  • How is the law of sines useful in calculating triangle area?

    -One formula for a triangle's area uses the law of sines, involving multiplying two side lengths by the sine of the angle between those sides. This can be done with any two sides.

  • Why doesn't SOHCAHTOA work for oblique triangles?

    -SOHCAHTOA only applies to right triangles with one 90 degree angle. Oblique triangles have no 90 degree angle, so we need to use the law of sines instead to relate sides and angles.

  • What units do we use for the law of sines calculations?

    -When applying the law of sines, side lengths are typically measured in units like feet or meters. The angles are measured in degrees for use in sine calculations.

Outlines
00:00
📐 Introducing the Law of Sines

Paragraph 1 introduces the law of sines, explaining that it is a relationship used to find sides and angles of oblique triangles. It states that for any triangle with angles A, B, C and opposite sides a, b, c; a/sin(A) = b/sin(B) = c/sin(C). Some applications are demonstrated.

😎 Using the Law of Sines Step-by-Step

Paragraph 2 walks through step-by-step examples of using the law of sines when given different known triangle parameters. Cases covered include side-angle-angle, angle-side-angle, and side-side-angle scenarios. The paragraph also notes special cases and considerations.

📏 Area Formula for Oblique Triangles

Paragraph 3 presents the formula for calculating the area of an oblique triangle using the law of sines. The area equals (1/2)ab*sin(C), where a, b are two side lengths and C is the angle between them.

Mindmap
Keywords
💡Oblique triangle
An oblique triangle is a triangle that does not contain a right angle (90 degrees). The law of sines can be used to solve for missing sides and angles in oblique triangles, unlike right triangles which can use SOHCAHTOA relationships. Oblique triangles are mentioned in the beginning of the transcript as the shapes that the law of sines can help analyze.
💡Law of sines
The law of sines is a mathematical relationship that can be used to solve oblique triangles. It states that the ratio of each side to the sine of its opposite angle is equal for all sides and angles. For example, a/sin(A) = b/sin(B) = c/sin(C) for any oblique triangle with angles A, B, and C. The law of sines is discussed extensively and several examples of its use are shown.
💡side angle angle (SAA)
A side angle angle triangle has one known side length and two known angle measures. The law of sines can be used with a SAA triangle to solve for the two missing sides and one missing angle. An example of solving a SAA triangle is shown in the middle of the transcript.
💡angle side angle (ASA)
An angle side angle triangle has two known angles and one known side length, specifically the side between the two known angles. The law of sines can solve an ASA triangle as shown in the transcript. The third angle is found by subtracting the known angles from 180 degrees.
💡side side angle (SSA)
A SSA triangle has two known sides and one known angle. The law of sines can sometimes prove a SSA triangle cannot exist if the sine of a calculated angle exceeds 1. This concept is mentioned near the end of the transcript.
💡Area formula
The area of an oblique triangle can be calculated using the law of sines formula: Area = (1/2)(a)(b)sin(C). This relates the area to two side lengths and the sine of the angle between those sides. The area formula is the last concept covered.
💡Sine function
The sine function gives the ratio of the opposite side to the hypotenuse in a right triangle, and is used throughout the law of sines calculations. Sine values are between -1 and 1, so a sine greater than 1 proves a triangle cannot exist.
💡Degrees
When using the law of sines, the angles are typically measured in degrees rather than radians. Degree values are plugged into a calculator to approximate side lengths.
💡Subtract from 180
With two known angles in a triangle, the third can always be found by subtracting the sum of the known angles from 180 degrees since all angles in a triangle add to 180 degrees.
💡Solve for missing values
A main application of the law of sines is to solve oblique triangles with missing side lengths or angle measures. At least 3 pieces of information allow the rest to be calculated using the sines ratio.
Highlights

The law of sines allows us to find sides and angles of oblique triangles where SOHCAHTOA does not apply.

The law states: A/sin(A) = B/sin(B) = C/sin(C) for sides a, b, c and opposite angles A, B, C.

We can use the law if we know at least 2 angles and 1 side, or 2 sides and 1 angle.

For side-angle-angle triangles, subtract known angles from 180 to get the third angle.

For angle-side-angle triangles, also subtract known angles from 180 for the third angle.

For side-side-angle triangles, use the law to find the angle opposite a known side.

SSA triangles may prove no triangle exists if sine of an angle exceeds 1.

SSA triangles can result in two solutions for the third angle.

Use degrees for angles and calculate approximate side lengths.

Oblique triangle area = (1/2)(a)(b)sin(C) where a, b are sides and C is between them.

There are 3 ways to calculate area since each angle separates 2 sides.

The law helps find sides and angles without needing a right angle.

Need to know at least 2 angles + 1 side or 2 sides + 1 angle to apply law.

Law relates ratios of sides and sines of opposite angles.

Can determine if some SSA triangles are impossible based on sine limits.

Transcripts

Browse More Related Video

Law of Cosines, Finding Angles & Sides, SSS & SAS Triangles - Trigonometry

How to Use the Law of Sines in Trigonometry (Precalculus - Trigonometry 32)

The Law of Cosines

Area of an Oblique Triangle - SAS & SSS - Heron's Formula, Trigonometry

Law of Sines, Basic Introduction, AAS & SSA - One Solution, Two Solutions vs No Solution, Trigonomet

Learn to find the missing angles for a triangle using inverse trig functions

Rate This

5.0 / 5 (0 votes)

Thanks for rating: