AP Precalculus Practice Exam Question 20

NumWorks
23 May 202303:53
EducationalLearning
32 Likes 10 Comments

TLDRIn the video script, the speaker is analyzing a trigonometric function graph to determine its expression. They start by identifying the midline and amplitude, which are common across all answer choices. The focus then shifts to the phase shift and whether it's a cosine or sine graph. After eliminating options with incorrect phase shifts, the speaker concludes that the correct answer is a sine function with a phase shift of pi/4, leading to the selection of choice C as the correct trigonometric expression for the graph.

Takeaways
  • πŸ“Š The script discusses identifying a trigonometric function from a graph.
  • πŸ“ The first step is to identify the midline of the trigonometric expression, which is at y = -1.
  • πŸ” All answer choices (A, B, C, D) have the same midline, so this does not help to eliminate any options.
  • πŸ“ The amplitude of the function is determined to be 3 units, as it travels from the midline to the maximum.
  • πŸ”„ All options also share the same amplitude of 3, so this does not narrow down the choices.
  • πŸ”’ The frequency of the function is stated to be 2 for all options.
  • πŸŒ€ The main difference to be identified is whether the graph represents a cosine or sine function and the phase shift.
  • 🚫 Options A and B, which are cosine functions, are eliminated because they do not match the phase shift of the graph.
  • πŸ“‰ The script concludes that the function must be a sine function due to the starting point and phase shift.
  • πŸ”„ The sine function starts on the midline and is increasing, indicating a phase shift to the right.
  • 🎯 The correct answer is identified as Choice C, which has the appropriate phase shift of pi/4 to align with the graph.
Q & A

  • What is the midline of the trigonometric function described in the script?

    -The midline of the trigonometric function is at the y-value of negative one, as identified in the script.

  • What is the amplitude of the trigonometric function in the script?

    -The amplitude of the function is three units, as it travels from the midline to the maximum value and back down to the minimum value.

  • What is the frequency of the trigonometric function discussed in the script?

    -The frequency of the function is two, as all the given options in the script have this frequency.

  • Why were options A and B eliminated as possible expressions for the function?

    -Options A and B were eliminated because they represent cosine functions with phase shifts of pi/4 and pi/8, which do not align with the max value at pi/2 as described in the script.

  • What is the phase shift of the correct trigonometric function according to the script?

    -The phase shift of the correct function is pi/4 to the right, which aligns the sine wave with the graph provided.

  • Why is the correct answer identified as Choice C in the script?

    -Choice C is identified as the correct answer because it is the only option with the appropriate phase shift of pi/4 for a sine graph, which matches the given graph.

  • What is the significance of identifying the starting value or phase shift of the trigonometric function?

    -Identifying the starting value or phase shift is crucial for determining the exact expression of the trigonometric function, as it affects the position of the graph on the coordinate plane.

  • How does the script differentiate between a cosine and a sine graph?

    -The script differentiates by noting where the function starts and the direction of increase. A cosine graph starts at a max value and is moved to the right, while a sine graph starts on the midline and increases.

  • What is the role of the midline in determining the type of trigonometric function?

    -The midline helps in identifying the vertical shift of the function and is the reference point for determining the amplitude and phase shift.

  • Why is amplitude important in the context of the script?

    -Amplitude is important as it represents the distance from the midline to the maximum or minimum value of the function, which is a key characteristic of the function's graph.

  • How does the script use the properties of trigonometric functions to eliminate incorrect options?

    -The script uses properties like amplitude, frequency, and phase shift to compare the given options with the characteristics of the graph, eliminating those that do not match.

Outlines
00:00
πŸ“Š Trigonometric Function Graph Analysis

The script discusses how to determine the expression of a trigonometric function given a graph. It emphasizes the importance of identifying the midline, amplitude, and frequency to narrow down the correct trigonometric expression. The speaker eliminates options based on these characteristics and concludes that the function is a sine graph with a phase shift, leading to the selection of the correct answer choice, which is not explicitly stated in the script but implied to be the one with a phase shift of pi over 4.

Mindmap
Keywords
πŸ’‘Trigonometric function
A trigonometric function is a periodic function that relates the angles of a triangle to the lengths of its sides. In the context of the video, the trigonometric function is being graphed to identify its properties such as midline, amplitude, and frequency. The script discusses identifying the trigonometric function by its graph, which is essential for solving the problem presented.
πŸ’‘Midline
The midline of a trigonometric function is the vertical line that passes through the average value of the function. In the video, the speaker identifies the midline as the y-value of -1, which is a crucial step in determining the correct trigonometric expression from the given options.
πŸ’‘Amplitude
Amplitude in a trigonometric function refers to the distance from the midline to the peak (or trough) of the function. The script mentions that the amplitude is three units, which is a key characteristic that helps in eliminating incorrect options for the function's expression.
πŸ’‘Frequency
Frequency in the context of a trigonometric function is the number of cycles the function completes in a given interval. The script notes that all options have a frequency of two, indicating that the function repeats its pattern twice within the observed interval.
πŸ’‘Cosine graph
A cosine graph represents the cosine function, which is one of the fundamental trigonometric functions. The video discusses the possibility of the graph being a cosine function and how it starts at a maximum value, which is used to eliminate certain options.
πŸ’‘Sine graph
A sine graph represents the sine function, another fundamental trigonometric function. The script concludes that the graph is likely a sine function after eliminating the cosine options and identifying the phase shift.
πŸ’‘Phase shift
Phase shift is the horizontal displacement of a trigonometric function from its standard position. In the script, the phase shift is identified as being to the right by Ο€/4, which helps in determining the correct function expression.
πŸ’‘Starting value
The starting value is the initial point on the x-axis where a trigonometric function begins its cycle. The script discusses the starting value in relation to the phase shift and how it helps in identifying the correct trigonometric expression.
πŸ’‘Graph
A graph in this context is a visual representation of the trigonometric function. The script uses the graph to analyze and deduce the properties of the function, such as midline, amplitude, and phase shift.
πŸ’‘Option C
In the script, 'Option C' is the correct answer that the speaker arrives at after analyzing the graph and properties of the trigonometric function. It represents the trigonometric expression that matches the graph with the appropriate phase shift.
πŸ’‘Elimination
Elimination in this context refers to the process of ruling out incorrect options for the trigonometric function's expression. The script uses the properties of the graph to eliminate options A, B, and D, leading to the selection of Option C.
Highlights

The task is to determine the expression for a trigonometric function f from a given graph.

Identifying the midline of the trigonometric expression is crucial for solving the problem.

All answer choices have a midline value of -1, which does not help in eliminating options.

The amplitude of the trigonometric function is determined to be 3 units.

All options have the same amplitude of 3, so this does not narrow down the choices.

The frequency of the function is stated to be two for all options.

The main difference to identify is whether the graph represents a cosine or sine function.

The phase shift of the function is a key factor in determining the correct trigonometric expression.

Cosine graphs start at a maximum value, but the phase shifts in options A and B do not match the graph.

Sine graphs start on the midline and increase, suggesting a phase shift analysis for sine.

The phase shift is determined to be between 0 and pi/2, specifically pi/4.

Choice C is identified as the correct answer due to the appropriate phase shift for a sine graph.

The process involves elimination based on the midline, amplitude, frequency, and phase shift.

The analysis of the graph's properties leads to the exclusion of cosine options A and B.

The sine graph with a phase shift of pi/4 aligns with the given graph, pointing to Choice C.

Understanding the properties of trigonometric functions is essential for graph analysis.

The methodical approach to identifying the trigonometric function is demonstrated through the transcript.

Transcripts

Browse More Related Video

Writing Equations for Sinusoidal Functions

How do you determine the phase shifts for sine and cosine graphs

How to Graph Phase Shifts of Trigonometric Functions (Precalculus - Trigonometry 16)

Graphing Transformations with Sine and Cosine (Precalculus - Trigonometry 12)

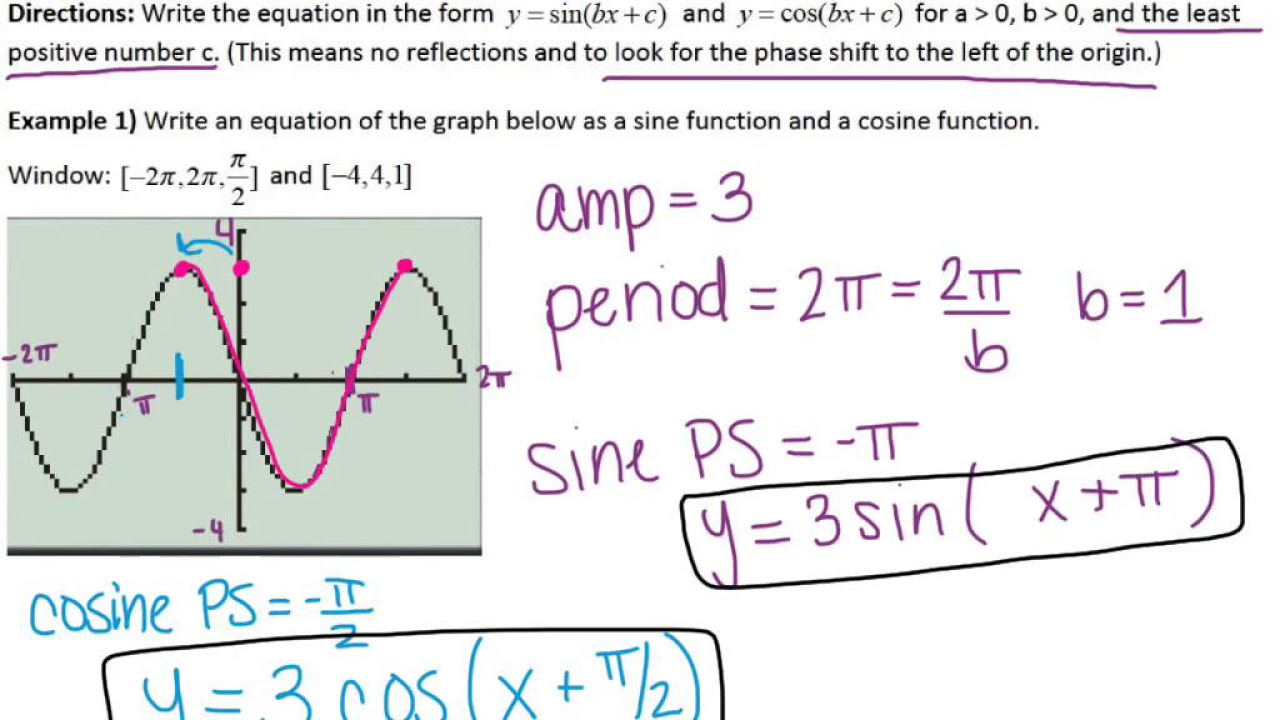
Precalc 5.5 Day 2 Notes

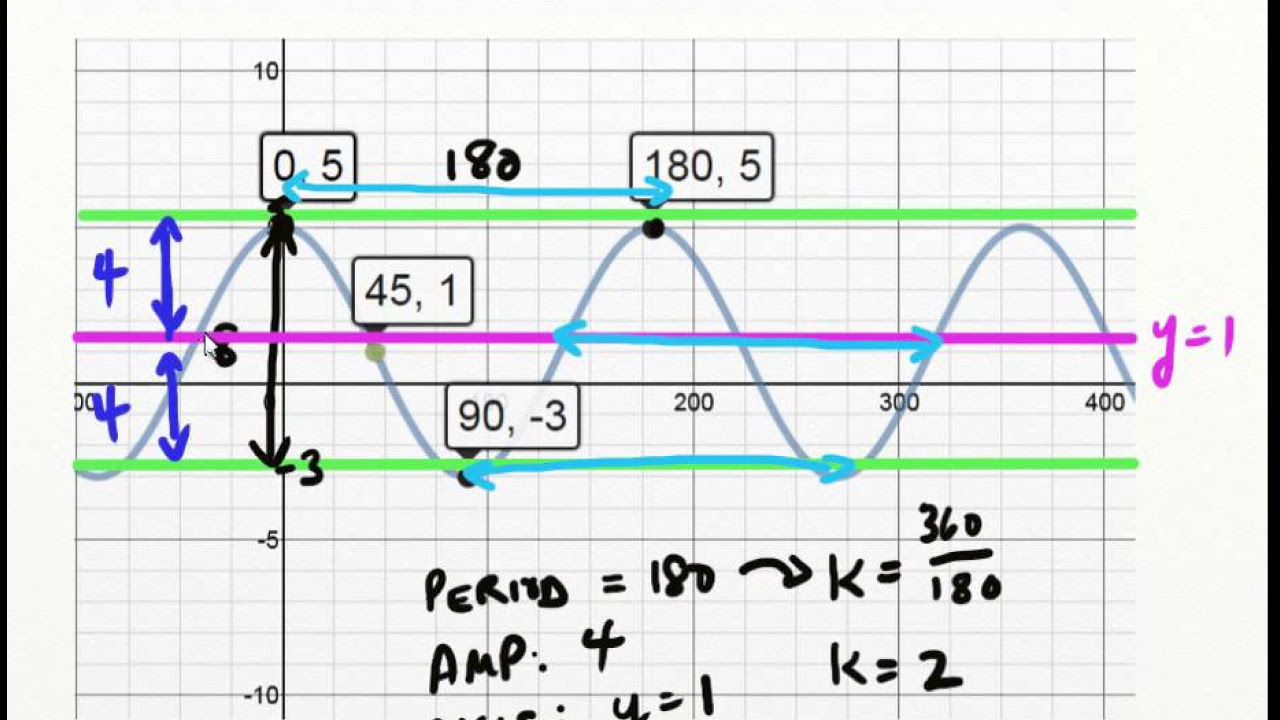
MCR3U UNIT 5 Writing Sine Cosine Equation Given Graph

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
TrigonometryGraph AnalysisAmplitudePhase ShiftCosine GraphSine GraphMidlineFrequencyTrig FunctionsMathematicsEducational