Equations of Motion Sums

Manocha Academy
11 Feb 202343:15
EducationalLearning
32 Likes 10 Comments

TLDRThis engaging physics class dives into the equations of motion, explaining their significance and usage. The instructor recaps the three key equations and demonstrates how to select the correct one for solving problems. Key concepts such as speed, velocity, and acceleration are clarified, with practical examples and tips provided. The video also highlights the importance of understanding units and the difference between accelerated and constant motion. Additionally, the class offers useful tips for students, including converting units and breaking down complex problems. Viewers are encouraged to explore more courses in various subjects offered by the academy.

Takeaways
  • 📚 The class focuses on equations of motion, an important topic in physics, and provides a recap of the three fundamental equations.
  • 🔍 The instructor emphasizes the importance of selecting the correct equation for solving motion problems based on the presence of acceleration.
  • 📉 The difference between speed and velocity is clarified, with speed being a scalar and velocity a vector due to its directional component.
  • ⏱ The formulas for average speed and average velocity are introduced, highlighting their use in calculating the overall rate of motion over a journey.
  • 🔢 A special formula for average velocity in uniformly accelerated motion is presented, which is the average of initial and final velocities.
  • 🧭 The symbols in the equations of motion (U for initial velocity, V for final velocity, a for acceleration, and s for displacement) are defined to avoid common mistakes.
  • 🌍 The value of acceleration due to gravity (g) is discussed, with the standard value being approximately 9.8 m/s², often rounded to 10 m/s² for simplicity.
  • 🔄 The concept of acceleration as both positive (acceleration) and negative (retardation) is explained, affecting how the equations are applied.
  • 📝 The instructor advises writing down the equations of motion to build muscle memory, facilitating recall during exams.
  • 📉 The application of the equations of motion is demonstrated through various problems, such as finding the speed of a car after a minute of acceleration and the time for a ball to fall from a height.
  • 🔑 The importance of unit consistency is stressed, particularly when converting from km/h to m/s for velocities, to ensure accurate calculations.
Q & A

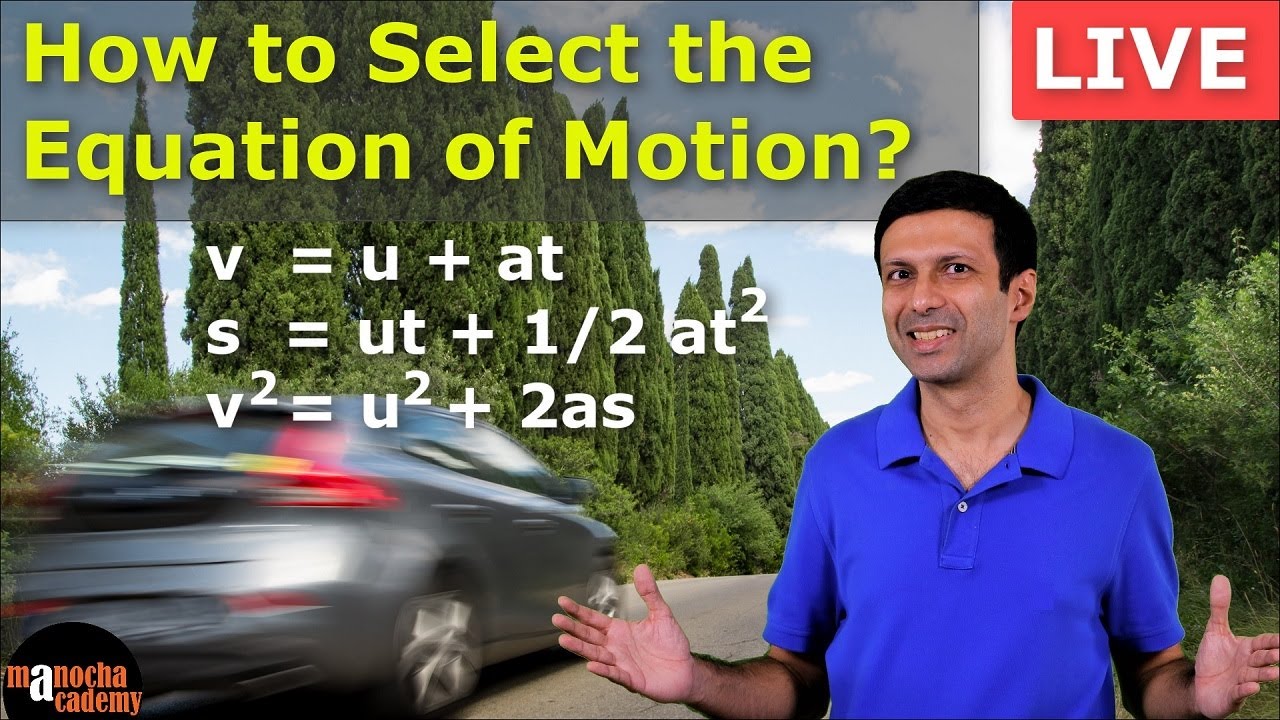
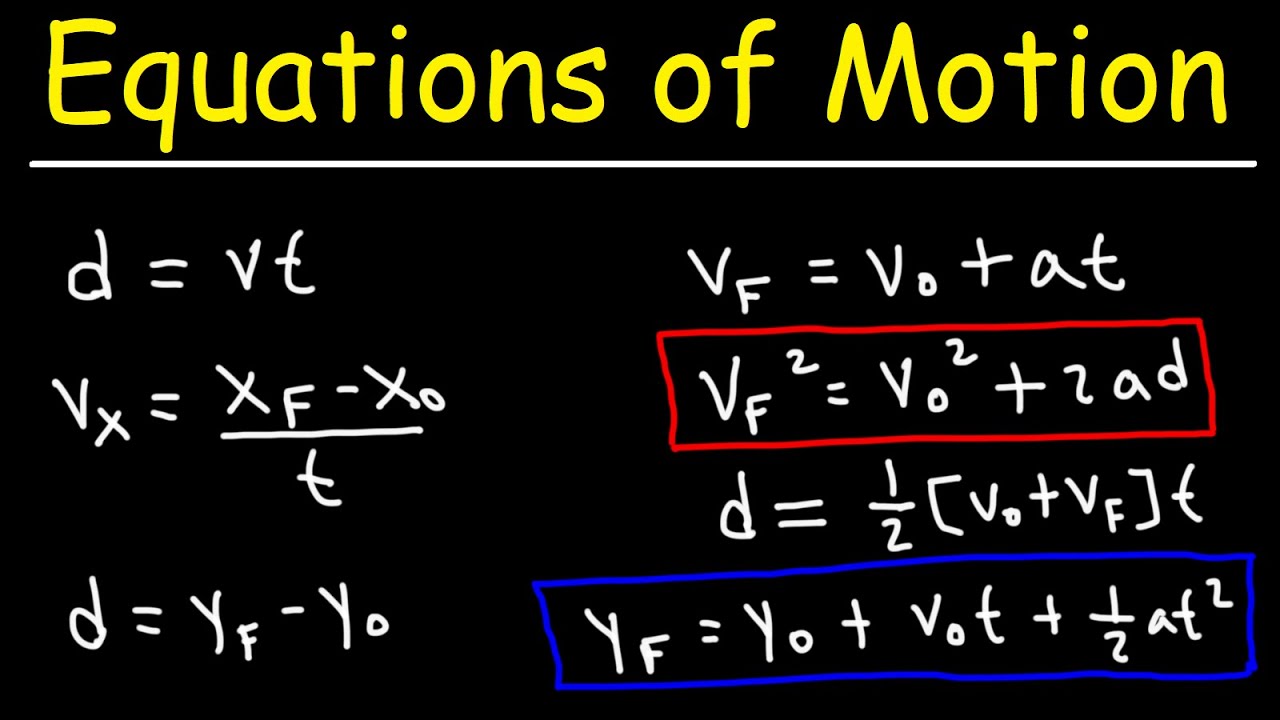
  • What are the three important equations of motion discussed in the class?

    -The three important equations of motion discussed are: 1) V = U + at, which relates final velocity (V), initial velocity (U), acceleration (a), and time (t). 2) s = ut + (1/2)at^2, which includes displacement (s), initial velocity (U), acceleration (a), and time (t). 3) V^2 = U^2 + 2as, which connects final velocity squared, initial velocity, acceleration, and displacement.

  • What is the difference between speed and velocity?

    -Speed is a scalar quantity that represents the rate of change of distance with time, without any direction, while velocity is a vector quantity that includes both speed and direction, calculated as displacement divided by time.

  • What is the formula for average speed?

    -The formula for average speed is the total distance traveled divided by the total time taken, which can be represented as 'Total Distance / Total Time'.

  • How is average velocity calculated when there is uniform acceleration or deceleration?

    -When there is uniform acceleration or deceleration, the average velocity is calculated as the average of the initial and final velocities, which is (U + V) / 2, where U is the initial velocity and V is the final velocity.

  • What is the unit of acceleration in the SI system?

    -In the SI system, the unit of acceleration is meters per second squared (m/s^2).

  • What is the standard value of acceleration due to gravity on Earth?

    -The standard value of acceleration due to gravity on Earth is approximately 9.8 m/s^2, often approximated to 10 m/s^2 for simplicity.

  • How do you find the final velocity of a car that starts from rest and has a uniform acceleration of 2 m/s^2 for one minute?

    -Using the first equation of motion (V = U + at), with U = 0 (since the car starts from rest), a = 2 m/s^2, and t = 60 seconds (1 minute), the final velocity V is calculated as 0 + 2 * 60 = 120 m/s.

  • What is the time taken for a ball to hit the ground when dropped from the top of an 80-meter high building?

    -Using the second equation of motion (s = ut + (1/2)at^2) with U = 0, s = 80 meters, and a = 10 m/s^2, solving for t gives t = √(2s/a) = √(2 * 80 / 10) = √16 = 4 seconds.

  • What is the retardation of a motorbike traveling at 72 km/h that reduces its speed to 36 km/h after a distance of 10 meters?

    -First, convert the velocities to m/s (72 km/h to 20 m/s and 36 km/h to 10 m/s). Then, use the third equation of motion (V^2 = U^2 + 2as) to find the acceleration a, which turns out to be -15 m/s^2, indicating a retardation of 15 m/s^2.

  • How much time does it take for a ball thrown upwards with a velocity of 5 m/s to come back to the thrower?

    -The time taken for the ball to go up and come back down is symmetrical. The time taken to go up is calculated using the first equation of motion, resulting in 0.5 seconds for the upward motion. Since the total time is the sum of upward and downward motion times, the total time is 0.5 seconds + 0.5 seconds = 1 second.

  • What speed should a ball be thrown upwards to reach a maximum height of 10 meters?

    -Using the third equation of motion (V^2 = U^2 + 2as) with V = 0 (at the maximum height), s = 10 meters, and a = -10 m/s^2 (since it's an upward motion and gravity acts downward), solving for U gives U = √(-2as) = √(-2 * -10 * 10) = 10√2 ≈ 14.14 m/s.

  • How much distance does a ball dropped from the top of a tall tower travel in the third second?

    -To find the distance traveled in the third second, calculate the total distance traveled in 3 seconds (S3) and in 2 seconds (S2) using the formula s = ut + (1/2)at^2, then subtract S2 from S3. The result is 25 meters.

Outlines
00:00
📚 Physics Class Introduction and Course Promotion

The script begins with a warm welcome to a physics class focused on equations of motion, an interesting topic that was previously introduced in another video. The instructor encourages students to review the foundational equations of motion and promises to offer tips for selecting and solving them effectively. Additionally, there's a promotional segment for other courses available on the website, including subjects like physics, chemistry, biology, and math for various grade levels. The promotion extends to coding courses in Python and Java, as well as specific offerings for the Cambridge IGCSE curriculum. The instructor also reminds students to subscribe to their YouTube channel and follow on social media for continued engagement with Manocha Academy.

05:02
🔍 Clarifying Speed, Velocity, and Equations of Motion

This paragraph delves into the distinction between speed and velocity, defining speed as a scalar quantity without direction and velocity as a vector that includes direction. The formulas for calculating both are presented, emphasizing the importance of understanding average speed and velocity, especially in variable motion scenarios. A special formula for average velocity in uniformly accelerated motion is introduced, followed by a detailed explanation of the three fundamental equations of motion. The paragraph also discusses the appropriate contexts for using these equations.

Mindmap
Keywords
💡Equations of Motion
Equations of motion are mathematical formulas that describe the relationship between an object's velocity, acceleration, time, and displacement. They are crucial in physics for solving problems involving motion. In the video, the instructor recaps the three main equations of motion and demonstrates how to apply them to different problems.
💡Acceleration
Acceleration is the rate at which an object's velocity changes over time. It can be positive (speeding up) or negative (slowing down), also known as retardation. The video explains how to identify and use acceleration in various motion equations and scenarios, such as a car accelerating from rest or a ball falling due to gravity.
💡Velocity
Velocity is the speed of an object in a given direction. It is a vector quantity, meaning it has both magnitude and direction. The video differentiates between velocity and speed, and explains how to calculate average velocity, particularly when an object is accelerating uniformly.
💡Displacement
Displacement is the shortest distance from the initial to the final position of an object, taking into account direction. It differs from distance, which measures the total path traveled regardless of direction. The video uses displacement in the context of equations of motion, such as when a ball is dropped from a height.
💡Uniform Acceleration
Uniform acceleration occurs when an object's velocity changes at a constant rate. This concept is key in solving problems with the equations of motion. The video provides examples, such as a car accelerating uniformly or a ball falling under gravity, to illustrate how to apply the equations in these cases.
💡Scalar and Vector Quantities
Scalars are quantities that have only magnitude, such as speed and distance. Vectors have both magnitude and direction, such as velocity and displacement. The video explains these concepts to help distinguish between terms like speed (scalar) and velocity (vector), and their relevance in equations of motion.
💡Retardation
Retardation, or negative acceleration, refers to the decrease in velocity over time. The video highlights this concept when discussing scenarios like a car slowing down after brakes are applied, and how to use the equations of motion to find retardation.
💡Free Fall
Free fall describes the motion of an object falling solely under the influence of gravity, with no other forces acting on it. The video uses examples of free-falling bodies to explain how to calculate displacement, velocity, and time using the equations of motion, considering the acceleration due to gravity.
💡SI Units
SI units (International System of Units) are standard units of measurement used in scientific calculations, such as meters for distance, seconds for time, and meters per second squared for acceleration. The video emphasizes the importance of converting all measurements to SI units to ensure accuracy in solving equations of motion.
💡Average Velocity
Average velocity is the total displacement divided by the total time taken. It is used when the velocity changes over time, providing a single value that represents the overall effect of the motion. The video explains how to calculate average velocity in cases of uniform acceleration and provides a specific formula for it.
Highlights

Introduction to the physics class focusing on equations of motion and tips for selecting the correct equation.

Recap of the three important equations of motion and their application in solving problems.

Explanation of the difference between speed and velocity, including their formulas and scalar/vector nature.

Discussion on average speed and velocity, including the special formula for average velocity with uniform acceleration.

Clarification on when to use equations of motion versus other formulas, based on the presence of acceleration.

Description of symbols used in equations of motion: U (initial velocity), V (final velocity), a (acceleration), and s (displacement).

Illustration of how to use the equations of motion to find an unknown variable when three others are given.

Review of SI units for velocity, time, acceleration, and displacement in the context of equations of motion.

Advice on learning and memorizing the equations of motion through writing and practice rather than passive reading.

Explanation of the value of acceleration for a freely falling body and its standard value (G).

Differentiation between the acceleration of a freely falling body and a body thrown upwards.

Emphasis on understanding acceleration as both positive (acceleration) and negative (retardation).

Walkthrough of a problem involving a car starting from rest with uniform acceleration to find its speed after one minute.

Solution of a problem calculating the time for a ball to hit the ground when dropped from a building.

Method to find the retardation of a motorbike that reduces its speed after brakes are applied.

Approach to calculate the time for a ball thrown upwards to return to the thrower.

Process to determine the speed required to throw a ball upwards to reach a specific maximum height.

Strategy to find the distance a ball travels in the third second when dropped from a tall tower.

Encouragement to explore other courses offered on the website, including subjects for CBSE and programming languages.

Transcripts

Browse More Related Video

AP Physics 1: Kinematics Review

Equation of Motion : How to Select the Right Equation?

College Physics 1: Lecture 10 - Solving 1-D Motion Problems

Equations of Motion

AP Physics 1 | Unit 1: Kinematics

Balancing Equations Made Easy

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Physics EducationMotion EquationsLearning TipsEducational ContentClassroom RecapSpeed CalculationVelocity AnalysisAcceleration ConceptsProblem SolvingScience Tutorials