Equations of Motion
TLDRThe video script discusses the fundamentals of motion equations, differentiating between constant speed and constant acceleration scenarios. It introduces key formulas such as distance (D = VT) for constant speed, and displacement, velocity, and acceleration calculations for constant acceleration, including the kinematic equations. The importance of understanding vectors like velocity versus scalars like speed is emphasized, as well as the practical application of these concepts in solving motion problems. The script also briefly touches on calculus applications for deriving velocity and position functions from acceleration.
Takeaways
- 📐 Identify the type of motion: constant speed or constant acceleration when solving motion problems.
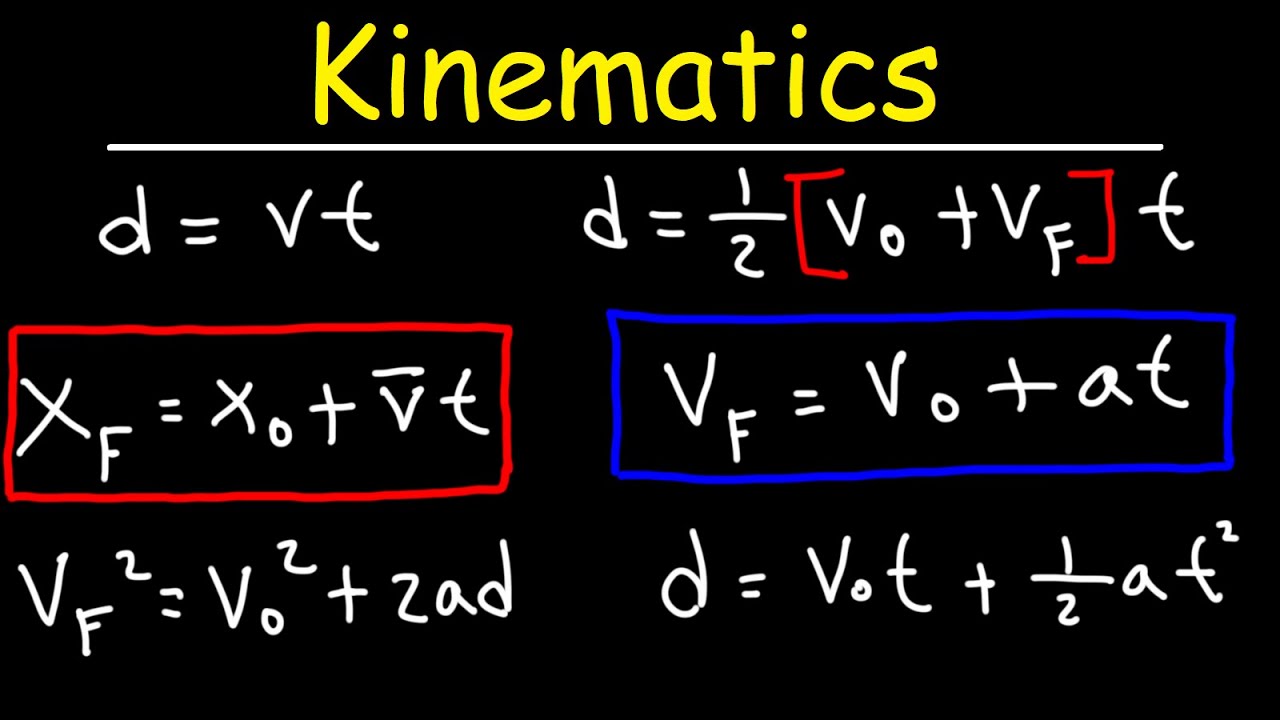
- 🏃 For constant speed motion, use the equation D = VT (distance or displacement equals velocity times time).
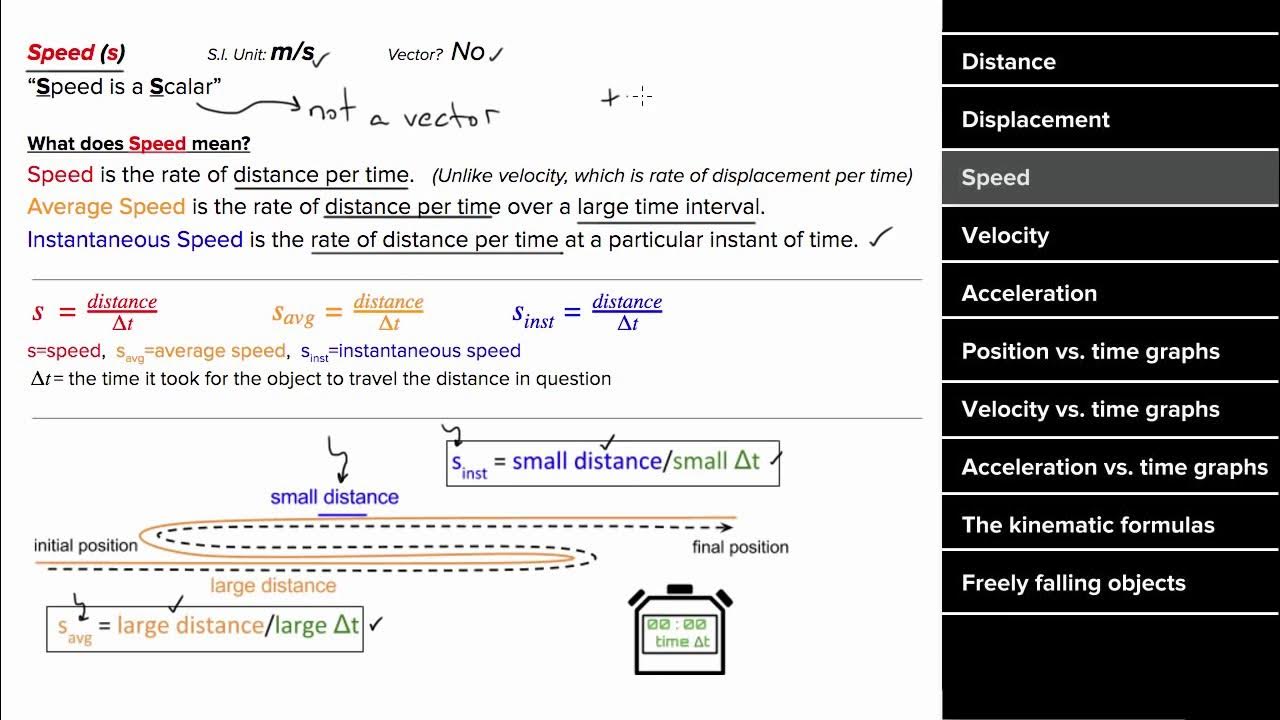
- 🔄 Understand the difference between speed and velocity: speed is a scalar and always positive; velocity is a vector with both magnitude and direction.
- 📊 Distance is a scalar quantity, always positive, and represents the total path length traveled.
- 🎯 Displacement is a vector quantity, representing the change in position, and can be positive or negative.
- 🚀 Calculate velocity in a specific direction by using the change in position (final minus initial) divided by time.
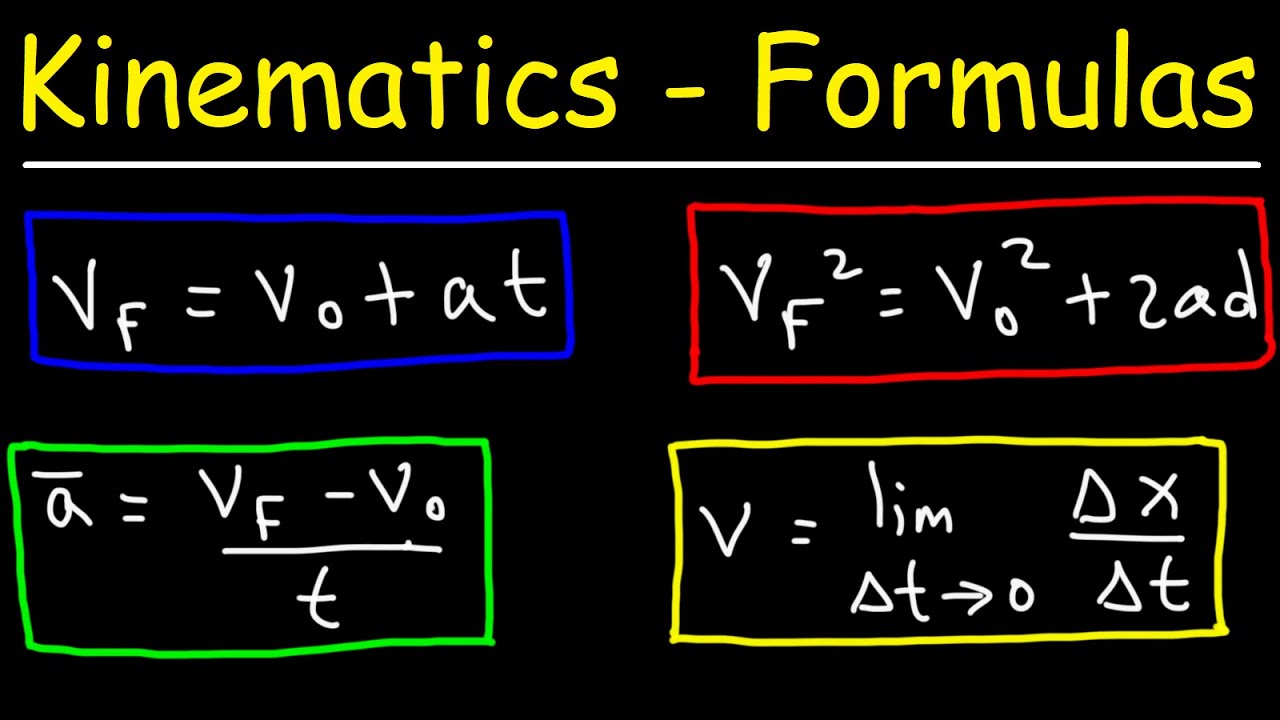
- 📈 For constant acceleration, acceleration is the change in velocity over time (ΔV/ΔT) and is the rate of change of velocity.
- 🌟 The formula V² = U² + 2ad is used for constant acceleration motion, where V is the final velocity, U is the initial velocity, a is acceleration, and d is displacement.
- 📌 Use the average velocity (initial + final / 2) when calculating displacement with constant acceleration.
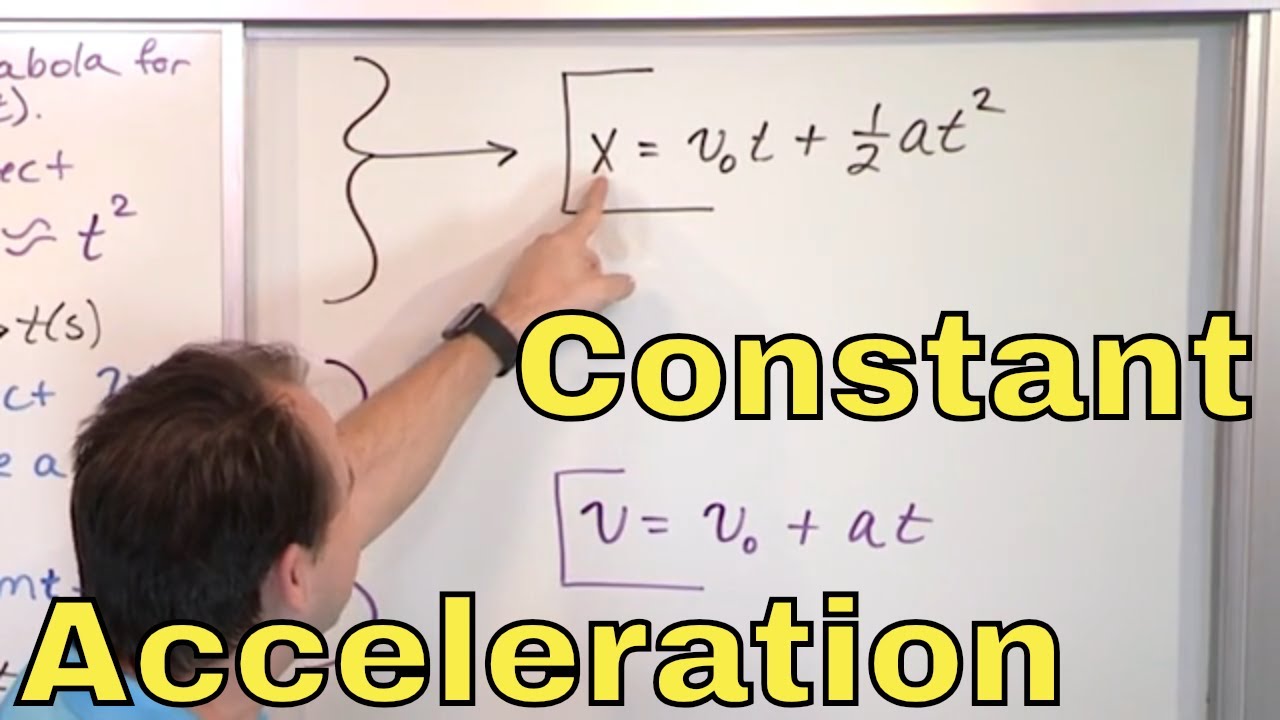
- 📐 Displacement can also be calculated using the formula: initial position + (initial velocity × time) + (1/2 × acceleration × time²).
- 🧮 For calculus-related problems, the derivative of position gives velocity, and the derivative of velocity gives acceleration; the antiderivative of acceleration gives velocity, and the integral of velocity gives position.
Q & A
What is the primary distinction between constant speed and constant acceleration motion?
-Constant speed motion implies that an object moves at a steady pace without changing its velocity, whereas constant acceleration motion involves a constant rate of change of velocity over time.
How is distance calculated when an object is moving at a constant speed?
-Distance (D) is calculated using the formula D = VT, where V represents velocity and T represents time.
What is the difference between velocity and speed?
-Velocity is a vector quantity that includes both magnitude and direction, while speed is a scalar quantity that only considers magnitude without direction. Speed is always positive, but velocity can be positive or negative.
How do you calculate the total distance traveled by an object?
-Total distance is the sum of all the individual distances traveled, regardless of direction. It is always a positive value.
How is displacement different from distance?
-Displacement is a vector quantity that represents the change in position from the initial to the final point, taking into account the direction of travel. Distance, on the other hand, is a scalar quantity that measures the total path length traveled without regard to direction.
What is the formula for calculating average acceleration?
-Average acceleration (a) is calculated using the formula a = (V_final - V_initial) / T, where V_final is the final velocity, V_initial is the initial velocity, and T is the time interval.
How do you find the displacement when an object is under constant acceleration?
-Displacement (s) can be found using the formula s = (V_initial + V_final) / 2 * T or s = V_initial * t + 0.5 * a * t^2, where V_initial and V_final are the initial and final velocities, t is the time, and a is the acceleration.
What is the relationship between final velocity, initial velocity, and acceleration as expressed in one of the kinematic equations?
-The relationship is given by the equation V_final^2 = V_initial^2 + 2 * a * d, where V_final is the final velocity, V_initial is the initial velocity, a is the acceleration, and d is the displacement.
How can you determine the vertical displacement in a motion problem?
-The vertical displacement (dy) is calculated by finding the difference in the final and initial vertical positions (y_final - y_initial) and can be determined using the formula dy = V_initial * t + 0.5 * a * t^2 when considering the acceleration in the y-direction.
What is the significance of the derivative and integral in the context of kinematics?
-The derivative of the position function gives the velocity function, while the integral of the velocity function gives the position function. The antiderivative of the acceleration function is used to find the velocity function when given an acceleration equation.
Where can one find example problems to practice using these kinematic formulas?
-Example problems and additional resources can be found in the description section below the video, where links to various kinematics-related tutorials such as projectile motion and free fall problems are provided.
Outlines
📐 Fundamentals of Motion: Constant Speed and Acceleration
This paragraph introduces the basics of equations of motion, focusing on identifying whether an object is moving at a constant speed or constant acceleration. It explains the key equation for constant speed, D = VT, where D represents distance or displacement, V is velocity, and T is time. The difference between distance (a scalar quantity) and displacement (a vector quantity) is clarified, with examples provided to illustrate the concept of displacement as the change in position. The paragraph also distinguishes between speed, which is always positive, and velocity, which can be positive or negative due to its directional component. The calculation of velocity in both horizontal (X) and vertical (Y) directions is discussed, emphasizing the importance of direction in velocity calculations.
📚 Kinematic Formulas and Calculus Concepts for Motion Analysis
The second paragraph delves into the formulas required for understanding constant acceleration and provides practical examples for their application. It introduces the concept of average acceleration, represented as ΔV/ΔT, and explains how to rearrange the equation to solve for final velocity. The paragraph also presents the formula for displacement under constant acceleration, highlighting the use of average velocity. Additionally, it covers the application of these formulas in different scenarios, such as projectile motion and free fall. The paragraph concludes with an introduction to calculus concepts related to motion, including the derivatives and integrals of position, velocity, and acceleration functions, and the role of constants of integration in these calculations. Links to example problems and further resources are mentioned for those seeking additional practice.
Mindmap
Keywords
💡Equations of Motion
💡Constant Speed
💡Constant Acceleration
💡Displacement
💡Velocity
💡Acceleration
💡Kinematic Formulas
💡Projectile Motion
💡Free Fall
💡Calculus
💡Vector and Scalar Quantities
Highlights
The importance of identifying whether an object is moving at constant speed or constant acceleration when dealing with equations of motion.
The key equation for constant speed motion: D = VT, where D represents distance or displacement, V is velocity, and T is time.
The distinction between distance and displacement, with distance being a scalar quantity and displacement being a vector quantity.
The definition of speed as the absolute value of velocity, with speed being always positive and velocity having both magnitude and direction.
The calculation of total distance traveled as the sum of individual segments, always resulting in a positive value.
The calculation of displacement by considering the direction of movement, resulting in a value that can be positive or negative.
The method to calculate velocity in a specific direction by using the change in position (displacement) over time.
The definition of acceleration as the change in velocity over time, given by the formula ΔV = aΔT.
The rearrangement of the acceleration formula to find the final velocity, given initial velocity, acceleration, and time.
The relationship between final velocity squared and the initial velocity squared, acceleration, and displacement, expressed by the formula Vf² = Vi² + 2ad.
The use of average velocity in calculating displacement for constant acceleration scenarios, derived from the initial and final velocities.
The application of the displacement formula for constant acceleration in both the X and Y directions, relevant for Free Fall and projectile motion problems.
The introduction of calculus concepts for position, velocity, and acceleration functions, where the derivative of position gives velocity, and the derivative of velocity gives acceleration.
The method to obtain the velocity function from acceleration by taking the antiderivative of the acceleration function, plus the constant of integration.
The process to find the position function by integrating the velocity function and adding the constant C.
The availability of example problems for practicing the use of these equations, accessible through links in the description section.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: