Calculus AB Homework 3.6 Derivatives of Inverses
TLDRThis video tutorial walks through the process of finding derivatives of various functions, focusing on problems 56 to 70 from a homework assignment. It covers the application of natural logarithm and chain rule derivatives, the quotient rule, and derivatives of inverse functions. The script demonstrates step-by-step solutions, including simplifying complex derivatives and using the derivative properties of functions like arcsine and arctan. It also explains how to find the equation of a tangent line to a graph and the derivative of an inverse function, providing a comprehensive guide for calculus students.
Takeaways
- 📚 The video is a tutorial on calculating derivatives of various functions, specifically focusing on problems 56 through 70.
- 🔍 The presenter uses the natural logarithm rule to find the derivative of a function in the form of the natural log of an expression.
- 📐 The chain rule is applied multiple times for functions that have inner functions, such as e^{2x} + 1 and \sqrt{5x - 2}.
- 📝 The process of finding derivatives involves identifying 'inside' and 'outside' functions, and then applying the appropriate rules to find the derivative of the composite function.
- 🔑 The quotient rule is used for functions that are the ratio of two other functions, like \ln(x) / (x - 2).
- 🧭 The video demonstrates the use of the inverse function's derivative, which is found by taking the reciprocal of the derivative of the original function.
- 📉 The script includes examples of finding the equation of a tangent line to a function, which requires both the slope and the point of tangency.
- 📈 The normal line to a function is also discussed, which has a slope that is the negative reciprocal of the tangent line's slope.
- 📌 The video provides a method for finding the derivative of an inverse function using a three-step process: finding the input that results in the desired output, evaluating the original function's derivative at that input, and taking the reciprocal of that value.
- 📚 The script covers a range of mathematical concepts, including the derivative of \arcsin(x), \arctan(x), and \sinh(x), using the chain rule.
- 📝 The process of finding derivatives for composite functions, inverse functions, and functions involving trigonometric and exponential components is thoroughly explained.
Q & A
What is the derivative of the natural log of a function in the form of ln(stuff)?
-The derivative of the natural log of a function in the form of ln(stuff) is 1/stuff * derivative of stuff.
How is the derivative of e^(2x) + 1 found in the script?
-The derivative of e^(2x) + 1 is found using the chain rule, resulting in e^(2x) * 2.
What is the process for finding the derivative of a function involving a natural log and an exponent, as shown in the script?
-The process involves identifying the inner function and the outer function, using the chain rule to find the derivative of the inner function, and then multiplying by the derivative of the outer function with respect to the inner function.
What is the derivative of ln(3 - 2x)?
-The derivative of ln(3 - 2x) is -2 / (3 - 2x), using the quotient rule for derivatives.
How is the derivative of the square root of 5x - 2 simplified in the script?
-The derivative of the square root of 5x - 2 is simplified to 5 / (2 * sqrt(5x - 2)) by using the chain rule and simplifying the expression.
What is the quotient rule used for finding the derivative of a function?
-The quotient rule is used for finding the derivative of a function that is a quotient of two other functions, and it involves multiplying the derivative of the numerator by the denominator and subtracting the product of the numerator and the derivative of the denominator, all divided by the square of the denominator.
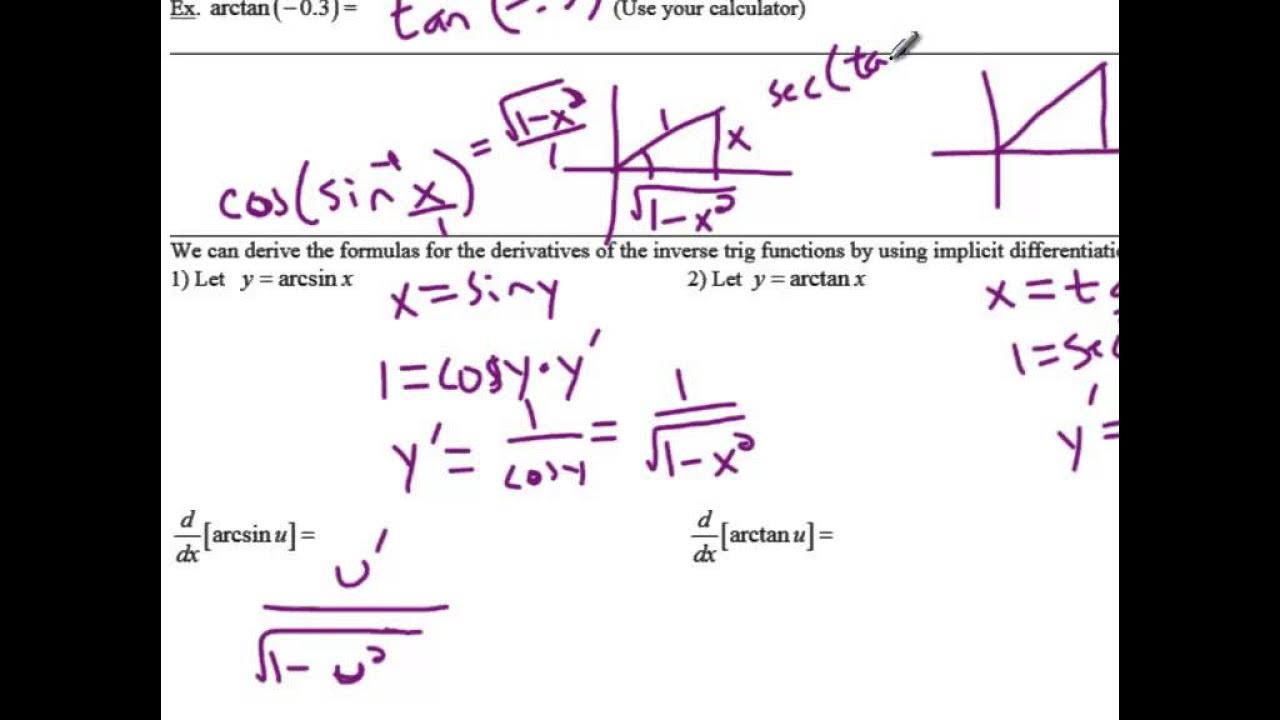
What is the derivative of arcsine of 5x in the script?
-The derivative of arcsine of 5x is 5 / sqrt(1 - 25x^2), using the chain rule and the derivative formula for arcsine.
How is the normal line to the graph of a function found in the script?
-The normal line to the graph of a function is found by using the derivative of the function to find the slope of the tangent line at a given point and then taking the negative reciprocal of this slope for the normal line.
What is the process for finding the equation of the tangent line to the graph of an inverse function, as shown in the script?
-The process involves finding the value of the inverse function at a given point, using the derivative of the original function to find the slope of the tangent line at that point, and then using the point-slope form of a line to write the equation of the tangent line.
How is the derivative of a function involving a natural log and a cube root, as in the script, found?
-The derivative is found by using the product rule for the derivative of the function and then simplifying the expression, taking into account the derivative of the natural log and the cube root components.
What is the derivative of the function f(x) = x^(5/3) * e^(x^2) evaluated at x = e in the script?
-The derivative of the function f(x) = x^(5/3) * e^(x^2) evaluated at x = e is found to be (3/11)e, and the reciprocal of this value is the final answer.
Outlines
📚 Derivative of Natural Logarithm Functions
This paragraph discusses the process of finding the derivatives of functions involving natural logarithms. The presenter uses the natural log rule, which states that the derivative of the natural log of a function is 1 over the function times the derivative of the function inside the log. Examples include finding the derivative of ln(e^(2x) + 1), simplifying it using the chain rule, and explaining the process step by step. The paragraph also covers other functions like ln(3 - 2x) and ln(√(5x - 2)), each with a detailed explanation of the derivative calculation.
🔍 Chain Rule Application in Derivatives
The paragraph delves into the application of the chain rule for finding derivatives of composite functions. It provides a step-by-step breakdown of how to identify the inner and outer functions, and how to apply the chain rule to calculate the derivative of the natural log of a square root function. The explanation includes simplifying the derivative and using the long form of the chain rule to ensure clarity. The paragraph also touches on the derivative of a quotient of functions and the process of simplifying complex fractions.
📉 Derivatives of Inverse Trigonometric Functions
This section focuses on the derivatives of inverse trigonometric functions, specifically arcsine and arctan. The presenter explains the derivative of arcsin(5x) by applying the chain rule and emphasizes the importance of the derivative of the inside function and the outside function. The explanation includes the simplification of the resulting expression. The paragraph also covers the derivative of arctan(1 - x), illustrating the process with a clear example and the final simplified form of the derivative.
🤔 Derivative of Tangent Inverse and Inverse Functions
The paragraph discusses the process of finding the derivative of the tangent inverse function and touches on the concept of differentiating inverse functions in general. It provides an example of finding the derivative of tan inverse (3/x) using the quotient rule and the power rule, leading to the simplified form of the derivative. The explanation also includes a three-step process for finding the derivative of an inverse function at a given point, which involves finding the original function's value, its derivative, and then taking the reciprocal of the derivative.
📝 Derivatives and Equations of Tangent Lines
This section covers the process of finding the derivatives of functions and using them to determine the equations of tangent lines. It includes examples of finding the tangent line to the graph of an inverse function at a specific point, using the derivative to find the slope of the tangent line and the function value to find the point of tangency. The explanation involves setting up the equation of the tangent line and simplifying it to the final form. The paragraph also discusses finding the equation of the normal line to a graph, which involves using the negative reciprocal of the slope of the tangent line.
🔢 Solving for Inverse Functions and Their Derivatives
The paragraph explains the method of finding the value of an inverse function at a specific point and then using that to determine the derivative of the inverse function. It provides a detailed example of finding F inverse prime of 2, which involves solving for the original function's value, evaluating the derivative at that point, and taking the reciprocal. The explanation also includes finding the equation of a tangent line to the graph of an inverse function at a given x-value, using the derivative and the function value to establish the slope and point of tangency.
📌 Derivatives of Composite Functions Involving Inverses
This section discusses the process of finding the derivatives of composite functions that involve inverse functions. It provides examples of setting up equations to find the value of the inverse function at a specific point and then using that to find the derivative of the inverse function. The explanation includes the use of the chain rule to find the derivative of the composite function and the process of simplifying the resulting expression to find the derivative at a given point.
📘 Derivative Calculations for Advanced Functions
The paragraph covers the process of finding derivatives for more complex functions, including those involving exponential and logarithmic components. It provides a detailed example of finding the derivative of a function that combines an exponential term with a logarithmic term, using the product rule and the chain rule. The explanation includes simplifying the derivative and finding the reciprocal to get the final answer.
📌 Final Derivative Calculations and Simplifications
This final paragraph wraps up the series of derivative calculations with a few more examples of finding derivatives of complex functions. It includes functions with exponential and inverse terms, where the presenter demonstrates the process of taking derivatives using the quotient rule and the chain rule. The explanation involves simplifying the derivatives and finding the reciprocal to get the final answers, showcasing the application of calculus concepts in solving these problems.
Mindmap
Keywords
💡Derivative
💡Natural Logarithm
💡Chain Rule
💡Quotient Rule
💡Arcsine
💡Arctangent
💡Inverse Functions
💡Tangent Line
💡Power Rule
💡Product Rule
💡Quotient Rule
Highlights
Derivative of natural log function using the natural log rule.
Use of the chain rule for derivatives of exponential functions.
Simplification of derivatives by rewriting as a fraction.
Application of the quotient rule for functions divided by other functions.
Derivative of arcsine function using the chain rule.
Derivative of arctan function with the chain rule.
Finding the derivative of sine inverse function with the chain rule.
Derivative of tangent inverse function using quotient rule and power rule.
Three-step process to find the derivative of an inverse function.
Finding the equation of a tangent line using the derivative.
Using the reciprocal of a derivative to find the slope of a normal line.
Solving for the inverse function value using a table of function values.
Finding the derivative of an inverse function using the reciprocal of the original function's derivative.
Using product rule and chain rule to find derivatives of composite functions.
Solving for the value of x that makes a function equal to a given number.
Evaluating the derivative of a function at a specific point.
Finding the derivative of a quotient of two functions using quotient rule.
Using the reciprocal of a derivative to find the derivative of an inverse function.
Solving complex derivative problems by breaking them down into simpler steps.
Transcripts
Browse More Related Video

Calculus AB Homework 3.3 The Quotient Rule
Inverse Trig Functions and Differentiation

AP Calculus AB: Lesson 3.5 Derivatives of Inverse Functions
Derivative of Logarithmic Functions

AP Calculus AB and BC Unit 3 Review [Differentiation: Composite, Implicit, and Inverse Functions]

Calculus AB Homework 3.2 The Product Rule
5.0 / 5 (0 votes)
Thanks for rating: