AP Calculus AB: Lesson 3.5 Derivatives of Inverse Functions

Michelle Krummel

5 Nov 202044:04

EducationalLearning

32 Likes 10 Comments

TLDRThis educational video script delves into the process of finding derivatives of inverse functions, focusing on natural logarithms, exponential functions, and inverse trigonometric functions. It explains step-by-step methods, including the use of the chain rule and properties of logarithms, to derive formulas for derivatives like ln(x) and arctan(x). The script also covers general strategies for differentiating inverse functions and provides examples to illustrate the concepts, aiming to solidify the viewer's understanding of these calculus principles.

Takeaways

📚 The video teaches how to find the derivative of an inverse function, focusing on natural logarithm and exponential functions, as well as inverse trigonometric functions.
🔑 To derive the natural logarithm function, the video demonstrates exponentiating both sides and using logarithmic properties to simplify the expression.
🧭 The derivative of the natural logarithm function, g'(x), is found to be 1/x, which is a key formula to memorize.
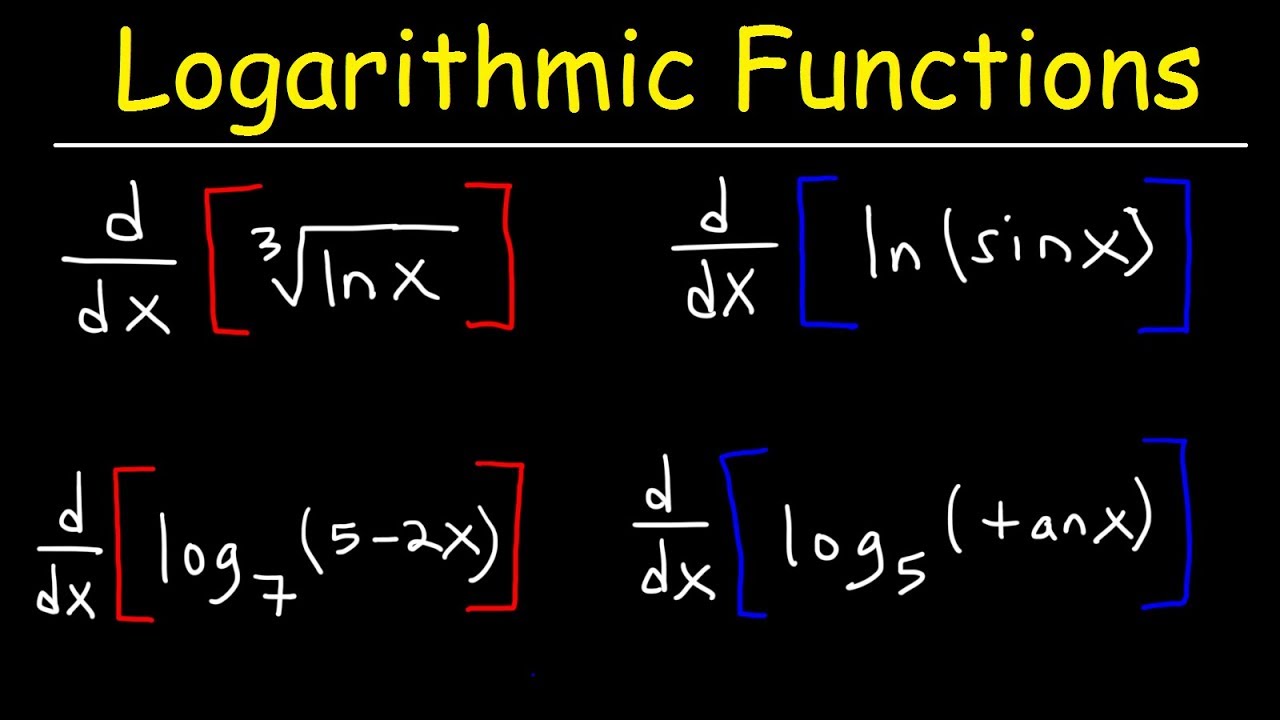
🔄 The chain rule is essential for finding the derivative of the natural logarithm of a composite function, resulting in 1/u * du/dx.
📉 The video provides examples of derivatives involving the natural logarithm, such as ln(t^2 + 1) and ln(1 - x), emphasizing the use of the chain rule.
🏗️ The process of finding the derivative of inverse trigonometric functions, like arcsine and arctangent, involves rewriting the function and applying the chain rule.
📌 The derivative of the arcsine function, d/dx(sin^-1(x)), is derived to be 1/sqrt(1 - x^2).
📐 Similarly, the derivative of the arctangent function, d/dx(tan^-1(x)), is found to be 1/(1 + x^2).
🔢 The video also covers the general method for finding the derivative of any inverse function, which involves the relationship between the function and its inverse, and the application of the chain rule.
📝 The final part of the video script includes practice problems that apply the concepts learned, such as finding the derivative of composite functions involving both elementary and inverse trigonometric functions.
📉 The video concludes with a summary of the rules for the derivatives of inverse sine and inverse tangent functions, which are crucial for solving related calculus problems.

Q & A

What is the main topic of the video?
-The main topic of the video is to teach how to find the derivative of an inverse function, covering various types of inverse functions and the process of deriving their formulas.
What is the first inverse function considered in the video?
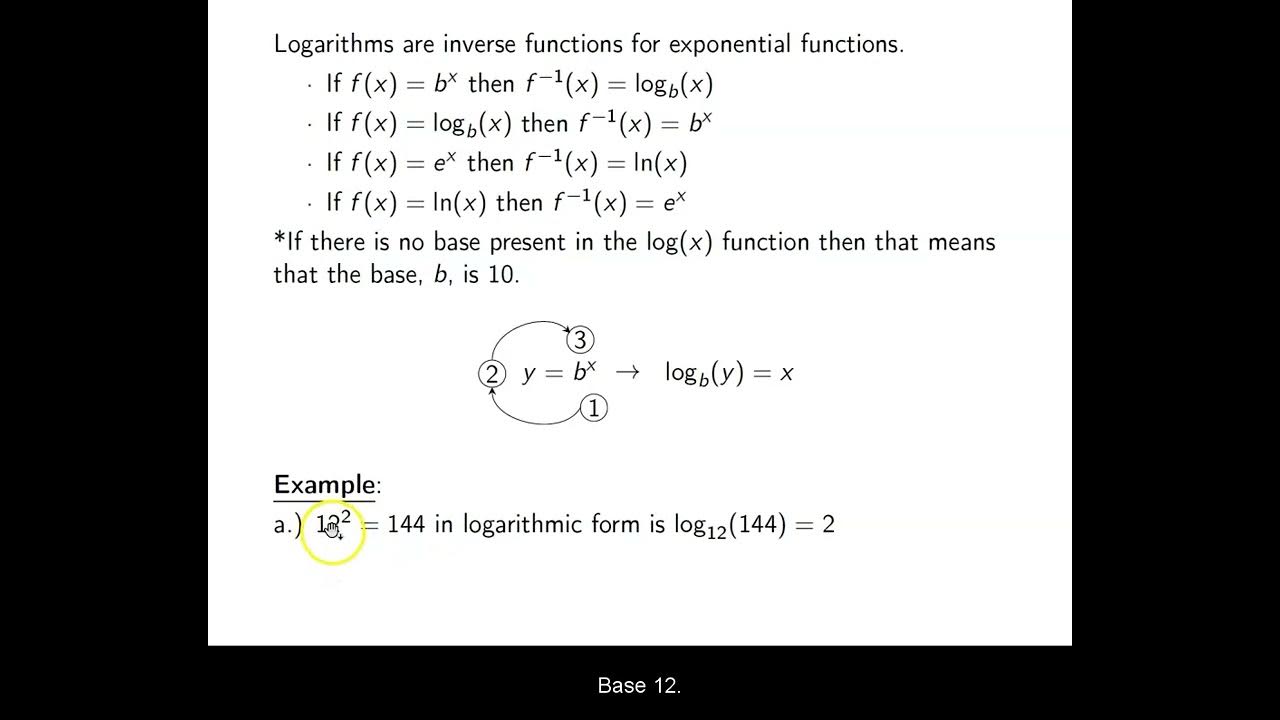
-The first inverse function considered in the video is g(x) = ln(x), which is the inverse of y = e^x.
How is the derivative of ln(x) derived in the video?
-The derivative of ln(x) is derived by exponentiating both sides of the equation e^(g(x)) = x to get e^(g(x)) = e^(ln(x)) which simplifies to x. Then, using the chain rule, the derivative g'(x) is found to be 1/x.
What is the general rule for finding the derivative of ln(u) with respect to x?
-The general rule for finding the derivative of ln(u) with respect to x is (1/u) * (du/dx), where u is a function of x.
How is the derivative of an inverse trigonometric function like arcsin(x) found?
-The derivative of an inverse trigonometric function like arcsin(x) is found by rewriting the function in its inverse form (x = sin(h(x))), then differentiating both sides using the chain rule, which results in the derivative being 1/√(1 - x^2).
What is the derivative of tan inverse x, also known as arctan x?
-The derivative of tan inverse x, or arctan x, is 1/(1 + x^2).
How can you find the derivative of a function that is a composition of other functions?
-To find the derivative of a composition of functions, you can use the chain rule, which involves differentiating the outer function and then multiplying by the derivative of the inner function.
What is the process for finding the derivative of an inverse function in general?
-The process for finding the derivative of an inverse function in general involves three steps: 1) Find the value of x for which the original function equals y (f(x) = y), 2) Substitute this value into the derivative of the original function (f'(x)), and 3) Take the reciprocal of the result.
How is the equation of the tangent line to the graph of a function at a certain point found?
-The equation of the tangent line to the graph of a function at a certain point is found using the point of tangency and the slope of the tangent line, which is the derivative of the function at that point. The equation is in the form y - y1 = m(x - x1), where m is the slope and (x1, y1) is the point of tangency.
What can be concluded about the graph of a function if the derivative at a certain point is negative?
-If the derivative of a function at a certain point is negative, it can be concluded that the function is decreasing at that point.

Outlines

00:00

📚 Introduction to Derivatives of Inverse Functions

This paragraph introduces the concept of finding the derivative of an inverse function. It begins with the natural logarithm function as an example and demonstrates the process of deriving its inverse function. The video script explains how to exponentiate both sides of the equation to simplify and then apply the chain rule to find the derivative of the natural logarithm, which is 1 over x. The paragraph also covers the general rule for differentiating the natural logarithm of a variable using the chain rule.

05:01

🔍 Derivatives of Composite Functions and Examples

This section delves into finding derivatives of composite functions, particularly focusing on the natural logarithm of a composite function. It explains the application of the chain rule for differentiating such functions and provides examples, including the derivative of the natural logarithm of a squared term plus one and the natural logarithm of one minus x. The paragraph also touches on the derivative of a constant, specifically the natural logarithm of pi, which is zero.

10:02

📘 Derivatives of Inverse Trigonometric Functions

The third paragraph explores the derivatives of inverse trigonometric functions, starting with the inverse sine function. It explains the process of deriving the formula for the derivative of the inverse sine function by using a right triangle and the Pythagorean theorem. The paragraph also covers the derivation of the inverse tangent function, again using the right triangle and its properties. The key takeaway is the memorization of the derivative rules for inverse sine and inverse tangent functions.

15:05

📌 Derivatives of Inverse Functions in General

This paragraph discusses the general process of finding the derivative of an inverse function. It explains the relationship between a function and its inverse and how to derive the formula for the derivative of an inverse function. The video script provides a clear three-step process: find the value of the inverse function at a given point, substitute this value into the original function's derivative, and then take the reciprocal to obtain the derivative of the inverse function.

20:11

📝 Applying the Derivative Rules to Specific Functions

The fifth paragraph applies the previously discussed derivative rules to specific functions, such as finding the derivative of a function that combines an exponential and a natural logarithm. It also covers the derivative of a function that involves an inverse tangent and a cubed term, demonstrating the use of the product, power, and chain rules in these scenarios.

25:11

📚 Estimating Derivatives and Analyzing Function Behavior

In the final paragraph, the script discusses estimating the value of a derivative at a specific point using the central difference method. It provides an example of estimating the derivative of a function at x equals negative one and concludes with an analysis of the function's behavior at that point, inferring that the function is decreasing based on the negative derivative.

Mindmap

Keywords

💡Derivative

A derivative in calculus is a measure of how a function changes as its input changes. It is the slope of the tangent line to the function's graph at a certain point. In the video, the derivative is the central theme, as it discusses how to find the derivative of various functions, including inverse functions, which is essential for understanding rates of change and the behavior of functions.

💡Inverse Function

An inverse function is a mathematical function that 'reverses' another function: if the function f applied to an input x gives a result of y, then applying its inverse function g to y gives the result x, i.e., g(f(x)) = x. The video focuses on finding the derivatives of inverse functions, such as the natural logarithm and trigonometric functions, which is crucial for understanding the relationship between the rates of change of a function and its inverse.

💡Natural Logarithm

The natural logarithm (ln) is the logarithm to the base e, where e is an irrational constant approximately equal to 2.71828. It is widely used in mathematics, especially in calculus. In the video, the natural logarithm is used as an example of an inverse function, and the process of finding its derivative, which is 1/x, is explained.

💡Chain Rule

The chain rule is a fundamental theorem in calculus for finding the derivative of a composite function. It states that the derivative of a function composed of two functions is the derivative of the outer function times the derivative of the inner function. The video script uses the chain rule to find derivatives of functions like ln(u) and sin^(-1)(x), demonstrating its importance in differentiating inverse functions.

💡Exponential Function

An exponential function is a function of the form f(x) = a * b^x, where a and b are constants and b > 0. These functions are used to model growth or decay processes. The video discusses the derivative of exponential functions, which is a key concept in understanding how these functions behave as their input changes.

💡Power Rule

The power rule is a basic principle in calculus that allows for the differentiation of polynomial functions. It states that if f(x) = x^n, then f'(x) = n * x^(n-1). The video mentions the power rule when differentiating x and x^2, which is essential for understanding the slope of power functions at any point.

💡Product Rule

The product rule is a formula used to find the derivative of a product of two functions. It states that the derivative of the product is the derivative of the first function times the second function plus the first function times the derivative of the second function. The video uses the product rule when differentiating functions like x^2 * ln(x), showcasing its application in more complex derivative problems.

💡Quotient Rule

The quotient rule is a method for finding the derivative of a quotient of two functions. It is used when differentiating a function that is divided by another function. The video script mentions the quotient rule in the context of differentiating a function like ln(t) / (e^t + 1), which is crucial for handling quotients in derivative problems.

💡Inverse Trigonometric Functions

Inverse trigonometric functions, such as arcsine (sin^(-1)(x)) and arctangent (tan^(-1)(x)), are the inverses of the basic trigonometric functions. They are used to find the angle when the ratio of the sides of a right triangle is known. The video explains how to find the derivatives of these functions, which is important for understanding the rates of change in trigonometric contexts.

💡Pythagorean Theorem

The Pythagorean theorem is a fundamental principle in geometry that states that in a right-angled triangle, the square of the length of the hypotenuse is equal to the sum of the squares of the lengths of the other two sides. In the video, the Pythagorean theorem is used to find the length of the missing side in a right triangle when two sides are known, which is necessary for calculating the derivatives of inverse trigonometric functions.

💡Differentiation Rules

Differentiation rules are the principles and formulas used to find the derivatives of functions. They include the power rule, product rule, quotient rule, and chain rule, among others. The video provides an overview of these rules and demonstrates their application in finding the derivatives of various types of functions, emphasizing their importance in calculus.

Highlights

Introduction to finding the derivative of an inverse function and its importance in calculus.

Explanation of the process to derive the formula for the derivative of the natural logarithm function.

Demonstration of exponentiation to simplify the natural logarithm function to its base form.

Use of the chain rule to find the derivative of an exponential function.

Isolation of the derivative g'(x) to find the derivative of the natural log function.

Derivation of the general rule for the derivative of the natural log function, 1/x.

Application of the chain rule to find the derivative of natural log of composite functions.

Examples of using the chain rule for functions involving natural log of squared terms and constants.

Differentiation of the natural log of a sum and difference of variables using the chain rule.

Clarification that the derivative of a constant is zero, using natural log of pi as an example.

Instruction to practice derivative problems for better understanding and skill development.

Use of multiple derivative rules for complex functions, such as product and quotient rules.

Derivation of the derivative for inverse trigonometric functions, specifically arcsine and arctan.

Explanation of the process to find the derivative of arcsine using a right triangle and Pythagorean theorem.

Derivation of the derivative for arctan, involving the use of tangent and secant squared.

General formula for the derivative of an inverse function, 1/f'(f^-1(x)).

Three-step process for finding the derivative of an inverse function at a specific point.

Examples demonstrating the application of the three-step process for various functions.

Estimation of the derivative of a function at a specific point using central difference.

Conclusion about the function's behavior at a certain point based on the sign of its derivative.

Summary of the lesson on derivatives of inverses and the importance of understanding the process.

Transcripts

Browse More Related Video

Derivative of Logarithmic Functions

Calculus AB Homework 3.6 Derivatives of Inverses

Derivatives of Exponential Functions

Derivatives of Inverse Functions

Ch. 4.3 Logarithmic Functions

Math1325 Lecture 11 1 Derivatives of Logarithm

Related Tags

Derivative Rules Inverse Functions Natural Log Trigonometry Chain Rule Calculus Tutorial Exponential Functions Educational Content Mathematics Learning Inverse Trigonometric