Lec 7: Exam 1 review | MIT 18.01 Single Variable Calculus, Fall 2007
TLDRThis lecture from an MIT OpenCourseWare series delves into the final remarks on exponents, focusing on the mathematical constant 'e' and its properties. The professor reviews the proof that the limit of (1 + 1/k)^k as k approaches infinity equals 'e', emphasizing the use of logarithms and the exponential function's inverse relationship. The lecture continues with a discussion on the natural logarithm's relevance in economics due to its use in ratios, which is more meaningful than absolute values in contexts like stock prices. The professor also covers the general and specific formulas for derivatives, including the chain rule, product rule, quotient rule, and the differentiation of exponential functions with base 'e'. Examples are provided to illustrate the application of these concepts, highlighting the importance of recognizing different perspectives in mathematical equations.
Takeaways
- 📚 The lecture is part of a series on exponents and derivatives from an MIT OpenCourseWare course, emphasizing the importance of understanding mathematical concepts and their applications.
- 📈 The professor reviews the limit of (1 + 1/k)^k as k approaches infinity, which converges to the mathematical constant e, and explains the proof with fewer symbols for clarity.
- 🧐 The class discusses the concept of taking the logarithm of an expression before finding its limit, a common technique when dealing with exponential functions, and how it leads to the conclusion that e^ln(a_k) = a_k.
- 🤔 The professor addresses a student's question about the log of a_k tending to 0, clarifying that a_k does not tend to 1 and explaining the balance between the parts of the expression that tend to 0 and infinity.
- 🔄 The lecture emphasizes the importance of reversing perspective in mathematics, showing that the limit definition of e can also be seen as a formula for e.
- 🌐 The professor introduces the concept of derivatives of powers, specifically for real numbers r, and demonstrates two methods for finding these derivatives: using base e and logarithmic differentiation.
- 📉 The natural logarithm is highlighted as particularly 'natural' for economics due to its use in calculating ratios, such as percentage changes in stock prices, which are more meaningful than absolute changes.
- 📝 The importance of understanding general formulas for derivatives, such as the sum rule, product rule, quotient rule, and chain rule, is stressed, as well as specific derivatives of functions like x^r, sine, cosine, and their inverses.
- 🔧 The chain rule is further explained with an example, showing how rates of change multiply when dealing with composite functions, and how it can simplify the process of differentiation.
- 📊 The professor provides several examples of differentiating complex functions using a combination of the rules discussed, such as differentiating e^(x tan^(-1) x) by applying the chain and product rules.
- ✏️ The lecture concludes with a review of Unit One, summarizing the general and specific formulas for derivatives, and the importance of understanding the geometric interpretation of derivatives as tangent lines.
Q & A
What is the significance of the number e in the context of this lecture?
-The number e is significant as it is the base of the natural logarithm and is defined as the limit of (1 + 1/k)^k as k approaches infinity. It is also the base for which the exponential function e^x has a slope of 1 at x = 0.
How does the professor explain the proof that the limit of a_k as k approaches infinity is e?
-The professor explains the proof by first taking the natural logarithm of a_k, which is k * ln(1 + 1/k), and showing that this limit tends to 1 as k approaches infinity. Then, by exponentiating this result, e^1, which is just e, and recognizing that this is equivalent to a_k, the proof is completed.
What is the purpose of reviewing the steps of the proof from the previous lecture?
-The purpose of reviewing the steps is to clarify the process and make the proof more understandable with fewer symbols, ensuring students grasp the concept and can follow the logical progression of the proof.
Why does the professor emphasize the property e^ln a = a?
-The professor emphasizes this property because it is fundamental to understanding the relationship between the exponential and logarithmic functions, which is crucial for the proof and for working with these functions in calculus.
What is the main concept behind logarithmic differentiation?
-Logarithmic differentiation is a method used to differentiate functions by taking the natural logarithm of both sides of an equation, which simplifies the differentiation process, especially for complex functions.
How does the professor demonstrate the use of base e in differentiation?
-The professor demonstrates it by rewriting x^r as (e^ln x)^r, which simplifies to e^(r ln x), and then applying the chain rule to differentiate it, resulting in r * x^(r-1).
What is the importance of understanding the chain rule in calculus?
-The chain rule is crucial in calculus as it allows for the differentiation of composite functions, which is a common task in calculus. It helps in finding the derivative of a function that is the result of multiple functions being composed.
Why does the professor suggest that the natural logarithm is 'natural' for economics?
-The natural logarithm is 'natural' for economics because it deals with ratios, which are more meaningful in economic contexts than absolute changes. For example, the change in stock prices is more relevant when expressed as a percentage of the original price.
What is the professor's advice on simplifying expressions during exams?
-The professor advises students not to try to simplify expressions unless instructed to do so. He suggests that students should focus on correctly applying the formulas and rules of differentiation, as simplifying is not always necessary and can sometimes complicate the answer.
How does the professor explain the process of deriving the derivative of the secant function?
-The professor explains that the derivative of the secant function can be derived by considering secant as the reciprocal of cosine, applying the chain rule, and then simplifying the expression to the standard form involving secant and tangent functions.
What is the main takeaway from the lecture regarding the application of derivatives?
-The main takeaway is that derivatives have multiple applications and interpretations, including understanding rates of change, analyzing the behavior of functions, and solving practical problems in fields like economics and navigation.
Outlines
📚 MIT OpenCourseWare Support and Exponents Review
The paragraph introduces the MIT OpenCourseWare initiative and its reliance on donations for providing free educational resources. It then transitions into a lecture on exponents, specifically revisiting the limit of (1 + 1/k)^k as k approaches infinity, which converges to the mathematical constant e. The professor clarifies the proof using logarithms and emphasizes the importance of understanding the steps and the philosophical approach to limits and derivatives.
🔍 Deep Dive into Exponential Functions and Derivatives
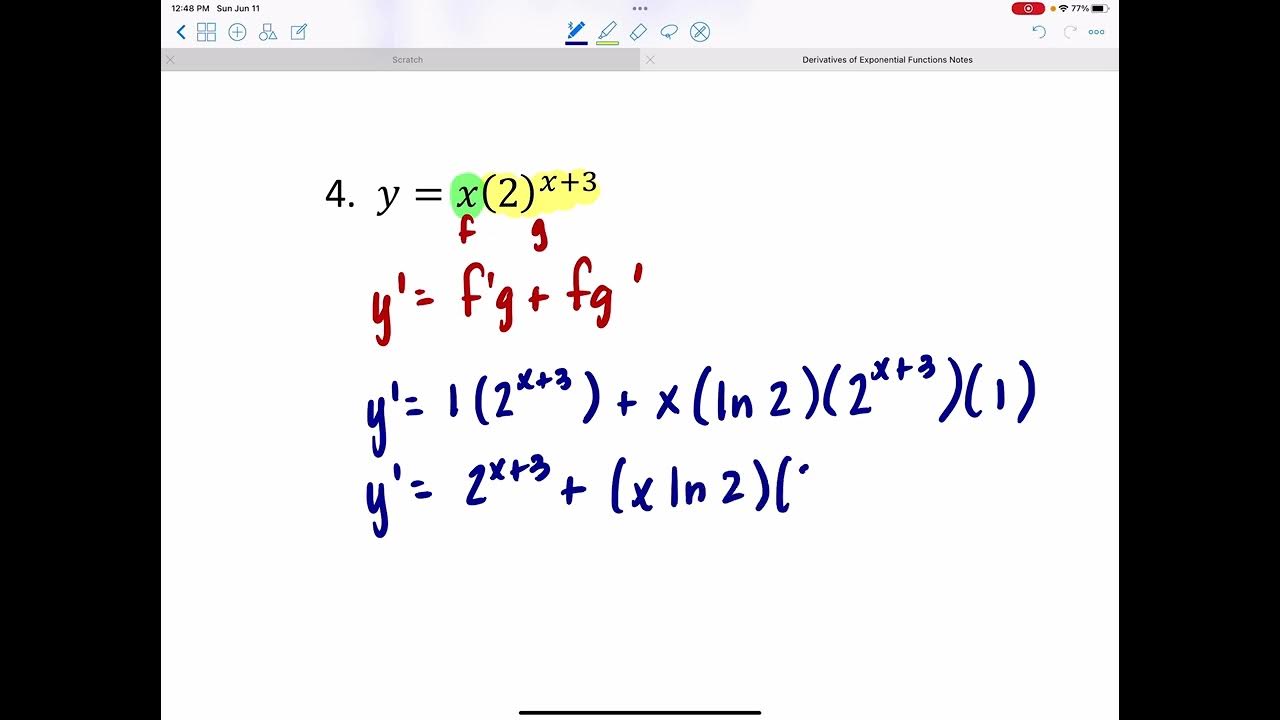
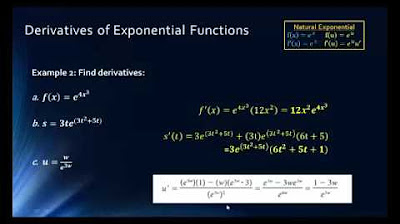
This section of the lecture focuses on the derivative of exponential functions, particularly the power rule for all real numbers r. Two methods are discussed: using base e and logarithmic differentiation. The professor demonstrates how to differentiate x^r using both methods, highlighting the chain rule and the properties of logarithmic differentiation. The importance of understanding different perspectives in calculus is emphasized.
📈 The Natural Logarithm's Role in Economics
The professor argues for the naturalness of the natural logarithm, especially in the context of economics. Using the example of stock prices, the discussion centers on the significance of ratios rather than absolute changes. The natural log is presented as the most straightforward and meaningful tool for such applications, as it simplifies expressions and calculations without introducing unnecessary complexity.
📘 Review of Derivatives and General Formulas
The lecture continues with a review of Unit One, covering general formulas for differentiation, such as the sum rule, product rule, quotient rule, and chain rule. The professor also touches on implicit differentiation and logarithmic differentiation as methods for finding derivatives. An overview of specific functions whose derivatives students are expected to know is provided, setting the stage for the upcoming test.
🔧 Chain Rule and Quotient Rule Applications
The professor delves deeper into the chain rule, providing an intuitive explanation and illustrating its application with a simple example. The quotient rule is also discussed, with an alternative approach using the chain rule and power rule presented to simplify its application. The importance of recognizing when to use these rules and the ability to apply them flexibly is highlighted.
📉 Derivatives of Trigonometric Functions and Exponential Expressions
Examples of differentiating more complex functions are provided, including the secant function and natural logarithm of the secant function. The professor emphasizes the standard forms of these derivatives and their importance in mathematical communication. The natural log's historical significance in navigation and its connection to exponents is also discussed.
📌 The Chain Rule for Complex Functions
The paragraph discusses the application of the chain rule to complex functions, such as differentiating a power of a sum. The professor advises against expanding such expressions due to their complexity and instead recommends using the chain rule for a more efficient solution. The importance of recognizing efficient methods in calculus is underlined.
📝 Exam Expectations and Derivative Definitions
The lecture concludes with a discussion about exam expectations, including the ability to derive and understand the derivative of the sine function. The professor also revisits the definition of a derivative, emphasizing the importance of recognizing and applying this fundamental concept. The goal of understanding the meaning behind derivatives is reiterated.
📐 Derivatives and Tangent Lines
This section focuses on the geometric interpretation of derivatives, specifically tangent lines. The professor outlines the expectations for computing tangent lines and differentiating functions, including implicit differentiation. The concept of recognizing differentiable functions by checking for consistent left and right tangents is introduced.
📉 Derivative Graphs and Inverse Tangent Derivation
The professor demonstrates how to graph a function and its derivative, using the natural logarithm function as an example. The importance of understanding the relationship between the function and its derivative is emphasized. The lecture concludes with a brief mention of deriving the inverse tangent function, promising a more detailed explanation in a future lecture.
Mindmap
Keywords
💡Exponents
💡Limit
💡Natural Logarithm
💡Derivative
💡Chain Rule
💡Logarithmic Differentiation
💡Product Rule
💡Quotient Rule
💡Implicit Differentiation
💡Tangent Line
💡Inverse Functions
Highlights
MIT OpenCourseWare provides high-quality educational resources for free, supported by donations.
Lecture seven concludes the first unit with a focus on exponents and a review of the limit of (1 + 1/k)^k as k approaches infinity, which equals e.
The proof of the limit of a_k as k approaches infinity is clarified with a simpler symbolic representation.
The natural logarithm is shown to be the inverse of the exponential function, which is key to deriving the limit of a_k.
The concept of e is explored as both a defined base of the exponential function and a calculated limit.
The importance of reversing perspective in mathematics is emphasized, highlighting the two-way nature of equations.
The derivative of powers with real numbers is introduced, extending the formula for rational numbers to include irrational numbers.
Two methods for handling exponential problems are discussed: using base e and logarithmic differentiation.
The chain rule is applied to differentiate x^r, resulting in r * x^(r-1), regardless of whether r is rational or irrational.
Logarithmic differentiation is demonstrated as an alternative method for differentiating x^r, yielding the same result.
The natural logarithm is advocated as the natural choice for economics due to its relevance in modeling price ratios.
The significance of the natural log in simplifying expressions and its preference over other logarithmic bases is discussed.
A review of Unit One covers general and specific formulas for differentiation, including the product, quotient, and chain rules.
The importance of understanding and applying the chain rule is highlighted, with examples to illustrate its use.
The quotient rule is derived using the chain rule and product rule, offering an alternative to memorization.
Differentiation of trigonometric functions, such as secant and the natural log of secant, is demonstrated.
The process of differentiating composite functions using the chain rule is explained with examples.
The definition of a derivative is reviewed, emphasizing its role as a rate of change and its calculation from the limit of a difference quotient.
Fundamental limits and their reverse application in finding derivatives are discussed, illustrating the interplay between limits and derivatives.
Implicit differentiation is introduced as a method for finding derivatives without explicitly solving for the function.
The geometric interpretation of derivatives as tangent lines is explored, with an example demonstrating how to compute and graph them.
The process of deriving the inverse tangent function using implicit differentiation and trigonometric identities is shown.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: