Second Derivative Test to find relative maximums and minimums (extrema)

Professor Monte
3 Apr 202104:37
EducationalLearning
32 Likes 10 Comments

TLDRIn this informative video, Professor Monty explains the process of using the second derivative test to identify relative maximums of a function. The video begins with finding the critical values by setting the first derivative of the function, 3x^2 - 6x - 24, equal to zero. After solving, the critical values obtained are x = 4 and x = -2. The second derivative test involves taking the second derivative of the function, 6x - 6, and substituting the critical values into it. A positive second derivative at x = 4 indicates a concave up shape, signifying a relative minimum, while a negative second derivative at x = -2 indicates a concave down shape, indicating a relative maximum. The video concludes with the function's graph, showing a relative minimum at (4, -75) and a relative maximum at (-2, 33). The presenter encourages viewers to practice the method for better understanding.

Takeaways
  • 📚 First, find the critical values by setting the first derivative equal to zero and solving for x.
  • 🔍 Factor the first derivative, 3x^2 - 6x - 24, to find x - 4 and x + 2 as the critical points.
  • 🚫 Remember that 3 cannot equal zero, so we disregard that factor.
  • 🔑 Solve x - 4 = 0 for x = 4 and x + 2 = 0 for x = -2 to find the critical values.
  • 📈 Use the second derivative test by taking the derivative of the first derivative (6x - 6).
  • 🔁 Plug the critical values into the second derivative to determine concavity.
  • ➡️ If the second derivative is positive (concave up), it indicates a minimum at that point.
  • ⬅️ If the second derivative is negative (concave down), it indicates a maximum at that point.
  • 📌 To find the y-values of the relative extrema, substitute the critical x-values into the original function.
  • 📊 For the given function, a relative minimum is found at (4, -75) and a relative maximum at (-2, 33).
  • 📈 The graph of a cubic function will have a shape influenced by its critical points and concavity.
  • 📝 Practice is key to mastering the second derivative test and applying it to different functions.
Q & A

  • What is the first step in using the second derivative test to find relative maxima?

    -The first step is to find the critical values by taking the first derivative of the function and setting it equal to zero.

  • What is the function given in the transcript for finding critical values?

    -The function given is 3x^2 - 6x - 24.

  • How does one factor the first derivative of the function to find critical values?

    -The first derivative is factored by taking out a common factor of 3, resulting in x^2 - 2x - 8, which further factors into (x - 4)(x + 2).

  • What are the critical values found in the transcript?

    -The critical values are x = 4 and x = -2.

  • What does the second derivative test involve after finding the critical values?

    -The second derivative test involves taking the second derivative of the function and then plugging in the critical values to determine if they correspond to a relative maximum, minimum, or neither.

  • How does the sign of the second derivative indicate the concavity of the function at a critical point?

    -If the second derivative is positive, the function is concave up, indicating a relative minimum. If the second derivative is negative, the function is concave down, indicating a relative maximum.

  • What is the second derivative of the given function?

    -The second derivative is 6x - 6.

  • What is the value of the second derivative at x = 4?

    -At x = 4, the second derivative is 6(4) - 6, which equals 18.

  • What is the value of the second derivative at x = -2?

    -At x = -2, the second derivative is 6(-2) - 6, which equals -18.

  • How can you find the y-values of the relative maximum and minimum?

    -To find the y-values, plug the x-values of the relative maximum and minimum back into the original function.

  • What are the coordinates of the relative maximum and minimum found in the transcript?

    -The relative minimum is at (4, -75) and the relative maximum is at (-2, 33).

  • What is the general shape of the graph of a cubic function with a positive leading coefficient?

    -The graph of a cubic function with a positive leading coefficient will start from negative infinity, have a local maximum and minimum, and approach positive infinity as x goes to positive or negative infinity.

  • Why is the second derivative test a useful method for determining relative extrema?

    -The second derivative test is useful because it provides a way to determine the nature of a critical point without constructing a sign chart or using other more complex methods.

Outlines
00:00
📚 Introduction to the Second Derivative Test

The video begins with an introduction to the second derivative test, a method for finding relative maximums and minimums of a function. The professor, Monty, explains the process of finding critical values by taking the first derivative of the given function (3x^2 - 6x - 24), setting it to zero, and solving for x. This results in two critical values: x = 4 and x = -2.

🔍 Applying the Second Derivative Test

Monty then demonstrates how to apply the second derivative test by finding the second derivative of the function (6x - 6) and evaluating it at the critical points. For x = 4, the second derivative is positive, indicating a concave up shape and thus a relative minimum. Conversely, for x = -2, the second derivative is negative, indicating a concave down shape and a relative maximum. The significance of the sign of the second derivative is emphasized as a key determinant in identifying the nature of the critical points.

📈 Calculating Function Values at Critical Points

After identifying the nature of the critical points, Monty shows how to find the corresponding y-values by substituting the x-values back into the original function. This results in the y-values for the relative minimum at x = 4 being -75, and for the relative maximum at x = -2 being 33. These points are then clearly defined as (4, -75) for the minimum and (-2, 33) for the maximum.

📈 Visualizing the Function's Graph

Monty briefly discusses how the function's graph can be visualized, noting that the function is a cubic and thus the graph will have a specific shape. He mentions that with the relative maximum at (-2, 33) and the relative minimum at (4, -75), the overall graph can be sketched accordingly. The ease of graphing is tied to the ability to factor and find critical values.

📝 Conclusion and Engagement

The video concludes with Monty summarizing the second derivative test and encouraging practice to master the technique. He also invites viewers to subscribe to his channel and like the video if they found it helpful, promising more informative content in future videos.

Mindmap
Keywords
💡Second Derivative Test
The Second Derivative Test is a mathematical method used to determine the nature of critical points of a function. In the video, it is used to find relative maximums and minimums. The test involves taking the second derivative of a function and evaluating it at critical points. If the second derivative at a point is positive, the function is concave up at that point, indicating a relative minimum. Conversely, if the second derivative is negative, the function is concave down, indicating a relative maximum. For example, the video demonstrates this by taking the second derivative of the function 3x^2 - 6x - 24 and evaluating it at the critical points x = 4 and x = -2.
💡Critical Values
Critical values are points on the x-axis where the derivative of a function is either zero or undefined. They are critical in calculus for identifying potential local maxima, minima, or points of inflection. In the context of the video, the critical values are found by setting the first derivative of the function equal to zero and solving for x, which yields x = 4 and x = -2. These points are then used to apply the second derivative test.
💡First Derivative
The first derivative of a function measures the rate at which the function is changing at any given point. It is a fundamental concept in calculus and is used to find critical points and analyze the behavior of a function. In the video, the first derivative of the function 3x^2 - 6x - 24 is calculated as 6x - 6, which is then used to find critical values by setting it equal to zero.
💡Concave Up
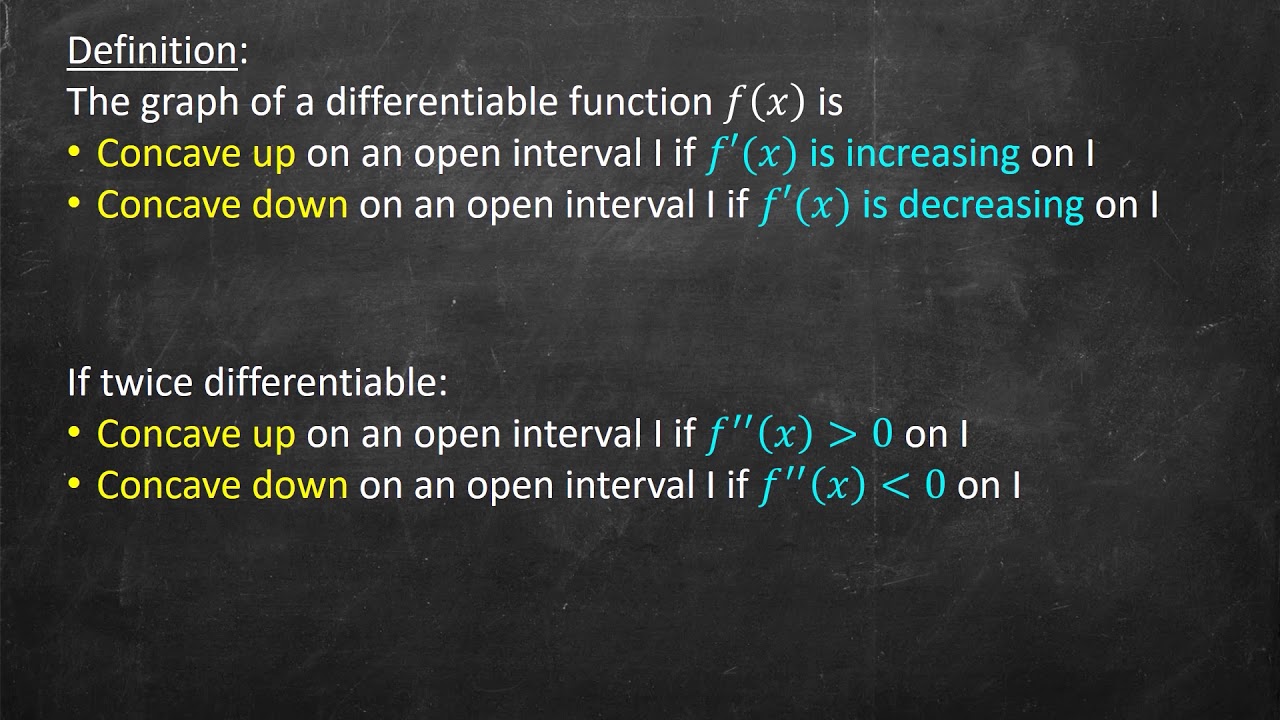
A function is said to be concave up on an interval if the graph of the function lies above its tangent at every point in that interval. In the video, when the second derivative of the function is positive, it indicates that the function is concave up. This is significant because a positive second derivative at a critical point suggests that the function has a local minimum at that point. For instance, f''(4) = 18, which is positive, indicating a local minimum at x = 4.
💡Concave Down
Concave down is the opposite of concave up. A function is concave down on an interval if the graph of the function lies below its tangent at every point in that interval. If the second derivative of a function is negative at a critical point, it indicates that the function is concave down at that point, suggesting a local maximum. In the video, f''(-2) = -18, which is negative, indicating a local maximum at x = -2.
💡Relative Maximum
A relative maximum is a point on the graph of a function where the function's value is higher than the values of the function at neighboring points. It is a local property, not requiring the value to be the highest overall. In the video, the relative maximum is found at x = -2, where the second derivative test indicates the function is concave down, and the function's value is 33.
💡Relative Minimum
A relative minimum is similar to a relative maximum but indicates a point where the function's value is lower than the values at neighboring points. It is a local property as well. In the video, the relative minimum is located at x = 4, where the second derivative test shows the function is concave up, and the function's value is -75.
💡Factoring
Factoring is a mathematical method of breaking down a polynomial into its constituent factors. It is a common technique used to find the roots of a polynomial. In the video, the first derivative of the function is factored to find its critical points. The factoring of the first derivative 3x^2 - 6x - 24 leads to (x - 4)(x + 2) = 0, which gives the critical values x = 4 and x = -2.
💡Graph of a Function
The graph of a function is a visual representation of the relationship between the input (x-axis) and the output (y-axis) of the function. It is a useful tool for understanding the behavior of the function, including its maxima and minima. In the video, the graph of the cubic function is described qualitatively, with the relative maximum at (-2, 33) and the relative minimum at (4, -75).
💡Cubic Function
A cubic function is a polynomial function of degree three. The general form of a cubic function is f(x) = ax^3 + bx^2 + cx + d, where a, b, c, and d are constants and a ≠ 0. In the video, the function 3x^2 - 6x - 24 is a quadratic function, not cubic, but the term is used to describe the general shape of the graph, which would be similar to a cubic's if it had a degree of three.
💡Derivative
The derivative of a function is a measure of the rate at which the function's output (y) changes with respect to its input (x). It is a fundamental concept in calculus and has many applications in mathematics and physics. In the video, both the first and second derivatives of the function are calculated to find critical points and determine the concavity of the function, which helps in identifying relative maxima and minima.
Highlights

The second derivative test is used to find relative maximums of a function.

First, find the critical values by taking the first derivative and setting it equal to zero.

For the given function 3x^2 - 6x - 24, the critical values are x = -2 and x = 4.

Next, take the second derivative of the function, which is 6x - 6.

Plug in the critical values into the second derivative to determine concavity.

If the second derivative is positive, the graph is concave up and it's a minimum.

If the second derivative is negative, the graph is concave down and it's a maximum.

For the given function, f''(4) = 18 (positive) so there's a minimum at x = 4.

For the given function, f''(-2) = -18 (negative) so there's a maximum at x = -2.

To find the y-values of the relative max/min, plug the x values back into the original function.

The relative minimum is at (x,y) = (4, -75).

The relative maximum is at (x,y) = (-2, 33).

The graph of the function is a cubic with a relative maximum at (-2, 33) and minimum at (4, -75).

The second derivative test is easier to apply when the function is easier to factor and find critical values.

The second derivative test does not require a sign chart or other complicated steps.

The second derivative test provides a straightforward way to find relative extrema of a function.

Practice applying the second derivative test to different functions to gain proficiency.

The video provides a clear, step-by-step explanation of the second derivative test.

Transcripts

Browse More Related Video

Maxima , minima and point of inflection

Fiding Relative Max, Min and Inflection Point with Derivatives F4

Concavity and the 2nd Derivative Test

Finding Concavity and Inflection Points

First Derivative Test to find increasing/decreasing and relative max/min

Calculus I: Finding Intervals of Concavity and Inflection point

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Derivative TestCalculusCritical ValuesRelative MaximaRelative MinimaCubic FunctionGraph AnalysisMath EducationMonty's TutorialEducational ContentMath Techniques