Lec 6 | MIT 18.01 Single Variable Calculus, Fall 2007
TLDRThis MIT OpenCourseWare lecture introduces exponentials and logarithms, fundamental functions essential for calculus. The professor explains the properties of exponential functions, including the base and exponent rules, and their graphical representation. The focus then shifts to calculating derivatives, particularly the derivative of a^x, which leads to the introduction of the natural logarithm and its properties. The lecture explores methods for differentiating exponential functions, including converting to base e and logarithmic differentiation, and concludes with a practical example of calculating the limit of (1 + 1/n)^n, revealing it to be e, a fundamental mathematical constant.
Takeaways
- 📚 The lecture introduces exponentials and logarithms as fundamental functions connected to calculus, emphasizing their importance alongside trigonometric functions.
- 🔑 The base 'a' of an exponential function is a positive number, with defined properties such as a^0 = 1 and a^(x_1 + x_2) = a^(x_1) * a^(x_2).
- 📈 Exponential functions are defined for all x by continuity, and their graphs, like y = 2^x, show a rapid increase with the most important point being (0,1).
- 🧭 The derivative of an exponential function a^x is approached by using the limit definition and properties of exponents, leading to a^x times a mystery number M(a).
- 🌟 The number 'e' is defined as the unique base for which the mystery number M(e) equals 1, simplifying the derivative of e^x to e^x itself.
- 🔍 The natural logarithm ln(x) is introduced as the inverse function of e^x, with properties derived from those of exponents, such as ln(x_1 * x_2) = ln(x_1) + ln(x_2).
- 📉 The graph of ln(x) is only defined for x > 0, with a slope of 1 at the point (1,0), contrasting with the always positive nature of e^x.
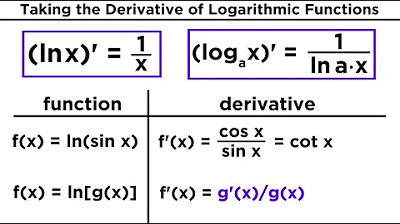
- 🔧 The derivative of ln(x) is computed using implicit differentiation, resulting in the formula 1/x, highlighting the inverse relationship with e^x.
- ✅ Two methods for differentiating exponential functions are presented: converting to base e and using the natural logarithm, both yielding the formula (ln a) a^x for the derivative of a^x.
- 🔄 Logarithmic differentiation is introduced as a technique for differentiating functions with moving exponents or bases, simplifying the process by focusing on the logarithm of the function.
- 🎯 The limit as n approaches infinity of (1 + 1/n)^n is evaluated using logarithms, revealing it to be equal to e, providing a numerical method to approximate the base of natural logarithms.
Q & A
What is the main topic of lecture six?
-The main topic of lecture six is exponentials and logarithms, and their connection with calculus.
What is the significance of the number 'a' in the context of the lecture?
-In the context of the lecture, 'a' is a positive number usually referred to as the base of an exponential function.
What are the initial properties of an exponential function mentioned in the lecture?
-The initial properties mentioned are that a to the power of 0 is always 1, and a^1 is a.
What is the general rule for exponents that the professor introduces?
-The general rule introduced for exponents is that a^(x_1 + x_2) is equal to a^(x_1) times a^(x_2).
How is the value of a^x defined for all x?
-The value of a^x is defined for all x by using the concept of taking the nth root of a^m, where m and n are integers, and filling in the values by continuity.
What is the graphical representation of the exponential function y = 2^x?
-The graphical representation of y = 2^x starts at the point (0,1), goes through the point (-1, 1/2), and increases rapidly, going off the board at x=2.
What is the definition of the derivative of an exponential function a^x?
-The derivative of an exponential function a^x is defined as the limit as delta x goes to 0 of (a^(x + delta x) - a^x) / delta x.
What is the significance of the number e in the context of derivatives?
-The number e is defined as the unique number such that the limit as delta x goes to 0 of (e^(delta x) - 1) / delta x equals 1, making the derivative of e^x equal to e^x itself.
What is the natural logarithm denoted as and what is its relationship to the exponential function?
-The natural logarithm is denoted as ln(x), and it is the inverse function of e^x, meaning that the log of y is equal to x when y = e^x.
How can you differentiate a^x using base e?
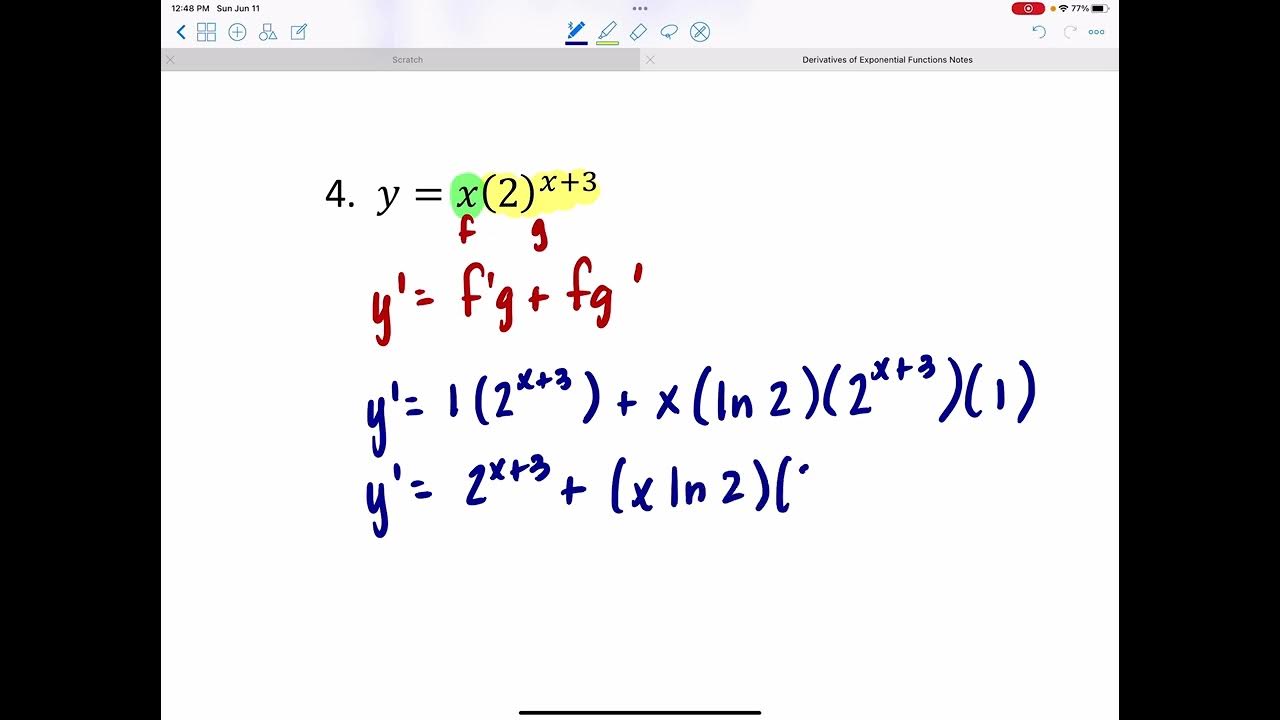
-You can differentiate a^x using base e by writing a^x as e^(x ln a) and then differentiating using the chain rule, resulting in ln a times e^(x ln a), which simplifies to (ln a) a^x.
What is the logarithmic differentiation method and when is it useful?
-Logarithmic differentiation is a method used when differentiating a function is difficult, but differentiating its logarithm is easier. It involves taking the logarithm of both sides of an equation, differentiating, and then using the relationship (ln u)' = u' / u to find the derivative of the original function.
Can you provide an example of using logarithmic differentiation to differentiate a function with a moving exponent and base?
-An example of using logarithmic differentiation for a function with a moving exponent and base is differentiating x^x. By taking the logarithm of both sides and differentiating, you can find that d/dx x^x = x^x (1 + ln x).
What is the significance of the limit as n goes to infinity of (1 + 1/n)^n and how is it related to the number e?
-The limit as n goes to infinity of (1 + 1/n)^n is equal to e, which provides a numerical way to approximate the number e. This limit demonstrates the relationship between exponential growth and the number e, and it is a fundamental concept in calculus.
Outlines
📚 Introduction to Exponentials and Logarithms
The video script begins with an introduction to the concepts of exponentials and logarithms, which are essential for understanding calculus. The professor emphasizes the importance of these functions, comparing them to trigonometric functions. The base 'a' of an exponential function is introduced, along with its properties, such as any positive number raised to the power of 0 equals 1. The script explains the rule for multiplying exponential functions and how to understand the range of values for a^x, including irrational exponents. The professor also illustrates the exponential function graphically, using y = 2^x as an example, and discusses the rapid growth of exponential functions.
🧐 Derivative of Exponential Functions
The script then delves into the calculus aspect of exponential functions, specifically focusing on finding their derivatives. The derivative is defined using the limit process, and the professor simplifies the expression for the derivative of a^x by factoring out a^x from the limit. A conceptual shift is introduced where 'delta x' becomes the variable while 'x' is held constant. This leads to the identification of a mystery number M(a), which is defined as the limit of (a^delta x - 1) / delta x as delta x approaches 0. The professor discusses the geometric interpretation of M(a) and its significance in determining the slope of the exponential function at x = 0.
🔍 The Mystery of Base 'e'
The professor introduces a new concept, the base 'e', defined as the unique number for which the mystery number M(e) equals 1. This leads to the fundamental derivative formula d/dx of e^x being simply e^x. The script explores the implications of this definition, showing how knowing the slope of the graph of e^x at x = 0 allows us to determine the slope at any point. The professor also discusses the process of stretching the function f(x) = 2^x by a factor k, resulting in a new base b = 2^k, and how this relates to finding the base 'e'.
📉 Natural Logarithm and Its Properties
The script introduces the natural logarithm, denoted as ln(x), and its properties derived from the properties of exponential functions. The professor explains the logarithm of a product rule, the logarithm of 1 being 0, and the logarithm of e being 1. These properties are illustrated graphically, showing the inverse relationship between the exponential function e^x and the natural logarithm ln(x). The graph of ln(x) is only defined for positive x, reflecting that e^x is always positive, and the slope of the tangent line to ln(x) at x = 1 is 1.
📝 Derivative of Logarithm Functions
The script explains how to find the derivative of a logarithm function using implicit differentiation. The process involves rewriting the logarithmic equation as an exponential equation, e^(ln x) = x, and then differentiating both sides with respect to x. The chain rule is applied, leading to the conclusion that the derivative of ln x with respect to x is 1/x. This is a key formula for understanding the rate of change of logarithmic functions.
🔢 Differentiating Exponential Functions Using Base e
The script presents a method for differentiating any exponential function a^x by converting it to base e. This involves expressing a^x as e^(x ln a) and then differentiating using the chain rule. The result is that the derivative of a^x is (ln a) a^x. The professor emphasizes the importance of understanding constants in the chain rule and how they affect the rate of change. This method simplifies the process of differentiating exponential functions.
🔄 Logarithmic Differentiation
The script introduces logarithmic differentiation, a technique useful when differentiating functions with moving exponents or bases is complex. The method involves taking the logarithm of the function, differentiating the resulting expression, and then using the chain rule to find the derivative of the original function. The professor illustrates this with the function u = x^x, showing how to apply logarithmic differentiation to find its derivative.
🎯 Evaluating the Limit of a Moving Exponent
The script discusses an example that goes beyond basic calculus, using the tools of logarithmic differentiation to evaluate the limit as n approaches infinity of (1 + 1/n)^n. The professor takes the natural logarithm of the expression, rewrites it in a form that fits the pattern for calculating the derivative of the log function, and shows that the limit of the logarithm is 1. This implies that the original limit is e, providing a numerical way to approximate the base 'e'.
🔚 Closing the Loop on Understanding e
The final paragraph wraps up the discussion by highlighting the significance of the limit evaluation in understanding the base 'e'. The professor points out that this provides a practical numerical method to approximate 'e', such as using (1 + 1/100)^100. This closes the loop on the coherent family of functions discussed in the script, which are well-defined and have practical methods for calculation.
Mindmap
Keywords
💡Exponentials
💡Logarithms
💡Derivative
💡Base 'e'
💡Limit
💡Chain Rule
💡Natural Logarithm
💡Logarithmic Differentiation
💡Product Rule
💡Differentiation Formulas
Highlights
Introduction to exponentials and logarithms as fundamental functions connected with calculus.
Definition of the base 'a' in exponential functions and its properties.
Explanation of the basic rule of exponents and the composition of exponential functions.
Understanding the values of a^x for rational and irrational exponents.
The process of 'filling in' the values of a^x using continuity.
Graphical representation of the exponential function y = 2^x.
The goal of applying calculus to find the derivative of exponential functions.
Derivation of the derivative of a^x using the limit definition and properties of exponents.
Introduction of the mystery number M(a) representing the slope of the exponential function at x = 0.
Geometric interpretation of M(a) and its significance in differentiating a^x.
The unique number e defined such that M(e) = 1, simplifying the derivative of e^x to e^x.
Existence proof of the number e through stretching functions and slopes.
Introduction of the natural logarithm ln(x) and its properties.
Derivation of the derivative of ln(x) using implicit differentiation.
Two methods for differentiating exponential functions: converting to base e and logarithmic differentiation.
Illustration of logarithmic differentiation on functions with moving exponents and bases.
Evaluation of the limit (1 + 1/n)^n as n approaches infinity using logarithms, concluding it equals e.
Practical numerical approximation method for calculating the number e.
Completion of the loop in understanding the coherent family of exponential and logarithmic functions with practical calculation methods.
Transcripts
Browse More Related Video

Math1325 Lecture 11 1 Derivatives of Logarithm

2.3 - Derivatives of Logarithmic Functions
Lec 7: Exam 1 review | MIT 18.01 Single Variable Calculus, Fall 2007
Derivatives of Exponential Functions

Calculus 2 Lecture 6.3: Derivatives and Integrals of Exponential Functions
Derivatives of Logarithmic and Exponential Functions
5.0 / 5 (0 votes)
Thanks for rating: