Math 11 - Sections 6.1- 6.2

Professor Monte

5 Apr 202076:02

EducationalLearning

32 Likes 10 Comments

TLDRThe video script is a comprehensive lecture on multivariate calculus, focusing on functions of several variables and their derivatives. It begins by contrasting single-variable functions, such as y = x^2 - 9x + 2, with multivariate functions, which depend on more than one independent variable, exemplified by a student's test score being influenced by homework completion and study hours. The lecture introduces notation for functions of multiple variables, such as f(x, y), and discusses evaluating these functions at given points, as demonstrated with the function f(x, y) = y^2 + 2xy. The crux of the script delves into partial derivatives, illustrating how to treat one variable as constant while differentiating with respect to another. This concept is applied to various functions, including those with multiple variables and constants. The lecture also covers second-order partial derivatives and their implications, emphasizing the need for consistency in results when deriving in different orders. Practical applications, such as the Cobb-Douglas production function in economics, highlight the significance of marginal productivities in decision-making. The script concludes with an interactive approach, encouraging students to solve problems and verify their understanding through practice.

Takeaways

📚 The lecture covers two sections: 6.1 on functions of several variables, and 6.2 on derivatives of such functions, with a focus on partial derivatives.
🔢 Functions can depend on more than one variable, which is a shift from the single-variable functions previously discussed.
📈 The concept of treating one variable as constant while differentiating with respect to another is a key idea in understanding partial derivatives.
🎓 Examples are provided to illustrate how to calculate function values at given points and how to work with different notations such as Newton's and Leibniz's notation.
🤔 The importance of understanding that constant multiples do not go to zero when taking derivatives is emphasized, which can be a common source of confusion.
📊 The application of partial derivatives is demonstrated through the Cobb-Douglas production function model, which is used to determine marginal productivities in economics.
🧮 Calculating second-order partial derivatives is shown to be a straightforward extension of first-order partial derivatives, with the added complexity of multiple differentiation steps.
🤓 The use of parentheses and the order of operations in calculations is highlighted as crucial for accuracy, especially when dealing with powers and division.
📉 The economic application of the Cobb-Douglas model helps in decision-making regarding the allocation of resources between labor and capital for production.
💡 The marginal productivity of labor and capital is calculated to determine the additional units produced by adding one more unit of either labor or capital.
📚 Students are encouraged to practice problems from both Chapter 3 and Chapter 6, as both will be covered in the upcoming exam.

Q & A

What are the two sections being discussed in the transcript?
-The two sections being discussed are 6.1 and 6.2, which cover functions of several variables and derivatives of functions of several variables, respectively.
What is the difference between a function of one variable and a function of several variables?
-A function of one variable, such as y = x^2 - 9x + 2, has a single independent variable (x), whereas a function of several variables depends on more than one independent variable, which can affect the outcome of the function.
How is the notation for functions of several variables typically written?
-Functions of several variables can be written using Newton's notation as f(x, y) or in Leibnitz notation as z = 3x - 9y^2, where x and y are independent variables and z is the dependent variable.
What is a partial derivative?
-A partial derivative is a derivative that takes the rate of change of one variable at a time, treating the other variables as constants.
How does the process of taking partial derivatives relate to the concept of slopes in a three-dimensional space?
-Taking partial derivatives is akin to finding the slope of a function at a particular point in a three-dimensional space. It shows how the function changes with respect to one variable while holding the other variables constant.
What is the Cobb-Douglas model mentioned in the transcript?
-The Cobb-Douglas model is a production function used in economics that represents the relationship between the quantity of output produced and the quantities of various inputs used. It is given by the formula P = A * X^(α) * Y^(β), where P is the production, X and Y are inputs, and α and β are constants that sum up to 1.
What are marginal productivities in the context of the Cobb-Douglas model?
-Marginal productivities in the context of the Cobb-Douglas model refer to the partial derivatives of the production function with respect to the inputs (labor and capital). They indicate the additional output that can be produced by adding one more unit of an input while holding the other input constant.
How does the transcript demonstrate the calculation of the marginal productivity of labor and capital?
-The transcript demonstrates the calculation by evaluating the partial derivatives of the Cobb-Douglas production function at specific levels of labor (X) and capital (Y). It shows that by adding one unit of labor, 7,680 more units are produced, while adding one unit of capital results in 360 more units.
What is the significance of finding the second-order partial derivatives in the transcript?
-Second-order partial derivatives provide information about the curvature of the function. In the context of the Cobb-Douglas model, they can be used to analyze the elasticity of the production function with respect to changes in inputs.
How does the transcript address the potential confusion between constant multiples and variables when taking derivatives?
-The transcript clarifies that constant multiples do not go to zero when taking derivatives if they are part of a product with a variable. It emphasizes the importance of understanding whether a term is a constant or a variable when applying the rules of differentiation.
What is the recommendation for students when practicing taking partial derivatives?
-The recommendation is to practice by pausing the video, working through the problem, and then resuming the video to compare answers. This helps in understanding the process and identifying any mistakes made during the calculation.

Outlines

00:00

📚 Introduction to Functions of Several Variables

The video begins with an introduction to functions involving multiple variables, contrasting them with single-variable functions. It explains that functions like Y = X^2 - 9X + 2 have one independent variable, X, whereas functions in several variables, such as F(X, Y), depend on more than one variable, like the number of homework problems done and hours studied affecting a student's test score. The notation for such functions is also discussed, including Newton's and Leibniz's notations, with examples provided to illustrate their use.

05:05

🔍 Evaluating Function Values at Specific Points

The script continues with an example of evaluating function values at specific points, such as F(-2, 0) and F(3, 2), using the given function F(X, Y) = Y^2 + 2XY + X^3. The process involves substituting the given X and Y values into the function and performing the necessary calculations, resulting in the function's value at those points, which are then interpreted in a three-dimensional context.

10:08

📈 Application: Calculating Stock Yield

An application of functions of several variables is demonstrated through the example of calculating the yield of a stock, which is a function of both the dividend and the stock's price. The formula for yield is given as Y = D/P, where D is the dividend and P is the price. Using historical data for the Texas Instrument stock price and dividend, the script shows how to calculate the yield on April 1st, 2014.

15:09

🧮 Understanding Partial Derivatives

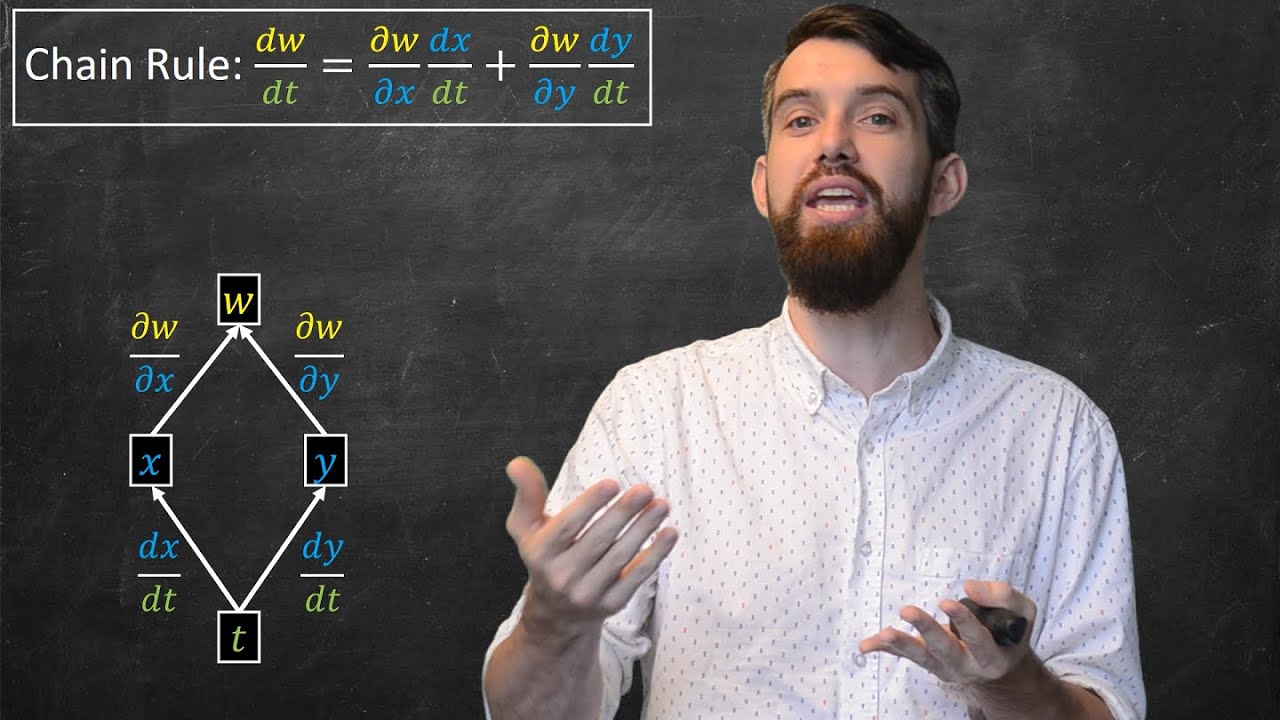
The concept of partial derivatives is introduced, emphasizing that they involve differentiating with respect to one variable while keeping the other variables constant. The script explains the notation and calculation process for partial derivatives, using a simple function f(X, Y) = 3X + 5Y to illustrate. It also discusses the importance of treating the other variables as constants when taking partial derivatives.

20:09

📈 Calculating Partial Derivatives and Their Values at Points

The script delves into calculating partial derivatives of more complex functions, such as Z = 2X^3 + 3XY - X, and evaluating these derivatives at specific points, like the partial of Z with respect to X at (-2/3, -3) and the partial of Z with respect to Y at (0, -5). The process involves applying the rules of differentiation to each term of the function, considering the other variable as constant.

25:16

🧐 Challenging Partial Derivatives with Logarithms and Exponents

The video tackles more complex scenarios involving partial derivatives, including functions with logarithms and exponents such as f(X, Y) = Y * ln(X) + 2Y. The script outlines the steps for finding partial derivatives with respect to both X and Y, emphasizing the use of the chain rule and product rule where applicable. It also encourages students to practice and review previous problems to solidify their understanding.

30:17

🔢 Second-Order Partial Derivatives and Their Applications

The script introduces second-order partial derivatives, which are the partial derivatives of first-order partial derivatives. It explains the four different types of second-order partial derivatives and emphasizes that the order of differentiation does not matter for certain pairs, resulting in equal values. Examples are provided to illustrate the calculation of these derivatives, and the script advises students to verify their work by comparing the results of different orders of differentiation.

35:20

🏭 Applying Partial Derivatives to the Cobb-Douglas Production Function

An application of second-order partial derivatives is shown using the Cobb-Douglas production function, which is used to determine the marginal productivities of labor and capital. The script calculates the production units based on given labor and capital units and then finds the partial derivatives with respect to both labor (X) and capital (Y). These derivatives are evaluated at specific points to determine the additional units produced by adding one unit of labor or capital, providing insight into the most efficient way to increase production.

40:20

📝 Wrapping Up the Lesson

The video concludes with a reminder for students to practice the material, especially the calculations of partial derivatives, as it will be part of the upcoming test. The instructor encourages students to complete their homework and to ask any questions they may have during the live session. The emphasis is on understanding the process of taking derivatives in functions of several variables and applying them to real-world scenarios.

Mindmap

Keywords

💡Function of Several Variables

A function of several variables is a mathematical concept where the output is determined by more than one input variable. In the video, this concept is central as it discusses how to handle functions that depend on multiple factors, such as the performance of a student on a test, which could depend on the number of homework problems done and hours studied.

💡Partial Derivative

A partial derivative is a derivative taken with respect to one variable of a function of several variables, while keeping the other variables constant. It's used to measure how a multi-variable function changes with respect to one variable. In the video, partial derivatives are extensively discussed, with examples such as finding the rate of change of a function with respect to x while treating y as a constant.

💡Newton's Notation

Newton's notation is a way of representing functions of several variables, often written as f(x, y) to denote a function of x and y. The video script mentions Newton's notation as a preferred method for the lecturer when dealing with functions of multiple variables and their derivatives.

💡Leibniz Notation

Leibniz notation is an alternative way of representing derivatives, often using the delta symbol (∆) to denote a small change, as in ∆Z/∆X for the partial derivative of Z with respect to X. The video contrasts Newton's notation with Leibniz notation, particularly when explaining how to write out partial derivatives.

💡Cobb-Douglas Model

The Cobb-Douglas model is a production function used in economics that relates the quantity of output to the quantities of inputs (like labor and capital). It is represented in the video by the formula P = 2400 * X^(2/5) * Y^(3/5), where P is the production, X is labor, and Y is capital. The model is used to calculate marginal productivities, which are essential for determining the efficiency of adding more labor or capital.

💡Marginal Productivity

Marginal productivity is the additional output that is produced by using one more unit of a variable input, while other inputs are held constant. In the video, the concept is used to evaluate how many more units of production are created when one more unit of labor or capital is added, which is crucial for economic decision-making.

💡Second-Order Partial Derivatives

Second-order partial derivatives are derivatives taken twice, either with respect to the same variable or with respect to two different variables. They provide information about the curvature of the function and are used in the video to further analyze the behavior of functions of several variables.

💡Constant Multiple Rule

The constant multiple rule states that the derivative of a constant times a function is the constant times the derivative of the function. This rule is applied in the video when taking partial derivatives, as constants are factored out and not reduced to zero.

💡Chain Rule

The chain rule is a fundamental rule in calculus used to compute the derivative of a composite function. In the context of the video, the chain rule is used when taking derivatives of functions that are composed of other functions, such as when differentiating (2x + y - 5)^2 with respect to x or y.

💡Product Rule

The product rule is a formula used to find the derivative of a product of two functions. In the video, the product rule is mentioned in the context of taking partial derivatives with respect to one variable when the function includes a product of that variable with another term.

💡Independent and Dependent Variables

In the context of functions, independent variables are the inputs that can be freely changed, while dependent variables are the outputs that result from the independent variables. In the video, the distinction between independent and dependent variables is essential when taking partial derivatives, as it determines which variables are treated as constants.

Highlights

Introduction to functions of several variables, emphasizing their dependence on more than one independent variable.

Explanation of how the performance on a test can be a function of multiple factors, such as homework completion and study hours.

Discussion on the stock market as an example of a function influenced by various variables like consumer sentiment and interest rates.

Illustration of different notations for functions of several variables, such as f(X, Y) and Z = 3X - 9Y^2.

Calculation of function values for given variable values, demonstrated through examples from a textbook.

Introduction to partial derivatives, highlighting their role in determining the rate of change of a function with respect to one variable while holding others constant.

Use of Newton's notation and Leibnitz notation in expressing derivatives and partial derivatives.

Application of partial derivatives in economics, specifically in the Cobb-Douglas production function model.

Calculation of marginal productivities to determine the additional output from one more unit of labor or capital.

Practical application of the Cobb-Douglas model to decide between hiring more labor or investing in more capital based on cost-effectiveness.

Emphasis on the importance of treating non-variable terms as constants when taking partial derivatives.

Demonstration of how to calculate second-order partial derivatives and the significance of their order.

Explanation of the product rule and chain rule in the context of taking partial derivatives.

Use of t-charts for visualizing the impact of changing one variable on the function while keeping the other constant.

Guidance on practicing problems by pausing the video and working through them independently to check understanding.

Clarification on the concept of slopes in the context of three-dimensional space and how partial derivatives relate to them.

Advice on checking one's work by comparing the results of different orders of partial derivatives to ensure accuracy.

Transcripts

Browse More Related Video

Partial derivatives - How to solve?

What Partial Derivatives Are (Hands-on Introduction) — Topic 67 of Machine Learning Foundations

Functions of Several Variables!

The Multi-Variable Chain Rule: Derivatives of Compositions

Partial Derivatives!

Managerial Economics 1.3: Partial Derivatives

Related Tags

Multivariate Calculus Partial Derivatives Functions Economics Finance Cobb-Douglas Model Derivative Calculations Stock Market Education Mathematical Analysis Productivity