Math 11 - Section 2.1

Professor Monte

2 Jun 202064:42

EducationalLearning

32 Likes 10 Comments

TLDRThe video script is an educational transcript that delves into the concepts of exponential and logarithmic functions, with a focus on the natural base e, commonly known as Euler's number. The speaker provides a comprehensive review suitable for those with a background in college or intermediate algebra. The lesson covers the definition of exponential functions, where the variable is in the exponent, contrasting it with power functions. It continues with an exploration of the base e, its mathematical significance, and its approximation. The script includes practical applications, such as compound interest calculations, to illustrate the use of exponential functions in real-world scenarios. Logarithms are introduced as the inverse of exponential functions, with an emphasis on their role in solving exponential equations. The properties of logarithms are reviewed, and the speaker demonstrates how to convert between logarithmic and exponential forms. The transcript concludes with examples of solving logarithmic equations and the application of these mathematical concepts to problems involving continuous growth, such as predicting the spending on organic food based on historical data. The summary is rich with mathematical insights and practical applications, aiming to solidify the viewer's understanding of these fundamental concepts.

Takeaways

📚 The session is a review of algebra concepts, primarily focusing on exponential and logarithmic functions, which are crucial for further studies in calculus.
📈 An exponential function is defined by having the variable in the exponent, such as y = 2^x, and these functions have a characteristic graph often described as a 'hockey stick' or 'banana' shape.
🔢 Euler's constant (e) is introduced as a base for natural logarithms, approximately equal to 2.71828, and is distinct from other irrational numbers like pi.
🛒 The concept of compound interest is explored using exponential functions, demonstrating how the amount in an account grows over time with repeated application of interest.
🧮 The use of a calculator for evaluating exponential functions, such as e^x, is demonstrated, highlighting the utility of the e button and the importance of understanding the display and entry methods.
📊 The domain of a logarithmic function is discussed, emphasizing that the input (x) must be positive since you cannot take the logarithm of a negative number.
✅ The process of solving exponential equations using logarithms is reviewed, including the use of properties of logarithms to simplify and solve for the variable.
💰 An application of logarithmic functions to real-world scenarios, such as pricing models for consumer goods, is presented to illustrate the practical use of these mathematical concepts.
📉 The behavior of logarithmic graphs is described, noting that they have a vertical asymptote and increase slowly, which is the inverse of the exponential function's behavior.
🔧 The formula for continuous compounding is applied to estimate future spending on organic food and beverages, showcasing how mathematical models can predict real-world trends.
⏱ The concept of doubling time in continuous growth is introduced, providing a formula to calculate the time it takes for an investment or value to double at a given growth rate.

Q & A

What is the primary focus of the chapter 2 review?
-The primary focus of the chapter 2 review is on exponential and logarithmic functions, particularly those of the natural base e, and their applications in various mathematical contexts.
How does the variable in an exponential function differ from that in a power function?
-In an exponential function, the variable is in the exponent, whereas in a power function, the exponent is a constant, not a variable.
What is Euler's constant (e) approximately equal to?
-Euler's constant (e) is approximately equal to 2.71828, often rounded to 2.7 for simplicity in calculations.
What is the general shape of the graph for exponential functions?
-The general shape of the graph for exponential functions is often described as a 'hockey stick' or 'banana' shape, where the function never goes below the x-axis and increases without bound.
How is compound interest calculated when compounded continuously?
-When compound interest is compounded continuously, the formula becomes A = P * e^(rt), where A is the amount in the account after t years, P is the principal amount, r is the annual interest rate (as a decimal), and t is the time in years.
What is the domain of the logarithmic function y = ln(5x - 2)?
-The domain of the logarithmic function y = ln(5x - 2) is x > 2/5, as the argument of the logarithm (5x - 2) must be greater than zero.
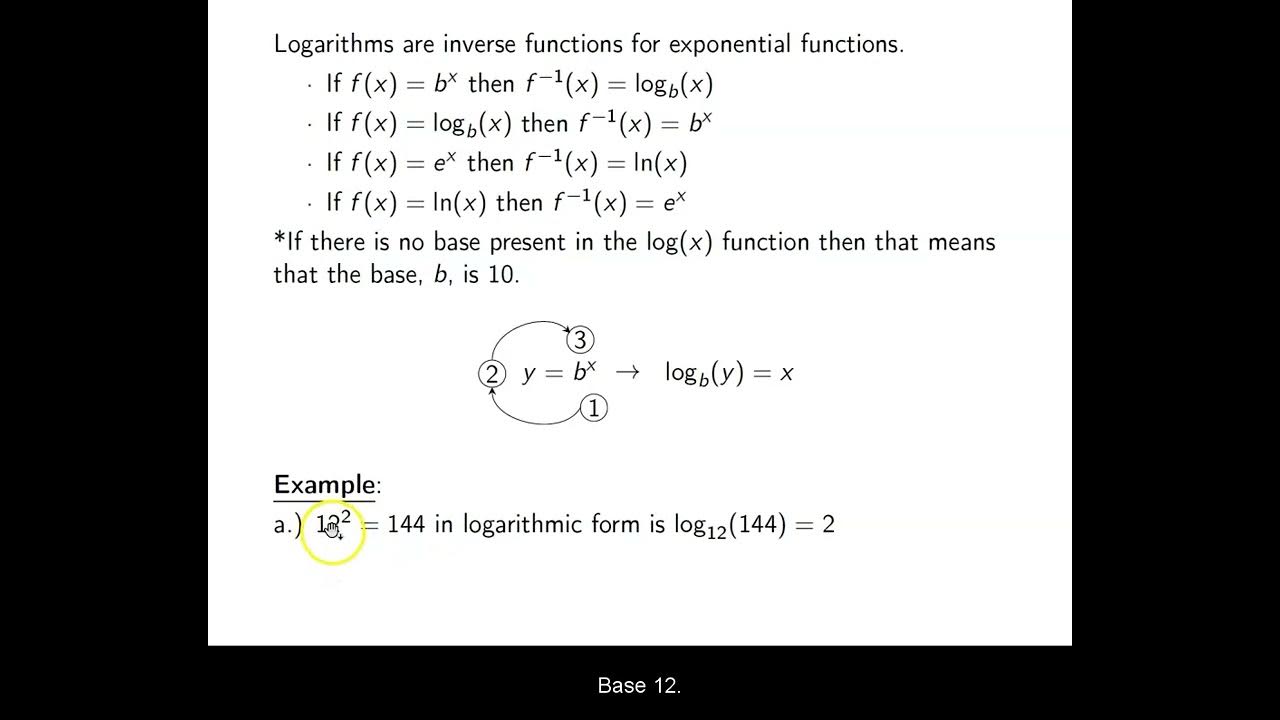
How can you convert a logarithmic equation into an exponential equation?
-You can convert a logarithmic equation, such as log base b of x = y, into an exponential equation by recognizing that it is asking 'to what power must b be raised to get x?', which is equivalent to b^y = x.
What is the formula for calculating the doubling time of an investment?
-The formula for calculating the doubling time of an investment is T = ln(2) / K, where T is the doubling time and K is the continuous growth rate.
What is the significance of the natural base e in mathematics?
-The natural base e, approximately equal to 2.71828, is significant in mathematics because it is the base for which the derivative of the exponential function e^x is itself. It frequently appears in mathematical analysis, particularly in calculus, and in many natural phenomena.
How does the compound interest formula change when interest is compounded more frequently?
-When interest is compounded more frequently, the formula A = P(1 + r/n)^(nt) still applies, but n, the number of times per year interest is compounded, increases. For example, if interest is compounded daily, n would be 365.
What is the relationship between the base of an exponential function and the shape of its graph?
-The base of an exponential function affects the 'stretch' or 'compression' of the graph. A base greater than 1 will stretch the graph vertically, making the function grow more rapidly as x increases. Conversely, a base between 0 and 1 will compress the graph, causing the function to grow more slowly.

Outlines

00:00

📚 Introduction to Exponential Functions

The video begins with an introduction to a new chapter focusing on algebraic review, specifically exponential and logarithmic functions. The presenter defines an exponential function as one where the variable is in the exponent, contrasting it with a power function where the exponent is a constant. The base 'e' is introduced as Euler's constant, an irrational number approximately equal to 2.71, which often appears in nature and mathematical functions. The video also covers the graphing of exponential functions, noting their upward trend without ever dipping below the x-axis.

05:04

🔢 Understanding Euler's Constant and Exponential Function Values

The speaker delves into Euler's constant 'e', explaining its significance and providing an approximation of 2.71. The video demonstrates how to calculate values of exponential functions using base 'e' and a graphing calculator. By plugging in various values for X, the presenter illustrates the resulting Y values and how they relate to the shape of the exponential function graph, which is similar to the graph of y = 2^X.

10:05

📈 Exponential Functions and Compound Interest

The application of exponential functions to compound interest is explored. The presenter explains the formula for compound interest, which involves the principal amount, interest rate, number of compounding periods, and time in years. An example calculation is shown, starting with a principal of $4,000 at a 6% interest rate compounded yearly for 10 years, and then compounded daily and continuously for comparison, highlighting the increasing growth of the investment over time.

15:07

🧮 Logarithms and Solving Exponential Equations

The video transitions into logarithms, explaining them as a way to rewrite exponential equations. Logarithms are presented as the inverse operation to exponentiation, useful for solving exponential equations. The natural logarithm, denoted as 'Ln', is introduced as the logarithm with base 'e'. The presenter demonstrates how to use a calculator to find the value of Ln(9) and emphasizes the importance of understanding logarithmic properties for solving equations.

20:10

📉 Logarithmic Functions and Their Domain

The concept of logarithmic functions is introduced, noting their use in solving exponential equations. The presenter discusses the properties of logarithmic functions, emphasizing that the domain of the variable X must be positive, as the logarithm of a negative number is undefined. An example problem is solved to find the domain and graph of the function y = Ln(5x - 2), noting the vertical asymptote at X = 2/5.

25:15

🛒 Applications of Exponential Growth: Organic Food Market

The presenter applies exponential growth concepts to real-world data, estimating the amount Americans will spend on organic food and beverages in 2017 based on a continuous growth rate. The calculation is performed using the compound interest formula with a growth rate of 10.4% per year. The presenter also calculates when the spending will exceed fifty billion dollars and when it will double the amount spent in 2014, using the doubling time formula.

30:16

💰 Pricing Strategy for Trail Master Mountain Bikes

The final part of the video addresses a pricing strategy for a mountain bike, given a formula that relates the price in dollars per unit to the number of units sold in thousands. The presenter calculates the price per unit for 150,000 bikes and then solves for the number of units that consumers will buy at a price of $400. The calculations involve logarithmic functions, and the presenter emphasizes the importance of providing practical answers to business questions.

35:18

📈 Summary of Exponential and Logarithmic Concepts

The video concludes with a summary of the key concepts covered, including exponential functions, logarithms, and their applications. The presenter reminds viewers that while the material may be a review for some, it is essential for understanding upcoming calculus topics. The video provides a condensed version of what would typically be multiple lectures in a college algebra class, covering essential material on exponential and logarithmic functions.

Mindmap

Keywords

💡Exponential Function

An exponential function is a mathematical function where the variable appears in the exponent. It is defined by the form y = a^x, where 'a' is a positive constant and 'x' is the variable. In the video, the concept is introduced as a review from algebra classes and is central to the discussion of growth and decay models. For instance, y = 2^x is an example used in the script to illustrate the function's behavior.

💡Logarithmic Function

A logarithmic function is the inverse of an exponential function and is written as y = log_b(x), where 'b' is the base and 'x' is the argument. Logarithms are used to solve exponential equations and are explained in the video as a method to rewrite exponential statements in a different form. The script discusses how the logarithmic form can be converted into exponential form and vice versa, using the example log base 2 of 8 equals 3.

💡Euler's Constant (e)

Euler's constant, commonly denoted as 'e', is an important mathematical constant approximately equal to 2.71828. It is an irrational number that is the base for natural logarithms. In the video, 'e' is introduced as a base for exponential and logarithmic functions, and its significance in various natural phenomena is highlighted. The script approximates 'e' to two decimal places as 2.7 for computational simplicity.

💡Compound Interest

Compound interest is the interest on a loan or deposit calculated based on both the initial principal and the accumulated interest from previous periods. It is a key application of exponential functions discussed in the video. The formula A = P(1 + r/n)^(nt) is explained, where 'A' is the amount of money accumulated after n years, including interest. The video uses this formula to illustrate how the frequency of compounding affects the total amount accrued.

💡Continuous Compounding

Continuous compounding is a method of calculating compound interest where the number of compounding periods per year approaches infinity. It is represented by the formula A = Pe^(rt), where 'A' is the amount of money accumulated, 'P' is the principal amount, 'r' is the annual interest rate, and 't' is the time in years. The video demonstrates that continuous compounding results in a slightly higher amount compared to daily compounding over a period of 10 years.

💡Graphing Exponential Functions

The process of graphing exponential functions is demonstrated in the video through the plotting of points and the observation of the graph's general shape. The graph of an exponential function, such as y = 2^x, is characterized by a curve that starts close to the x-axis and increases rapidly. This shape is described as resembling a hockey stick or a banana, and it's a central part of the visual understanding of exponential growth.

💡Graphing Logarithmic Functions

Similar to exponential functions, logarithmic functions are also graphed in the video. The graph of a logarithmic function, such as y = log base 2 of x, has a characteristic 'S' shape or a vertical asymptote at x = 0, indicating that the function is not defined for negative values of x. The video emphasizes that logarithmic functions only yield positive values for y, regardless of the input x.

💡Domain of a Function

The domain of a function refers to the set of all possible input values (x-values) for which the function is defined. In the context of the video, when discussing the logarithmic function y = log base 5 of (x - 2), the domain is all real numbers greater than 2/5, as the logarithm is not defined for non-positive arguments. The domain is crucial for understanding the range of values over which a function's graph is valid.

💡Solving Exponential Equations

Solving exponential equations involves finding the value of the variable for which the equation holds true. In the video, this is demonstrated by using properties of logarithms to rewrite the equation in logarithmic form and then solving for the variable. An example given is the equation e^(2t) = 1000, which is solved by taking the natural logarithm of both sides to isolate the variable t.

💡Solving Logarithmic Equations

Solving logarithmic equations involves finding the value of the variable that, when plugged into the logarithm, satisfies the equation. The video demonstrates converting logarithmic equations into exponential form to solve for the variable. For example, the equation 3ln(x) = 5 is solved by dividing by 3 and then using the exponential form e^(5/3) to find the value of x.

💡Doubling Time

Doubling time is the period it takes for an investment or quantity to double in size. It is calculated using the formula T = ln(2) / K, where 'T' is the doubling time and 'K' is the growth rate. In the video, this concept is applied to determine when the amount spent on organic food and beverages will double, using the growth rate provided in the problem statement.

Highlights

Introduction to a new chapter focusing on algebraic review, specifically College Algebra and Intermediate Algebra.

Definition and explanation of exponential functions with variables in the exponent.

Demonstration of how to plot points for an exponential function, using y = 2^x as an example.

Description of the graph of an exponential function, often resembling a 'hockey stick' or 'banana' shape.

Introduction to Euler's constant (e), an irrational number approximately equal to 2.71828.

Explanation of how to use a calculator to work with the base e, including using the e button for calculations.

Application of exponential functions to model compound interest, including formulas for different compounding frequencies.

Use of a graphing calculator to solve for the amount accrued with continuous compounding.

Transition to logarithms as a method to solve exponential equations, with a review of logarithmic properties.

Illustration of converting between logarithmic and exponential forms of equations.

Discussion on the domain of logarithmic functions and why the logarithm of negative numbers is undefined.

Procedure for graphing logarithmic functions and their characteristic curves.

Solution of logarithmic equations by converting them into exponential form.

Application of logarithms to real-world problems, such as calculating the spending on organic food and beverages.

Use of the doubling time formula to estimate when a certain amount of money will double at a given growth rate.

Problem-solving approach for determining the price and quantity in a market scenario using logarithmic functions.

Emphasis on the importance of not rounding off intermediate steps when performing calculations to avoid errors.

Summary of key concepts covered in the lecture, including exponential and logarithmic functions, their properties, and applications.

Transcripts

Browse More Related Video

2.3 - Derivatives of Logarithmic Functions

Calculus 2 Lecture 6.3: Derivatives and Integrals of Exponential Functions

Business Calculus -- Math 1329 -- Section 4.2 -- Logarithmic Functions

Business Calculus - Math 1329 - Section 4.1 - Exponential Functions and Continuous Compounding

Math 11 - Section 2.2

Ch. 4.3 Logarithmic Functions

Related Tags

Exponential Functions Logarithmic Functions Algebra Review Finance Math Economics Continuous Growth Calculator Usage Compound Interest Euler's Constant Educational Content