Derivatives of Exponential Functions
TLDRThe video script offers an in-depth exploration into the derivatives of exponential functions, starting with a refresher on the chain rule, which is pivotal for differentiating compositions of functions. The chain rule is then applied to generalize the power rule, demonstrating how to handle expressions where the base or exponent is not a simple variable. The lesson delves into the specifics of exponential functions, highlighting that the derivative of an exponential function with a base other than e is the natural logarithm of the base times the original function. When the base is e, the derivative is identical to the original function, a concept illustrated through graphical analysis. The script also touches on the product rule when dealing with the product of an exponential function and a linear function, and the quotient rule for the division of such functions. The instructor connects these mathematical concepts to the concept of compound interest, revealing e as the limit of (1 + 1/n)^n as n approaches infinity, a direct link to the continuous compounding formula. The lesson is not only a mathematical exposition but also an attempt to bridge the gap between abstract calculus and real-world applications like finance.
Takeaways
- 📚 The Chain Rule states that the derivative of a composition of functions is the derivative of the outer function times the derivative of the inner function.
- 🔢 When applying the Power Rule to a function like y = (5x - 1)^3, you multiply by the exponent and then reduce the exponent by 1, also using the Chain Rule if necessary.
- 📈 The derivative of an exponential function y = a^x is the original function a^x multiplied by the natural log of the base 'a'.
- ⛓ If the exponent is not simply 'x' but a more complex expression like 3x or (2x - 1), you apply the Chain Rule by multiplying by the derivative of that expression.
- 🎓 The natural log of e (Euler's number) is equal to 1, which means the derivative of e^x is e^x itself.
- 🔧 When differentiating exponential functions with a base of 'e', you can generalize the rule by multiplying by the derivative of the exponent when it's not just 'x'.
- 🤝 The Product Rule for derivatives is used when you have a product of two functions, and it's expressed as (f(x) * g(x))' = f'(x) * g(x) + f(x) * g'(x).
- 🧩 Factoring is an optional step when simplifying derivatives, which can be helpful when terms share a common factor.
- 📉 The Quotient Rule is applied when differentiating a quotient of two functions, expressed as (f(x) / g(x))' = (f'(x) * g(x) - f(x) * g'(x)) / (g(x))^2.
- 📌 To find the slope of the tangent line at a specific point, substitute the x-value into the derivative of the function.
- 🏛 The mathematical constant e is defined as the limit of (1 + 1/n)^n as n approaches infinity, which is directly related to the concept of continuous compounding interest.
Q & A
What is the chain rule in calculus?
-The chain rule is used to find the derivative of a composition of functions. It states that the derivative of a function composed of two other functions is the derivative of the outer function times the derivative of the inner function.
How does the power rule apply when the base is not just x but a different function?
-When the base is not just x but a different function, like 5x - 1, you still apply the power rule by multiplying by the exponent and then reducing the exponent by 1. You then multiply by the derivative of the inner function (in this case, 5).
What is the derivative of an exponential function with a base greater than zero and not equal to one?
-The derivative of an exponential function is the original function multiplied by the natural logarithm of the base. For example, if y = a^x, then y' = a^x * ln(a).
How do you find the derivative of an exponential function when the exponent is not just x?
-When the exponent is not just x (for example, 3x or x^2), you apply the chain rule. You take the derivative of the outer function (the exponential part), and then multiply by the derivative of the inner function (the transformed x part).
What is Euler's number e?
-Euler's number e is an irrational number, approximately equal to 2.72, which cannot be written as a precise fraction. It is a natural base for exponential functions and has significant applications in areas such as compound interest.
Why is the derivative of e^x the same as the original function?
-The derivative of e^x is the same as the original function because the natural logarithm of e is equal to 1. Therefore, applying the derivative rule for exponential functions with base e results in e^x * ln(e) = e^x * 1 = e^x.
How does the concept of compound interest relate to the number e?
-The number e is derived from the formula for compound interest when compounded continuously. As the number of compounding periods per year (n) approaches infinity, the formula for compound interest converges to the value of e.
What is the product rule in calculus?
-The product rule is used to find the derivative of a product of two functions. It states that the derivative of a product is the derivative of the first function times the second function plus the first function times the derivative of the second function (f'g + fg').
What is the quotient rule in calculus?
-The quotient rule is used to find the derivative of a quotient of two functions. It states that the derivative of a quotient is the denominator times the derivative of the numerator minus the numerator times the derivative of the denominator, all divided by the square of the denominator (g*f' - f*g') / g^2.
How do you find the slope of the tangent line to a function at a specific point?
-To find the slope of the tangent line at a specific point, you substitute the x value of the point into the derivative of the function. The result is the slope of the tangent line at that point.
What is the significance of the limit definition of e in calculus?
-The limit definition of e is significant because it provides a precise mathematical definition of e as the limit of (1 + 1/n)^n as n approaches infinity. This definition is derived from the formula for continuous compound interest and is fundamental to various areas of calculus and mathematical analysis.
Outlines
📚 Introduction to Derivatives of Exponential Functions
The video begins with a recap of the chain rule from a previous lesson, which is essential for understanding the derivatives of exponential functions. The chain rule is used to find the derivative of a function composed of two others. It's then applied to generalize the power rule, explaining how to find the derivative of functions like x^3 and (5x - 1)^3. The concept of an exponential function with a variable exponent is introduced, and the base of these functions is shown to be any real number greater than zero, excluding one. The video emphasizes the importance of the chain rule in deriving exponential functions.
🔢 Derivative Rules for Exponential Functions
This paragraph delves into the specifics of finding derivatives of exponential functions. The rule for the derivative of an exponential function is presented: it is the original function multiplied by the natural log of the base. The chain rule is then used to generalize this for cases where the exponent is not simply x, such as 3x or x^3. Examples are given to illustrate the process, including the derivative of 5^x and (1/3)^x. The video also touches on the product rule when dealing with the product of an exponential function and a linear function, providing a step-by-step guide to differentiating such expressions.
🎓 Euler's Number e and Exponential Functions
The video introduces Euler's number e, an irrational number similar to Pi, and its significance in calculus and compound interest. The natural logarithm of e is shown to be equal to one, which leads to the derivative of e^x being e^x itself. This is a unique property of the exponential function with e as its base. The video also discusses the graphical representation of e^x and its derivative, showing how the slopes of the tangent lines to the curve increase in a manner that mirrors the original function.
📈 Applying the Chain Rule to e^x
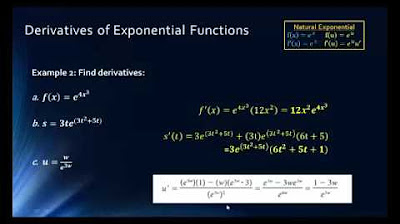
The paragraph explains how to find the derivative of e raised to the power of a variable expression, such as e^(2x) or e^(-3x^2). It emphasizes that when the exponent is not simply x, the chain rule must be applied, multiplying the derivative of the inner function by the derivative of e^x. The video provides examples and simplifies the expressions to show the final forms of the derivatives. It also discusses the possibility of factoring out common terms in the derivative for a cleaner expression.
🤔 Simplifying Derivatives and Factoring
The video continues with examples of differentiating exponential functions, including using the product rule for functions like (1 + 2x)e^(4x). It shows how to simplify the derivative by combining like terms and factoring out common factors. The instructor also mentions that while factoring is not always necessary, it can lead to a more preferred form of the answer. The video assures viewers that all presented forms of the answer are acceptable and encourages understanding over strict adherence to a single method.
🧮 Derivatives at Specific Points and e as a Limit
The final paragraph discusses finding the derivative of a function at a specific point, using the example of a function involving e^(x^3). It demonstrates how to plug in the value of x to find the slope at that point. The video then connects back to the concept of compound interest and provides a detailed explanation of e as a limit, showing how e emerges from the formula for compound interest compounded continuously. The instructor uses a table to illustrate how the value of e is approached as the number of compounding periods per year (n) increases towards infinity.
Mindmap
Keywords
💡Chain Rule
💡Power Rule
💡Exponential Function
💡Natural Logarithm
💡Product Rule
💡Quotient Rule
💡Euler's Number (e)
💡Limit Definition
💡Continuous Compound Interest
💡Derivative
💡Slope of a Tangent Line
Highlights
The Chain Rule is essential for differentiating compositions of functions, stating that the derivative of a composition is the derivative of the outer function times the derivative of the inner function.
The Power Rule can be generalized using the Chain Rule for functions like ( y = (5x - 1)^3 ) by multiplying by the derivative of the inner function (5 in this case).
Exponential functions have a variable exponent and a base greater than zero, excluding one, as one raised to any power equals one, which is not exponential.
The derivative of an exponential function is the original function multiplied by the natural log of the base, such as ( (e^x)' = e^x ln(e) ).
When the exponent is not simply ( x ), the Chain Rule is applied to the derivative of exponential functions, multiplying by the derivative of the exponent.
For exponential functions with base ( e ), the derivative is the function itself, as ( ln(e) = 1 ), resulting in ( (e^x)' = e^x ).
The natural log of ( e ) is equal to one, which is why the derivative of an exponential function with base ( e ) is the function itself.
The graphical representation of the derivative of ( e^x ) shows that the slope of the tangent line increases in the same manner as the original function, demonstrating their similarity.
The Product Rule is used when differentiating a product of two functions, combining it with the rules for exponential functions when necessary.
The Quotient Rule is applied when differentiating a quotient of two functions, which can be simplified by factoring out common terms.
The concept of continuous compounding in finance is related to the mathematical constant ( e ), which is the limit of ( (1 + 1/n)^n ) as ( n ) approaches infinity.
The value of ( e ) is approximately 2.72, and it is an irrational number with ties to various mathematical and financial applications.
The Chain Rule can be used to generalize the derivative of any function, including those with bases other than ( e ), by multiplying by the derivative of the variable within the function.
When differentiating exponential functions, it's important to recognize when the base is not ( e ) and adjust the derivative calculation accordingly.
The derivative of a function at a specific point can be found by substituting the value of ( x ) into the derivative, which gives the slope of the tangent line at that point.
The concept of limits is fundamental in calculus, as demonstrated by the definition of ( e ) and its relation to continuous compounding interest.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: